Word search on time words

The more words you encounter and understand, the broader your day-to-day vocabulary will become. Our word games and puzzles, such as this Time Word Search, are an excellent way to help to reinforce spellings in your mind.

Especially helpful are exercises that are focussed on a theme or topic as these provide word retention practice so you can be confident to read, write, speak and listen successfully. We have many activities to get your English up to speed in no time.

Cambridge English Examinations:

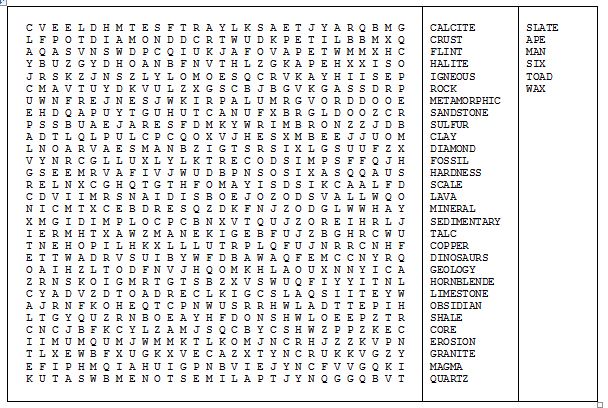
The word-search puzzle is a string-search problem in which several words are to be located within an n ´ m array filled with letters. The following table shows a 30 ´ 30 array and some words that must be found in it.

Each word must occur entirely in a row, a column or a diagonal, that is, it cannot be bent from one direction into another. Words can be placed forward or backward, but not scrambled. Furthermore, words can share characters. A brute-force approach is extremely inefficient: if the puzzle has n rows and m columns, and if the average length of the words to search for is q, then the time complexity would be nmq. For typical values of n = 30, m = 30, and q = 5, the time complexity would be 4500, which is much higher than 110.

If the fingerprints are the same (pFP == tFP), the actual match of the pattern against the target is attempted. A mismatching character causes the matching loop to be aborted, while a complete match makes the function to return with the position of the pattern in the target string. If the fingerprints are not equal, the fingerprint substring of the target being matched (tFP) is updated by considering the effect of “sliding” the pattern under the target one character position to the right. The beauty of the fingerprint approach is that words have the same fingerprint whether they are forward or backward in any direction.

The following function KRsearch, implements a variation on the “fingerprinting” method of Karp-Rabin (Sedgewick, Robert, Algorithms, 1990, pp. 289-291). Function fp is used to compute the initial fingerprint of the pattern (pFP) and of the corresponding prefix of the target (tFP). The fingerprint is simply the sum of the ASCII codes of the characters.

int KRsearch( char p[], char t[] )
{
   int i, j, k, m = strlen( p ), n = strlen( t ),
       pFP, tFP;
 
   pFP = fp( p, 0, m );   tFP = fp( t, 0, m );
 
   for ( i = 0; i <= n-m; ++i ) {
      if ( pFP == tFP ) {
         for ( j = 0, k = i; j < m; ++j, ++k )
            if ( p[ j ] != t[ k ] )
               break;
         if ( j == m )  return i;
      }
      else if ( i + m < n ) tFP += t[ i+m ] - t[ i ]; else break;
   }
   return -1;
}
 
int fp( char s[], int i, int m )
{
   int j, sum;
 
   sum = 0;
   for ( j = i; j < m; ++j )
      sum += s[ j ];
   return sum;
}

Assume that the puzzle is given in the text file wpuzzle.txt. The first line contains two numbers (n and m) separated by a blank space. Those numbers are the rows and columns of the puzzle and are followed by n lines of m characters each. Assume that the words to be found are given in the text file words.txt, one word per line. No assumptions should be made as to whether or not the words appear in the puzzle. The program must fill (and print) a solution array with the words in the position and direction in which they were found, as shown below for the example puzzle.

The global variables to be used and the main function are as follows.

#define maxN 50
#define maxM 50
 
char puzzle[ maxN ][ maxM ], 
     puzsol[ maxN ][ maxM ], 
       line[ maxM + 1 ];     
 int n,                      
     m,                      
     maxOFnm,                
     pFP;                    
 
void main()
{
   FILE *fp;
    int len;
 
   if ( LoadPuzzle() ) {
      ShowPuzzle();
      if ( (fp = fopen( "words.txt", "r" )) != NULL ) {
         while ( fgets( line, maxM + 1, fp ) != NULL ) {
            len = removeNL( line );
            FindWord( line, len );
         }
         fclose( fp );
         ShowSolution();
      }
   }
}

The trivial auxiliary functions are defined as follows.

int LoadPuzzle()
{
   FILE *fp;
    int ok = 0, i, j;
 
   if ( (fp = fopen( "wpuzzle.txt", "r" ))
        != NULL ) {
      fscanf( fp, "%d %dn", &n, &m );
      if (    0 < n && n <= maxN
           && 0 < m && m <= maxM ) {
         maxOFnm = n < m ? m: n;
         for ( i = 0; i < n; ++i ) {
            fgets( line, maxM+1, fp );
            removeNL( line );
            strcpy( &(puzzle[ i ][ 0 ]),
                    line );
         }
         fclose( fp );
         ok = 1;
      }
   }
   return ok;
}
 
int removeNL( char *str )
{
   int l = strlen( str ) - 1;
   str[ l ] = '';
   return l;
}
 
void ShowPuzzle()
{
   int i, j;
 
   printf( "Puzzle array: (%dx%d)n",
           n, m );
   for ( i = 0; i < n; ++i ) {
      for ( j = 0; j < m; ++j ) {
         printf( "%c ", puzzle[ i ][ j ] );
         puzsol[ i ][ j ] = ' ';
      }
      printf( "n" );
   }
}

void ShowSolution()
{
   int i, j;
 
   printf( "nWords found:n" );
   for ( i = 0; i < n; ++i ) {
      for ( j = 0; j < m; ++j )
         printf( "%c ",
                 puzsol[ i ][ j ] );
      printf( "n" );
   }
}

To find a word, conduct a search in the horizontal, vertical, and diagonal directions, stopping the search when the word is found in any direction. The diagonal directions involve a search either in the southeast (upper-left corner to lower-right corner) or in the southwest (upper-right corner to lower-left corner) directions.

void FindWord( char *w, int wLen )
{
   pFP = hvFP( w, 0, wLen, 1 );
   printf( "searching for '%s', fp = %d", w, pFP );
 
   if ( !( hKRsearch( w, wLen )         
           ||
           vKRsearch( w, wLen )         
           ||
           seKRsearch( w, wLen )        
           ||
           swKRsearch( w, wLen ) ) )    
      printf( " not" );
   printf( " foundn" );
}

The implementation of functions hKRsearch, vKRsearch, seKRsearch, and swKRsearch rely on adaptations of the basic KRsearch function to perform searches over a two-dimensional array. Function hvFP, to compute the fingerprint in the horizontal and vertical directions of the puzzle, is trivially used to obtain the fingerprint of a word as if it were in a horizontal direction.

Since the fingerprint of a word is the same whether the word occurs foward or backward in the puzzle, it is convenient to define a global structure in which the search functions can return the position of the array at which a word was found, and the word’s direction.

typedef enum { forw, back } FillDir; 
                                     
 
typedef struct { 
       int pos;  
   FillDir dir;  
} FIstr;

FIstr finfo;

The following figure shows typical searches in the vertical (column 3) and horizontal (row 1) directions.

The computation of the initial fingerprint of the target and the actual search in these directions can be done in the same way. To reach the “next” character in the horizontal direction an increment of 1 is needed, while for the vertical direction we an increment of maxM is needed. This is the third argument to functions hvFP (horizontal/vertical fingerprint) and hvKRsearch (horizontal/ vertical Karp-Rabin search) defined below.

int hvFP( char s[], int i, int len, int d )
{
   int j, sum;
 
   sum = 0;
   for ( j = i; j < len; j += d )
      sum += s[ j ];
   return sum;
}
 
int hvKRsearch( char *p, char *t, int pLen, int tLen, int d )
{
   int i, j, k, tFP, pMaxIdx = pLen*d, tMaxIdx = (tLen-pLen)*d;
 
   tFP = hvFP( t, 0, pMaxIdx, d );
 
   for ( i = 0; i <= tMaxIdx; i += d ) {
      if ( pFP == tFP ) {
         for ( j = 0, k = i; j < pLen; ++j, k += d ) 
            if ( p[ j ] != t[ k ] )
               break;
         if ( j == pLen ) {
            finfo.pos = i;  finfo.dir = forw;  return 1;
         }
         else {
            for ( j = pLen-1, k = i; j >= 0; --j, k +=d ) 
               if ( p[ j ] != t[ k ] )
                  break;
            if ( j == -1 ) {
               finfo.pos = i;  finfo.dir = back;  return 1;
            }
         }
      }
      else
         tFP += t[ i + pMaxIdx ] - t[ i ];
   }
   return 0;
}

Filling a word in the solution depends on whether it was found forward or backward in the vertical or horizontal direction. This is taken care of by function hvFillWord.

void hvFillWord( char *psol, char *w, int wLen, int d )
{
   int i, j = finfo.pos;
 
   if ( finfo.dir == forw )
      for ( i = 0; i < wLen; ++i ) {
         psol[ j ] = w[ i ];
         j += d;
      }
   else 
      for ( i = wLen-1; i >= 0; --i ) {
         psol[ j ] = w[ i ];
         j += d;
      }
}

Horizontal and vertical searches are implemented by the two functions below.

int hKRsearch( char *w, int wLen )
{
   int i;

   for ( i = 0; i < n; ++i )
      if ( hvKRsearch( w, &(puzzle[ i ][ 0 ]), wLen, m, 1 ) ) {
         hvFillWord( &(puzsol[ i ][ 0 ]), w, wLen, 1 );
         break;
      }
   return i < n;
}
 
int vKRsearch( char *w, int wLen )
{
   int j;
 
   for ( j = 0; j < m; ++j )
      if ( hvKRsearch( w, &(puzzle[ 0 ][ j ]), wLen, n, maxM ) ) {
         hvFillWord( &(puzsol[ 0 ][ j ]), w, wLen, maxM );
         break;
      }
   return j < m;
}

Searches along the diagonals are performed in a similar way. The following figure shows typical searches.

In the southeast direction, the increment must be maxM + 1 to reach the “next” character, while in the southwest direction, the increment must be maxM – 1. Because all functions use upper bounds for the indices, it is not a good idea to conduct searches in the north-east direction.

The functions to compute the initial fingerprint, and to search in the diagonal directions are defined below.

int dgFP( char s[], int i, int len, int d )
{
   int j, sum;
 
   sum = 0;
   for ( j = i; j < len; j += d + 1 )
      sum += s[ j ];
   return sum;
}
 
int dKRsearch( char *p, char *t, int pLen, int tLen, int d )
{
   int i, j, k, tFP,
       pMaxIdx = pLen*(d+1), tMaxIdx = (tLen-pLen)*(d+1);
 
   tFP = dgFP( t, 0, pMaxIdx, d );
 
   for ( i = 0; i <= tMaxIdx; i += d + 1 ) {
      if ( pFP == tFP ) {
         for ( j = 0, k = i; j < pLen; ++j, k += d + 1 ) 
            if ( p[ j ] != t[ k ] )
               break;
         if ( j == pLen ) {
            finfo.pos = i;
            finfo.dir = forw;
            return 1;
         }
         else {
            for ( j = pLen-1, k = i; j >= 0; --j, k +=d + 1 ) 
               if ( p[ j ] != t[ k ] )
                  break;
            if ( j == -1 ) {
               finfo.pos = i;
               finfo.dir = back;
               return 1;
            }
         }
      }
      else
         tFP += t[ i + pMaxIdx ] - t[ i ];
   }
   return 0;
}

The function to fill words in the diagonal directions is similar to the one to fill words in the horizontal and vertical directions.

void dgFillWord( char *psol, char *w, int wLen, int d )
{
   int i, j = finfo.pos;
 
   if ( finfo.dir == forw )
      for ( i = 0; i < wLen; ++i ) {
         psol[ j ] = w[ i ];
         j += d + 1;
      }
   else 
      for ( i = wLen-1; i >= 0; --i ) {
         psol[ j ] = w[ i ];
         j += d + 1;
      }
}

To find a word in the diagonals, start at the main diagonals, and search for as long as a diagonal contains at least as many characters as the word being sought.

int seKRsearch( char *w, int wLen )
{
   int i, tLen, found = 0;
 
   tLen = maxOFnm;
   for ( i = 0; wLen <= tLen; ++i ) {
      if ( dKRsearch( w, &(puzzle[ i ][ 0 ]), wLen, tLen, maxM ) ) {
         found = 1;
         dgFillWord( &(puzsol[ i ][ 0 ]), w, wLen, maxM );
         break;
      }
      --tLen;
   }
   if ( tLen < wLen ) {
      tLen = maxOFnm - 1;
      for ( i = 1; wLen <= tLen; ++i ) {
         if ( dKRsearch( w, &(puzzle[ 0 ][ i ]), wLen, tLen, maxM ) ) {
            found = 1;
            dgFillWord( &(puzsol[ 0 ][ i ]), w, wLen, maxM );
            break;
         }
         --tLen;
      }
   }
   return found;
}
 
int swKRsearch( char *w, int wLen )
{
   int i, tLen, found = 0;
 
   tLen = maxOFnm;
   for ( i = m-1; wLen <= tLen; --i ) {
      if ( dKRsearch( w, &(puzzle[ 0 ][ i ]), wLen, tLen, maxM-2 ) ) {
         found = 1;
         dgFillWord( &(puzsol[ 0 ][ i ]), w, wLen, maxM-2 );
         break;
      }
      --tLen;
   }
   if ( tLen < wLen ) {
      tLen = maxOFnm - 1;
      for ( i = 1; wLen <= tLen; ++i ) {
         if ( dKRsearch( w, &(puzzle[ i ][ m-1 ]), wLen, tLen, maxM-2 ) ) {
            found = 1;
            dgFillWord( &(puzsol[ i ][ m-1 ]), w, wLen, maxM-2 );
            break;
         }
         --tLen;
      }
   }
   return found;
}