10000+ results for ‘maths word questions’
maths questions
Quiz
by Anonymous
maths word wall
Find the match
by Anonymous
E3 Numeracy — Vocabulary Quiz
Quiz
by Lucieg1
Adult Education
ESL
Maths
Maths
Maths Questions match up
Match up
by Anonymous
Maths Questions
Match up
by Anonymous
Engage Task: Mental Maths Quiz (Mixed Topics)
Quiz
by Hstrickland1
KS2
Y4
Y5
Y6
Maths
Mental Maths
Maths Word Problems
Gameshow quiz
by Jeni4
Maths Word Jumble
Unjumble
by Hannahdeans
Maths year 5 questions
Random wheel
by Shahi221313
Ceistean Matamataig — Maths Questions
Maze chase
by Scott536
Maths Assessment
Quiz
by Intelligentlearning
Maths Assessment — Advanced
E3N Match the Number with the Name
Match up
by Lgegg
Adult Education
ESL
Maths
Maths
GCSE Maths Word Memory Challenge
Conveyor belt
by Mkelly10
Ice Breaker acitivity- maths word
Anagram
by Njussab
Number 1-10 (Maths questions)
Quiz
by Weidu78
Maths and ordering letters and numbers
Quiz
by Ninas2011
Y2
Y3
Y4
Y5
Y6
Y7
Y8
Y9
Y10
Y11
Y12
Y13
University
Maths
Thinking maths
maths wordsearch
Wordsearch
by Anonymous
Word order in questions
Missing word
by Leighanne
maths match-up
Match up
by Anonymous
maths
Quiz
by Jasbyr
Question word questions
Unjumble
by Dilingual
Adult Education
ESOL
Question words
Time Word problems
Quiz
by Mafaldagiudice
Maths
Word order in questions
Missing word
by U49266076
Present simple questions- word order
Unjumble
by Hgeloo
Adult Education
ESOL
Q3) N5 Maths Exam Type Questions
Open the box
by Missyoungmaths
2) N5 Maths Exam Type Questions
Open the box
by Missyoungmaths
1) N5 Maths Exam Type Questions
Open the box
by Missyoungmaths
Match up1 questions word Spanish Y9
Match up
by Christelle
KS3
Spanish
E1 Mental addition: reordering. Tips: add up large nos. first and/or look for pairs that add up to 10.
Match up
by Skillsworkshop
Entry Level 1 Functional Skills
Adult Education
Functional Skills Maths
Maths
E1.3 Add numbers which total up to 20
Maths
Anagram
by Tsdaniell8
Elementary School
High school
Technical education
Higher Education/University Education
KS1
KS2
KS3
Reception
Y1
Y2
Y3
Y4
Y5
Y6
Y7
Y8
maths
Crossword
by 19obayliss
Maths
Open the box
by Lpaget
maths anagram
Anagram
by Musicdept
maths quiz
Quiz
by 22strypkoy
Maths
Random wheel
by Fionaingham
Maths
Anagram
by 19mteixera
Maths
Anagram
by Dwhite10
Maths
Airplane
by Udege001209
Maths Quiz
Quiz
by Adewj029303
maths
Flip tiles
by Shahi221313
Maths
Random wheel
by Hannahhughes3
Maths
Open the box
by Libertyrosemay
Maths
Match up
by Sha21005793
maths quiz
Quiz
by 20shapter
Elementary School
Maths
Quiz
by Lshuck302
Maths
Open the box
by 20qejvanir
Maths Hangman
Hangman
by Seast
Maths
Quiz
by Keyuran
KS2
Y5
Y6
Maths
Rank order
by Ahamilton
Maths Quiz
Quiz
by Joannesutton
maths
Quiz
by Reads1
Maths quiz
Quiz
by 20eatont
Maths!
Quiz
by 20isaacsa
maths
Gameshow quiz
by 19hblay
Maths quiz
Quiz
by Musicdept
maths
Wordsearch
by U53733739
Elementary School
High school
Technical education
Higher Education/University Education
KS1
KS2
KS3
KS4
KS5
Reception
Y1
Y2
Y3
Y4
Y5
Y6
Y7
Y8
Y9
Y10
Y11
Y12
Y13
University
Adult Education
Workplace Training
maths
Airplane
by 19tcullimore
maths
Maze chase
by Mcramphorn
English
airplane maths
Airplane
by Izzydrazin
maths
Airplane
by 19eselkirk
This article is for parents who think about how to help with math and support their children. The math word problems below provide a gentle introduction to common math operations for schoolers of different grades.
What are math word problems?
During long-time education, kids face various hurdles that turn into real challenges. Parents shouldn’t leave their youngsters with their problems. They need an adult’s possible help, but what if the parents themselves aren’t good at mathematics? All’s not lost. You can provide your kid with different types of support. Not let a kid burn the midnight oil! Help him/ her to get over the challenges thanks to these captivating math word examples.
Math word problems are short math questions formulated into one or several sentences. They help schoolers to apply their knowledge to real-life scenarios. Besides, this kind of task helps kids to understand this subject better.
Addition for the first and second grades
These math examples are perfect for kids that just stepped into primary school. Here you find six easy math problems with answers:
1. Peter has eight apples. Dennis gives Peter three more. How many apples does Peter have in all?
Show answer
Answer: 8 apples + 3 apples = 11 apples.
2. Ann has seven candies. Lack gives her seven candies more. How many candies does Ann have in all?
Show answer
Answer: 7 candies + 7 candies = 14 candies.
3. Walter has two books. Matt has nine books. If Matt gives all his books to Walter, how many books will Walter have?
Show answer
Answer: 2 books + 9 books = 11 books.
4. There are three crayons on the table. Albert puts five more crayons on the table. How many crayons are on the table?
Show answer
Answer: 3 crayons + 5 crayons = 8 crayons.
5. Bill has nine oranges. His friend has one orange. If his friend gives his orange to Bill, how many oranges will Bill have?
Show answer
Answer: 9 oranges + 1 orange = 10 oranges.
6. Jassie has four leaves. Ben has two leaves. Ben gives her all his leaves. How many leaves does Jessie have in all?
Show answer
Answer: 4 leaves + 2 leaves = 6 leaves.
Subtraction for the first and second grades
1. There were three books in total at the book shop. A customer bought one book. How many books are left?
Show answer
Answer: 3 books – 1 book = 2 books.
2. There are five pizzas in total at the pizza shop. Andy bought one pizza. How many pizzas are left?
Show answer
Answer: 5 pizzas – 1 pizza = 4 pizzas.
3. Liza had eleven stickers. She gave one of her stickers to Sarah. How many stickers does Liza have?
Show answer
Answer: 11 stickers – 1 sticker = 10 stickers.
4. Adrianna had ten stones. But then she left two stones. How many stones does Adrianna have?
Show answer
Answer: 10 stones – 2 stones = 8 stones.
5. Mary bought a big bag of candy to share with her friends. There were 20 candies in the bag. Mary gave three candies to Marissa. She also gave three candies to Kayla. How many candies were left?
Show answer
Answer: 20 candies – 3 candies – 3 candies = 14 candies.
6. Betty had a pack of 25 pencil crayons. She gave five to her friend Theresa. She gave three to her friend Mary. How many pencil crayons does Betty have left?
Show answer
Answer: 25 crayons – 5 crayons – 3 crayons = 17 crayons.
Multiplication for the 2nd grade and 3rd grade
See the simple multiplication word problems. Make sure that the kid has a concrete understanding of the meaning of multiplication before.
Bill is having his friends over for the game night. He decided to prepare snacks and games.
1. He makes mini sandwiches. If he has five friends coming over and he made three sandwiches for each of them, how many sandwiches did he make?
Show answer
Answer: 5 x 3 = 15 sandwiches.
2. He also decided to get some juice from fresh oranges. If he used two oranges per glass of juice and made six glasses of juice, how many oranges did he use?
Show answer
Answer: 2 x 6 = 12 oranges.
3. Then Bill prepared the games for his five friends. If each game takes 7 minutes to prepare and he prepared a total of four games, how many minutes did it take for Bill to prepare all the games?
Show answer
Answer: 7 x 4 = 28 minutes.
4. Bill decided to have takeout food as well. If each friend and Bill eat three slices of pizza, how many slices of pizza do they have in total?
Show answer
Answer: 6 (5 friends and Bill) x 3 slices of pizza = 18 slices of pizza.
Mike is having a party at his house to celebrate his birthday. He invited some friends and family.
1. He and his mother prepared cupcakes for dessert. Each box had 8 cupcakes, and they prepared four boxes. How many cupcakes have they prepared in the total?
Show answer
Answer: 8 x 4 = 32 cupcakes.
2. They also baked some cookies. If they baked 6 pans of cookies, and there were 7 cookies per pan, how many cookies did they bake?
Show answer
Answer: 6 x 7 = 42 cookies.
3. Mike planned to serve some cold drinks as well. If they make 7 pitchers of drinks and each pitcher can fill 5 glasses, how many glasses of drinks are they preparing?
Show answer
Answer: 7 x 5 = 35 glasses.
4. At the end of the party, Mike wants to give away some souvenirs to his 6 closest friends. If he gives 2 souvenir items for each friend, how many souvenirs does Mike prepare?
Show answer
Answer: 6 x 2 = 12 souvenirs.
Division: best for 3rd and 4th grades
1. If you have 10 books split evenly into 2 bags, how many books are in each bag?
Show answer
Answer: 10 : 2 = 5 books.
2. You have 40 tickets for the fair. Each ride costs 2 tickets. How many rides can you go on?
3. The school has $20,000 to buy new equipment. If each piece of equipment costs $100, how many pieces can the school buy in total?
Show answer
Answer: $20,000 : $100= 200.
4. Melissa has 2 packs of tennis balls for $10 in total. How much does 1 pack of tennis balls cost?
5. Jack has 25 books. He has a bookshelf with 5 shelves on it. If Jack puts the same number of books on each shelf, how many books will be on each shelf?
6. Matt is having a picnic for his family. He has 36 cookies. There are 6 people in his family. If each person gets the same number of cookies, how many cookies will each person get?
Division with remainders for fourth and fifth grades
1. Sarah sold 35 boxes of cookies. How many cases of ten boxes, plus extra boxes does Sarah need to deliver?
Show answer
Answer: 35 boxes divided by 10 boxes per case = 3 cases and 5 boxes.
2. Candies come in packages of 16. Mat ate 46 candies. How many whole packages of candies did he eat, and how many candies did he leave? 46 candies divided by 16 candies = 2 packages and 2 candies left over.
3. Mary sold 24 boxes of chocolate biscuits. How many cases of ten boxes, plus extra boxes does she need to deliver?
Show answer
Answer: 24 boxes divided by 10 boxes per case = 2 cases and four boxes.
4. Gummy bears come in packages of 25. Suzie and Tom ate 30 gummy bears. How many whole packages did they eat? How many gummy bears did they leave?
Show answer
Answer: 30 divided by 25 = 1 package they have eaten and 20 gummy bears left over.
5. Darel sold 55 ice-creams. How many cases of ten boxes, plus extra boxes does he need to deliver?
Show answer
Answer: 55 boxes divided by 10 boxes per case = 5 cases and 5 boxes.
6. Crackers come in packages of 8. Mat ate 20 crackers. How many whole packages of crackers did he eat, and how many crackers did he leave?
Show answer
Answer: 20 divided by 8 = 2 packages eaten and 4 crackers are left.
Mixed operations for the fifth grade
These math word problems involve four basic operations: addition, multiplication, subtraction, and division. They suit best for the fifth-grade schoolers.
200 planes are taking off from the airport daily. During the Christmas holidays, the airport is busier — 240 planes are taking off every day from the airport.
1. During the Christmas holidays, how many planes take off from the airport in each hour if the airport opens 12 hours daily?
Show answer
Answer: 240÷12=20 planes take off from this airport each hour during the Christmas holidays.
2. Each plane takes 220 passengers. How many passengers depart from the airport every hour during the Christmas holidays? 20 x 220 = 4400.
Show answer
Answer: 4400 passengers depart from the airport every hour.
3. Compared with a normal day, how many more passengers are departing from the airport in a day during the Christmas holidays?
Show answer
Answer: (240-200) x 220 = 8800 more passengers departing from the airport in a day during the Christmas holidays.
4. During normal days on average 650 passengers are late for their plane daily. During the Christmas holidays, 1300 passengers are late for their plane. That’s why 14 planes couldn’t take off and are delayed. How many more passengers are late for their planes during Christmas week?
Show answer
Answer: 1300 – 650 = 650 more passengers are late for their planes each day during the Christmas holidays.
5. According to the administration’s study, an additional 5 minutes of delay in the overall operation of the airport is caused for every 27 passengers that are late for their flights. What is the delay in the overall operation if there are 732 passengers late for their flights?
Show answer
Answer: 732 ÷ 27 × 5 = 136. There will be a delay of 136 minutes in the overall operation of the airport.
Extra info math problems for the fifth grade
1. Ann has 7 pairs of red socks and 8 pairs of pink socks. Her sister has 12 pairs of white socks. How many pairs of socks does Ann have?
2. Kurt spent 17 minutes doing home tasks. He took a 3-minute snack break. Then he studied for 10 more minutes. How long did Kurt study altogether?
Show answer
Answer: 17 + 10 = 27 minutes.
3. There were 15 spelling words on the test. The first schooler spelled 9 words correctly. Miguel spelled 8 words correctly. How many words did Miguel spell incorrectly?
4. In the morning, Jack gave his friend 2 gummies. His friend ate 1 of them. Later Jack gave his friend 7 more gummies. How many gummies did Jack give his friend in all?
5. Peter wants to buy 2 candy bars. They cost 8 cents, and the gum costs 5 cents. How much will Peter pay?
Finding averages for 5th grade
We need to find averages in many situations in everyday life.
1. The dog slept 8 hours on Monday, 10 hours on Tuesday, and 900 minutes on Wednesday. What was the
average number of hours the dog slept per day?
Show answer
Answer: (8+10+(900:60)) : 3 = 11 hours.
2. Jakarta can get a lot of rain in the rainy season. The rainfall during 6 days was 90 mm, 74 mm, 112 mm, 30 mm, 100 mm, and 44 mm. What was the average daily rainfall during this period?
Show answer
Answer: (90+74+112+30+100+44) : 6 = 75 mm.
3. Mary bought 4 books. The prices of the first 3 books were $30, $15, and $18. The average price she paid for the 4 books was $25 per books. How much did she pay for the 4th books?

Math & logic courses for kids
Times more complex than school, extremely fun, interactive and rewarding to keep 7-13 years old kids engaged. We’re gonna make them love math!
learn more
Ordering and number sense for the 5th grade
1. There are 135 pencils, 200 pens, 167 crayons, and 555 books in the bookshop. How would you write these numbers in ascending order?
Show answer
Answer: 135, 167, 200, 555
2. There are five carrots, one cabbage, eleven eggs, and 15 apples in the fridge. How would you write these numbers in descending order?
3. Peter has completed exercises on pages 279, 256, 264, 259, and 192. How would you write these numbers in ascending order?
Show answer
Answer: 192, 256, 259, 264, 279.
4. Mary picked 32 pants, 15 dresses, 26 pairs of socks, 10 purses. Put all these numbers in order.
5. The family bought 12 cans of tuna, 23 potatoes, 11 onions, and 33 pears. Put all these numbers in order.
Fractions for the 6th-8th grades
1. Jannet cooked 12 lemon biscuits for her daughter, Jill. She ate up 4 biscuits. What fraction of lemon biscuits did Jill eat?
Show answer
Answer: 1/3 of the lemon biscuits.
2. Guinet travels a distance of 7 miles to reach her school. The bus covers only 5 miles. Then she has to walk 2 miles to reach the school. What fraction of the distance does Guinet travel by bus?
Show answer
Answer: 5/7 of the distance
3. Bob has 24 pencils in a box. Eighteen pencils have #2 marked on them, and the 6 are marked #3. What fraction of pencils are marked #3?
Show answer
Answer: 1/4 of the pencils.
4. My mother places 15 tulips in a glass vase. It holds 6 yellow tulips and 9 red tulips. What fraction of tulips are red?
Show answer
Answer: 3/5 of the tulips.
5. Bill owns 14 pairs of socks, of which 7 pairs are white, and the rest are brown. What fraction of pairs of socks are brown?
Show answer
Answer: 1/2 of the pairs of socks.
6. Bred spotted a total of 39 birds in an aviary at the Zoo. He counted 18 macaws and 21 cockatoos. What fraction of macaws did Bred spot at the aviary?
Show answer
Answer: 6/13 of the birds.
Decimals for the 6th grade
Write in words the following decimals:
- 0,004
- 0,07
- 2,1
- 0,725
- 46,36
- 2000,19
Show answer
Answer:
- 0,004 = four thousandths.
- 0,07 = seven hundredths.
- 2,1 = two and one tenth.
- 0,725 = seven hundred twenty five thousandths.
- 46,36 = foury six and thirty six hundredths.
- 2000,19 = two thousand and nineteen hundredths.
Comparing and sequencing for the 6th grade
1. The older brother picked 42 apples at the orchard. The younger brother picked only 22 apples. How many more apples did the older brother pick?
Show answer
Answer: 42 – 22 = 20 apples more.
2. There were 16 oranges in a basket and 66 oranges in a barrel. How many fewer oranges were in the basket than were in the barrel?
Show answer
Answer: 66 – 16 = 50 fewer oranges.
3. There were 40 parrots in the flock. Some of them flew away. Then there were 25 parrots in the flock. How many parrots flew away?
Show answer
Answer: 40 – 25 = 15 parrots flew away.
4. One hundred fifty is how much greater than fifty-three?
5. On Monday, the temperature was 13°C. The next day, the temperature dropped by 8 degrees. What was the temperature on Tuesday?
6. Zoie picked 15 dandelions. Her sister picked 22 ones. How many more dandelions did her sister pick than Zoie?
Show answer
Answer: 22-15 = 7 dandelions more.
Time for the 4th grade
1. The bus was scheduled to arrive at 7:10 p.m. However, it was delayed for 45 minutes. What time was it when the bus arrived?
2. My mother starts her 7-hour work at 9:15 a.m. What time does she get off from work?
3. Jack’s walk started at 6:45 p.m. and ended at 7:25 p.m. How long did his walk last?
4. The school closes at 9:00 p.m. Today, the school’s principal left 15 minutes after the office closed, and his secretary left the office 25 minutes after he left. When did the secretary leave work?
5. Suzie arrives at school at 8:20 a.m. How much time does she need to wait before the school opens? The school opens at 8:35 a.m.
6. The class starts at 9:15 a.m.. The first bell will ring 20 minutes before the class starts. When will the first bell ring?
Money word problems for the fourth grade
1. James had $20. He bought a chocolate bar for $2.30 and a coffee cup for $5.50. How much money did he have left?
Show answer
Answer: $20.00 – $2.30 – $5.50 = $12.20. James had $12.20 left.
2. Coffee mugs cost $1.50 each. How much do 7 coffee mugs cost?
Show answer
Answer: $1.5 x 7 = $10.5.
3. The father gives $32 to his four children to share equally. How much will each of his children get?
4. Each donut costs $1.20. How much do 6 donuts cost?
Show answer
Answer: $1.20 * 6 = $7,2.
5. Bill and Bob went out for takeout food. They bought 4 hamburgers for $10. Fries cost $2 each. How much does one hamburger with fries cost?
Show answer
Answer: $10 ÷ 4 = $2.50. One hamburger costs $2.50. $2.50 + $2.00 = $4.50. One hamburger with fries costs $4.50.
6. A bottle of juice costs $2.80, and a can is $1.50. What would it cost to buy two cans of soft drinks and a bottle of juice?
Show answer
Answer: $1.50 x 2 + $2.80 = $5.80.
Measurement word problems for the 6th grade
The task is to convert the given measures to new units. It best suits the sixth-grade schoolers.
- 55 yd = ____ in.
- 43 ft = ____ yd.
- 31 in = ____ ft.
- 29 ft = ____ in.
- 72 in = ____ ft.
- 13 ft = ____ yd.
- 54 lb = ____ t.
- 26 t = ____ lb.
- 77 t = ____ lb.
- 98 lb = ____ t.
- 25 lb = ____ t.
- 30 t = ____ lb.
Show answer
Answer:
- 55 yd = 1.980 in
- 43 ft = 14 yd 1 ft
- 31 in = 2 ft 7 in
- 29 ft = 348 in
- 72 in = 6 ft
- 13 ft = 4 yd 1 ft.
- 54 lb = 0,027 t
- 26 t = 52.000 lb
- 77 t = 154.000 lb
- 98 lb = 0,049 t
- 25 lb = 0?0125 t
- 30 t = 60.000 lb.
Ratios and percentages for the 6th-8th grades
It is another area that children can find quite difficult. Let’s look at simple examples of how to find percentages and ratios.
1. A chess club has 25 members, of which 13 are males, and the rest are females. What is the ratio of males to all club members?
2. A group has 8 boys and 24 girls. What is the ratio of girls to all children?
3. A pattern has 4 red triangles for every 12 yellow triangles. What is the ratio of red triangles to all triangles?
4. An English club has 21 members, of which 13 are males, and the rest are females. What is the ratio of females to all club members?
5. Dan drew 1 heart, 1 star, and 26 circles. What is the ratio of circles to hearts?
6. Percentages of whole numbers:
- 50% of 60 = …
- 100% of 70 = …
- 90% of 70 = …
- 20% of 30 = …
- 40% of 10 = …
- 70% of 60 = …
- 100% of 20 = …
- 80% of 90 = …
Show answer
Answer:
- 50% of 60 = 30
- 100% of 70 = 70
- 90% of 70 = 63
- 20% of 30 = 6
- 40% of 10 = 4
- 70% of 60 = 42
- 100% of 20 = 20
- 80% of 90 = 72.
Probability and data relationships for the 8th grade
1. John ‘s probability of winning the game is 60%. What is the probability of John not winning the game?
2. The probability that it will rain is 70%. What is the probability that it won’t rain?
3. There is a pack of 13 cards with numbers from 1 to 13. What is the probability of picking a number 9 from the pack?
4. A bag had 4 red toy cars, 6 white cars, and 7 blue cars. When a car is picked from this bag, what is the probability of it being red or blue?
5. In a class, 22 students like orange juice, and 18 students like milk. What is the probability that a schooler likes juice?
Geometry for the 7th grade
The following task is to write out equations and find the angles. Complementary angles are two angles that sum up to 90 degrees, and supplementary angles are two angles that sum up to 180 degrees.
1. The complement of a 32° angle = …
2. The supplement of a 10° angle = …
3. The complement of a 12° angle = …
4. The supplement of a 104° angle = …
Variables/ equation word problems for the 5th grades
1. The park is 𝑥 miles away from Jack’s home. Jack had to drive to and from the beach with a total distance of 36 miles. How many miles is Jack’s home away from the park?
Show answer
Answer: 2𝑥 = 36 → 𝑥 = 18 miles.
2. Larry bought some biscuits which cost $24. He paid $x and got back $6 of change. Find x.
Show answer
Answer: x = 24 + 6 = $30.
3. Mike played with his children on the beach for 90 minutes. After they played for x minutes, he had to remind them that they would be leaving in 15 minutes. Find x.
Show answer
Answer: x = 90 – 15 = 75 minutes.
4. At 8 a.m., there were x people at the orchard. Later at noon, 27 of the people left the orchard, and there were 30 people left in the orchard. Find x.
Show answer
Answer: x = 30 + 27 = 57 people
Travel time word problems for the 5th-7th grades
1. Tony sprinted 22 miles at 4 miles per hour. How long did Tony sprint?
Show answer
Answer: 22 miles divided by 4 miles per hour = 5.5 hours.
2. Danny walked 15 miles at 3 miles per hour. How long did Danny walk?
Show answer
Answer: 15 miles divided by 3 miles per hour = 5 hours.
3. Roy sprinted 30 miles at 6 miles per hour. How long did Roy sprint?
Show answer
Answer: 30 miles divided by 6 miles per hour = 5 hours.
4. Harry wandered 5 hours to get Pam’s house. It is 20 miles from his house to hers. How fast did Harry go?
Show answer
Answer: 20 miles divided by 5 hours = 4 miles per hour.
STEM subjects for kids
STEM courses for kids ages 7-13 in physics, chemistry, math and logic in interactive game format
learn more
There has been an absolute boatload of changes to the mathematics curriculum over the last few years. Ignore what your parents tell you, exams are not getting easier. In fact, they’re getting significantly harder. While some of the increase in difficulty does involve more difficult questions being moved down to the foundation paper and even some A-Level topics being introduced earlier, a lot of it is from “contextual questions” being used a lot more.
Contextual questions sound terrible, they sound like what you have to do when you write essays in history. Take sources and put them into context. This is maths, not history why should we care about putting things into context? I know it sounds stupid, but context is actually really important in maths. What’s the point in knowing how to work out the percentage of something if you never know how to actually use it? Or why Pythagoras is important if you want to be an architect? That’s what contextual questions help us to do.
That’s just a big word though, what it really means is wordy questions. Loads of writing that doesn’t really make much sense. It is easy to get it to make sense though. Not just is it easy to get it to make sense, but it’s easy to pick up some of the marks even you still struggle to get the final answer.
Take this question for example.
The first thing to do when you see this question is to stop screaming. It will get your thrown out of the exam hall and no-one wants that. The second thing to do is calmly read through it and look for maths words in the question. The maths words will give you an idea of what working out you need to do in the question. If you can work out where the maths words are, what they mean and how to use them IN CONTEXT to the question.
If you look through the question you will see at least one of those words in there standing right out at you. Here it is again.
I’ve made it red for you. The difference is the maths word. The difference means takeaway. So the first step for this question is for you to take away the first reading from the second reading. That means the sum you will have to work out is 2159 – 1962 giving us an answer of 197.
If I write down 2159 – 1962 = 197 on my exam paper I would get 2 marks. 2 marks out of 4 just for doing that. Notice how so far we haven’t really explained what the question is asking? We still don’t really know what it wants. All we have done is looked for maths words. A keyword if you like, and we have done what it told us.
This is an incredibly important part of making sure that you gain the most possible marks on your exam paper. If you see a contextual question and don’t understand it, search the question for maths words and then carry out what they tell you to do. Even though so far I haven’t got a clue what this question wants me to do, I’ve still managed to pick up half of the marks just by finding a maths word. It might sound simple and like I’m tricking you, but I’m really not. The maths paper isn’t trying to trick you, it’s just trying to find out if you know how to do what it tells you.
So to the second part of the question. This part is a little more tricky, but not too much. We already know that the number of units of gas used was 197. We worked that out, the question said: “The difference in meter readings gives the number of units of gas used.” So using our incredible brains we have worked out that 197 units of gas were used. What next?
ESSENTIAL INFO 🤔 🖐️
The cost of gas is 21p for each unit of gas used.
Work out the cost of gas used.
Give your answer in pounds (£).
I was going to put the important parts in red, but for the last part of the question, most would have been in red really. Let’s break it down though, what we already know and what it is asking us to do with it. We know we have 197 units used. We know that each unit costs 21p. They want to know the total cost of gas. They want that total in pounds. Okay, so your first 2 marks were picked up with relative ease. They’re making you work for those final 2.
First things first, the two mentions of money in the question are in different forms. Pence and pounds. We need to convert one to the other. How do we decide which one to change? That’s easy. We know they want the answer in pounds. That means that we are going to convert the pence value into pounds.
There are a number of ways to do this. The easiest way is to just remember how many pence are in 1 pound. It’s 100 right? So all you do is divide how many pence you have by 100 and that gives you that pence value in pounds. We have 21 pence as the cost of gas per unit, so our sum is 21 ➗100 which gives us the answer 0.21. Now we’ve converted our pence value into pounds we can get on with finding out the final answer. We’re on the final stretch for this now.
So the final part that the question asked for was the total price. What information do we have for this last bit? We know that 197 units of gas were used. We know that each unit costs £0.21. All we have to do is multiply the two numbers together. 197 X £0.21 gives us £41.37. That is your final 2 marks. We’ve picked up 4 marks, and none of that was anything complicated at all. All we had to do was find out the important parts the question told us and then do what it told us.
Let’s go through quickly the important parts of how we found that answer. First, we looked for maths words. Then we did what the maths word told us to do. Then we used what we found out to find out the final answer. It was as simple as that.
Let’s try another question.
Here is a diagram of Jim’s garden.
JIM’S GARDEN 🤔 🖐️
Jim wants to cover his garden with grass seed to make a lawn.
Grass seed is sold in bags.
There is enough grass seed in each bag to cover 20m2 of garden.
Each bag of grass seed costs £4.99
Work out the least cost of putting grass seed on Jim’s garden.
This is slightly different from the first question we tried, the maths in this question is slightly more hidden. It’s not in there as words, it is still telling you what to do though. Look through the question. Is there anything in the first line? Nope. All it is telling you is what the diagram represents. The diagram itself doesn’t tell us much of us, yet!
Look through the rest of the question before you check the next part. See if you can spot the maths.
JIM’S GARDEN 🤔 🖐️
Jim wants to cover his garden with grass seed to make a lawn.
Grass seed is sold in bags.
There is enough grass seed in each bag to cover 20m2 of garden.
Each bag of grass seed costs £4.99
Work out the least cost of putting grass seed on Jim’s garden.
Did you spot it? The maths this time wasn’t in words, but it was in the numbers. Look at the part highlighted in red. What is that telling us? M2 means metres squared. What are metres squared an example of? An area.
With that information coupled with the diagram also shown at the start, we need to think about what we’re going to do. They have told us that each bag of grass covers a certain area. They have given us a shape, which represents the garden, maybe if we work out the area of that shape it will tell us the area we need to cover? Let’s try it.
This is awkward, the shape isn’t that easy to work out. There are two ways to work out the area of this shape. The first way is to treat it as a compound shape. In case you didn’t already know, to work out the area of a compound shape we split it into parts and from there we work out each part then add up the area of each part to give us the total area. Simple, right? Let’s try splitting it up first and go from there.
See how we have split the shape into two separate shapes? We have a rectangle that is 9m by 12m. We also have a triangle that is 9m high, but we don’t know the length of the base. To work that out we need to look at the length of the two parallel sides that are perpendicular to the red line. In simple words, the two sides opposite each other that go in the opposite direction to the red line. Notice how the base of the triangle starts where the 12m line ends. That means that the extra distance on the line at the bottom is what makes up the base of the triangle. As the bottom line is 18m and the top line is 12m, we now know that the base of the triangle is 6m, because 18 takeaway 12 is 6.
From here we can work out the area of the two separate shapes. First the rectangle. The rectangle has sides of 12m and 9m. So (12 times 9=108 mathrm{~m}^{2}). The triangle has a base of 6m and a height of 9m. So 6 × 9 = 54, then 54 ÷ 2 = 27. So the area of the triangle is (27 mathrm{~m}^{2}) Adding the two areas together gives us (108+27=135 mathrm{~m}^{2}).
The second way to work out the area is to recognise that the shape is a trapezium. To work out the area of a trapezium we add together the top and bottom lengths and then divide the total by 2. So that is 18 + 12 = 30. Then we do 30 ÷ 2 = 15. Once we have done this we multiply it by the height of the trapezium. So we have (15 times 9=135 mathrm{~m}^{2}).
Notice the two areas both equal the same? It doesn’t matter which method you choose to work out the area. Both are perfectly acceptable because both give you exactly the same answer.
Now, from here we have picked up 3 out of the 5 marks available. Again, without even really understanding what the question is asking. We just looked for the maths in it and did the logical thing. Of course, we want the full 5 marks so we’re going to go back and find out how to get them.
JIM’S GARDEN 🤔 🖐️
Jim wants to cover his garden with grass seed to make a lawn.
Grass seed is sold in bags.
There is enough grass seed in each bag to cover 20m2 of garden.
Each bag of grass seed costs £4.99
Work out the least cost of putting grass seed on Jim’s garden.
All of the parts highlighted in red are the important parts, as well as the total area of the garden being (135 mathrm{~m}^{2}) which we worked out earlier. We know that each bag of grass seed covers (20 mathrm{~m}^{2}) so there are two ways to work out how many bags of seed we need. First, we could divide the total area needed by the area covered by each bag. So that would be 135 ÷ 20 which gives us 6.75. We can’t buy 0.75 of a bag of seed though, so we would need 7 bags. The other way would be to count up in the 20s until we hit the first number higher than 135. That goes 20, 40, 60, 80, 100, 120, 140, and altogether that is 7 lots of 20.
So now we know how many bags of seed we need. We’re hitting the final stretch for those 5 marks now. Each bag costs £4.99. We need 7 bags. So we do £4.99 × 7 which gives us £34.93. There we picked up 5 marks, without really having to worry about what the question was asking. All we had to do was look for the maths in the question and then just do what came naturally.
Does that mean we shouldn’t bother reading the question and just skim it for the important parts? The answer to that is of course not. Always read the question, but you should be looking for the maths while you do it. If you are struggling to understand what the question is asking then don’t panic and leave the question, just remember what we did. Look for the maths. Once you have found it, do whatever makes sense with it. For both of those questions, we picked up half marks without even reading the question.
Never panic and skip ahead when you see a worded question, as long as you find the maths in the question you will always pick up some of the marks.
Solving word problems at KS1 and KS2 is an essential part of the new maths curriculum. Here you can find expert guidance on how to solve maths word problems as well as examples of the many different types of word problems primary school children will encounter with links to hundreds more.
What is a word problem?
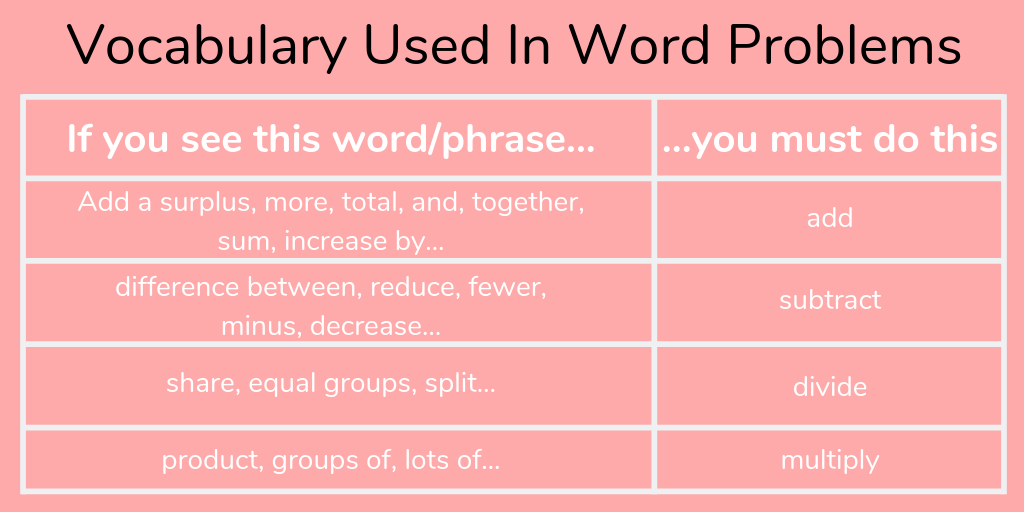
A word problem in maths is a maths question written as one sentence or more that requires children to apply their maths knowledge to a ‘real-life’ scenario.
This means that children must be familiar with the vocabulary associated with the mathematical symbols they are used to, in order to make sense of the word problem.
For example:
Importance of word problems within the national curriculum
The National Curriculum states that its mathematics curriculum “aims to ensure that all pupils:
- become fluent in the fundamentals of mathematics, including through varied and frequent practice with increasingly complex problems over time, so that pupils develop conceptual understanding and the ability to recall and apply knowledge rapidly and accurately;
- reason mathematically by following a line of enquiry, conjecturing relationships and generalisations, and developing an argument, justification or proof using mathematical language;
- can solve problems by applying their mathematics to a variety of routine and non-routine problems with increasing sophistication, including breaking down problems into a series of simpler steps and persevering in seeking solutions.”
To support this schools are adopting a ‘mastery’ approach to maths
The National Centre for Excellence in the Teaching of Mathematics (NCETM) have defined “teaching for mastery”, with some aspects of this definition being:
- Maths teaching for mastery rejects the idea that a large proportion of people ‘just can’t do maths’.
- All pupils are encouraged by the belief that by working hard at maths they can succeed.
- Procedural fluency and conceptual understanding are developed in tandem because each supports the development of the other.
- Significant time is spent developing deep knowledge of the key ideas that are needed to underpin future learning. The structure and connections within the mathematics are emphasised, so that pupils develop deep learning that can be sustained.
(The Essence of Maths Teaching for Mastery, 2016)

Year 3 to 6 Rapid Reasoning Worksheet for Weeks 1-6
Download for FREE 6 weeks of Rapid Reasoning worksheets. That include six weeks of daily reasoning and problem-solving questions for years 3, 4, 5 and 6!
Mastery helps children to explore maths in greater depth
One of NCETM’s Five Big Ideas in Teaching for Mastery (2017) is “Mathematical Thinking: if taught ideas are to be understood deeply, they must not merely be passively received but must be worked on by the student: thought about, reasoned with and discussed with others”.
In other words – yes, fluency in arithmetic is important; however, with this often lies the common misconception that once a child has learnt the number skills appropriate to their level/age, they should be progressed to the next level/age of number skills.
The mastery approach encourages exploring the breadth and depth of these concepts (once fluency is secure) through reasoning and problem solving.
See the following example:
| Year 6 objective | Fluency | Reasoning | Problem solving |
|---|---|---|---|
| Solve addition and subtraction multi-step problems in contexts, deciding which operations and methods to use and why. | 7,208 females attended a concert as well as 8,963 males. There were originally 20,000 seats on sale. How many empty seats were there at the concert? | Abdul says, “If I add any two 4-digit numbers together, it will make a 5-digit number.” Do you agree? Explain why. | Three pandas are eating bamboo sticks. There are 51 altogether. They all eat an odd number of sticks. How many bamboo sticks did they each eat? How many different ways can you do it? |
What sort of word problems might my child encounter at school?
In Key Stage 2, there are nine ‘strands’ of maths – these are then further split into ‘sub-strands’. For example, ‘number and place value’ is the first strand: a Year 3 sub-strand of this is to “find 10 or 100 more or less than a given number”; a Year 6 sub-strand of this is to “determine the value of each digit in numbers up to 10 million”. The table below shows how the ‘sub-strands’ are distributed across each strand and year group in KS2.
| Strand | Year 3 | Year 4 | Year 5 | Year 6 | Total |
|---|---|---|---|---|---|
| Number and place value | 6 | 9 | 7 | 5 | 27 |
| Calculations | 7 | 8 | 15 | 9 | 39 |
| Fractions, decimals and percentages | 7 | 10 | 12 | 11 | 40 |
| Ratio and proportion | 0 | 0 | 0 | 4 | 4 |
| Algebra | 0 | 0 | 0 | 5 | 5 |
| Measurement | 17 | 9 | 10 | 8 | 44 |
| Geometry: properties of shape | 5 | 4 | 6 | 7 | 22 |
| Geometry: position and direction | 0 | 3 | 1 | 2 | 6 |
| Statistics | 2 | 2 | 2 | 2 | 8 |
How to teach children to solve word problems?
Here are two simple strategies that can be applied to many word problems before solving them.
- What do you already know?
- How can this problem be drawn/represented pictorially?
Let’s see how this can be applied to a word problem to help achieve the answer.
Solving a simple word problem
There are 28 pupils in a class.
The teacher has 8 litres of orange juice.
She pours 225 millilitres of orange juice for every pupil.
How much orange juice is left over?
1. What do you already know?
- There are 1,000ml in 1 litre
- Pours = liquid leaving the bottle = subtraction
- For every = multiply
- Left over = requires subtraction at some point
2. How can this problem be drawn/represented pictorially?
The bar model is always a brilliant way of representing problems, but if you are not familiar with this, there are always other ways of drawing it out.
Read more: What is a bar model
For example, for this question, you could draw 28 pupils (or stick man x 28) with ‘225 ml’ above each one and then a half-empty bottle with ‘8 litres’ marked at the top.
Now to put the maths to work. This is a Year 6 multi-step problem, so we need to use what we already know and what we’ve drawn to break down the steps.
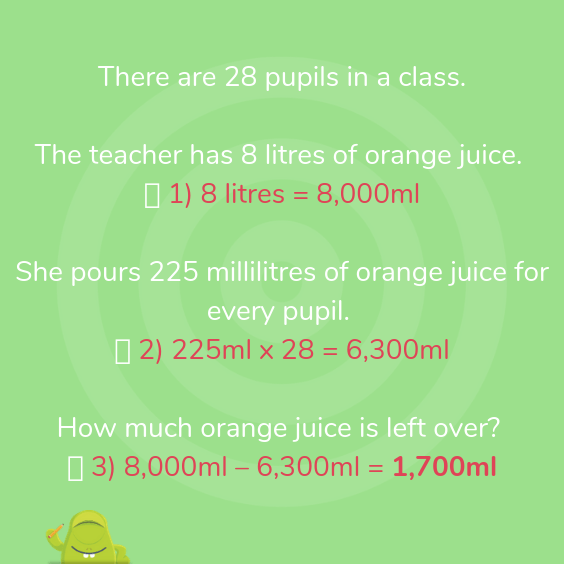
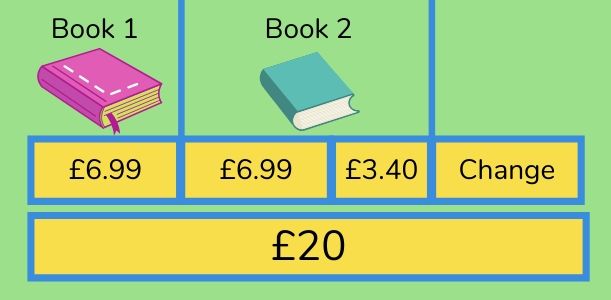
Solving a more complex word problem
Mara is in a bookshop.
She buys one book for £6.99 and another that costs £3.40 more than the first book.
She pays using a £20 notes.
What change does Mara get?
1. What do you already know?
- More than = add
- Using decimals means I will have to line up the decimal points correctly in calculations
- Change from money = subtract
2. How can this problem be drawn/represented pictorially?
See this example of bar modelling for this question:
Now to put the maths to work using what we already know and what we’ve drawn to break down the steps.
Mara is in a bookshop.
She buys one book for £6.99 and another that costs £3.40 more than the first book. 1) £6.99 + (£6.99 + £3.40) = £17.38
She pays using a £20 note.
What change does Mara get? 2) £20 – £17.38 = £2.62
Maths word problems for years 1 to 6
The more children learn about maths as the go through primary school, the trickier the word problems they face will become.
Below you will find some information about the types of word problems your child will be coming up against on a year by year basis, and how word problems apply to each primary year group
Word problems in Year 1
Throughout Year 1 a child is likely to be introduced to word problems with the help of concrete resources (pieces of physical apparatus like coins, cards, counters or number lines) to help them understand the problem.
An example of a word problem for Year 1 would be:
Chris is going to buy a cake for his mum which costs 80p. How many 20p coins would he need to do this?
Word problems in Year 2
Year 2 is a continuation of Year 1 when it comes to word problems, with children still using concrete maths resources to help them understand and visualise the problems they are working on
An example of a word problem for Year 2 would be:
A class of 10 children each have 5 pencils in their pencil cases. How many pencils are there in total?
Word problems in Year 3
With word problems for year 3, children will move away from using concrete resources when solving word problems, and move towards using written methods. Teachers will begin to demonstrate the four operations such as addition and subtraction word problems, multiplication and division problems too.
This is also the year in which 2-step word problems will be introduced. This is a problem which requires two individual calculations to be completed.
Year 3 word problem: Geometry properties of shape
Shaun is making 3-D shapes out of plastic straws.
At the vertices where the straws meet, he uses blobs of modelling clay to fix them together
Here are some of the shapes he makes:
| Shape | Number of straws | Number of blobs of modelling clay |
|---|---|---|
| A | 8 | 5 |
| B | 12 | 8 |
| C | 6 | 4 |
One of Sean’s shapes is a cuboid. Which is it? Explain your answer.
Answer: shape B as a cuboid has 12 edges (straws) and 8 vertices (clay)
Year 3 word problem: Statistics
Year 3 are collecting pebbles. This pictogram shows the different numbers of pebbles each group finds.
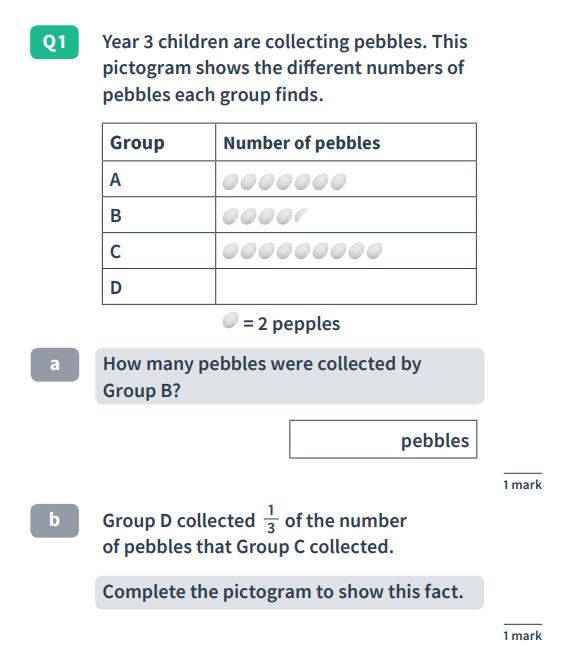
Answer: a) 9 b) 3 pebbles drawn
Top tip
By the time children are in Year 3 many of the word problems, even one-step story problems tend to be a variation on a multiplication problem. For this reason learning times tables becomes increasingly essential at this stage. One of the best things you can do to help with Year 3 maths at home is support your child to do this.
Word problems in Year 4
At this stage of their primary school career, children should feel confident using the written method for each of the four operations.
Word problems for year 4 will include a variety of problems, including 2-step problems and be children will be expected to work out the appropriate method required to solve each one.
Year 4 word problem: Number and place value
My number has four digits and has a 7 in the hundreds place.
The digit which has the highest value in my number is 2.
The digit which has the lowest value in my number is 6.
My number has 3 fewer tens than hundreds.
What is my number?
Answer: 2,746
Word problems in Year 5
One and 2-step word problems continue with word problems for year 5, but this is also the year that children will be introduced to word problems containing decimals.
These are some examples of Year 5 maths word problems.
Year 5 word problem: Fractions, decimals and percentages
Stan, Frank and Norm are washing their cars outside their houses.
Stan has washed 0.5 of his car.
Frank has washed 1/5 of his car.
Norm has washed 5% of his car.
Who has washed the most?
Explain your answer.
Answer: Stan (he has washed 0.5 whereas Frank has only washed 0.2 and Norm 0.05)
Word problems in Year 6
Word problems for year 6 shift from 2-step word problems to multi-step word problems. These will include fractions, decimals, percentages and time word problems.
Here are some examples of the types of maths word problems Year 6 will have to solve.
Year 6 word problem – Ratio and proportion
This question is from the 2018 key stage 2 SATs paper. It is worth 1 mark.
The Angel of the North is a large statue in England. It is 20 metres tall and 54 metres wide.
Ally makes a scale model of the Angel of the North. Her model is 40 centimetres tall. How wide is her model?
Answer: 108cm
Year 6 word problem – Algebra
This question is from the 2018 KS2 SATs paper. It is worth 2 marks as there are 2 parts to the answer.
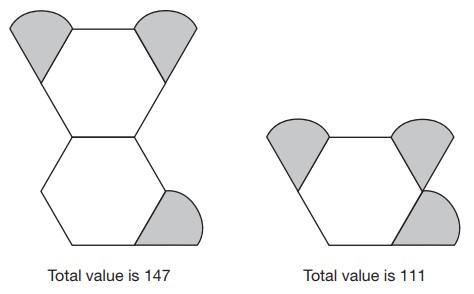
Amina is making designs with two different shapes.
She gives each shape a value.
Calculate the value of each shape.
Answer: 36 (hexagon) and 25.
Year 6 word problem: Measurement
This question is from the 2018 KS2 SATs paper. It is worth 3 marks as it is a multi-step problem.
There are 28 pupils in a class.
The teacher has 8 litres of orange juice.
She pours 225 millilitres of orange juice for every pupil.
How much orange juice is left over?
Answer: 1.7 litres or 1,700ml
Topic based word problems
The following examples give you an idea of the kinds of maths word problems your child will encounter for each of the 9 strands of maths in KS2.
Place value word problems
Place value word problem Year 5
This machine subtracts one hundredth each time the button is pressed. The starting number is 8.43. What number will the machine show if the button is pressed six times? Answer: 8.37
Download free number and place value word problems for Years 3, 4, 5 and 6
Addition and subtraction word problems
Addition and subtraction word problem Year 3
In Year 3 pupils will solve addition word problems and subtraction word problems with 2 and 3 digits.
Sam has 364 sweets. He gets given 142 more. He then gives 277 away. How many sweets is he left with? Answer: 229
Download free addition and subtraction word problems for Years 3, 4, 5 and 6
Addition word problem Year 3
Alfie thinks of a number. He subtracts 70. His new number is 12. What was the number Alfie thought of? Answer: 82
Subtraction word problem Year 6
The temperature at 7pm was 4oC. By midnight, it had dropped by 9 degrees. What was the temperature at midnight? Answer: -5oC
More here: 25 addition and subtraction word problems
Multiplication and division word problems
Multiplication and division word problem Year 3
A baker is baking chocolate cupcakes. She melts 16 chocolate buttons to make the icing for 9 cakes. How many chocolate buttons will she need to melt to make the icing for 18 cakes? Answer: 32
Multiplication word problem Year 4
Eggs are sold in boxes of 12. The egg boxes are delivered to stores in crates. Each crate holds 9 boxes. How many eggs are in a crate? Answer: 108
Download free multiplication word problems for Years 3, 4, 5 and 6
Division word problem Year 6
A factory produces 1,692 paintbrushes every day. They are packaged into boxes of 9. How many boxes does the factory produce every day? Answer: 188
Download our free division word problems worksheets for Years 3, 4, 5 and 6.
More here: 20 multiplication word problems
More here: 25 division word problems
Free resource: Use these four operations word problems to practise addition, subtraction, multiplication and division all together.
Fraction word problems
Fraction word problem Year 5
At the end of every day, a chocolate factory has 1 and 2/6 boxes of chocolates left over. How many boxes of chocolates are left over by the end of a week? Answer: 9 and 2/6 or 9 and 1/3
Download free fractions and decimals word problems worksheets for Years 3, 4, 5 and 6
More here: 28 fraction word problems
Decimals word problem Year 4 (crossover with subtraction)
Which two decimals that have a difference of 0.5? 0.2, 0.25, 0.4, 0.45, 0.6, 0.75. Answer: 0.25 and 0.75
Download free decimals and percentages word problems resources for Years 3, 4, 5 and 6
Percentage word problem Year 5
There are 350 children in a school. 50% are boys. How many boys are there? Answer: 175
Measurement word problem Year 3 (crossover with subtraction)
Lucy and Ffion both have bottles of strawberry smoothie. Each bottle contains 1 litre. Lucy drinks ½ of her bottle. Ffion drinks 300ml of her bottle. How much does each person have left in both bottles? Answer: Lucy = 500ml, Ffion = 300ml
More here: 25 percentage word problems
Money word problem Year 3
James and Lauren have different amounts of money. James has twelve 2p coins. Lauren has seven 5p coins. Who has the most money and by how much? Answer: Lauren by 11p.
More here: 25 money word problems
Area word problem Year 4
A rectangle measures 6cm by 5cm.
What is its area? Answer: 30cm2
Perimeter word problem Year 4
The swimming pool at the Sunshine Inn hotel is 20m long and 7m wide. Mary swims around the edge of the pool twice. How many metres has she swum? Answer: 108m
Ratio word problem Year 6 (crossover with measurement)
A local council has spent the day painting double yellow lines. They use 1 pot of yellow paint for every 100m of road they paint. How many pots of paint will they need to paint a 2km stretch of road? Answer: 20 pots
More here: 24 ratio word problems
Bodmas word problem Year 6
Draw a pair of brackets in one of these calculations so that they make two different answers. What are the answers?
50 – 10 × 5 =
50 – 10 × 5 =
Volume word problem Year 6
This large cuboid has been made by stacking shipping containers on a boat. Each individual shipping container has a length of 6m, a width of 4m and a height of 3m. What is the volume of the large cuboid? Answer: 864m3
How important are word problems when it comes to the SATs?
In the KS1 SATs, 58% (35/60 marks) of the test is comprised of maths ‘reasoning’ (word problems).
In KS2, this increases to 64% (70/110 marks) spread over two reasoning papers, each worth 35 marks. Considering children have, in the past, needed approximately 55-60% to reach the ‘expected standard’, it’s clear that children need regular exposure to and a solid understanding of how to solve a variety of word problems.
Children have the opportunity to practice SATs style word problems in Third Space Learning’s online one-to-one SATs revision programme. Personalised to meet the needs of each student, our programme helps to fill gaps and give students more confidence going in to the SATs exams.
Remember: The word problems can change but the maths won’t
It can be easy for children to get overwhelmed when they first come across word problems in KS2, but it is important that you remind them that whilst the context of the problem may be presented in a different way, the maths behind it remains the same.
Word problems are a good way to bring maths into the real world and make maths more relevant for your child, so help them practise, or even ask them to turn the tables and make up some word problems for you to solve.
This article while written by a teacher for teachers is also suitable for those at home supporting children with home learning. More free home learning resources are also available.
Do you have pupils who need extra support in maths?
Every week Third Space Learning’s maths specialist tutors support thousands of pupils across hundreds of schools with weekly online 1-to-1 lessons and maths interventions designed to plug gaps and boost progress.
Since 2013 we’ve helped over 145,000 primary and secondary school pupils become more confident, able mathematicians. Learn more or request a personalised quote for your school to speak to us about your school’s needs and how we can help.
Grade 6 maths word problems with answers are presented. Some of these problems are challenging and need more time to solve. Also detailed solutions and full explanations are included.
- Two numbers N and 16 have LCM = 48 and GCF = 8. Find N.
- If the area of a circle is 81pi square feet, find its circumference.
- Find the greatest common factor of 24, 40 and 60.
-
In a given school, there are 240 boys and 260 girls.
a) What is the ratio of the number of girls to the number of boys?
b) What is the ratio of the number of boys to the total number of pupils in the school? - If Tim had lunch at $50.50 and he gave 20% tip, how much did he spend?
-
Find k if 64 � k = 4. - Little John had $8.50. He spent $1.25 on sweets and gave to his two friends $1.20 each. How much money was left?
- What is x if x + 2y = 10 and y = 3?
- A telephone company charges initially $0.50 and then $0.11 for every minute. Write an expression that gives the cost of a call that lasts N minutes.
- A car gets 40 kilometers per gallon of gasoline. How many gallons of gasoline would the car need to travel 180 kilometers?
- A machine fills 150 bottles of water every 8 minutes. How many minutes it takes this machine to fill 675 bottles?
- A car travels at a speed of 65 miles per hour. How far will it travel in 5 hours?
- A small square of side 2x is cut from the corner of a rectangle with a width of 10 centimeters and length of 20 centimeters. Write an expression in terms of x for the area of the remaining shape.
- A rectangle A with length 10 centimeters and width 5 centimeters is similar to another rectangle B whose length is 30 centimeters. Find the area of rectangle B.
- A school has 10 classes with the same number of students in each class. One day, the weather was bad and many students were absent. 5 classes were half full, 3 classes were 3/4 full and 2 classes were 1/8 empty. A total of 70 students were absent. How many students are in this school when no students are absent?
-
A large square is made of 16 congruent squares. What is the total number of squares of different sizes are there?.
- The perimeter of square A is 3 times the perimeter of square B. What is the ratio of the area of square A to the area of square B.
- John gave half of his stamps to Jim. Jim gave gave half of his stamps to Carla. Carla gave 1/4 of the stamps given to her to Thomas and kept the remaining 12. How many stamps did John start with?
- Two balls A and B rotate along a circular track. Ball A makes 4 full rotations in 120 seconds. Ball B makes 3 full rotation in 60 seconds. If they start rotating now from the same point, when will they be at the same starting point again?
-
A segment is 3 units long. It is divided into 9 parts. What fraction of a unit are 2 parts of the segment? -
Mary wants to make a box. She starts with a piece of cardboard whose length is 15 centimeters and width is 10 centimeters. Then she cuts 4 congruent squares with sides of 3 centimeters at the four corners and folded at the broken lines to make the box. What is the volume of the box?
. - A car is traveling 75 kilometers per hour. How many meters does the car travel in one minute?
- Carla is 5 years old and Jim is 13 years younger than Peter. One year ago, Peter’s age was twice the sum of Carla’s & Jim’s age. Find the present age of each one of them.
- Linda spent 3/4 of her savings on furniture. She then spent 1/2 of her remaining savings on a fridge. If the fridge cost her $150, what were her original savings?
- The distance bewteen Harry and Kate is 2500 meters. Kate and Harry start walking toward one another and Kate’ dog start running back and forth between Harry and Kate at a speed of 120 meters per minute. Harry walks at the speed of 40 meters per minute while Kate walks at the speed of 60 meters per minute. What distsnce will the dog have travelled when Harry and Kate meet each other?
Answers to the Above Questions
- 24
- 18 Pi feet
- 4
- a) 13:12 b)12:25
- $60.60
- 16
- 4.85
- 4
- 0.50 + N * 0.11
- 4.5 gallons
- 36 minutes
- 325 miles
- 200 — 4x2
- 450 centimeters squared
- 200 pupils
- 30
- 9:1
- 64 stamps
- 60 seconds
- 2/3
- 108 cubic centimeters
- 1250 meters/minute
- Carla:5 years, Jim: 6 years, Peter: 19 years.
- $1200
- 3000 meters
More Middle School Maths (Grades 6, 7, 8, 9) — Free Questions and Problems With Answers
More High School Maths (Grades 10, 11 and 12) — Free Questions and Problems With Answers
More Primary Maths (Grades 4 and 5) with Free Questions and Problems With Answers
Home Page