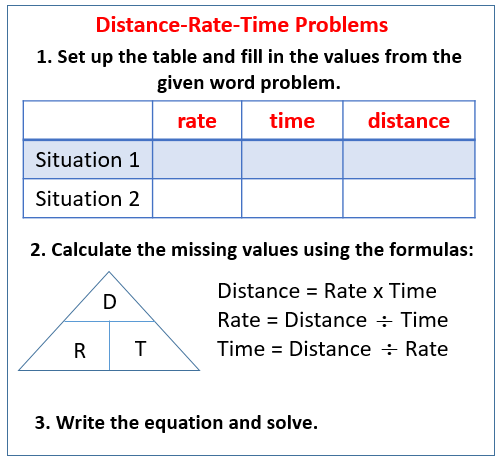
Distance problems are word problems that involve the distance
an object will travel at a certain average rate for a given
period of time.
The formula for distance problems is: distance =
rate × time or
d = r × t.
Things to watch out for:
Make sure that you change the units when necessary. For example, if the rate is given in miles per
hour and the time is given in minutes then change the units appropriately.
It would be helpful to use a table to organize the information for distance problems. A table helps
you to think about one number at a time instead being confused by the question.
The following diagrams give the steps to solve Rate Time Distance Word Problems. Scroll down
the page for examples and solutions.
Distance Problems: Traveling In Opposite Directions
Example:
A bus and a car leave the same place and traveled in opposite directions. If the bus is
traveling at 50 mph and the car is traveling at 55 mph, in how many hours will they be 210 miles apart?
Solution:
Step 1: Set up a rtd table.
| r | t | d | |
|---|---|---|---|
| bus | |||
| car |
Step 2: Fill in the table with information given in the question.
If the bus is traveling at 50 mph and the car is traveling
at 55 mph, in how many hours will they be 210 miles apart?
Let t = time when they are 210 miles apart.
| r | t | d | |
|---|---|---|---|
| bus | 50 | t | |
| car | 55 | t |
Step 3: Fill in the values for d using the formula d = rt
| r | t | d | |
|---|---|---|---|
| bus | 50 | t | 50t |
| car | 55 | t | 55t |
Step 4: Since the total distance is 210, we get the equation:
50t + 55t = 210
105t = 210
Isolate variable t
Answer: They will be 210 miles apart in 2 hours.
Example of a distance word problem with vehicles moving in opposite directions
In this video, you will learn to solve introductory distance or motion word problems — for example,
cars traveling in opposite directions, bikers traveling toward each other, or one plane overtaking
another. You should first draw a diagram to represent the relationship between the distances
involved in the problem, then set up a chart based on the formula rate times time = distance.
The chart is then used to set up the equation.
Example:
Two cars leave from the same place at the same time and travel in opposite directions. One car
travels at 55 mph and the other at 75 mph. After how many hours will they be 520 miles apart?
- Show Video Lesson
Rate-Time-Distance Problem
Solve this word problem using uniform motion rt = d formula:
Example:
Two cyclists start at the same corner and ride in opposite directions. One cyclist rides twice as
fast as the other. In 3 hours, they are 81 miles apart. Find the rate of each cyclist.
- Show Video Lesson
Distance — Opposite Directions
Example:
Brian and Jennifer both leave the convention at the same time traveling in opposite directions.
Brian drove at 35 mph and Jennifer drove at 50 mph. After how much time were they 340 miles apart?
- Show Video Lesson
Distance — Opposite Directions find t
Example:
Two joggers start from opposite ends of an 8 mile course running towards each other. One
jogger is running at a rate of 4 mph. The other is running at a rate of 6 mph. After how
long will the joggers meet?
-
Show Video Lesson
Distance — Opposite Directions find r
Example:
Bob and Fred start from the same point and walk in opposite directions. Bob walks 2 mph faster
than Fred. After 3 hours they are 30 miles apart. How fast does each walk?
- Show Video Lesson
GMAT Challenge Question: Distance/Rate/Time
Example:
Trains A and B left stations R and S simultaneously on two separate parallel rail tracks that
are 350 miles long. The trains pass each other at point X after traveling for a certain amount
of time. How many miles of the rail tracks has train A traveled when the two trains passed each other?
- Up to point X, the average speed of train B was 25% less than the average speed of train A.
- Up to point X, the average speed of train B was 60 mph and it took two and a half hours for
train B to arrive at point X.
- Show Video Lesson
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.
To solve rate word problems, knowledge of solving systems of equations is necessary. Rate word problems include problems dealing with rates, distances, time and wind or water current. Other types of word problems using systems of equations include money word problems and age word problems.
Alright guys some of the most scary Math problems that you see in your Algebra classes have to do with rates, distances and time. It’s like things traveling and they come in word problems which makes them scary to begin with and then also a lot of times people joke about these problems you know you see like the train leave Pittsburgh and the other train leaves New York and what time is it in Hawaii or whatever. They all use like really, they look like they’re scary tricky problems that have to do with how fast things are traveling and for how long.
We’re going to be looking at those types of problems and they’re really scary but you guys the thing you want to remember is this one formula. Distance equals rate times time some people will call this the dirt formula like distance equals dert it’s not kind of funny, not really mass joke. So this is the thing you want to keep in mind, you can also rearrange this like sometimes you wanted the rate all by itself you could dived both sides by time and sometimes you might see this formula written like this it’s the same thing just rewritten. This is the one most important formula you’re going to be using for these problems.
The other thing you’ll have to keep in mind when you see these problems, is what’s constant for both of the moving things and what’s different. Like maybe they both move the same amount of time. Or maybe they both travel the same distance but different speeds and times that they’re traveling, things like that. Keep in mind what’s the same for both of your 2 different moving items.
The other thing that you want to think about is a lot of times you see problems that have to do with air speed or current so if you have a plane that’s traveling, I have a little plane I want to show you. You have a little plane that’s flying through the air with the wind right? The wind is going to make it go faster, instead of going like this if the wind is pushing it it’s going to go woosh woosh a lot faster. And your formula for rate is going to involve rate plus wind speed, as opposed to if this airplane’s flying into the wind and your rate quantity here you’re going to be using how fast the plane is going take away that wind speed. The wind is going to slow it down, here it comes plane hits the wind slower that’s going to affect the rate in this problem. So again guys when you’re doing these problems this is the formula you’re going to use if it involves air speed or water currents speed it’s going to have like a change in your r quantity here. But the most important thing is to keep in mind what’s the same for both of your moving airplanes or moving boats or whatever.
Is the category for this document correct?
- Math
Thank you for your participation!
No more boring flashcards learning!
Learn languages, math, history, economics, chemistry and more with free Studylib Extension!
- Distribute all flashcards reviewing into small sessions
- Get inspired with a daily photo
- Import sets from Anki, Quizlet, etc
- Add Active Recall to your learning and get higher grades!
Add to Chrome
It’s free
Chapter 8: Rational Expressions
8.8 Rate Word Problems: Speed, Distance and Time
Distance, rate and time problems are a standard application of linear equations. When solving these problems, use the relationship rate (speed or velocity) times time equals distance.
[latex]rcdot t=d[/latex]
For example, suppose a person were to travel 30 km/h for 4 h. To find the total distance, multiply rate times time or (30km/h)(4h) = 120 km.
The problems to be solved here will have a few more steps than described above. So to keep the information in the problem organized, use a table. An example of the basic structure of the table is below:
| Who or What | Rate | Time | Distance |
|---|---|---|---|
The third column, distance, will always be filled in by multiplying the rate and time columns together. If given a total distance of both persons or trips, put this information in the distance column. Now use this table to set up and solve the following examples.
Joey and Natasha start from the same point and walk in opposite directions. Joey walks 2 km/h faster than Natasha. After 3 hours, they are 30 kilometres apart. How fast did each walk?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Natasha | [latex]r[/latex] | [latex]text{3 h}[/latex] | [latex]text{3 h}(r)[/latex] |
| Joey | [latex]r + 2[/latex] | [latex]text{3 h}[/latex] | [latex]text{3 h}(r + 2)[/latex] |
The distance travelled by both is 30 km. Therefore, the equation to be solved is:
[latex]begin{array}{rrrrrrl} 3r&+&3(r&+&2)&=&30 \ 3r&+&3r&+&6&=&30 \ &&&-&6&&-6 \ hline &&&&dfrac{6r}{6}&=&dfrac{24}{6} \ \ &&&&r&=&4 text{ km/h} end{array}[/latex]
This means that Natasha walks at 4 km/h and Joey walks at 6 km/h.
Nick and Chloe left their campsite by canoe and paddled downstream at an average speed of 12 km/h. They turned around and paddled back upstream at an average rate of 4 km/h. The total trip took 1 hour. After how much time did the campers turn around downstream?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Downstream | [latex]text{12 km/h}[/latex] | [latex]t[/latex] | [latex]text{12 km/h } (t)[/latex] |
| Upstream | [latex]text{4 km/h}[/latex] | [latex](1 — t)[/latex] | [latex]text{4 km/h } (1 — t)[/latex] |
The distance travelled downstream is the same distance that they travelled upstream. Therefore, the equation to be solved is:
[latex]begin{array}{rrlll} 12(t)&=&4(1&-&t) \ 12t&=&4&-&4t \ +4t&&&+&4t \ hline dfrac{16t}{16}&=&dfrac{4}{16}&& \ \ t&=&0.25&& end{array}[/latex]
This means the campers paddled downstream for 0.25 h and spent 0.75 h paddling back.
Terry leaves his house riding a bike at 20 km/h. Sally leaves 6 h later on a scooter to catch up with him travelling at 80 km/h. How long will it take her to catch up with him?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Terry | [latex]text{20 km/h}[/latex] | [latex]t[/latex] | [latex]text{20 km/h }(t)[/latex] |
| Sally | [latex]text{80 km/h}[/latex] | [latex](t — text{6 h})[/latex] | [latex]text{80 km/h }(t — text {6 h})[/latex] |
The distance travelled by both is the same. Therefore, the equation to be solved is:
[latex]begin{array}{rrrrr} 20(t)&=&80(t&-&6) \ 20t&=&80t&-&480 \ -80t&&-80t&& \ hline dfrac{-60t}{-60}&=&dfrac{-480}{-60}&& \ \ t&=&8&& end{array}[/latex]
This means that Terry travels for 8 h and Sally only needs 2 h to catch up to him.
On a 130-kilometre trip, a car travelled at an average speed of 55 km/h and then reduced its speed to 40 km/h for the remainder of the trip. The trip took 2.5 h. For how long did the car travel 40 km/h?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Fifty-five | [latex]text{55 km/h}[/latex] | [latex]t[/latex] | [latex]text{55 km/h }(t)[/latex] |
| Forty | [latex]text{40 km/h}[/latex] | [latex](text{2.5 h}-t)[/latex] | [latex]text{40 km/h }(text{2.5 h}-t)[/latex] |
The distance travelled by both is 30 km. Therefore, the equation to be solved is:
[latex]begin{array}{rrrrrrr} 55(t)&+&40(2.5&-&t)&=&130 \ 55t&+&100&-&40t&=&130 \ &-&100&&&&-100 \ hline &&&&dfrac{15t}{15}&=&dfrac{30}{15} \ \ &&&&t&=&2 end{array}[/latex]
This means that the time spent travelling at 40 km/h was 0.5 h.
Distance, time and rate problems have a few variations that mix the unknowns between distance, rate and time. They generally involve solving a problem that uses the combined distance travelled to equal some distance or a problem in which the distances travelled by both parties is the same. These distance, rate and time problems will be revisited later on in this textbook where quadratic solutions are required to solve them.
Questions
For Questions 1 to 8, find the equations needed to solve the problems. Do not solve.
- A is 60 kilometres from B. An automobile at A starts for B at the rate of 20 km/h at the same time that an automobile at B starts for A at the rate of 25 km/h. How long will it be before the automobiles meet?
- Two automobiles are 276 kilometres apart and start to travel toward each other at the same time. They travel at rates differing by 5 km/h. If they meet after 6 h, find the rate of each.
- Two trains starting at the same station head in opposite directions. They travel at the rates of 25 and 40 km/h, respectively. If they start at the same time, how soon will they be 195 kilometres apart?
- Two bike messengers, Jerry and Susan, ride in opposite directions. If Jerry rides at the rate of 20 km/h, at what rate must Susan ride if they are 150 kilometres apart in 5 hours?
- A passenger and a freight train start toward each other at the same time from two points 300 kilometres apart. If the rate of the passenger train exceeds the rate of the freight train by 15 km/h, and they meet after 4 hours, what must the rate of each be?
- Two automobiles started travelling in opposite directions at the same time from the same point. Their rates were 25 and 35 km/h, respectively. After how many hours were they 180 kilometres apart?
- A man having ten hours at his disposal made an excursion by bike, riding out at the rate of 10 km/h and returning on foot at the rate of 3 km/h. Find the distance he rode.
- A man walks at the rate of 4 km/h. How far can he walk into the country and ride back on a trolley that travels at the rate of 20 km/h, if he must be back home 3 hours from the time he started?
Solve Questions 9 to 22.
- A boy rides away from home in an automobile at the rate of 28 km/h and walks back at the rate of 4 km/h. The round trip requires 2 hours. How far does he ride?
- A motorboat leaves a harbour and travels at an average speed of 15 km/h toward an island. The average speed on the return trip was 10 km/h. How far was the island from the harbour if the trip took a total of 5 hours?
- A family drove to a resort at an average speed of 30 km/h and later returned over the same road at an average speed of 50 km/h. Find the distance to the resort if the total driving time was 8 hours.
- As part of his flight training, a student pilot was required to fly to an airport and then return. The average speed to the airport was 90 km/h, and the average speed returning was 120 km/h. Find the distance between the two airports if the total flying time was 7 hours.
- Sam starts travelling at 4 km/h from a campsite 2 hours ahead of Sue, who travels 6 km/h in the same direction. How many hours will it take for Sue to catch up to Sam?
- A man travels 5 km/h. After travelling for 6 hours, another man starts at the same place as the first man did, following at the rate of 8 km/h. When will the second man overtake the first?
- A motorboat leaves a harbour and travels at an average speed of 8 km/h toward a small island. Two hours later, a cabin cruiser leaves the same harbour and travels at an average speed of 16 km/h toward the same island. How many hours after the cabin cruiser leaves will it be alongside the motorboat?
- A long distance runner started on a course, running at an average speed of 6 km/h. One hour later, a second runner began the same course at an average speed of 8 km/h. How long after the second runner started will they overtake the first runner?
- Two men are travelling in opposite directions at the rate of 20 and 30 km/h at the same time and from the same place. In how many hours will they be 300 kilometres apart?
- Two trains start at the same time from the same place and travel in opposite directions. If the rate of one is 6 km/h more than the rate of the other and they are 168 kilometres apart at the end of 4 hours, what is the rate of each?
- Two cyclists start from the same point and ride in opposite directions. One cyclist rides twice as fast as the other. In three hours, they are 72 kilometres apart. Find the rate of each cyclist.
- Two small planes start from the same point and fly in opposite directions. The first plane is flying 25 km/h slower than the second plane. In two hours, the planes are 430 kilometres apart. Find the rate of each plane.
- On a 130-kilometre trip, a car travelled at an average speed of 55 km/h and then reduced its speed to 40 km/h for the remainder of the trip. The trip took a total of 2.5 hours. For how long did the car travel at 40 km/h?
- Running at an average rate of 8 m/s, a sprinter ran to the end of a track and then jogged back to the starting point at an average of 3 m/s. The sprinter took 55 s to run to the end of the track and jog back. Find the length of the track.
Answer Key 8.8
Scholar-Practitioner Project: Final Report
Having studied and evaluated different challenges and opportunities associated with the use of secondary data, and having learned how to evaluate, analyze, and present information generated from the analysis of secondary data, now is your opportunity to synthesize this information and present your work.This week, you will compile all of the Assignments and your Instructor’s recommended changes into a Final Scholar-Practitioner Project Report.To prepare:Review the Ostchega, et al. (2012) article located in this week’s Learning Resources as an example for formatting your final report.Your Final Scholar-Practitioner Project Report should be 20 pages (excluding title page and references).Make sure to include the following in your report:Title pageResearch topic and backgroundResearch questionSecondary data set usedHow data collected in this data setValidity and reliability of the data setData analysis plan (variables and statistical procedures)Data dictionary and data tableResults and interpretation (descriptive and inferential tables)Descriptive analysis tables or graphs with interpretationInferential analysis tables or graphs with interpretationLimitations of studyTwo recommendations for future researchImplications for social changeReferencesSupport your paper with additional scholarly resources. Use APA formatting for your paper and to cite your resources.ReferencesOstchega, Y., Zhang, G., Sorlie, P., Hughes, J. P., Reed-Gillette, D. S., Nwankwo, T., & Yoon, S. (2012). Blood pressure randomized methodology study comparing automatic oscillometric and mercury sphygmomanometer devices: National Health and Nutrition Examination Survey, 2009–2010. National Health Statistics Reports, no 59. Retrieved from http://www.cdc.gov/nchs/data/nhsr/nhsr059.pdf