Word problems on conversion

Word Problems on Conversion of Units: Conversion of units is a multi-step process that involves multiplication or division by a numerical factor. In Mathematics, while solving numerical problems, it is required to convert the units. Thus the conversion of units should be needed to solve the required calculations wherever it is necessary.

For example, if we need to calculate the area of the rectangle, in which length is given in centimetres (left( {{rm{cm}}} right)), and the width is given in metres ({rm{m}}), then it is necessary to convert any one unit either length or width, so that both the units become the same.

Therefore, to solve the word problems in mathematics, learning the conversion of units is necessary. This article will discuss the conversion of units in more detail.

Definition of Word Problems on Conversion of Units

Conversion of units is a multi-step process that involves multiplication or division by a numerical factor. Word problems on the conversion of units consist of a few sentences describing a real-life scenario where mathematical definitions and concepts of converting units from one unit to another unit are used to solve a problem.

The conversion of units may also require selecting the correct number of significant digits and rounding off. In mathematics, we convert the units from one unit to the other unit for better understanding. 

For example, the length of a garden is measured in yards, whereas the length of a table is measured in inches. But we cannot measure the length of a finger in miles. To measure different quantities, different units of measurements are needed.

The conversion of units should be needed to solve the required calculations wherever it is necessary. For example, if we need to calculate the area of the rectangle, in which length is given in centimetres (left( {{rm{cm}}} right)), and the width is given in metres (left( {{rm{m}}} right)), then it is necessary to convert any one unit, either length or width, to make them uniform.

Converting Metric Units Word Problems

In mathematics, we have metric systems such as measuring units of length and distance, weight, and capacity. As we discussed, to solve the word problems correctly, we need to learn the conversion of units, and it is necessary too.

The metric system was introduced in France in the year (1790). The metric system of measuring units is based on the decimal system. The base units for length is metre, for weight kilogram and seconds for the time.

Unit conversion is a multi-step process that involves multiplication or division by a numerical factor. To convert any bigger unit to a smaller unit, we should multiply with the conversion factor. Similarly, to convert any smaller unit to a bigger unit, we should divide it with the conversion factor.

Word Problems on Conversion of Units of Length

Length is a one-dimensional scalar quantity, which measures the line segment. The basic unit used for measuring the length is a metre ({rm{(m)}}). Depending on the specimen or object used for measurement, we have different types of units like ({rm{cm,}},{rm{km,}},{rm{inches,}},{rm{ft}}), etc.

For example, the length of a garden is measured in yards, whereas the length of a table is measured in inches. But we cannot measure the length of a finger in miles. To measure different quantities, different units of measurements are needed. 

The below chart gives the conversion of length:

To convert a unit from a metre to a centimetre, we should multiply by (100) such that (1,{rm{m}}, = ,100,{rm{cm}})

Example:

Asit and Keerthi each ran on a treadmill exactly for (90) minutes. Asit’s treadmill showed he had run (18500) meters. Keerthi’s treadmill showed she had run (20) kilometres. Who ran farther, and how much?

The time took by the Keerthi and Asit are the same, that is (90) minutes.

Here, the distance covered by Asit and Keerthi has different measuring units. For uniformity in the calculation, we have to convert the units from ({rm{km}}) to ({rm{m}}) or ({rm{m}}) to ({rm{km}}).

Let us convert ({rm{km}},) to ({rm{m}},).

So, the distance covered by the Asit on the treadmill ( = 18,500,{rm{m}})

And, the distance covered by the Keerthi on treadmill ( = 20,,{rm{km}}{rm{ = }}{rm{20}} times {rm{1000}}{rm{m  = }}{rm{20,}},{rm{000}},{rm{m}})

The difference in their distances is ({rm{20,}},{rm{000}},{rm{m}},{rm{ – }},{rm{18500}},{rm{m}},{rm{ = 1500}},{rm{m}})

So, Keerthi ran farther as compared with Asit by ({rm{20,}},{rm{000}},{rm{m}},{rm{ – }},{rm{18500}},{rm{m}},{rm{ = 1500}},{rm{m}})

So, Keerthi ran farther as compared with Asit by ({rm{1500}},{rm{m}}) or ({rm{1.5}},{rm{km}}).

Word Problems on Conversion of Units of Weight

Weight is the one-dimensional vector quantity, which is used for the measurement of objects. Generally, the weight of the object is measured in the base unit kilogram (left( {{rm{kg}}} right).)  We know that weight of the person is measured in ({rm{(kg)}}) and the weight of the small pieces of gold is measured in grams. So, it is important to convert the units of the weights while solving word problems for uniformity in the calculation.

The below figure shows the conversion of weights from one unit to another unit:

Example:

Nag is carrying (2.5,{rm{kg}}) of apples and (5,{rm{g}}) of carrying bag. Find the total weight she is holding in her hand.

The total weight ( = 2.5 times 1000,{rm{g}},{rm{ +  5g}},{rm{ = }},{rm{2505}},{rm{g}})

Word Problems on Conversion of Units of Time

We know that seconds are the basic unit for measuring time. We have to convert the units of time from one unit to another unit for solving the problems. The below chart gives the conversion of time:

Example:
The time taken to reach the shop is (30) minutes and from the due to heavy traffic, the time taken to reach the house is (1) hour. Find the total time taken?

The total time taken ( = 30min {rm{utes}} + 1 times 60min {rm{utes}} = 90min {rm{utes}},)

Word Problems on Conversion of Units of Area

The area is the two-dimensional property. We have different units for measuring the area. The below figure shows the conversion of area units:

Example:

The area of the parking lot is (12{{rm{m}}^2}) and (50,{rm{c}}{{rm{m}}^{rm{2}}}) Find the total area?

The total area ( = ,12, times ,{100^2},{rm{c}}{{rm{m}}^2}, + ,500,{rm{c}}{{rm{m}}^2}, = ,1440000, + 500, = ,1440500,{rm{c}}{{rm{m}}^2})

Word Problems on Conversion of Units of Capacity

Capacity describes the volume of the object. The different types of units used for measuring the capacity are given below:

Example:

The capacity of the water bottle is (1,{rm{l}}) and the cap is (1,{rm{ml}}) Find the total capacity of the bottle.

The total capacity ( = 1 times 1000,{rm{ml}} + 1,{rm{ml}} = 1001,{rm{ml}})

Solved Examples – Word Problems on Conversion of Units

Q.1. The distance between the two places in a city is (31,,680, feet) Express the distance in miles.
Ans: Given the distance between the places ({rm{ = 31,680}},{rm{feet}})
We know that ({rm{1feet}} = frac{1}{{5280}}{rm{miles}})
So, total distance in miles ( = frac{{31,680}}{{5280}} = 6,{rm{miles}})

Q.2. Asit’s mom took (30) minutes to cut the vegetables, and she took (1) hour in cooking. Find how many minutes she took to complete the whole cooking?
Ans: The time taken for cutting the vegetables ( = 30,{rm{minutes}})
The time taken for cooking ( = 1,{rm{hour}} = 60,{rm{minutes}})
Total time taken for whole cooking ( = ,30, + ,60, = ,90,{rm{minutes}})

Q.3. Vicky has (14,500,{rm{g}}) of sand in his sandbox, and he bought (7500,{rm{g}}) of sand from the beach. Total how many kilograms of sand Vicky has in his sandbox.
Ans:Initial sand in the box is (14,500,{rm{g}},{rm{ = }},frac{{14,500}}{{1000}},{rm{kg}}, = ,14.,5,{rm{kg}})
The sand bough from the beach ( = 7500,{rm{g}},{rm{ = }},frac{{7500}}{{1000}},{rm{kg}}, = ,7.,5,{rm{kg}})
Total sand in the box ( = 14.5,{rm{kg}},{rm{ + }},7.5,{rm{kg}}, = ,22,{rm{kg}})

Q.4. Jessica measures two line segments. The first line segment is (30,{rm{cm}}) long. The second line segment is (500,{rm{mm}}) long. How long are the two line segments together? (answer in cm)
Ans: The length of the first line segment ( = ,,30,{rm{cm}})
The length of the second line segment ({rm{ = }},{rm{500}},{rm{mm}},{rm{ = }},frac{{500}}{{10}},{rm{cm}},{rm{ = }},{rm{50}},{rm{cm}})
The total length of two-line segments ( = ,,30,{rm{m}},{rm{ + }},{rm{50}},{rm{m}}, = ,80,{rm{m}})

Q.5. The length of the box is (2,{rm{m}}) and the width is (40,{rm{cm}}) Find the area of the box in ({rm{c}}{{rm{m}}^2})
Ans: Given the length of the box (2,{rm{m}})
Width of the box ({rm{ = }},{rm{40}},{rm{cm}})
Area of the box ({rm{ = }},{rm{length}}, times ,{rm{width}},{rm{ = }},{rm{200}},, times ,40, = ,8000,{rm{c}}{{rm{m}}^2})

Summary

In this article, we have discussed various methods of the conversion of units from one unit to another unit. This article also discusses the conversion of metric units and word problems on the conversion of metric units.
In this article, we have studied the word problems on the conversion of units of length, weight, area, time and capacity, along with the solved examples that help us solve the numerical problems easily.

Frequently Asked Questions – Word Problems on Conversion of Units

Q.1. How do you solve unit conversion problems?
Ans: The following steps are to be followed to do unit conversion problems.
1. Read the data and the given units.
2. Now, multiply or divide as required conversion with the conversion factor.
3. Then, solve the problems using the proper formulas and operations.

Q.2. How do you solve metric word problems?
Ans: The metric word problems are to be solved by using the unit conversion. Convert any bigger unit to the smaller unit, and we should multiply with the conversion factor. Similarly, to convert any smaller unit to a bigger unit, we should divide it with the conversion factor.

Q.3. Why is unit conversion important?
Ans: To solve many real-life problems, unit conversion is very important because we cannot perform basic operations like addition, subtraction, multiplication, division etc., unless the two quantities are in the same units.

Q.4. What are the three basic metric units?
Ans: The three basic metric units are metre for length, gram for weight and litre for capacity.

Q.5. What is unit conversion?
Ans: Conversion of units is a multi-step process that involves multiplication or division by a numerical factor.

Learn about Measurement here

We hope you find this article on ‘Word Problems on Conversion of Units‘ helpful. In case of any queries, you can reach back to us in the comments section, and we will try to solve them. 

---

Chapter 1: Algebra Review

One application of rational expressions deals with converting units. Units of measure can be converted by multiplying several fractions together in a process known as dimensional analysis.

The trick is to decide what fractions to multiply. If an expression is multiplied by 1, its value does not change. The number 1 can be written as a fraction in many different ways, so long as the numerator and denominator are identical in value. Note that the numerator and denominator need not be identical in appearance, but rather only identical in value. Below are several fractions, each equal to 1, where the numerator and the denominator are identical in value. This is why, when doing dimensional analysis, it is very important to use units in the setup of the problem, so as to ensure that the conversion factor is set up correctly.

 If 1 pound = 16 ounces, how many pounds are in 435 ounces?

[latex]begin{array}{rrll} 435text{ oz}&=&435cancel{text{oz}}times dfrac{1text{ lb}}{16cancel{ text{oz}}} hspace{0.2in}& text{This operation cancels the oz and leaves the lbs} \ \ &=&dfrac{435text{ lb}}{16} hspace{0.2in}& text{Which reduces to } \ \ &=&27dfrac{3}{16}text{ lb} hspace{0.2in}& text{Solution} end{array}[/latex]

The same process can be used to convert problems with several units in them. Consider the following example.

A student averaged 45 miles per hour on a trip. What was the student’s speed in feet per second?

[latex]begin{array}{rrll} 45 text{ mi/h}&=&dfrac{45cancel{text{mi}}}{cancel{text{hr}}}times dfrac{5280 text{ ft}}{1cancel{ text{mi}}}times dfrac{1cancel{text{hr}}}{3600text{ s}}hspace{0.2in}&text{This will cancel the miles and hours} \ \ &=&45times dfrac{5280}{1}times dfrac{1}{3600} text{ ft/s}hspace{0.2in}&text{This reduces to} \ \ &=&66text{ ft/s}hspace{0.2in}&text{Solution} end{array}[/latex]

Convert 8 ft³ to yd³.

[latex]begin{array}{rrll} 8text{ ft}^3&=&8text{ ft}^3 times dfrac{(1text{ yd})^3}{(3text{ ft})^3}&text{Cube the parentheses} \ \ &=&8text{ }cancel{text{ft}^3}times dfrac{1text{ yd}^3}{27text{ }cancel{text{ft}^3}}&text{This will cancel the ft}^3text{ and replace them with yd}^3 \ \ &=&8times dfrac{1text{ yd}^3}{27}&text{Which reduces to} \ \ &=&dfrac{8}{27}text{ yd}^3text{ or }0.296text{ yd}^3&text{Solution} end{array}[/latex]

A room is 10 ft by 12 ft. How many square yards are in the room? The area of the room is 120 ft² (area = length × width).

Converting the area yields:

[latex]begin{array}{rrll} 120text{ ft}^2&=&120text{ }cancel{text{ft}^2}times dfrac{(1text{ yd})^2}{(3text{ }cancel{text{ft}})^2}&text{Cancel ft}^2text{ and replace with yd}^2 \ \ &=&dfrac{120text{ yd}^2}{9}&text{This reduces to} \ \ &=&13dfrac{1}{3}text{ yd}^2&text{Solution} \ \ end{array}[/latex]

The process of dimensional analysis can be used to convert other types of units as well. Once relationships that represent the same value have been identified, a conversion factor can be determined.

A child is prescribed a dosage of 12 mg of a certain drug per day and is allowed to refill his prescription twice. If there are 60 tablets in a prescription, and each tablet has 4 mg, how many doses are in the 3 prescriptions (original + 2 refills)?

[latex]begin{array}{rrll} 3text{ prescriptions}&=&3cancel{text{pres.}}times dfrac{60cancel{text{tablets}}}{1cancel{text{pres.}}}times dfrac{4cancel{text{mg}}}{1cancel{text{tablet}}}times dfrac{1text{ dosage}}{12cancel{text{mg}}}&text{This cancels all unwanted units} \ \ &=&dfrac{3times 60times 4times 1}{1times 1times 12}text{ or }dfrac{720}{12}text{ dosages}&text{Which reduces to} \ \ &=&60text{ daily dosages}&text{Solution} \ \ end{array}[/latex]

Metric and Imperial (U.S.) Conversions

Distance

[latex]begin{array}{rrlrrl} 12text{ in}&=&1text{ ft}hspace{1in}&10text{ mm}&=&1text{ cm} \ 3text{ ft}&=&1text{ yd}&100text{ cm}&=&1text{ m} \ 1760text{ yds}&=&1text{ mi}&1000text{ m}&=&1text{ km} \ 5280text{ ft}&=&1text{ mi}&&& end{array}[/latex]

Imperial to metric conversions:

[latex]begin{array}{rrl} 1text{ inch}&=&2.54text{ cm} \ 1text{ ft}&=&0.3048text{ m} \ 1text{ mile}&=&1.61text{ km} end{array}[/latex]

Area

[latex]begin{array}{rrlrrl} 144text{ in}^2&=&1text{ ft}^2hspace{1in}&10,000text{ cm}^2&=&1text{ m}^2 \ 43,560text{ ft}^2&=&1text{ acre}&10,000text{ m}^2&=&1text{ hectare} \ 640text{ acres}&=&1text{ mi}^2&100text{ hectares}&=&1text{ km}^2 end{array}[/latex]

Imperial to metric conversions:

[latex]begin{array}{rrl} 1text{ in}^2&=&6.45text{ cm}^2 \ 1text{ ft}^2&=&0.092903text{ m}^2 \ 1text{ mi}^2&=&2.59text{ km}^2 end{array}[/latex]

Volume

[latex]begin{array}{rrlrrl} 57.75text{ in}^3&=&1text{ qt}hspace{1in}&1text{ cm}^3&=&1text{ ml} \ 4text{ qt}&=&1text{ gal}&1000text{ ml}&=&1text{ litre} \ 42text{ gal (petroleum)}&=&1text{ barrel}&1000text{ litres}&=&1text{ m}^3 end{array}[/latex]

Imperial to metric conversions:

[latex]begin{array}{rrl} 16.39text{ cm}^3&=&1text{ in}^3 \ 1text{ ft}^3&=&0.0283168text{ m}^3 \ 3.79text{ litres}&=&1text{ gal} end{array}[/latex]

Mass

[latex]begin{array}{rrlrrl} 437.5text{ grains}&=&1text{ oz}hspace{1in}&1000text{ mg}&=&1text{ g} \ 16text{ oz}&=&1text{ lb}&1000text{ g}&=&1text{ kg} \ 2000text{ lb}&=&1text{ short ton}&1000text{ kg}&=&1text{ metric ton} end{array}[/latex]

Imperial to metric conversions:

[latex]begin{array}{rrl} 453text{ g}&=&1text{ lb} \ 2.2text{ lb}&=&1text{ kg} end{array}[/latex]

Temperature

Fahrenheit to Celsius conversions:

[latex]begin{array}{rrl} ^{circ}text{C} &= &dfrac{5}{9} (^{circ}text{F} — 32) \ \ ^{circ}text{F}& =& dfrac{9}{5}(^{circ}text{C} + 32) end{array}[/latex]

Celsius to Fahrenheit Conversion Scale

°F	−40°F	−22°F	−4°F	14°F	32°F	50°F	68°F	86°F	104°F	122°F	140°F	158°F	176°F	194°F	212°F
°C	−40°C	−30°C	−20°C	−10°C	0°C	10°C	20°C	30°C	40°C	50°C	60°C	70°C	80°C	90°C	100°C

Questions

For questions 1 to 18, use dimensional analysis to perform the indicated conversions.

1. 7 miles to yards
2. 234 oz to tons
3. 11.2 mg to grams
4. 1.35 km to centimetres
5. 9,800,000 mm to miles
6. 4.5 ft² to square yards
7. 435,000 m² to square kilometres
8. 8 km² to square feet
9. 0.0065 km³ to cubic metres
10. 14.62 in³ to square centimetres
11. 5500 cm³ to cubic yards
12. 3.5 mph (miles per hour) to feet per second
13. 185 yd per min. to miles per hour
14. 153 ft/s (feet per second) to miles per hour
15. 248 mph to metres per second
16. 186,000 mph to kilometres per year
17. 7.50 tons/yd² to pounds per square inch
18. 16 ft/s² to kilometres per hour squared

For questions 19 to 27, solve each conversion word problem.

1. On a recent trip, Jan travelled 260 miles using 8 gallons of gas. What was the car’s miles per gallon for this trip? Kilometres per litre?
2. A certain laser printer can print 12 pages per minute. Determine this printer’s output in pages per day.
3. An average human heart beats 60 times per minute. If the average person lives to the age of 86, how many times does the average heart beat in a lifetime?
4. Blood sugar levels are measured in milligrams of glucose per decilitre of blood volume. If a person’s blood sugar level measured 128 mg/dL, what is this in grams per litre?
5. You are buying carpet to cover a room that measures 38 ft by 40 ft. The carpet cost $18 per square yard. How much will the carpet cost?
6. A cargo container is 50 ft long, 10 ft wide, and 8 ft tall. Find its volume in cubic yards and cubic metres.
7. A local zoning ordinance says that a house’s “footprint” (area of its ground floor) cannot occupy more than ¼ of the lot it is built on. Suppose you own a [latex]frac{1}{3}[/latex]-acre lot (1 acre = 43,560 ft²). What is the maximum allowed footprint for your house in square feet? In square metres?
8. A car travels 23 km in 15 minutes. How fast is it going in kilometres per hour? In metres per second?
9. The largest single rough diamond ever found, the Cullinan Diamond, weighed 3106 carats. One carat is equivalent to the mass of 0.20 grams. What is the mass of this diamond in milligrams? Weight in pounds?

Answer Key 1.6

Conversion
is the main way of forming verbs in Modern English. Verbs can be
formed from nouns of different semantic groups and have different
meanings because of that, e.g.

a)
verbs have instrumental meaning if they are formed from nouns
denoting parts of a human body e.g. to eye, to finger, to elbow, to
shoulder etc. They have instrumental meaning if they are formed from
nouns denoting tools, machines, instruments, weapons, e.g. to hammer,
to machine-gun, to rifle, to nail,

b)
verbs can denote an action characteristic of the living being denoted
by the noun from which they have been converted, e.g. to crowd, to
wolf, to ape,

c)
verbs can denote acquisition, addition or deprivation if they are
formed from nouns denoting an object, e.g. to fish, to dust, to peel,
to paper,

d)
verbs can denote an action performed at the place denoted by the noun
from which they have been converted, e.g. to park, to garage, to
bottle, to corner, to pocket,

e)
verbs can denote an action performed at the time denoted by the noun
from which they have been converted e.g. to winter, to week-end .

Verbs
can be also converted from adjectives, in such cases they denote the
change of the state, e.g. to tame (to become or make tame) , to
clean, to slim etc.

Nouns
can also be formed by means of conversion from verbs. Converted nouns
can denote:

a)
instant of an action e.g. a jump, a move,

b)
process or state e.g. sleep, walk,

c)
agent of the action expressed by the verb from which the noun has
been converted, e.g. a help, a flirt, a scold ,

d)
object or result of the action expressed by the verb from which the
noun has been converted, e.g. a burn, a find, a purchase,

e)
place of the action expressed by the verb from which the noun has
been converted, e.g. a drive, a stop, a walk.

Many
nouns converted from verbs can be used only in the Singular form and
denote momentaneous actions. In such cases we have partial
conversion. Such deverbal nouns are often used with such verbs as :
to have, to get, to take etc., e.g. to have a try, to give a push, to
take a swim .

4. Different problems of conversion:

a)
synchronic and diachronic approaches to the present day conversion
pairs;

Conversion
pairs are distinguished by the structural identity of the root and
phonetic identity of the stem of each of the two words.

Synchronically
we deal with pairs of words related through conversion that coexist
in contemporary English. The two words, e.g. to
break and
a
break, being
phonetically identical, the question arises whether they have the
same or identical stems, as some linguists are inclined to believe.¹
It will be recalled that the stem carries quite a definite
part-of-speech meaning; for instance, within the word-cluster to
dress
— dress
— dresser
— dressing
— dressy,
the
stem dresser
— carries
not only the lexical meaning of the root-morpheme dress-,
but
also the meaning of substantivity, the stem dressy-
the
meaning of quality, etc. These two ingredients
— the
lexical meaning of the root-morpheme and the part-of-speech meaning
of the stem
— form
part of the meaning of the whole word. It is the stem that requires a
definite paradigm; for instance, the word dresser
is
a noun primarily because it has a noun-stem and not only because of
the noun paradigm; likewise, the word materialise
is
a verb, because first and foremost it has a verbal stem possessing
the lexico-grammatical meaning of process or action and requiring a
verb paradigm.

What
is true of words whose root and stem do not coincide is also true of
words with roots and stems that coincide: for instance, the word atom
is
a noun because of the substantival character of the stem requiring
the noun paradigm. The word sell
is
a verb because of the verbal character of its stem requiring the verb
paradigm, etc. It logically follows that the stems of two words
making up a conversion pair cannot be regarded as being the same or
identical: the stem hand-
of
the noun hand,
for
instance, carries a substantival meaning together with the system of
its meanings, such as: 1)
the
end of the arm beyond the wrist; 2)
pointer
on a watch or clock; 3)
worker
in a factory; 4)
source
of information, etc.; the stem hand-
of
the verb hand
has
a different part-of-speech meaning, namely that of the verb, and a
different system of meanings: 1)
give
or help with the hand, 2)
pass,
etc. Thus, the stems of word-pairs related through conversion have
different part-of-speech and denotational meanings. Being
phonetically identical they can be regarded as homonymous stems.

A
careful examination of the relationship between the lexical meaning
of the root-morpheme and the part-of-speech meaning of the stem
within a conversion pair reveals that in one of the two words the
former does not correspond to the latter. For instance, the lexical
meaning of the root-morpheme of the noun hand
corresponds
to the part-of-speech meaning of its stem: they are both of a
substantival character; the lexical meaning of the root-morpheme of
the verb hand,
however,
does not correspond to the part-of-speech meaning of the stem: the
root-morpheme denotes an object, whereas the part-of-speech meaning
of the stem is that of a process. The same is true of the noun fall
whose
stem is of a substantival character (which is proved by the noun
paradigm fall
— falls
— fall’s
— falls’,
whereas
the root-morpheme denotes a certain process.

It
will be recalled that the same kind of non-correspondence is typical
of the derived word in general. To give but two examples, the
part-of-speech meaning of the stem blackness
—
is
that of substantivity, whereas the root-morpheme black-denotes a
quality; the part-of-speech meaning of the stem eatable-
(that
of qualitativeness) does not correspond to the lexical meaning of the
root-morpheme denoting a process. It should also be pointed out here
that in simple words the lexical meaning of the root corresponds to
the part-of-speech meaning of the stem, cf. the two types of meaning
of simple words like black
a,
eat
v,
chair
n,
etc.
Thus, by analogy with the derivational character of the stem of a
derived word it is natural to regard the stem of one of the two words
making up a conversion pair as being of a derivational character as
well. The essential difference between affixation and conversion is
that affixation is characterised by both semantic and structural
derivation (e.g. friend
— friendless, dark
— darkness,
etc.),
whereas conversion displays only semantic derivation, i.e. hand
— to hand, fall
— to
fall, taxi
— to
taxi, etc.;
the difference between the two classes of words in affixation is
marked both by a special derivational affix and a paradigm, whereas
in conversion it is marked only by paradigmatic forms.

A
diachronic
semantic
analysis of a conversion pair reveals that in the course of time the
semantic structure of the base may acquire a new meaning or several
meanings under the influence of the meanings of the converted word.
This semantic process has been termed reconversion
in linguistic literature.²
There is an essential difference between conversion and reconversion:
being a way of forming words conversion leads to a numerical
enlargement of the English vocabulary, whereas reconversion only
brings about a new meaning correlated with one of the meanings of the
converted word. Research has shown that reconversion only operates
with denominal verbs and deverbal nouns. As an illustration the
conversion pair smoke
n
— smoke
v
may
be cited. According to the Oxford
English Dictionary some
of the meanings of the two words are:

SMOKE
я

1.
the
visible volatile product given off by burning or smouldering
substances (1000)¹
c)
the act of smoke coming out into a room instead of passing up the
chimney (1715)

SMOKE
v

1.
intr.
to
produce or give forth smoke (1000)

‘c)
of a room, chimney, lamp, etc.: to be smoky, to emit smoke as the
result of imperfect draught or improper burning (1663)

Comparison
makes it possible to trace the semantic development of each word. The
verb smoke
formed
in 1000
from
the noun smoke
in
the corresponding meaning had acquired by 1663
another
meaning by a
metaphorical
transfer which, in turn, gave rise to a correlative meaning of the
noun smoke
in
1715
through
reconversion.

b)
the word-building means in conversion.

Соседние файлы в предмете [НЕСОРТИРОВАННОЕ]

• #
• #
• #
• #
• #
• #
• #
• #

  13.04.2015341.5 Кб43.doc

• #
• #
• #

Practice the
questions given in the worksheet on conversion of measuring length.

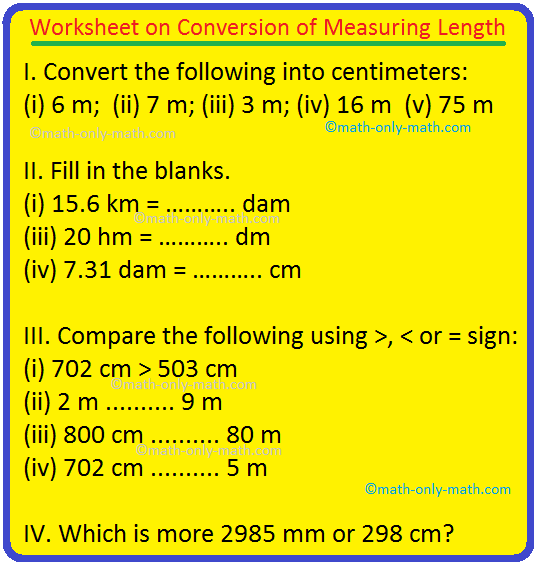
I. Convert the following into centimeters:

Hint: To convert meters into centimeters, multiply the number of meters by 100.

(i) 8 m = 8 × 100 cm = 800 cm

(ii) 4 m

(iii) 14 m  

(iv) 25 m  

(v) 50 m  

(vi) 6 m  

(vii) 7 m 

(viii) 3 m

(ix) 16 m  

(x) 75 m  

II. Convert the
following into meters:

Hint: To convert kilometers into meters, multiply
the number of kilometers by 1000.

(i) 8 km = 8 × 1000 m = 8000 m

(ii) 15 km  

(iii) 10 km  

(iv) 22 km  

(v) 9 km  

(vi) 5 km  

(vii) 2 km  

(viii) 7 km  

(ix) 13 km  

(x) 56 km  

III. Convert the
following into meters:

Hint: To convert centimeters into meters, divide
the number of centimeters by 100.

(i) 500 cm = (500 ÷ 100) m = 5 m

(ii) 400 cm  

(iii) 1400 cm  

(iv) 1900 cm  

(v) 600 cm  

(vi) 900 cm  

(vii) 1000 cm  

(viii) 300 cm  

(ix) 3200 cm  

(x) 4600 cm  

IV. Convert the
following into kilometers:

Hint: To convert meters into kilometers, divide
the number of meters by 1000.

(i) 7000 m = (7000 ÷ 1000) km = 7 km

(ii) 12000 m

(iii) 15000 m

(iv) 19000 m

(v) 62000 m

(vi) 11000 m

(vii) 6000 m  

(viii) 8000 m

(ix) 53000 m  

(x) 79000 m  

V. Convert the following centimeters into meters and centimeters:

Hint: To convert centimeters into meters and centimeters, divide the number of centimeters by 100, the quotient represents
meter and remainder represents centimeters.

(i) 287 cm = (287 ÷ 100) m = 2 m 87 cm

(ii) 620 cm

(iii) 932 cm

(iv) 465 cm

(v) 592 cm

(vi) 507 cm

(vii) 802 cm

(viii) 120 cm

(ix) 1020 cm

(x) 333 cm

VI. Convert the
following meters into kilometers and meters:

Hint: To convert meters into kilometers and meters, dive the number of meters by by 1000, the quotient represents kilometers and remainder represents meters.

(i) 1235 m = (1235 ÷ 1000) km = 1 km 235 cm

(ii) 4689 m

(iii) 7248 m

(iv) 8824 m

(v) 2326 m

(vi) 6205 m

(vii) 5320 m

(viii) 9007 m

(ix) 1030 m

(x) 2876 m

VII. Compare the following using >, < or = sign:

Hint: To compare the measurement of length by cm, m and km, we observe that cm < m < km.

(i) 702 cm > 503 cm

(ii) 2 m ………. 9 m

(iii) 800 cm ………. 80 m

(iv) 702 cm ………. 5 m

(v) 8 km ………. 7000 m

(vi) 625 cm ………. 9 m

(vii) 10 m ………. 4 cm

(viii) 1000 m ………. 1000 km

(ix) 100 cm ………. 100 m

(x) 1 km ………. 1000 m

VIII. Convert the following into millimeters.

(i) 0.625 m

(ii) 9 hm

(iii) 8 m

(iv) 27 dam

(v) 517 cm

(vi) 15 dm

IX. Convert the following into kilometers.

(i) 14 m

(ii) 59 hm

(iii) 136 dam

(iv) 207 dam

(v) 5600 cm

(vi) 10000 cm

X. Convert the following into centimeters.

(i) 66 mm

(ii) 32 dm

(iii) 4 cm 8 mm

(iv) 63 km

(v) 16 m

(vi) 1.5 dm

XI. Fill in the blanks.

(i) 15.6 km = ……….. dam

(ii) 20 hm = ……….. dm

(iii) 7.31 dam = ……….. cm

(iv) 45 hm = ……….. m

(v) 2.5 dm = ……….. cm

(vi) 7 cm = ……….. m

(vii) 325 mm = ……….. m

(viii) 900 cm = ……….. m

Word Problems on Conversion of Measuring Length:

XII. The height of one floor of a building is 304 cm. If the
building has 7 floors, what is the height of building in meters?

XIII. Which is more 2985 mm or 298 cm?

Answers for the worksheet on conversion of measuring length are given below.

Answers:

I. (ii) 4 × 100 cm, 400 cm

(iii) 14 × 100 cm, 1400 cm

(iv) 25 × 100 cm, 2500 cm

(v) 50 × 100 cm, 5000 cm

(vi) 6 × 100 cm, 600 cm

(vii) 7 × 100 cm, 700 cm

(viii) 3 × 100 cm, 300 cm

(ix) 16 × 100 cm, 1600 cm

(x) 75 × 100 cm, 7500 cm

II. (ii) 15 × 1000 m, 15000 m

(iii) 10 × 1000 m, 10000 m

(iv) 22 × 1000 m, 22000 m

(v) 9 × 1000 m, 9000 m

(vi) 5 × 1000 m, 5000 m

(vii) 2 × 1000 m, 2000 m

(viii) 7 × 1000 m, 7000 m

(ix) 13 × 1000 m, 13000 m

(x) 56 × 1000 m, 56000 m

III. (ii) (400 ÷ 100) m = 4 m

(iii) (1400 ÷ 100) m = 14 m

(iv) (1900 ÷ 100) m = 19 m

(v) (600 ÷ 100) m = 6 m

(vi) (900 ÷ 100) m = 9 m

(vii) (1000 ÷ 100) m = 10 m

(viii) (300 ÷ 100) m = 3 m

(ix) (3200 ÷ 100) m = 32 m

(x) (4600 ÷ 100) m = 46 m

IV.  (ii) (12000 ÷ 1000) km = 12 km

(iii) (15000 ÷ 1000) km = 15 km

(iv) (19000 ÷ 1000) km = 19 km

(v) (62000 ÷ 1000) km = 62 km

(vi) (11000 ÷ 1000) km = 11 km

(vii) (6000 ÷ 1000) km = 6 km

(viii) (8000 ÷ 1000) km = 8 km

(ix) (53000 ÷ 1000) km = 53 km

(x) (79000 ÷ 1000) km = 79 km

V. (ii) (620 ÷ 100) m = 6 m 20 cm

(iii)(932 ÷ 100) m = 9 m 32 cm

(iv) (465 ÷ 100) m = 4 m 65 cm

(v) (592 ÷ 100) m = 5 m 92 cm

(vi) (507 ÷ 100) m = 5 m 7 cm

(vii) (802 ÷ 100) m = 8 m 2 cm

(viii) (120 ÷ 100) m = 1 m 20 cm

(ix) (1020 ÷ 100) m = 10 m 20 cm

(x) (333 ÷ 100) m = 3 m 33 cm

VI.  (ii)(4689 ÷ 1000) km = 4 km 689 cm

(iii) (7248 ÷ 1000) km = 7 km 248 cm

(iv) (8824 ÷ 1000) km = 8 km 824 cm

(v) (2326 ÷ 1000) km = 2 km 326 cm

(vi) (6205 ÷ 1000) km = 6 km 205 cm

(vii) (5320 ÷ 1000) km = 5 km 320 cm

(viii) (9007 ÷ 1000) km = 9 km 7 cm

(ix) (1030 ÷ 1000) km = 1 km 30 cm

(x) (2876 ÷ 1000) km = 2 km 876 cm

VII. (ii) <

(iii) <

(iv) >

(v) >

(vi) <

(vii) >

(viii) <

(ix) <

(x) =

VIII. (i) 625 mm

(ii) 900000 mm

(iii) 8000 mm

(iv) 270000 mm

(v) 5170 mm

(vi) 1500 mm

IX. (i) 0.014 km

(ii) 5.9 km

(iii) 13.6 km

(iv) 20.7 km

(v) 0.056 km

(vi) 0.1 km

X. (i) 6.6 cm

(ii) 320 cm

(iii) 4.8 cm

(iv) 6300000 cm

(v) 1600 cm

(vi) 15 cm

XI. (i) 1560

(ii) 20000

(iii) 7310

(iv) 4500

(v) 25

(vi) 0.07

(vii) 0.325

(viii) 9 m

XII. 21.28 cm

XIII. 2.985 mm

Measurement of Length:

• Standard Unit of Length 
• Conversion of Standard Unit of Length
• Addition
  of Length
• Subtraction
  of Length
• Addition and Subtraction of Measuring Length
• Addition and Subtraction of Measuring Mass
• Addition and Subtraction of Measuring Capacity

3rd Grade Math Worksheets

3rd Grade Math Lessons

From Worksheet on Conversion of Measuring Length to HOME PAGE

Word Problems & Conversion pdf.

Prior to teaching this lesson, each student should have a paper copy of p. 7 of the SB file. I simply copied the page and made a classroom set from the PDF. For students who need the SB file to look at during instruction, I copied the whole file.  It keeps them on task and gives me something to point to on their desk to draw their attention back during whole class instruction.

 Using the SB, I opened the lesson with an example of a conversion word problem. I wanted students to read the problem silently first.  I led the discussion using a few questions from the second page about what strategies we would use to solve the problem.

As we worked through the word problems, students were able to ask questions about problem solving. I used explicit instruction showing them that it was important to form the situation equation. I told them to think about what was going on in the problem and create an equation using the units given. Then, move to creating the solution equation by converting to the units needed to solve the problem. I showed them how to convert, using a T chart. This satisfies the section of the standard that requires students to convert in a two column chart.

When we got to the 5th page of the SB file, students were reminded to use their strategies, create situation equations and solution equations as they solved the problems together. I asked  a student to come to the SB and show how she converted. She was confused about the use of the t chart and had the concept wrong. T chart conversion We worked together to get it corrected so others weren’t confused.T chart Misunderstanding Corrected. For some reason, she thought she had to convert both numbers. I think this is where the «how» gets in the way of the «why» with students. She was not thinking about why she was converting, she just knew she needed to. We solved the problem and finished it up. Students copied it step by step in their notebooks. I insisted on equations with variables and proper labels.

We worked on the problem on page 6 together. This one was challenging because we needed to convert kg to g. Students set up their KWS chart and a T chart in their notebooks. I roved the classroom checking progress and fluency.