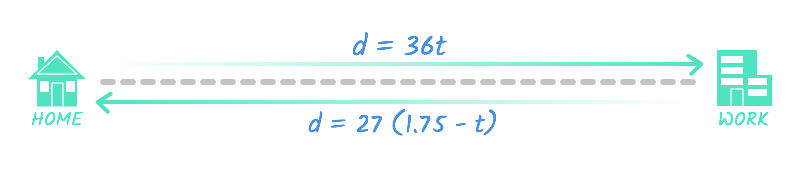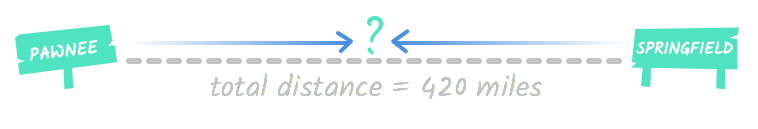Related Pages
Rate Distance Time Word Problems
Distance Problems
Average Speed Problems
What Are Distance Word Problems Or Distance Rate Time Problems?
Distance problems are word problems that involve the distance
an object will travel at a certain average rate for a given
period of time.
The formula for distance problems is:
distance = rate × time or
d = r × t
Things to watch out for:
Make sure that you change the units when necessary. For example, if the rate is given in miles
per hour and the time is given in minutes then change the units appropriately.
It would be helpful to use a table to organize the information for distance problems. A table
helps you to think about one number at a time instead being confused by the question.
The following diagrams give the steps to solve Distance-Rate-Time Problems. Scroll down the page
for examples and solutions.
Distance Problems: Traveling At Different Rates
Example:
A bus traveling at an average rate of 50 kilometers per hour made the trip to town in 6 hours.
If it had traveled at 45 kilometers per hour, how many more minutes would it have taken to make
the trip?
Solution:
Step 1: Set up a rtd table.
| r | t | d | |
| Case 1 | |||
| Case 2 |
Step 2: Fill in the table with information given in the question.
A bus traveling at an average rate of 50 kilometers per hour
made the trip to town in 6 hours. If it had traveled at
45 kilometers per hour, how many more minutes would it
have taken to make the trip?
Let t = time to make the trip in Case 2.
| r | t | d | |
| Case 1 | 50 | 6 | |
| Case 2 | 45 | t |
Step 3: Fill in the values for d using the formula d = rt
| r | t | d | |
| Case 1 | 50 | 6 | 50 × 6 = 300 |
| Case 2 | 45 | t | 45t |
Step 4: Since the distances traveled in both cases are the same,
we get the equation:
45t = 300
Isolate variable t
Step 5: Beware — the
question asked for “how many more minutes would it
have taken to make the trip”, so we need to deduct the original 6 hours taken.
Answer: The time taken would have been 40 minutes longer.
How to solve different types of distance, rate, time problems?
The following examples illustrate three types of problems involving distance, rate and time: opposite directions, same direction and roundtrip.
Example 1:
Lea left home and drove toward the ferry office at an average speed of 22 km.h. Trevon left at the same time and drove in the opposite direction with an average speed of 43 km/h. How long does Trevon need to drive before they are 65 km apart?
Example 2:
Maria left home and traveled toward the capital at an average speed of 80 km/h. Some time later, Imani left traveling in the opposite direction with an average speed of 45 km/h. After Maria has traveled for six hours they were 705 km apart. Find the number of hours Imani traveled.
Example 3:
A passenger train traveled to the repair yards and back. On the trip there, it traveled 68 mph and on the return trip it went 85 mph. How long did the trip there take if the return trip took four hours?
Example 4:
A submarine traveled to St. Vincent and back. It took three hours less time to get there than it did to get back. The average speed on the trip there was 30 km/h. The average speed on the way back was 20 km/h. How many hours did the trip there take?
Example 5:
Micaela left the hardware store and traveled toward her friend’s house at an average speed of 25 km/h. Nicole left some time later traveling in the same direction at the average speed of 30 km/h. After traveling for five hours Nicole caught up with Micaela. How long did Micaela travel before Nicole caught up?
Example 6:
A passenger plane left New York and flew east. A jet left one hour later flying at 280 km/h in a effort to catch up to the passenger plane. After flying for seven hours the jet finally caught up. Find the passenger plane’s average speed.
- Show Video Lesson
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.
Lesson 10: Distance Word Problems
/en/algebra-topics/introduction-to-word-problems/content/
What are distance word problems?
Distance word problems are a common type of algebra word problems. They involve a scenario in which you need to figure out how fast, how far, or how long one or more objects have traveled. These are often called train problems because one of the most famous types of distance problems involves finding out when two trains heading toward each other cross paths.
In this lesson, you’ll learn how to solve train problems and a few other common types of distance problems. But first, let’s look at some basic principles that apply to any distance problem.
The basics of distance problems
There are three basic aspects to movement and travel: distance, rate, and time. To understand the difference among these, think about the last time you drove somewhere.
The distance is how far you traveled. The rate is how fast you traveled. The time is how long the trip took.
The relationship among these things can be described by this formula:
distance = rate x time
d = rt
In other words, the distance you drove is equal to the rate at which you drove times the amount of time you drove. For an example of how this would work in real life, just imagine your last trip was like this:
- You drove 25 miles—that’s the distance.
- You drove an average of 50 mph—that’s the rate.
- The drive took you 30 minutes, or 0.5 hours—that’s the time.
According to the formula, if we multiply the rate and time, the product should be our distance.
And it is! We drove 50 mph for 0.5 hours—and 50 ⋅ 0.5 equals 25, which is our distance.
What if we drove 60 mph instead of 50? How far could we drive in 30 minutes? We could use the same formula to figure this out.
60 ⋅ 0.5 is 30, so our distance would be 30 miles.
Solving distance problems
When you solve any distance problem, you’ll have to do what we just did—use the formula to find distance, rate, or time. Let’s try another simple problem.
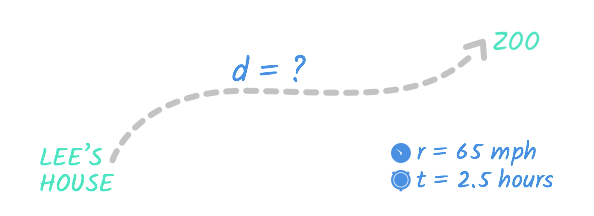
On his day off, Lee took a trip to the zoo. He drove an average speed of 65 mph, and it took him two-and-a-half hours to get from his house to the zoo. How far is the zoo from his house?
First, we should identify the information we know. Remember, we’re looking for any information about distance, rate, or time. According to the problem:
- The rate is 65 mph.
- The time is two-and-a-half hours, or 2.5 hours.
- The distance is unknown—it’s what we’re trying to find.
You could picture Lee’s trip with a diagram like this:
This diagram is a start to understanding this problem, but we still have to figure out what to do with the numbers for distance, rate, and time. To keep track of the information in the problem, we’ll set up a table. (This might seem excessive now, but it’s a good habit for even simple problems and can make solving complicated problems much easier.) Here’s what our table looks like:
| distance | rate | time |
|---|---|---|
| d | 65 | 2.5 |
We can put this information into our formula: distance = rate ⋅ time.
We can use the distance = rate ⋅ time formula to find the distance Lee traveled.
d = rt
The formula d = rt looks like this when we plug in the numbers from the problem. The unknown distance is represented with the variable d.
d = 65 ⋅ 2.5
To find d, all we have to do is multiply 65 and 2.5. 65 ⋅ 2.5 equals 162.5.
d = 162.5
We have an answer to our problem: d = 162.5. In other words, the distance Lee drove from his house to the zoo is 162.5 miles.
Be careful to use the same units of measurement for rate and time. It’s possible to multiply 65 miles per hour by 2.5 hours because they use the same unit: an hour. However, what if the time had been written in a different unit, like in minutes? In that case, you’d have to convert the time into hours so it would use the same unit as the rate.
Solving for rate and time
In the problem we just solved we calculated for distance, but you can use the d = rt formula to solve for rate and time too. For example, take a look at this problem:
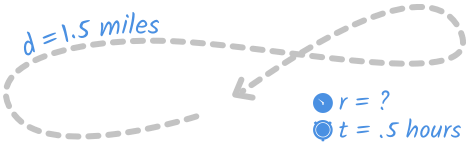
After work, Janae walked in her neighborhood for a half hour. She walked a mile-and-a-half total. What was her average speed in miles per hour?
We can picture Janae’s walk as something like this:
And we can set up the information from the problem we know like this:
| distance | rate | time |
|---|---|---|
| 1.5 | r | 0.5 |
The table is repeating the facts we already know from the problem. Janae walked one-and-a-half miles or 1.5 miles in a half hour, or 0.5 hours.
As always, we start with our formula. Next, we’ll fill in the formula with the information from our table.
d = rt
The rate is represented by r because we don’t yet know how fast Janae was walking. Since we’re solving for r, we’ll have to get it alone on one side of the equation.
1.5 = r ⋅ 0.5
Our equation calls for r to be multiplied by 0.5, so we can get r alone on one side of the equation by dividing both sides by 0.5:
1.5 / 0.5 = 3.
3 = r
r = 3, so 3 is the answer to our problem. Janae walked 3 miles per hour.
In the problems on this page, we solved for distance and rate of travel, but you can also use the travel equation to solve for time. You can even use it to solve certain problems where you’re trying to figure out the distance, rate, or time of two or more moving objects. We’ll look at problems like this on the next few pages.
Two-part and round-trip problems
Do you know how to solve this problem?
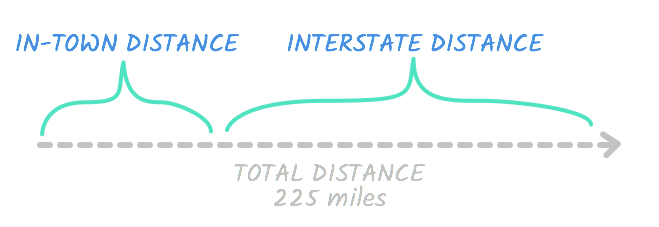
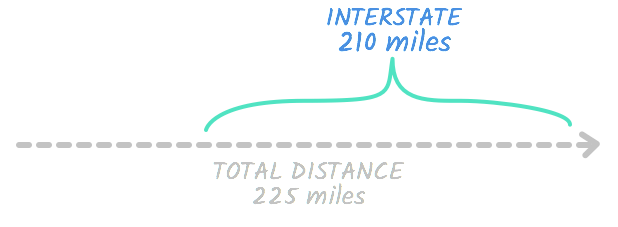
Bill took a trip to see a friend. His friend lives 225 miles away. He drove in town at an average of 30 mph, then he drove on the interstate at an average of 70 mph. The trip took three-and-a-half hours total. How far did Bill drive on the interstate?
This problem is a classic two-part trip problem because it’s asking you to find information about one part of a two-part trip. This problem might seem complicated, but don’t be intimidated!
You can solve it using the same tools we used to solve the simpler problems on the first page:
- The travel equation d = rt
- A table to keep track of important information
Let’s start with the table. Take another look at the problem. This time, the information relating to distance, rate, and time has been underlined.
Bill took a trip to see a friend. His friend lives 225 miles away. He drove in town at an average of 30 mph, then he drove on the interstate at an average of 70 mph. The trip took three-and-a-half hours total. How far did Bill drive on the interstate?
If you tried to fill in the table the way we did on the last page, you might have noticed a problem: There’s too much information. For instance, the problem contains two rates—30 mph and 70 mph. To include all of this information, let’s create a table with an extra row. The top row of numbers and variables will be labeled in town, and the bottom row will be labeled interstate.
| distance | rate | time | |
|---|---|---|---|
| in town | 30 | ||
| interstate | 70 |
We filled in the rates, but what about the distance and time? If you look back at the problem, you’ll see that these are the total figures, meaning they include both the time in town and on the interstate. So the total distance is 225. This means this is true:
Interstate distance + in-town distance = Total distance
Together, the interstate distance and in-town distance are equal to the total distance. See?
In any case, we’re trying to find out how far Bill drove on the interstate, so let’s represent this number with d. If the interstate distance is d, it means the in-town distance is a number that equals the total, 225, when added to d. In other words, it’s equal to 225 — d.
We can fill in our chart like this:
| distance | rate | time | |
|---|---|---|---|
| in town | 225 — d | 30 | |
| interstate | d | 70 |
We can use the same technique to fill in the time column. The total time is 3.5 hours. If we say the time on the interstate is t, then the remaining time in town is equal to 3.5 — t. We can fill in the rest of our chart.
| distance | rate | time | |
|---|---|---|---|
| in town | 225 — d | 30 | 3.5 — t |
| interstate | d | 70 | t |
Now we can work on solving the problem. The main difference between the problems on the first page and this problem is that this problem involves two equations. Here’s the one for in-town travel:
225 — d = 30 ⋅ (3.5 — t)
And here’s the one for interstate travel:
d = 70t
If you tried to solve either of these on its own, you might have found it impossible: since each equation contains two unknown variables, they can’t be solved on their own. Try for yourself. If you work either equation on its own, you won’t be able to find a numerical value for d. In order to find the value of d, we’ll also have to know the value of t.
We can find the value of t in both problems by combining them. Let’s take another look at our travel equation for interstate travel.
While we don’t know the numerical value of d, this equation does tell us that d is equal to 70t.
d = 70t
Since 70t and d are equal, we can replace d with 70t. Substituting 70t for d in our equation for interstate travel won’t help us find the value of t—all it tells us is that 70t is equal to itself, which we already knew.
70t = 70t
But what about our other equation, the one for in-town travel?
225 — d = 30 ⋅ (3.5 — t)
When we replace the d in that equation with 70t, the equation suddenly gets much easier to solve.
225 — 70t = 30 ⋅ (3.5 — t)
Our new equation might look more complicated, but it’s actually something we can solve. This is because it only has one variable: t. Once we find t, we can use it to calculate the value of d—and find the answer to our problem.
To simplify this equation and find the value of t, we’ll have to get the t alone on one side of the equals sign. We’ll also have to simplify the right side as much as possible.
225 — 70t = 30 ⋅ (3.5 — t)
Let’s start with the right side: 30 times (3.5 — t) is 105 — 30t.
225 — 70t = 105 — 30t
Next, let’s cancel out the 225 next to 70t. To do this, we’ll subtract 225 from both sides. On the right side, it means subtracting 225 from 105. 105 — 225 is -120.
— 70t = -120 — 30t
Our next step is to group like terms—remember, our eventual goal is to have t on the left side of the equals sign and a number on the right. We’ll cancel out the -30t on the right side by adding 30t to both sides. On the right side, we’ll add it to -70t. -70t + 30t is -40t.
— 40t = -120
Finally, to get t on its own, we’ll divide each side by its coefficient: -40. -120 / — 40 is 3.
t = 3
So t is equal to 3. In other words, the time Bill traveled on the interstate is equal to 3 hours. Remember, we’re ultimately trying to find the distance Bill traveled on the interstate. Let’s look at the interstate row of our chart again and see if we have enough information to find out.
| distance | rate | time | |
|---|---|---|---|
| interstate | d | 70 | 3 |
It looks like we do. Now that we’re only missing one variable, we should be able to find its value pretty quickly.
To find the distance, we’ll use the travel formula distance = rate ⋅ time.
d = rt
We now know that Bill traveled on the interstate for 3 hours at 70 mph, so we can fill in this information.
d = 3 ⋅ 70
Finally, we finished simplifying the right side of the equation. 3 ⋅ 70 is 210.
d = 210
So d = 210. We have the answer to our problem! The distance is 210. In other words, Bill drove 210 miles on the interstate.
Solving a round-trip problem
It might have seemed like it took a long time to solve the first problem. The more practice you get with these problems, the quicker they’ll go. Let’s try a similar problem. This one is called a round-trip problem because it describes a round trip—a trip that includes a return journey. Even though the trip described in this problem is slightly different from the one in our first problem, you should be able to solve it the same way. Let’s take a look:
Eva drove to work at an average speed of 36 mph. On the way home, she hit traffic and only drove an average of 27 mph. Her total time in the car was 1 hour and 45 minutes, or 1.75 hours. How far does Eva live from work?
If you’re having trouble understanding this problem, you might want to visualize Eva’s commute like this:
As always, let’s start by filling in a table with the important information. We’ll make a row with information about her trip to work and from work.
1.75 — t to describe the trip from work. (Remember, the total travel time is 1.75 hours, so the time to work and from work should equal 1.75.)
From our table, we can write two equations:
- The trip to work can be represented as d = 36t.
- The trip from work can be represented as d = 27 (1.75 — t).
In both equations, d represents the total distance. From the diagram, you can see that these two equations are equal to each other—after all, Eva drives the same distance to and from work.
Just like with the last problem we solved, we can solve this one by combining the two equations.
We’ll start with our equation for the trip from work.
d = 27 (1.75 — t)
Next, we’ll substitute in the value of d from our to work equation, d = 36t. Since the value of d is 36t, we can replace any occurrence of d with 36t.
36t = 27 (1.75 — t)
Now, let’s simplify the right side. 27 ⋅(1.75 — t) is 47.25.
36t = 47.25 — 27t
Next, we’ll cancel out -27t by adding 27t to both sides of the equation. 36t + 27t is 63t.
63t = 47.25
Finally, we can get t on its own by dividing both sides by its coefficient: 63. 47.25 / 63 is .75.
t = .75
t is equal to .75. In other words, the time it took Eva to drive to work is .75 hours. Now that we know the value of t, we’ll be able to can find the distance to Eva’s work.
If you guessed that we were going to use the travel equation again, you were right. We now know the value of two out of the three variables, which means we know enough to solve our problem.
d = rt
First, let’s fill in the values we know. We’ll work with the numbers for the trip to work. We already knew the rate: 36. And we just learned the time: .75.
d = 36 ⋅ .75
Now all we have to do is simplify the equation: 36 ⋅ .75 = 27.
d = 27
d is equal to 27. In other words, the distance to Eva’s work is 27 miles. Our problem is solved.
Intersecting distance problems
An intersecting distance problem is one where two things are moving toward each other. Here’s a typical problem:
Pawnee and Springfield are 420 miles apart. A train leaves Pawnee heading to Springfield at the same time a train leaves Springfield heading to Pawnee. One train is moving at a speed of 45 mph, and the other is moving 60 mph. How long will they travel before they meet?
This problem is asking you to calculate how long it will take these two trains moving toward each other to cross paths. This might seem confusing at first. Even though it’s a real-world situation, it can be difficult to imagine distance and motion abstractly. This diagram might help you get a sense of what this situation looks like:
If you’re still confused, don’t worry! You can solve this problem the same way you solved the two-part problems on the last page. You’ll just need a chart and the travel formula.
Pawnee and Springfield are 420 miles apart. A train leaves Pawnee heading toward Springfield at the same time a train leaves Springfield heading toward Pawnee. One train is moving at a speed of 45 mph, and the other is moving 60 mph. How long will they travel before they meet?
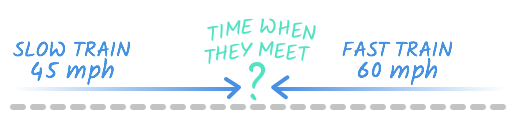
Let’s start by filling in our chart. Here’s the problem again, this time with the important information underlined. We can start by filling in the most obvious information: rate. The problem gives us the speed of each train. We’ll label them fast train and slow train. The fast train goes 60 mph. The slow train goes only 45 mph.
We can also put this information into a table:
| distance | rate | time | |
|---|---|---|---|
| fast train | 60 | ||
| slow train | 45 |
We don’t know the distance each train travels to meet the other yet—we just know the total distance. In order to meet, the trains will cover a combined distance equal to the total distance. As you can see in this diagram, this is true no matter how far each train travels.
This means that—just like last time—we’ll represent the distance of one with d and the distance of the other with the total minus d. So the distance for the fast train will be d, and the distance for the slow train will be 420 — d.
| distance | rate | time | |
|---|---|---|---|
| fast train | d | 60 | |
| slow train | 420 — d | 45 |
Because we’re looking for the time both trains travel before they meet, the time will be the same for both trains. We can represent it with t.
| distance | rate | time | |
|---|---|---|---|
| fast train | d | 60 | t |
| slow train | 420 — d | 45 | t |
The table gives us two equations: d = 60t and 420 — d = 45t. Just like we did with the two-part problems, we can combine these two equations.
The equation for the fast train isn’t solvable on its own, but it does tell us that d is equal to 60t.
d = 60t
The other equation, which describes the slow train, can’t be solved alone either. However, we can replace the d with its value from the first equation.
420 — d = 45t
Because we know that d is equal to 60t, we can replace the d in this equation with 60t. Now we have an equation we can solve.
420 — 60t = 45t
To solve this equation, we’ll need to get t and its coefficients on one side of the equals sign and any other numbers on the other. We can start by canceling out the -60t on the left by adding 60t to both sides. 45t + 60t is 105t.
420 = 105t
Now we just need to get rid of the coefficient next to t. We can do this by dividing both sides by 105. 420 / 105 is 4.
4 = t
t = 4. In other words, the time it takes the trains to meet is 4 hours. Our problem is solved!
If you want to be sure of your answer, you can check it by using the distance equation with t equal to 4. For our fast train, the equation would be d = 60 ⋅ 4. 60 ⋅ 4 is 240, so the distance our fast train traveled would be 240 miles. For our slow train, the equation would be d = 45 ⋅ 4. 45 ⋅ 4 is 180, so the distance traveled by the slow train is 180 miles.
Remember how we said the distance the slow train and fast train travel should equal the total distance? 240 miles + 180 miles equals 420 miles, which is the total distance from our problem. Our answer is correct.
Practice problem 1
Here’s another intersecting distance problem. It’s similar to the one we just solved. See if you can solve it on your own. When you’re finished, scroll down to see the answer and an explanation.
Jon and Dani live 270 miles apart. One day, they decided to drive toward each other and hang out wherever they met. Jon drove an average of 65 mph, and Dani drove an average of 70 mph. How long did they drive before they met up?
Problem 1 answer
Here’s practice problem 1:
Jon and Dani live 270 miles apart. One day, they decided to drive toward each other and hang out wherever they met. Jon drove an average of 65 mph, and Dani drove 70 mph. How long did they drive before they met up?
Answer: 2 hours.
Let’s solve this problem like we solved the others. First, try making the chart. It should look like this:
| distance | rate | time | |
|---|---|---|---|
| Jon | d | 65 | t |
| Dani | 270 — d | 70 | t |
Here’s how we filled in the chart:
- Distance: Together, Dani and Jon will have covered the total distance between them by the time they meet up. That’s 270. Jon’s distance is represented by d, so Dani’s distance is 270 — d.
- Rate: The problem tells us Dani and Jon’s speeds. Dani drives 65 mph, and Jon drives 70 mph.
- Time: Because Jon and Dani drive the same amount of time before they meet up, both of their travel times are represented by t.
Now we have two equations. The equation for Jon’s travel is d = 65t. The equation for Dani’s travel is 270 — d = 70t. To solve this problem, we’ll need to combine them.
The equation for Jon tells us that d is equal to 65t. This means we can combine the two equations by replacing the d in Dani’s equation with 65t.
270 — 65t = 70t
Let’s get t on one side of the equation and a number on the other. The first step to doing this is to get rid of -65t on the left side. We’ll cancel it out by adding 65t to both sides: 70t + 65t is 135t.
270 = 135t
All that’s left to do is to get rid of the 135 next to the t. We can do this by dividing both sides by 135: 270 / 135 is 2.
2 = t
That’s it. t is equal to 2. We have the answer to our problem: Dani and Jon drove 2 hours before they met up.
Overtaking distance problems
The final type of distance problem we’ll discuss in this lesson is a problem in which one moving object overtakes—or passes—another. Here’s a typical overtaking problem:
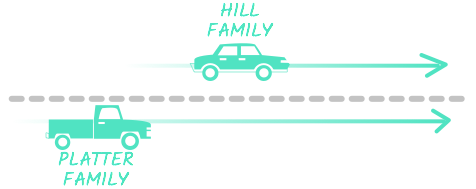
The Hill family and the Platter family are going on a road trip. The Hills left 3 hours before the Platters, but the Platters drive an average of 15 mph faster. If it takes the Platter family 13 hours to catch up with the Hill family, how fast are the Hills driving?
You can picture the moment the Platter family left for the road trip a little like this:
The problem tells us that the Platter family will catch up with the Hill family in 13 hours and asks us to use this information to find the Hill family’s rate. Like some of the other problems we’ve solved in this lesson, it might not seem like we have enough information to solve this problem—but we do. Let’s start making our chart. The distance can be d for both the Hills and the Platters—when the Platters catch up with the Hills, both families will have driven the exact same distance.
| distance | rate | time | |
|---|---|---|---|
| the Hills | d | ||
| the Platters | d |
Filling in the rate and time will require a little more thought. We don’t know the rate for either family—remember, that’s what we’re trying to find out. However, we do know that the Platters drove 15 mph faster than the Hills. This means if the Hill family’s rate is r, the Platter family’s rate would be r + 15.
| distance | rate | time | |
|---|---|---|---|
| the Hills | d | r | |
| the Platters | d | r + 15 |
Now all that’s left is the time. We know it took the Platters 13 hours to catch up with the Hills. However, remember that the Hills left 3 hours earlier than the Platters—which means when the Platters caught up, they’d been driving 3 hours more than the Platters. 13 + 3 is 16, so we know the Hills had been driving 16 hours by the time the Platters caught up with them.
| distance | rate | time | |
|---|---|---|---|
| the Hills | d | r | 16 |
| the Platters | d | r + 15 | 13 |
Our chart gives us two equations. The Hill family’s trip can be described by d = r ⋅ 16. The equation for the Platter family’s trip is d = (r + 15) ⋅ 13. Just like with our other problems, we can combine these equations by replacing a variable in one of them.
The Hill family equation already has the value of d equal to r ⋅ 16. So we’ll replace the d in the Platter equation with r ⋅ 16. This way, it will be an equation we can solve.
r ⋅ 16 = (r + 15) ⋅ 13
First, let’s simplify the right side: r ⋅ 16 is 16r.
16r = (r + 15) ⋅ 13
Next, we’ll simplify the right side and multiply (r + 15) by 13.
16r = 13r + 195
We can get both r and their coefficients on the left side by subtracting 13r from 16r : 16r — 13r is 3r.
3r = 195
Now all that’s left to do is get rid of the 3 next to the r. To do this, we’ll divide both sides by 3: 195 / 3 is 65.
r = 65
So there’s our answer: r = 65. The Hill family drove an average of 65 mph.
You can solve any overtaking problem the same way we solved this one. Just remember to pay special attention when you’re setting up your chart. Just like the Hill family did in this problem, the person or vehicle who started moving first will always have a greater travel time.
Practice problem 2
Try solving this problem. It’s similar to the problem we just solved. When you’re finished, scroll down to see the answer and an explanation.
A train moving 60 mph leaves the station at noon. An hour later, a train moving 80 mph leaves heading the same direction on a parallel track. What time does the second train catch up to the first?
Problem 2 answer
Here’s practice problem 2:
A train moving 60 mph leaves the station at noon. An hour later, a train moving 80 mph leaves heading the same direction on a parallel track. What time does the second train catch up to the first?
Answer: 4 p.m.
To solve this problem, start by making a chart. Here’s how it should look:
| distance | rate | time | |
|---|---|---|---|
| fast train | d | 80 | t |
| slow train | d | 60 | t + 1 |
Here’s an explanation of the chart:
- Distance: Both trains will have traveled the same distance by the time the fast train catches up with the slow one, so the distance for both is d.
- Rate: The problem tells us how fast each train was going. The fast train has a rate of 80 mph, and the slow train has a rate of 60 mph.
- Time: We’ll use t to represent the fast train’s travel time before it catches up. Because the slow train started an hour before the fast one, it will have been traveling one hour more by the time the fast train catches up. It’s t + 1.
Now we have two equations. The equation for the fast train is d = 80t. The equation for the slow train is d = 60 (t + 1). To solve this problem, we’ll need to combine the equations.
The equation for the fast train says d is equal to 80t. This means we can combine the two equations by replacing the d in the slow train’s equation with 80t.
80t = 60 (t + 1)
First, let’s simplify the right side of the equation: 60 ⋅ (t + 1) is 60t + 60.
80t = 60t + 60
To solve the equation, we’ll have to get t on one side of the equals sign and a number on the other. We can get rid of 60t on the right side by subtracting 60t from both sides: 80t — 60t is 20t.
20t = 60
Finally, we can get rid of the 20 next to t by dividing both sides by 20. 60 divided by 20 is 3.
t = 3
So t is equal to 3. The fast train traveled for 3 hours. However, it’s not the answer to our problem. Let’s look at the original problem again. Pay attention to the last sentence, which is the question we’re trying to answer.
A train moving 60 mph leaves the station at noon. An hour later, a train moving 80 mph leaves heading the same direction on a parallel track. What time does the second train catch up to the first?
Our problem doesn’t ask how long either of the trains traveled. It asks what time the second train catches up with the first.
The problem tells us that the slow train left at noon and the fast one left an hour later. This means the fast train left at 1 p.m. From our equations, we know the fast train traveled 3 hours. 1 + 3 is 4, so the fast train caught up with the slow one at 4 p.m. The answer to the problem is 4 p.m.
Before you get into distance, time and speed word problems, take a few minutes to read this first and understand: How to build your credit score in USA as an international student.
Problems involving Time, Distance and Speed are solved based on one simple formula.
Distance = Speed * Time
Which implies →
Speed = Distance / Time and
Time = Distance / Speed
Let us take a look at some simple examples of distance, time and speed problems.
Example 1. A boy walks at a speed of 4 kmph. How much time does he take to walk a distance of 20 km?
Solution
Time = Distance / speed = 20/4 = 5 hours.
Example 2. A cyclist covers a distance of 15 miles in 2 hours. Calculate his speed.
Solution
Speed = Distance/time = 15/2 = 7.5 miles per hour.
Example 3. A car takes 4 hours to cover a distance, if it travels at a speed of 40 mph. What should be its speed to cover the same distance in 1.5 hours?
Solution
Distance covered = 4*40 = 160 miles
Speed required to cover the same distance in 1.5 hours = 160/1.5 = 106.66 mph
Now, take a look at the following example:
Example 4. If a person walks at 4 mph, he covers a certain distance. If he walks at 9 mph, he covers 7.5 miles more. How much distance did he actually cover?
Now we can see that the direct application of our usual formula Distance = Speed * Time or its variations cannot be done in this case and we need to put in extra effort to calculate the given parameters.
Let us see how this question can be solved.
Solution
For these kinds of questions, a table like this might make it easier to solve.
| Distance | Speed | Time |
| d | 4 | t |
| d+7.5 | 9 | t |
Let the distance covered by that person be ‘d’.
Walking at 4 mph and covering a distance ‘d’ is done in a time of ‘d/4’
IF he walks at 9 mph, he covers 7.5 miles more than the actual distance d, which is ‘d+7.5’.
He does this in a time of (d+7.5)/9.
Since the time is same in both the cases →
d/4 = (d+7.5)/9 → 9d = 4(d+7.5) → 9d=4d+30 → d = 6.
So, he covered a distance of 6 miles in 1.5 hours.
Example 5. A train is going at 1/3 of its usual speed and it takes an extra 30 minutes to reach its destination. Find its usual time to cover the same distance.
Solution
Here, we see that the distance is same.
Let us assume that its usual speed is ‘s’ and time is ‘t’, then
| Distance | Speed | Time |
| d | s | t min |
| d | S+1/3 | t+30 min |
s*t = (1/3)s*(t+30) → t = t/3 + 10 → t = 15.
So the actual time taken to cover the distance is 15 minutes.
Note: Note the time is expressed in terms of ‘minutes’. When we express distance in terms of miles or kilometers, time is expressed in terms of hours and has to be converted into appropriate units of measurement.
Solved Questions on Trains
Example 1. X and Y are two stations which are 320 miles apart. A train starts at a certain time from X and travels towards Y at 70 mph. After 2 hours, another train starts from Y and travels towards X at 20 mph. At what time do they meet?
Solution
Let the time after which they meet be ‘t’ hours.
Then the time travelled by second train becomes ‘t-2’.
Now,
Distance covered by first train+Distance covered by second train = 320 miles
70t+20(t-2) = 320
Solving this gives t = 4.
So the two trains meet after 4 hours.
Example 2. A train leaves from a station and moves at a certain speed. After 2 hours, another train leaves from the same station and moves in the same direction at a speed of 60 mph. If it catches up with the first train in 4 hours, what is the speed of the first train?
Solution
Let the speed of the first train be ‘s’.
Distance covered by the first train in (2+4) hours = Distance covered by second train in 4 hours
Therefore, 6s = 60*4
Solving which gives s=40.
So the slower train is moving at the rate of 40 mph.
Questions on Boats/Airplanes
For problems with boats and streams,
Speed of the boat upstream (against the current) = Speed of the boat in still water – speed of the stream
[As the stream obstructs the speed of the boat in still water, its speed has to be subtracted from the usual speed of the boat]
Speed of the boat downstream (along with the current) = Speed of the boat in still water + speed of the stream
[As the stream pushes the boat and makes it easier for the boat to reach the destination faster, speed of the stream has to be added]
Similarly, for airplanes travelling with/against the wind,
Speed of the plane with the wind = speed of the plane + speed of the wind
Speed of the plane against the wind = speed of the plane – speed of the wind
Let us look at some examples.
Example 1. A man travels at 3 mph in still water. If the current’s velocity is 1 mph, it takes 3 hours to row to a place and come back. How far is the place?
Solution
Let the distance be ‘d’ miles.
Time taken to cover the distance upstream + Time taken to cover the distance downstream = 3
Speed upstream = 3-1 = 2 mph
Speed downstream = 3+1 = 4 mph
So, our equation would be d/2 + d/4 = 3 → solving which, we get d = 4 miles.
Example 2. With the wind, an airplane covers a distance of 2400 kms in 4 hours and against the wind in 6 hours. What is the speed of the plane and that of the wind?
Solution
Let the speed of the plane be ‘a’ and that of the wind be ‘w’.
Our table looks like this:
| Distance | Speed | Time | |
| With the wind | 2400 | a+w | 4 |
| Against the wind | 2400 | a-w | 6 |
4(a+w) = 2400 and 6(a-w) = 2400
Expressing one unknown variable in terms of the other makes it easier to solve, which means
a+w = 600 → w=600-a
Substituting the value of w in the second equation,
a-w = 400
a-(600-a) = 400 → a = 500
The speed of the plane is 500 kmph and that of the wind is 100 kmph.
More solved examples on Speed, Distance and Time
Example 1. A boy travelled by train which moved at the speed of 30 mph. He then boarded a bus which moved at the speed of 40 mph and reached his destination. The entire distance covered was 100 miles and the entire duration of the journey was 3 hours. Find the distance he travelled by bus.
Solution
| Distance | Speed | Time | |
| Train | d | 30 | t |
| Bus | 100-d | 40 | 3-t |
Let the time taken by the train be ‘t’. Then that of bus is ‘3-t’.
The entire distance covered was 100 miles
So, 30t + 40(3-t) = 100
Solving which gives t=2.
Substituting the value of t in 40(3-t), we get the distance travelled by bus is 40 miles.
Alternatively, we can add the time and equate it to 3 hours, which directly gives the distance.
d/30 + (100-d)/40 = 3
Solving which gives d = 60, which is the distance travelled by train. 100-60 = 40 miles is the distance travelled by bus.
Example 2. A plane covered a distance of 630 miles in 6 hours. For the first part of the trip, the average speed was 100 mph and for the second part of the trip, the average speed was 110 mph. what is the time it flew at each speed?
Solution
Our table looks like this.
| Distance | Speed | Time | |
| 1st part of journey | d | 100 | t |
| 2nd part of journey | 630-d | 110 | 6-t |
Assuming the distance covered in the 1st part of journey to be ‘d’, the distance covered in the second half becomes ‘630-d’.
Assuming the time taken for the first part of the journey to be ‘t’, the time taken for the second half becomes ‘6-t’.
From the first equation, d=100t
The second equation is 630-d = 110(6-t).
Substituting the value of d from the first equation, we get
630-100t = 110(6-t)
Solving this gives t=3.
So the plane flew the first part of the journey in 3 hours and the second part in 3 hours.
Example 2. Two persons are walking towards each other on a walking path that is 20 miles long. One is walking at the rate of 3 mph and the other at 4 mph. After how much time will they meet each other?
Solution
| Distance | Speed | Time | |
| First person | d | 3 | t |
| Second person | 20-d | 4 | t |
Assuming the distance travelled by the first person to be ‘d’, the distance travelled by the second person is ’20-d’.
The time is ‘t’ for both of them because when they meet, they would have walked for the same time.
Since time is same, we can equate as
d/3 = (20-d)/4
Solving this gives d=60/7 miles (8.5 miles approximately)
Then t = 20/7 hours
So the two persons meet after 2 6/7 hours.
Practice Questions for you to solve
Problem 1: Click here
Answer 1: Click here
Problem 2: Click here
Answer 2: Click here
Chapter 8: Rational Expressions
8.8 Rate Word Problems: Speed, Distance and Time
Distance, rate and time problems are a standard application of linear equations. When solving these problems, use the relationship rate (speed or velocity) times time equals distance.
[latex]rcdot t=d[/latex]
For example, suppose a person were to travel 30 km/h for 4 h. To find the total distance, multiply rate times time or (30km/h)(4h) = 120 km.
The problems to be solved here will have a few more steps than described above. So to keep the information in the problem organized, use a table. An example of the basic structure of the table is below:
| Who or What | Rate | Time | Distance |
|---|---|---|---|
The third column, distance, will always be filled in by multiplying the rate and time columns together. If given a total distance of both persons or trips, put this information in the distance column. Now use this table to set up and solve the following examples.
Joey and Natasha start from the same point and walk in opposite directions. Joey walks 2 km/h faster than Natasha. After 3 hours, they are 30 kilometres apart. How fast did each walk?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Natasha | [latex]r[/latex] | [latex]text{3 h}[/latex] | [latex]text{3 h}(r)[/latex] |
| Joey | [latex]r + 2[/latex] | [latex]text{3 h}[/latex] | [latex]text{3 h}(r + 2)[/latex] |
The distance travelled by both is 30 km. Therefore, the equation to be solved is:
[latex]begin{array}{rrrrrrl} 3r&+&3(r&+&2)&=&30 \ 3r&+&3r&+&6&=&30 \ &&&-&6&&-6 \ hline &&&&dfrac{6r}{6}&=&dfrac{24}{6} \ \ &&&&r&=&4 text{ km/h} end{array}[/latex]
This means that Natasha walks at 4 km/h and Joey walks at 6 km/h.
Nick and Chloe left their campsite by canoe and paddled downstream at an average speed of 12 km/h. They turned around and paddled back upstream at an average rate of 4 km/h. The total trip took 1 hour. After how much time did the campers turn around downstream?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Downstream | [latex]text{12 km/h}[/latex] | [latex]t[/latex] | [latex]text{12 km/h } (t)[/latex] |
| Upstream | [latex]text{4 km/h}[/latex] | [latex](1 — t)[/latex] | [latex]text{4 km/h } (1 — t)[/latex] |
The distance travelled downstream is the same distance that they travelled upstream. Therefore, the equation to be solved is:
[latex]begin{array}{rrlll} 12(t)&=&4(1&-&t) \ 12t&=&4&-&4t \ +4t&&&+&4t \ hline dfrac{16t}{16}&=&dfrac{4}{16}&& \ \ t&=&0.25&& end{array}[/latex]
This means the campers paddled downstream for 0.25 h and spent 0.75 h paddling back.
Terry leaves his house riding a bike at 20 km/h. Sally leaves 6 h later on a scooter to catch up with him travelling at 80 km/h. How long will it take her to catch up with him?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Terry | [latex]text{20 km/h}[/latex] | [latex]t[/latex] | [latex]text{20 km/h }(t)[/latex] |
| Sally | [latex]text{80 km/h}[/latex] | [latex](t — text{6 h})[/latex] | [latex]text{80 km/h }(t — text {6 h})[/latex] |
The distance travelled by both is the same. Therefore, the equation to be solved is:
[latex]begin{array}{rrrrr} 20(t)&=&80(t&-&6) \ 20t&=&80t&-&480 \ -80t&&-80t&& \ hline dfrac{-60t}{-60}&=&dfrac{-480}{-60}&& \ \ t&=&8&& end{array}[/latex]
This means that Terry travels for 8 h and Sally only needs 2 h to catch up to him.
On a 130-kilometre trip, a car travelled at an average speed of 55 km/h and then reduced its speed to 40 km/h for the remainder of the trip. The trip took 2.5 h. For how long did the car travel 40 km/h?
| Who or What | Rate | Time | Distance |
|---|---|---|---|
| Fifty-five | [latex]text{55 km/h}[/latex] | [latex]t[/latex] | [latex]text{55 km/h }(t)[/latex] |
| Forty | [latex]text{40 km/h}[/latex] | [latex](text{2.5 h}-t)[/latex] | [latex]text{40 km/h }(text{2.5 h}-t)[/latex] |
The distance travelled by both is 30 km. Therefore, the equation to be solved is:
[latex]begin{array}{rrrrrrr} 55(t)&+&40(2.5&-&t)&=&130 \ 55t&+&100&-&40t&=&130 \ &-&100&&&&-100 \ hline &&&&dfrac{15t}{15}&=&dfrac{30}{15} \ \ &&&&t&=&2 end{array}[/latex]
This means that the time spent travelling at 40 km/h was 0.5 h.
Distance, time and rate problems have a few variations that mix the unknowns between distance, rate and time. They generally involve solving a problem that uses the combined distance travelled to equal some distance or a problem in which the distances travelled by both parties is the same. These distance, rate and time problems will be revisited later on in this textbook where quadratic solutions are required to solve them.
Questions
For Questions 1 to 8, find the equations needed to solve the problems. Do not solve.
- A is 60 kilometres from B. An automobile at A starts for B at the rate of 20 km/h at the same time that an automobile at B starts for A at the rate of 25 km/h. How long will it be before the automobiles meet?
- Two automobiles are 276 kilometres apart and start to travel toward each other at the same time. They travel at rates differing by 5 km/h. If they meet after 6 h, find the rate of each.
- Two trains starting at the same station head in opposite directions. They travel at the rates of 25 and 40 km/h, respectively. If they start at the same time, how soon will they be 195 kilometres apart?
- Two bike messengers, Jerry and Susan, ride in opposite directions. If Jerry rides at the rate of 20 km/h, at what rate must Susan ride if they are 150 kilometres apart in 5 hours?
- A passenger and a freight train start toward each other at the same time from two points 300 kilometres apart. If the rate of the passenger train exceeds the rate of the freight train by 15 km/h, and they meet after 4 hours, what must the rate of each be?
- Two automobiles started travelling in opposite directions at the same time from the same point. Their rates were 25 and 35 km/h, respectively. After how many hours were they 180 kilometres apart?
- A man having ten hours at his disposal made an excursion by bike, riding out at the rate of 10 km/h and returning on foot at the rate of 3 km/h. Find the distance he rode.
- A man walks at the rate of 4 km/h. How far can he walk into the country and ride back on a trolley that travels at the rate of 20 km/h, if he must be back home 3 hours from the time he started?
Solve Questions 9 to 22.
- A boy rides away from home in an automobile at the rate of 28 km/h and walks back at the rate of 4 km/h. The round trip requires 2 hours. How far does he ride?
- A motorboat leaves a harbour and travels at an average speed of 15 km/h toward an island. The average speed on the return trip was 10 km/h. How far was the island from the harbour if the trip took a total of 5 hours?
- A family drove to a resort at an average speed of 30 km/h and later returned over the same road at an average speed of 50 km/h. Find the distance to the resort if the total driving time was 8 hours.
- As part of his flight training, a student pilot was required to fly to an airport and then return. The average speed to the airport was 90 km/h, and the average speed returning was 120 km/h. Find the distance between the two airports if the total flying time was 7 hours.
- Sam starts travelling at 4 km/h from a campsite 2 hours ahead of Sue, who travels 6 km/h in the same direction. How many hours will it take for Sue to catch up to Sam?
- A man travels 5 km/h. After travelling for 6 hours, another man starts at the same place as the first man did, following at the rate of 8 km/h. When will the second man overtake the first?
- A motorboat leaves a harbour and travels at an average speed of 8 km/h toward a small island. Two hours later, a cabin cruiser leaves the same harbour and travels at an average speed of 16 km/h toward the same island. How many hours after the cabin cruiser leaves will it be alongside the motorboat?
- A long distance runner started on a course, running at an average speed of 6 km/h. One hour later, a second runner began the same course at an average speed of 8 km/h. How long after the second runner started will they overtake the first runner?
- Two men are travelling in opposite directions at the rate of 20 and 30 km/h at the same time and from the same place. In how many hours will they be 300 kilometres apart?
- Two trains start at the same time from the same place and travel in opposite directions. If the rate of one is 6 km/h more than the rate of the other and they are 168 kilometres apart at the end of 4 hours, what is the rate of each?
- Two cyclists start from the same point and ride in opposite directions. One cyclist rides twice as fast as the other. In three hours, they are 72 kilometres apart. Find the rate of each cyclist.
- Two small planes start from the same point and fly in opposite directions. The first plane is flying 25 km/h slower than the second plane. In two hours, the planes are 430 kilometres apart. Find the rate of each plane.
- On a 130-kilometre trip, a car travelled at an average speed of 55 km/h and then reduced its speed to 40 km/h for the remainder of the trip. The trip took a total of 2.5 hours. For how long did the car travel at 40 km/h?
- Running at an average rate of 8 m/s, a sprinter ran to the end of a track and then jogged back to the starting point at an average of 3 m/s. The sprinter took 55 s to run to the end of the track and jog back. Find the length of the track.
Answer Key 8.8
A 12 min read
GMAT Word problems on Time, and Speed, and Distance are based on the simple formula of D = S x T, where D is the distance, S is speed and T is time. These GMAT word problems might look easy at first but if your concepts are not clear you will end up cringing in front of the computer screen. You’ll end up wasting valuable time as well as your morale. Therefore, it’s essential you understand how to easily solve Distance Speed Time Problems. This article cites the importance of average speed and its different applications in solving questions on time, speed, and distance. We will learn these concepts through Illustrative Examples.
To get the best learning out of it, you should know some basics such as:
- You are expected to possess a conceptual understanding of the basics of Distance topic
- And the relationship between the three variables; time, speed, and distance – in the form of D = S x T formula
The highlights of this article on GMAT Word Problems on Time, Speed and Distance are as follows
- What will you learn from this article
- The concept of average speed explained with illustrative GMAT word problems
- Calculation of Average Speed when the journey is divided into more than two parts
- Takeaways
What will you learn from this article?
The concept of Average Speed is an important one in the context of GMAT. Hence, the basic objective of this article is to:
- Provide conceptual clarity of the topic, Average Speed
- Solving relevant examples explaining the concepts
- Looking into different learnings and important takeaways
Guillermo scored a Q50 on the GMAT and received an admit for The Wharton School. Take a look at his success story.
For any strategic advice about the GMAT or MBA Admissions, write to us at acethegmat@e-gmat.com. Sign up for a free trial and get unlimited access to concept files, live sessions, and practice questions.
The concept of Average Speed
In theoretical terms, average speed = (Total distance traveled/ Total time taken to travel that distance)
Let’s take an example to have a more detailed explanation of the definition.
Example 1
A bus covers a certain distance at an average speed of 40 mph, while returning the same distance it runs at an average speed of 60 mph. What is the average speed of the bus throughout the journey?
- 20 mph
- 36 mph
- 48 mph
- 50 mph
- 54 mph
Solution
The information in the question can be collated in the following DST table:
| Distance | Speed | Time | |
| Onward Journey | D | 40 mph | – |
| Return Journey | D | 60 mph | – |
A Common Mistake:
While apparently, it may look like the average speed is the arithmetic average of the two speeds given, i.e. (40+60)/2= 50 mph, this is not the correct approach.
If we assume the distance on the onward journey as D, return journey distance will also be D.
- Therefore, the total distance traveled = 2D
Now, the time taken for the onward journey = (D/40) hrs and time taken for the return journey = (D/60) hrs.
- Therefore, the average speed = (Total distance travelled/ total time taken) = 2D/ [(D/40) + (D/60)] = 48 mph
Key Takeaways
As you have observed, although we assumed the value of the unknown distance as D, the final answer doesn’t contain D.
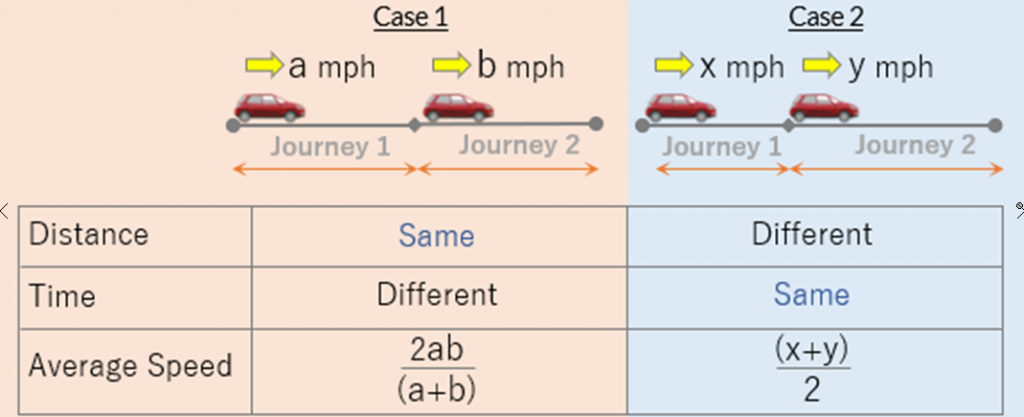
It happens whenever you have the distance as constant and the journey is divided into two equal parts. This can be generalized as follows:
The generalized formula:
Let us assume that a journey has two equidistant part, D each, covered at an average speed of a and b.
Time taken to complete the first half of the journey = D/a
Time taken to complete the second half of the journey = D/b
Therefore, the overall average speed of the journey = 2D/ [(D/40) + (D/60)] = 2ab/a+b
The above specific case shows the example when the journey is divided into two equal parts.
Let’s take another example to understand the application of the above takeaway.
BTW, do you know how to identify a regular polygon? Read our article on Polygons to know more.
Example 2
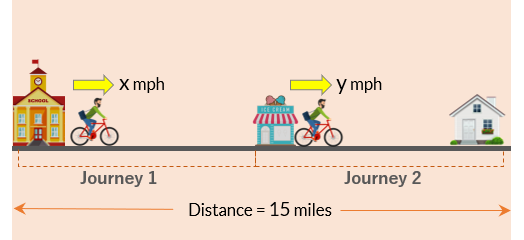
Joey covered his journey of 15 miles in two parts. He covered the 1st part of his journey at x mph and the second part in y mph. What is Joey’s average speed throughout the whole journey?
Statement 1: x = 2.5, y = 5
Statement 2: Distance covered in both parts of the journey is the same
Solution
In this question it is given that:
- Joey covered a journey of total distance 15 miles in two parts
- Speed in the 1st part of the journey = x mph
- Speed in the 2nd part of the journey = y mph
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | D1 | x | |
| 2nd part of the journey | D2 | y | |
| Total | D1 + D2 = 15 |
As we need to find out the average speed of Joey’s whole journey, we need to know two things:
- The total distance, which is already provided as 15 miles
- The total time taken to cover that distance, which is the sum of the individual times taken to cover the 1st and 2nd part separately
Analyzing Statement 1:
Now, analyzing Statement 1 independently, it says x = 2.5 mph and y = 5 mph.
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 2.5 | ||
| 2nd part of the journey | 5 | ||
| Total | 15 |
- Considering this statement only, we get the information about the individual speeds only
- But this does not provide any information about the distances covered in each individual part of the journey – so, we cannot calculate the time taken for the parts of the journey
Hence, Statement 1 is not sufficient to answer the question.
Analyzing Statement 2:
Analyzing Statement 2 independently, it says the distance covered in both parts of the journey is the same.
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 7.5 | x | |
| 2nd part of the journey | 7.5 | y | |
| Total | 15 |
- It means each of the individual parts of the journey is of 7.5 miles.
- But this does not provide any information about the speeds in each individual part of the journey – so, we cannot calculate the time taken for the parts of the journey
Hence, Statement 2 is not sufficient to answer the question.
Combining Both Statements:
Now, combining the information from both the statements, we have:
- Individual speeds in each part, x = 2.5 mph and y = 5 mph
- Distance in each part of the journey = 7.5 miles
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 7.5 | 2.5 | |
| 2nd part of the journey | 7.5 | 5 | |
| Total | 15 |
Considering both together, we can calculate the time taken for individual parts of the journey, and hence the total journey time. From that, we can calculate the average speed.
As we are getting the answer by combining both the statements, correct answer choice is option C.
Note:-
Although for the question you don’t need to calculate the final value, the average speed here will be as follows:
Average Speed = (Total distance traveled)/(Total time taken to travel that distance)
= 15/ [(7.5/2.5) + (7.5/5)] = 15/4.5 = 3.33 mph
Because the distance is divided into two equal parts, we can also calculate the average speeds as
= (2×2.5×5)/(2.5+5) = 3.33 mph [application of the 2ab/a+b formula]
The devil is in the details! Planning out the finer details of your study schedule takes you one step closer to your target GMAT score. Learn the other benefits of a well defined study plan in this article.
Save 60+ hours of GMAT preparation by crafting a well-defined study plan in just 3 steps:
Let us take another example to solidify our understanding and then move to the next topic.
Example 3
Mike started his road trip on his bike and moved at a constant speed of 50 mph. After completing p% of his total journey, his bike started malfunctioning, and therefore, he had to complete his journey at half of his normal speed. What is the average of Mike’s whole journey, in terms of p?
Solution
In this question, first, we can consolidate all the given information.
- Here Mike’s journey is getting divided into two parts –
- 1st part is p% of total journey distance, completed at 50 mph
- 2nd or the remaining part of the journey, completed at 25 mph
We need to find out the average speed of Mike throughout the whole journey.
| Distance | Speed | Time | |
| Before bike malfunction | p % of total journey distance | 50 mph | – |
| After bike malfunction | (100-p) % of the total journey distance | 25 mph | – |
Now you can see that the total distance is not mentioned here. If we assume the total distance to be D, then
1st part of the journey = p% of D = pD/100, and remaining part of the journey = (D-(pD/100))
As we have the individual distances and speeds for every segment of the journey, we can now calculate the time taken for each segment as follows:
Time taken to complete the 1st part of the journey = (pD/100)/50 hrs = pD/5000 hrs
And, time taken to complete the 2nd part of the journey = [(D-pD)/100]/25 hrs = (100D-pD) /2500 hrs
Now that we know the total distance and total time for the journey, we can calculate the average speed of the whole journey as follows:
Average Speed = D/ [(pD/5000) + (100D-pD)/2500] = 5000/ (200-p)
Key Takeaways
- Although we assumed the value of the total distance as D, the final answer is independent of D. This is happening because the distance remains constant and does not influence the average speed.
- Although the journey is taking place in two separate parts, the overall journey distance remains constant. In cases like this, one can assume any constant value as the total distance for the ease of calculation. For example, in this case, if the distance is assumed to be 100 in place of D, the overall simplification process becomes very easy. Check the following:
As the total distance is assumed to be 100, then
- 1st part of the journey = p% of 100 = p, and remaining part of the journey = (100 – p)
As we have the individual distances and speeds for every segment of the journey, we can now calculate the time taken for each segment as follows:
Time taken to complete the 1st part of the journey = p/50 hrs
And, time taken to complete the 2nd part of the journey = (100-p)/25 hrs
Now that we know the total distance and total time for the journey, we can calculate the average speed of the whole journey as follows:
Average speed = 100/(p/50) + (100-p)/25 = 5000/ (200-p)
Example 4
On a certain day, Rosita started driving towards Stevie’s place at a speed of 45 mph. After moving for 2 hours, she realized that she needed to reach Stevie’s place in another 2 hours only. Therefore, she increased her speed to 65 mph and reached Stevie’s place exactly on time. What is the average speed of her journey?
Solution
In this question, the journey has happened in two parts.
- In the 1st part, Rosita moved at 45 mph for 2 hrs. Therefore, the distance covered = (45 × 2) = 90 miles
- In the 2nd part, Rosita moved at 65 mph for 2 hrs. Therefore, the distance covered = (65 × 2) = 130 miles
| Distance (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 45 x 2 = 90 | 45 | 2 |
| 2nd part of the journey | 65 x 2 = 130 | 65 | 2 |
Total distance travelled by Rosita = (90 + 130) miles = 220 miles
Total time taken to travel that distance = (2 + 2) hrs = 4 hrs
Hence, the average speed of the journey = Total distance travelled/ Total time taken = 220/4 mph = 55 mph
Key Takeaways
If looked closely, one can see that the average speed, in this case, is the arithmetic average of the two given speeds. Let us see the logic behind that:
Assume a scenario when a moving body travels at a speed of x mph for t hrs and at a speed of y mph for next ‘t’ hrs
- In the 1st t hours, the distance covered = xt miles
- In the next t hours, the distance covered = yt miles
- The total distance covered = (xt + yt) miles = t (x + y) miles
- Total time taken to cover the distance = (t + t) hrs = 2t hrs
Therefore, the average speed = Total distance travelled/ Total time taken to travel that distance = t(x+y)/2t = (x+y)/2 mph
- which is essentially the numerical average of two speeds given
Hence, we can say if the journey is divided into two parts, in which every part is traveled for the same amount of time, the average speed of the journey is the numerical average of the two individual speeds.
This logic can also be generalized for journeys which are divided into more than two parts, and the time taken to cover each part is same.
Let’s say we are considering a scenario where the journey is divided into 3 parts, with the speeds in the three parts are x, y, and z respectively and the time taken to cover each part of the journey is same.
- In such a scenario, we can say the average speed of the whole journey is nothing but the average or arithmetic mean of the three individual speeds, i.e. x+y+z/3
The same concept can also be generalized for journeys which are divided into multiple parts, with each part takes the same time to cover.
Want to boost your Quant score but no able to define the path to your Target Score? Attend our Quant Workshop and get personalised feedback from Subject Matter Experts which will help you hit your Target GMAT Scores.
Example 5
A bus covers a certain distance at an average speed of 100 mph without any stoppages. While returning the same journey the bus covers the distance at an average speed of 60 mph with stoppages. What is the average stoppage time per hour taken by the bus?
Solution
This question can be solved using multiple methods:
Method 1:
Let’s assume the total journey distance in either direction is D
Therefore, time taken for the onward journey = D/100 hrs
And time taken for the return journey = D/60 hrs
The difference in journey time indicates the stoppage time, which is (D/60)-(D/100) = D/150 hrs
In the journey of D/60 hrs, stoppage time is D/150 hrs
Therefore, the average stoppage time per hour = (D/150)/ (D/60) hrs = 60/150 hrs = 2/5 hrs = 24 minutes
Method 2:
Let’s assume the distance to be 300 miles (LCM of 100 and 60).
Therefore, time for the onward journey = 300/100 = 3 hrs
And time for the return journey = 300/60 = 5 hrs
The extra time indicates the stoppage time, which is (5-3) = 2 hrs
In the journey of 5 hrs, stoppage time is 2 hrs, therefore, average stoppage time per hour = 2/5 hrs = 24 minutes
Method 3:
As the decrease in speed is happening due to stoppages, that decrease would have been absent if there were no stoppages. Here the decrease in speed is (100 – 60) = 40 miles per hour.
Now, when the bus was running without any stoppages, the average speed was 100 mph. At this speed, the bus could have completed 40 miles distance in a time of 40/100 hrs or 24 minutes, which is effectively the stoppage time.
On a similar note, if the decrease in speed was 80 miles per hour, then the bus would have completed that 80 miles distance in a time of 80/100 hrs or 48 minutes.
Key Takeaway
Although the distance remains the same in both onward and return journey, the average speed decreases because of the increase in the total journey time due to stoppages.
Generic case: Calculation of Average Speed when the journey is divided into more than two parts
Till now we have mostly taken up those examples where the average speed is calculated in the journeys divided into two parts. However, the concept of average speed is applicable to cases where the journey is divided into more than 2 parts. Let’s consider the following example:
Example 6
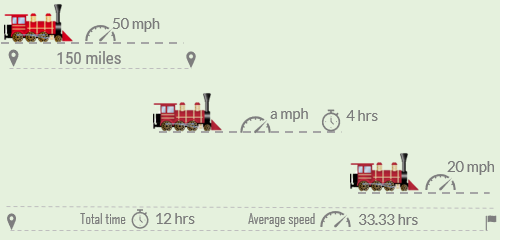
A train covered first 150 miles of its journey at an average speed of 50 mph. After that, it met with a small accident and its speed decreases to ‘a’ mph. It continued its journey for 4 hrs and then its speed decreased further to 20 mph. Running at this speed, the train completed the journey in total 12 hrs. Find the value of ‘a’, if the average speed of the whole journey is 33.33 mph.
Solution
In this question, the journey is separated into 3 parts, with each of them having different speeds. We can consider the whole information in the following tabular form:
| Distance covered (in miles) | Speed (in mph) | Time Taken (in hrs) | |
| 1st part of the journey | 150 | 50 | 3 |
| 2nd part of the journey | a | 4 | |
| 3rd part of the journey | 100 | 20 | |
| Total | 12 |
As the total journey time is 12 hrs, the journey time for the 3rd part = 12 – (3 + 4) hrs = 5 hrs
Also, we know average speed = Total distance traveled/ Total time taken
Or, 33.33 = Total distance travelled/12
Or, Total Distance travelled = 33.33×12 = 400 miles
Therefore, the distance traveled in the 2nd part of the journey = 400 – (150 + 100) miles = 150 miles
So, the average speed in the 2nd part of the journey = 150/4 = 37.5 mph
Key Takeaways
In this question, we can see the application of average speed where the journey is completed in more than two parts. But the procedural approach of the concept remains same –
- Average speed is used as per the basic definition, i.e. the ratio of total distance traveled, and total time taken
- Whether the journey is divided into two or more than two parts, the procedure of calculating the average speed remains the same
Key Takeaways from the article | GMAT Word Problems | Time, Speed, and Distance
- By definition, the average speed of a journey = (total distance traveled)/(total time taken to travel that distance)
- If a journey is divided into two equal parts, with individual speeds a and b respectively, then the average speed of the whole journey is denoted by 2ab/(a+b). However, this formula cannot be generalized for a journey which is divided into more than 2 parts
- If a journey is divided into multiple parts, and the time taken to cover every part is same, then the average speed of the whole journey is denoted by the average or arithmetic mean of the individual speeds at which the individual parts are covered.
Questions on time, speed and distance are very commonly asked on the GMAT. Ace GMAT Quant by signing up for our free trial and get access to 400+ questions. We are the most reviewed online GMAT Prep company with 2400+ reviews on GMATClub.