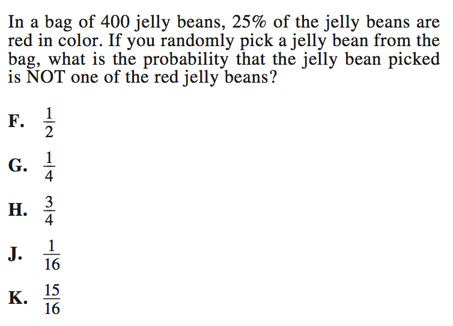Graph »
Number Line »
Challenge »
Examples »
FAQ »
Correct Answer 
Let’s Try Again 
Try to further simplify
Number Line
Graph
Hide Plot »
Sorry, your browser does not support this application
Examples
-
mathrm{Lauren’s:age:is:half:of:Joe’s:age.:Emma:is:four:years:older:than:Joe.:The:sum:of:Lauren,:Emma,:and:Joe’s:age:is:54.:How:old:is:Joe?}
-
mathrm{Kira:went:for:a:drive:in:her:new:car.:She:drove:for:142.5:miles:at:a:speed:of:57:mph.:For:how:many:hours:did:she:drive?}
-
mathrm{Bob’s:age:is:twice:that:of:Barry’s.:Five:years:ago,:Bob:was:three:times:older:than:Barry.:Find:the:age:of:both.}
-
mathrm{Two:men:who:are:traveling:in:opposite:directions:at:the:rate:of:18:and:22:mph:respectively:started:at:the:same:time:at:the:same:place.:In:how:many:hours:will:they:be:250:apart?}
-
mathrm{If:2:tacos:and:3:drinks:cost:12:and:3:tacos:and:2:drinks:cost:13:how:much:does:a:taco:cost?}
Frequently Asked Questions (FAQ)
-
How do you solve word problems?
- To solve word problems start by reading the problem carefully and understanding what it’s asking. Try underlining or highlighting key information, such as numbers and key words that indicate what operation is needed to perform. Translate the problem into mathematical expressions or equations, and use the information and equations generated to solve for the answer.
-
How do you identify word problems in math?
- Word problems in math can be identified by the use of language that describes a situation or scenario. Word problems often use words and phrases which indicate that performing calculations is needed to find a solution. Additionally, word problems will often include specific information such as numbers, measurements, and units that needed to be used to solve the problem.
-
Is there a calculator that can solve word problems?
- Symbolab is the best calculator for solving a wide range of word problems, including age problems, distance problems, cost problems, investments problems, number problems, and percent problems.
-
What is an age problem?
- An age problem is a type of word problem in math that involves calculating the age of one or more people at a specific point in time. These problems often use phrases such as «x years ago,» «in y years,» or «y years later,» which indicate that the problem is related to time and age.
word-problems-calculator
en
We recommend reading any word problem at least three times. By the conclusion of the third reading, you should be magically whisked away to your home in Kansas.
Read it once…
…to get a general idea of what’s going on. Is the problem about money? Height and width? Distances? Money? Yeah, we already said money, but it’s important. Can you draw a picture in the margin that helps you visualize the problem?
Twice…
…to translate from English into math. Read the problem carefully this time, figuring out which pieces of information are important and which ones aren’t. Then ignore the unnecessary bits. This may sound a bit harsh, but they’re big boys. They’ll get over it.
Three times…
…a lady. Wait, that’s not it.
…to make sure you answered the right question. To be sure, read the question one last time before drawing a box around your final answer. Even if this method of double-checking saves you only once out of every 100 times, it’ll help you in the long run. One out of 100 is better than zero out of 100, but now we’re moving into some advanced mathematics.
Sample Problem
The local department store was having a sale. Holla! Gabe bought a pair of shoes for $21, although they would’ve been cheaper if he’d bought penny loafers. He also bought some shirts that were on sale for 25% off their normal retail price of $18 each. Gabe, always the bargain hunter, spent $75 total. How many shirts did he buy? Also, does he really think any of them will go with those shoes?
Read the problem:
Once…
…for a general idea of what’s going on: Gabe went shopping and spent money. Sounds like an old familiar story. Time for an intervention, friends of Gabe. Preferably before he maxes out his Diner’s Club card.
Now we need to figure out how many shirts he bought, so we read the problem:
Twice…
…to translate from English into math. Remember, we can translate a bit at a time, sort of like how Gabe pays for some of his major purchases when he has them on layaway. Sheesh, Gabe. Get a hold of yourself.
(total amount Gabe spent) = (amount he spent on shoes) + (amount he spent on shirts)
We know he spent $75 total, of which $21 was spent on shoes. This gives us the equation:
$75 = 21 + (amount he spent on shirts)
The problem has gotten smaller. It’s depressing when that happens with cake, but great when it happens to a word problem. Now all we need to do is come up with a symbolic expression for how much Gabe spent on shirts, or the cost per shirt times the number of shirts:
(amount he spent on shirts) = (cost per shirt)(number of shirts)
Since the shirts are 25% off their normal price of $18, they cost $18 – 0.25(18) = $13.50 each. What a deal, and real polyester, too!
We need to introduce a variable for the number of shirts; s will do the trick nicely.
(amount he spent on shirts) = 13.5s
When we plug this into the earlier equation, we find that:
75 = 21 + 13.5s
Finally, we’ve reduced this thing to a super-simple-looking equation! Good riddance, vestiges of language! Begone, nouns and verbs!
Things look much nicer now, right? No worrying about shirts or shoes or prices or Gabe’s uncontrollable shopping addiction. For the moment, we can forget about the word problem and solve the equation. The answer is s = 4, by the way. In case you were interested.
Three times…
…to make sure we’re answering the right question. We want to know how many shirts Gabe bought. Is that the answer we arrived at? We found that s = 4, and s was the number of shirts Gabe bought, so we’re all done. Four new shirts for Gabe, and three of them feature a Hawaiian pattern. Gabe, if you insist on buying far more clothes than you need, can’t you at least have a decent fashion sense?
When translating from English into math, some information can be ignored. We don’t care that «the local department store was having a sale.» Gabe might, but we certainly don’t. We care about statements that tell us numbers, and statements that tell us what the question is. Any extraneous information has been placed there simply as a decoy. We’re not going to fall for that. Wait a second…two for one? We’ll grab our jacket and meet you there.
Some people find it helpful to underline the important pieces of information in a word problem. You’re like an actor highlighting in a script the lines that are important for him to remember. Unlike an actor, however, you can always look back at the original problem if you draw a blank. Also, you don’t need to wear any stage makeup.
In the problem we just did, the important bits might look something like this:
The local department store was having a sale. Gabe bought a pair of shoes for $21 and some shirts that were on sale for 25% off their normal retail price of $18 each. If Gabe spent $75 total, how many shirts did he buy?
As you practice, you’ll become better at figuring out which parts of the word problem you can ignore and which parts are important.
Dynamically Created Word Problems
Here is a graphic preview for all of the word problems worksheets. You can select different variables to customize these word problems worksheets for your needs. The word problems worksheets are randomly created and will never repeat so you have an endless supply of quality word problems worksheets to use in the classroom or at home. Our word problems worksheets are free to download, easy to use, and very flexible.
These word problems worksheets are a great resource for children in 3rd Grade, 4th Grade, and 5th Grade.
Click here for a Detailed Description of all the Word Problems Worksheets.
Quick Link for All Word Problems Worksheets
Click the image to be taken to that Word Problems Worksheet.
Words to Symbols
|
Addition Word Problems
|
Addition Word Problems
|
Addition Word Problems
|
Addition Word Problems
|
Addition Word Problems
|
Subtraction Word Problems
|
Subtraction Word Problems
|
Addition and Subtraction
|
Addition and Subtraction
|
Addition and Subtraction
|
Addition and Subtraction
|
Multi-Step Problems
|
Multiplication Word Problems
|
Multiplication Word Problems
|
Multiplication Word Problems
|
Division Word Problems
|
Division Word Problems
|
Division Word Problems
|
Division Word Problems
|
Multiplication and Division
|
Mixed Operations Problems
|
One Step Equation
|
Two Step Equation
|
Multi-Step All Operations
|
Fractions Word Problems
|
Fractions Word Problems
|
Fractions Word Problems
|
Fractions Word Problems
|
Fractions Word Problems
|
U. S. Coins Word Problems
|
Advanced Adding U. S. Coins
|
U. S. Money Word Problems
|
U. S. Money Word Problems
|
U. S. Money Word Problems
|
Travel Time
|
Reading a Calendar
|
Elapsed Dates
|
Ratios and Rate
|
Percentage
|
Mixed Operations
|
U.S. Money
|
Detailed Description for All Word Problems Worksheets
Words to Symbols Handout
This Word Problems Worksheet will produce a great handout to help students learn the symbols for different words and phrases in word problems.
Addition Word Problems Worksheets Using 1 Digit with 2 Addends
These addition word problems worksheets will produce 1 digit problems with two addends, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition Word Problems Worksheets Using 2 Digits with 2 Addends
These addition word problems worksheets will produce 2 digits problems with two addends, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition Word Problems Worksheets Using 1 Digit with 3 Addends
These addition word problems worksheets will produce 1 digit problems with three addends, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition Word Problems Worksheets Using 2 Digits with 3 Addends
These addition word problems worksheets will produce 2 digits problems with three addends, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition Word Problems Worksheets 2 Digits Missing Addends
These addition word problems worksheet will produce 2 digits problems with missing addends, with ten problems per worksheet. You may select between regrouping and non-regrouping type of problems. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Subtraction Word Problems Worksheets Using 1 Digit
These subtraction word problems worksheets will produce 1 digit problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Subtraction Word Problems Worksheets Using 2 Digits
These subtraction word problems worksheets will produce 2 digits problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition and Subtraction Word Problems Worksheets Using 1 Digit
These addition and subtraction word problems worksheets will produce 1 digit problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition and Subtraction Word Problems Worksheets Using 2 Digits
These addition and subtraction word problems worksheets will produce 2 digits problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition and Subtraction Word Problems Worksheets 2 Digits With No Regrouping
These addition and subtraction word problems worksheets will produce 2 digits problems with no regrouping and ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Addition and Subtraction Word Problems Worksheets Using 3 Digits
These addition and subtraction word problems worksheets will produce 3 digits problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Multi-Step Problems Addition and Subtraction
These multi-step addition and subtraction word problems worksheets will produce 10 problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Multiplication Word Problems Worksheets Using 1 Digit
These multiplication word problems worksheets will produce 1 digit problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Multiplication Word Problems Worksheets Using Dozens
These multiplication word problems worksheets will produce problems using dozens, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Multiplication Word Problems Worksheets Using 2 Digits
These multiplication word problems worksheets will produce 2 digits problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Division Word Problems Worksheets Using 1 Digit in Divisor
These division word problems worksheets will produce 1 digit divisor problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Division Word Problems Worksheets Using Dozens in Divisor
These division word problems worksheets will produce problems using dozens in the divisor, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Division Word Problems Worksheets Using Multiple Digits in Divisor
These division word problems worksheets will produce multiple digits in the divisor for the problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Dividing with Fractions Worksheets
These division Word Problems Worksheets will produce problems that focus on division with fractions. You have the option to select the range of denominators, as well as the types of fractions displayed. These word problems worksheets are appropriate for 4th Grade, 5th Grade, and 6th Grade.
Multiplication and Division Problems Using 1 Digit
These multiplication and division word problems worksheets will produce 1 digit problems, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Mixed Operations Word Problems Using 1 or 2 Digits
These mixed operations word problems worksheets will produce addition, multiplication, subtraction and division problems with 1 or 2 digit numbers. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
One Step Equation Word Problems
These equations worksheets will produce one step word problems. These worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Two Step Equation Word Problems
These equations worksheets will produce two step word problems. These worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Multi-Step All Operations Word Problems
These Word Problems worksheets will produce word problems involving all basic operations. You may choose the format of the answers. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Adding Two Fractions Word Problems
These fractions word problems worksheets will produce problems with the addition of two fractions. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Adding Three Fractions Word Problems
These fractions word problems worksheets will produce problems with the addition of three fractions. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Subtracting Fractions Word Problems
These fractions word problems worksheets will produce problems involving subtracting two fractions. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Adding Two Mixed Numbers Word Problems
These fractions word problems worksheets will produce problems involving adding two mixed numbers. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Subtracting Two Mixed Numbers Word Problems
These fractions word problems worksheets will produce problems involving subtracting two mixed numbers. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Adding U.S. Coins Word Problems
This U.S. coins word problems worksheet will produce coin addition problems. These worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Advanced Adding U.S. Coins Word Problems
These Word Problems Worksheets will produce word problems that focus on adding up different denominations of US coin currency. You have the option to select any combination of pennies, nickels, dimes, quarters, and half dollars for each worksheet. These worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
U.S. Money Adding Two Items Word Problems
These U.S. money word problems worksheest will produce purchase problems for adding two items. These worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
U.S. Money Adding Three Items Word Problems
These U.S. money word problems worksheets will produce purchase problems for adding three items. These worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
U.S. Money Change from a Purchase Word Problems
These U.S. money word problems worksheets will produce problems for calculating change from a purchase. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are a good resource for students in the 5th Grade through the 8th Grade.
Travel Time Word Problems Worksheets
These time word problems worksheets produce problems for finding the duration, start, and end times of trips. Users may select the units of time to use in the problems. These word problems worksheets will produce ten problems per worksheet. These word problems worksheets are appropriate for students in the 5th Grade through the 8th Grade.
Reading a Calendar Word Problems Worksheets
These calendar worksheets will produce word problems revolving around reading and understanding a monthly calendar. You may select the month and the year within a range of the years 1800 to 3999. These word problems worksheets will produce nine problems per worksheet. These word problems worksheets are appropriate for students in the 5th Grade through the 8th Grade.
Elapsed Dates Word Problems Worksheets
These time word problems worksheets will produce questions with elapsed days, weeks, months, and years, with ten problems per worksheet. These word problems worksheets are appropriate for 3rd Grade, 4th Grade, and 5th Grade.
Ratios and Rates Word Problems Worksheets
These ratio word problems worksheets will produce eight ratio and rates word problems for the students to solve. These ratio word problems worksheets are appropriate for 3rd Grade, 4th Grade, 5th Grade, 6th Grade, and 7th Grade.
Percentage Word Problems Worksheets
These Percentage Word Problems Worksheets will produce problems that focus on finding and working with percentages. You have the option to select the types of numbers, as well as the types of problem you want. These percentage word problems worksheets are appropriate for 3rd Grade, 4th Grade, 5th Grade, 6th Grade, and 7th Grade.
Mixed Word Problems with Key Phrases Worksheets
These Word Problems Worksheets will produce addition, multiplication, subtraction and division problems using clear key phrases to give the student a clue as to which type of operation to use. These word problems worksheets are appropriate for 4th Grade, 5th Grade, 6th Grade, and 7th Grade.
U.S. Money Change from a Purchase Multiplication Word Problems
These Word Problems Worksheets will produce problems that ask students to use multiplication to calculate the monetary value of a purchase and then find how much change is given from the purchase.
If you need help figuring out how old someone is (or how old you are) given a birthday, you can use this great Age
Calculator. to answer those questions and many more!
Eight interesting and fun least common multiple word problems you can give to your students to tease them. If your students can solve these problems, they can probably solve any word problems about the least common multiple.
Word problem #1
Today, both the soccer team and the basketball team had games. The soccer team plays every 3 days and the basketball team plays every 5 days. When will both teams have games on the same day again?
Word problem #2
A manager at a restaurant can buy hamburger buns in packages of 8 and hamburger patties in packages of 6. Suppose that the manager cannot buy part of a package. What is the least number of packages of each product he can buy to have an equal number of hamburger patties and buns?
Word problem #3
A man smiles at his beautiful wife every 3 seconds while the wife smiles back at him every 6 seconds. When will both husband and wife smile at each other at the same time?
Word problem #4
Steve can save 9 dollars every day while Maria can save 12 dollars every day. What is the least number of days it will take each person to save the same amount of money?
More Interesting and fun least common multiple word problems
Word problem #5
Boxes that are 12 inches tall are being piled next to boxes that are 10 inches tall. What is the least height in feet at which the two piles will be the same height?
Word problem #6
A radio station plays «yesterday» by the Beatles once every 2 days. Another radio station plays the same song once every 3 days. How many times in 30 days will both radio stations play the same song on the same day?
Word problem #7
Two men running a marathon took a sip of water at the same time 72 minutes after they started the race. If the first man took a sip of water every 9 minutes, how often did the other man take a sip of water?
Word problem #8
A train to New York city leaves a station every 7 minutes. Another train to Boston leaves the station every 6 minutes. Suppose it is 6:30 am right now. At what time will both trains leave the station together?
A least common multiple word problem about barking dogs
Five dogs in a neighborhood were barking consistently last night. The names of the dogs are Lucy, Max, Murphy, Daisy, and Sam. The dogs started barking at 10 P.M.
Then, Lucy barked every 5 minutes, Max barked every 3 minutes, Murphy barked every 4 minutes, Daisy barked every 3 minutes, and Sam barked every minute. Why did Mr. Smith suddenly awaken at 11 P.M.?
Recent Articles
-
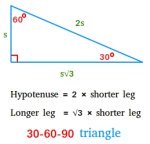
30-60-90 Triangle
Apr 03, 23 05:08 PM
What is a 30-60-90 triangle? Definition, proof, area, and easy to follow real-world examples.
Read More
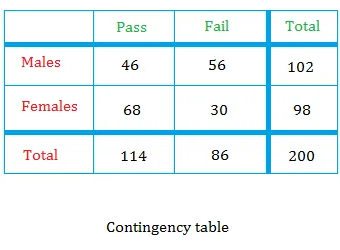
-
Calculate the Conditional Probability using a Contingency Table
Mar 29, 23 10:19 AM
Learn to calculate the conditional probability using a contingency table. This contingency table can help you understand quickly and painlessly.
Read More
You may love GMAT word problems or you may hate them, but you can’t get around them if you want to ace the GMAT Quant section. No matter what your feelings are about this problem type, though, Magoosh’s experts have put together everything you need to know (and practice!) GMAT word problems in order to master them before test day.
Table of Contents
- What to Expect from GMAT Word Problems
- Strategy Guide: What’s the Trick to Mastering GMAT Word Problems?
- Translate from Words to Math
- Learn to Work with Variables
- Plug in Numbers (the Smart Way)
- Understand Your Strengths and Weaknesses
- GMAT Word Problem Practice Questions
- A Final Word on GMAT Word Problems
What to Expect from GMAT Word Problems
Think of GMAT word problems as questions that ask you to turn real-world situations into math problems. Of course, this can be a lot more complicated than it sounds. After all, it’s one thing to understand algebra in the abstract, and quite another to think about where the rubber meets the road.
Think about it this way: the reason human beings created algebra was to solve problems about real-world situations, and the GMAT loves asking math problems about numbers and about real-world situations, a.k.a. word problems! Even folks who can do algebra in the abstract sometimes find word problems challenging.
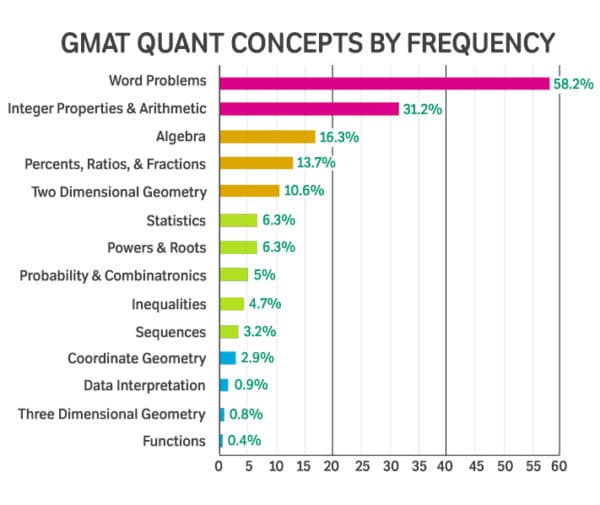
You’ll find GMAT word problems in the GMAT Quant section. How much of the GMAT is word problems? Within the Quant section, actually a whole lot! A study of official GMAT questions from actual tests show that word problems account for 58.2% of all GMAT math questions.
In other words, test-takers should anticipate a word problem cropping up (on average) in three out of every five questions you’ll see in Quant. Because of GMAT word problems’ prevalence, you can expect to see both Data Sufficiency and Problem Solving questions in this format. The question format and answer choices may look different, but the basic premise will be the same.
You may be feeling the pressure, but hang in there! If you’re worried about how to master word problems on the GMAT, keep reading for our GMAT Word Problems strategy guide.
Strategy Guide: What’s the Trick to Mastering GMAT Word Problems?
First, the disappointing news: there’s no one strategy that will work to immediately solve every word problem, every time (where would the fun in that be?). The good news: by using and combining a variety of strategies, you can put together the tools you need to ace even the most complex GMAT word problems, every single time!
With that in mind, here are the four key strategies you’ll need.
1. Translate from Words to Math
Suppose we have the following sentence in a word problem:”Three-fifths of x is 14 less than twice y squared.” How do we change words to math? Here’s a quick guide:
- The verb “is/are” is the equivalent of an equal sign; the equal sign in an equation is, in terms of “mathematical grammar,” the equivalent of a verb in a sentence. Every sentence has a verb and every equation has an equal sign.
- The word “of” means multiply (often used with fractions and percents). Ex. “26% of x” means (0.26x)
- The words “more than” or “greater than” mean addition. Ex. “5 greater than x” means (x + 5) and “7 more than y” means (y + 7)
- The words “less than” means subtraction. Ex. “8 less than Q” means (Q – 8). Notice that the first element is always subtracted: in other words, “J less than K” means (K – J).
With that in mind, let’s go back to the sentence from the hypothetical problem above.
- “three fifths of x” means [(3/5)*x]
- “is” marks the location of the equal sign
- “twice y squared” means 2(y^2)
- “14 less than twice y squared” means 2(y^2) – 14
Altogether, the equation we get is:
Using this strategy, it’s straightforward to translate from a verbal statement about numbers to an equation.
2. Learn to Work with Variables
Working with Variables Part I: Assigning Variables
Most GMAT word problem concern real world quantities and are stated in real world terms, and we need to assign algebraic variables to these real-world quantities.
Sometimes, one quantity is directly related to every other quantity in the problem. For example:
“Sarah spends 2/5 of her monthly salary on rent, 1/12 of her monthly salary on auto costs including gas and insurance, and 1/10 of her monthly salary automatically goes into saving each month. With what she has left each month, she spend she spends $800 on groceries and …”
In that problem, everything is related to “monthly salary,” so it would make a lot of sense to introduce just one variable for that, and express everything else in terms of that variable. Also, please don’t always use the boring choice of x for a variable! If we want a variable for salary, you might use the letter S, which will help you remember what the variable means! If we are given multiple variables that are all related to each other, it’s often helpful to assign a letter to the variable with the lowest value, and then express everything else in terms of this letter.
If there are two or more quantities that don’t depend directly on each other, then you may well have to introduce a different variable for each. Just remember that it’s mathematically problematic to litter a problem with a whole slew of different variables. You see, for each variable, you need an equation to solve it. If we want to solve for two different variables, we need two different equations (this is a common Word Problem scenario). If we want to solve for three different variables, we need three different equations (considerably less common). While the mathematical pattern continues to extend upward from there, more than three completely separate variables is almost unheard of on GMAT math.
When you assign variables, always be hyper-vigilant and over-the-top explicit about exactly what each variable means. Write a quick note to yourself on the scratch paper: T = the price of one box of tissue, or whatever the problem wants. What you want to avoid is the undesirable situation of solving for a number and not knowing what that number means in the problem!
Practice Question
Here’s a word problem practice question that’s a bit easier than what you might see on the GMAT!
Andrew and Beatrice each have their own savings account. Beatrice’s account has $600 less than three times what Andrew’s account has. If Andrew had $300 more dollars, then he would have exactly half what is currently in Beatrice’s account. How much does Beatrice have?
Click here for a text answer and explanation
The obvious choices for variables are A = the amount in Andrew’s account and B = the amount in Beatrice’s account. The GMAT will be good about giving you word problems involving people whose names start with a different letter so that it’s easier to assign variables. We can turn the second & third sentences into equations.
second sentence: B = 3A – 600
Both equations are solved for B, so simply set them equal.
3A – 600 = 2(A + 300)
3A – 600 = 2A + 600
A – 600 = 600
A = 1200
We can plug this into either equation to find B. (BTW, if you have time, an excellent check is to plug it into both equations, and make sure the value of B you get is the same!)
B = 3000
Thus, Andrew has $1200 in his account, and Beatrice, $3000 in hers.
Working with Variables Part II: Choosing Your Approach
When questions have variables in the answer choices, you can decide whether you’d rather take an algebraic approach or a numerical approach. Neither one of these is “better” than the other— it all depends on what works best for you.
An algebraic approach is what you most likely learned back in high school. This means that, to solve the problem, you’ll manipulate the variables according to mathematical rules. For a super-basic example, to solve ( 2x = y ), you would divide both sides by two and end up with ( x = y/2 ).
However, you could also to a numerical approach to this (and many other!) problems. This means putting numbers into both the question and the answer choices. So let’s take the previous example—which, again, is much, much easier than anything you’d see on the GMAT:
If ( 2x = y ), what is x in terms of y?
A. (y/20)
B. (y/2)
C. (4/y)
D. (20/y)
Here, you could pick a number to stand in for x in the original equation. Let’s say x is 2. If x = 2, then the original equation tells us that y = 4.
Now, plug that into the question and the answer. The question becomes: “What is 2 in terms of 4?” It’s ½, or .5. Then, look for the answer choice that gives you this answer. Plugging in the numbers, you get:
A. (y/20) = 0.2
B. (y/2) = 0.5
C. (4/y) = 1
D. (20/y) = 5
So B must be correct.
What does this look like in practice? For some harder examples, take a look over at Mike’s post on Variables in GMAT Answer Choices: 2 Approaches.
3. Plug in Numbers (the Smart Way)
If you choose to use the numerical approach described above, keep in mind that there are some key tips for plugging in numbers that you should use!
Here’s a quick summary of how to quick the best numbers for a particular problem:
- Remember that the GMAT has a broad definition of “number” that goes beyond positive integers! Zero, fractions, and negatives are all included. Work on developing number sense to help select the best numbers in a given scenario.
- For percent problems, think outside the box: GMAT test writers know lots of students pick 100. Try 500 or 1000 instead.
- Don’t try to pick numbers for questions involving more than one percent increase or decrease.
- Pay attention to units and convert them appropriately. This is particularly important in solutions and mixing problems!
- Don’t pick 1 as a number—it has too many unique properties.
A separate case involving plugging in, rather than picking, numbers: When all the answer choices are numerical, one further strategy we have at our disposal is backsolving. Using this strategy, we can pick one answer, plug it into the problem, and see whether it works. If this choice is too big or too small, it guides us in what other answer choices to eliminate. Typically, we would start with answer choice (C), but if another answer choice is a particularly convenient choice, then we would start there.
4. Understand Your Strengths and Weaknesses
With all of the above strategies at your disposal, you have everything you need to improve your answers to GMAT word problems. The most efficient way to do this is to keep an error log of word problems you’ve answered wrong in your practice, then review it. As you go through, think about the following:
- What concept or concepts was the question testing?
- What was tricky about the wording of the question?
- Were you already familiar with the methods used in the explanation video?
- Once you watched the explanation video, could you explain how to solve the problem to somebody else?
Your answers to these questions can help you craft a better strategy for GMAT Math word problems, identifying exactly what you need to review to get better!
GMAT Word Problem Practice Questions
Now that you’ve learned how to approach word problems, we’ve put together a collection of them, direct from Magoosh’s product, for you to try! Video and text answers and explanations follow each question.
- Ann and Bob planted trees on Friday. What is the ratio of the number of trees that Bob planted to the number of trees that Ann planted?
(1) Ann planted 20 trees more than Bob planted.
(2) Ann planted 10 percent more trees than Bob planted.
A. Statement 1 ALONE is sufficient to answer the question, but statement 2 alone is NOT sufficient.
B. Statement 2 ALONE is sufficient to answer the question, but statement 1 alone is NOT sufficient.
C. BOTH statements 1 and 2 TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient.
D. Each statement ALONE is sufficient to answer the question.
E. Statement 1 and 2 TOGETHER are NOT sufficient to answer the question.
Click here for a video answer and explanation to GMAT Word Problem 1!
Click here for a text answer and explanation to GMAT Word Problem 1!
Our task is to determine the ratio of Bob’s trees to Ann’s trees. Let’s label these numbers of trees with variables:
Bob’s trees→B, Ann’s trees→A
With these variables, we can express the ratio we want to determine:
(B/A) =?
Statement 1:
Ann planted 20 trees more than Bob planted.
Let’s translate this into an equation using A and B:
( A=B+20 )
Now we can substitute this into our ratio, replacing A:
(B/A) = ( B/(B+20) )
No matter what simplifications we make, we cannot find a numerical value for this fraction. We would need a value for B. We cannot determine the ratio. Statement 1 by itself is not sufficient.
Statement 2:
Ann planted 10 percent more trees than Bob planted.
Let’s translate this into an equation using A and B:
(A=1.10 x B )
Again, let’s substitute this in for A in our ratio:
(B/A) = ( B/(1.10B) )
= (1/1.1 )
We found a value for the ratio of Bob’s trees to Ann’s trees. Statement 2 alone is sufficient.
- The Townville museum was open for 7 consecutive days. If the number of visitors each day was 3 greater than the previous day, how many visitors were there on the first day?
(1) There were a total of 126 visitors for the 7 days.
(2) The number of visitors on the seventh day was three times the number of visitors on the first day.
A. Statement 1 ALONE is sufficient to answer the question, but statement 2 alone is NOT sufficient.
B. Statement 2 ALONE is sufficient to answer the question, but statement 1 alone is NOT sufficient.
C. BOTH statements 1 and 2 TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient.
D. Each statement ALONE is sufficient to answer the question.
E. Statement 1 and 2 TOGETHER are NOT sufficient to answer the question.
Click here for a video answer and explanation to GMAT Word Problem 2!
Click here for a text answer and explanation to GMAT Word Problem 2!
If x is the number of visitors on the first day, then:
x = # of visitors on the 1st day
x + 3 = # of visitors on the 2nd day
x + 6 = # of visitors on the 3rd day
x + 9 = # of visitors on the 4th day
x + 12 = # of visitors on the 5th day
x + 15 = # of visitors on the 6th day
x + 18 = # of visitors on the 7th day
1) Adding up the number of visitors gives us:
x + (x + 3) + (x + 9) + (x + 12) + (x + 15) + (x + 18) = 126
We could simplify and solve this for x. So Statement 1 is sufficient.
2) x + 18 = 3x
Again, we can simplify this and solve for x. So Statement 2 is sufficient.
Answer: (D)
- Two teachers, Ms. Ames and Mr. Betancourt, each had N cookies. Ms. Ames was able to give the same number of cookies to each one of her 24 students, with none left over. Mr. Betancourt was also able to give the same number of cookies to each one of his 18 students, with none left over. If N > 0, what is the value of N?
(1) N<100
(2) N > 50
A. Statement 1 ALONE is sufficient to answer the question, but statement 2 alone is NOT sufficient.
B. Statement 2 ALONE is sufficient to answer the question, but statement 1 alone is NOT sufficient.
C. BOTH statements 1 and 2 TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient.
D. Each statement ALONE is sufficient to answer the question.
E. Statement 1 and 2 TOGETHER are NOT sufficient to answer the question.
Click here for a video answer and explanation to GMAT Word Problem 3!
Click here for a text answer and explanation to GMAT Word Problem 3!
This question is really about common multiples and the LCM (note that it is different than finding the set of all multiples, though!). If Ms. Ames can give each of her 24 students k cookies, so that they all get the same and none are left over, then 24k = N. Similarly, in Mr. Betancourt’s class, 18s = N.
What are the common multiples of 18 and 24?
18 = 2×9 = 2×3×3 = 6×3
24 = 3×8 = 2×2×2×3 = 6×4
From the prime factorizations, we see that GCF = 6, so the LCM is
LCM = 6×3×4 = 72
and all other common multiples of 18 and 24 are the multiples of 72: {72, 144, 216, 288, 360, …}
Statement #1: if N<100, the only possibility is N = 72. This statement, alone and by itself, is sufficient.
Statement #2: if N > 50, then N could be 72, or 144, or 216, or etc. Many possibilities. This statement, alone and by itself, is not sufficient.
Answer = (A)
- A certain zoo has mammals and reptiles and birds, and no other animals. The ratio of mammals to reptiles to birds is 11:8:5. How many birds are in the zoo?
(1) there are twelve more mammals in the zoo than there are reptiles
(2) if the zoo acquired 16 more mammals, the ratio of mammals to birds would be 3:1
A. Statement 1 ALONE is sufficient to answer the question, but statement 2 alone is NOT sufficient.
B. Statement 2 ALONE is sufficient to answer the question, but statement 1 alone is NOT sufficient.
C. BOTH statements 1 and 2 TOGETHER are sufficient to answer the question, but NEITHER statement ALONE is sufficient.
D. Each statement ALONE is sufficient to answer the question.
E. Statement 1 and 2 TOGETHER are NOT sufficient to answer the question.
Click here for a video answer and explanation to GMAT Word Problem 4!
Click here for a text answer and explanation to GMAT Word Problem 4!
A short way to do this problem. The prompt gives us ratio information. Each statement gives use some kind of count information, so each must be sufficient on its own. From that alone, we can conclude: answer = D. This is all we have to do for Data Sufficiency.
Here are the details, if you would like to see them.
Statement (1): there are twelve more mammals in the zoo than there are reptiles
From the ratio in the prompt, we know mammals are 11 “parts” and reptiles are 8 “parts”, so mammals have three more “parts” than do reptiles. If this difference of three “parts” consists of 12 mammals, that must mean there are four animals in each “part.” We have five bird “parts”, and if each counts as four animals, that’s 5*4 = 20 birds. This statement, alone and by itself, is sufficient.
Statement (2): if the zoo acquired 16 more mammals, the ratio of mammals to birds would be 3:1
Let’s say there are x animals in a “part”—this means there are currently 11x mammals and 5x birds. Suppose we add 16 mammals. Then the ratio of (11x + 16) mammals to 5x birds is 3:1.
(11x + 16)/(5x) = 3/1 = 3
11x + 16 = 3*(5x) = 15x
16 = 15x – 11x
16 = 4x
4 = x
So there are four animals in a “part”. The birds have five parts, 5x, so that’s 20 birds. This statement, alone and by itself, is sufficient.
Both statements are sufficient. Answer = D.
A Final Word on Word Problems
So, what is the trick to GMAT word problems? As you’ve seen in this post, there’s no one-size-fits-all trick—but there are plenty of strategies!
The strategies you’ve read about here can be used to take the given information and identify key words in a question. With them, you’ll be able to find everything from average speed to total distance traveled, from total time to total amount.
The key now is to put them into practice. Jot down these techniques or bookmark this post so you can come back as you continue your practice with GMAT word problems. You can also check out our posts on compound interest and Venn diagrams for more practice with GMAT word problems. Good luck!
This post was written with contributions from our Magoosh content creator, Rachel Kapelke-Dale.
-
Mike served as a GMAT Expert at Magoosh, helping create hundreds of lesson videos and practice questions to help guide GMAT students to success. He was also featured as «member of the month» for over two years at GMAT Club. Mike holds an A.B. in Physics (graduating magna cum laude) and an M.T.S. in Religions of the World, both from Harvard. Beyond standardized testing, Mike has over 20 years of both private and public high school teaching experience specializing in math and physics. In his free time, Mike likes smashing foosballs into orbit, and despite having no obvious cranial deficiency, he insists on rooting for the NY Mets. Learn more about the GMAT through Mike’s Youtube video explanations and resources like What is a Good GMAT Score? and the GMAT Diagnostic Test.
View all posts
Welcome to our Ratio Word Problems page.
Here you will find our range of Fifth Grade Ratio Problem worksheets
which will help your child apply and practice their Math skills to solve a range of ratio problems.
Ratio Word Problems
Here you will find a range of problem solving worksheets about ratio.
The sheets involve using and applying knowledge to ratios to solve problems.
The sheets have been put in order of difficulty, with the easiest first.
Each problem sheet comes complete with an answer sheet.
Using these sheets will help your child to:
- apply their ratio skills;
- apply their knowledge of fractions;
- solve a range of word problems.
All the free 5th Grade Math sheets in this section support Elementary Math Benchmarks for Fifth Grade.
Ratio Word Problems
-
Ratio Problems 1
-
Answers
-
PDF version
-
Ratio Problems 2
-
Answers
-
PDF version
-
Ratio Problems 3
-
Answers
-
PDF version
-
Ratio Problems 4
-
Answers
-
PDF version
Ratio and Probability Problems
-
Ration and Probability Problems 1
-
Sheet 1 Answers
-
PDF version
-
Ration and Probability Problems 2
-
Sheet 2 Answers
-
PDF version
More Recommended Math Worksheets
Take a look at some more of our worksheets similar to these.
More Ratio Worksheets
These sheets are a great way to introduce ratio of one object to another using visual aids.
The sheets in this section are at a more basic level than those on this page.
We also have some ratio and proportion worksheets to help learn these interrelated concepts.
More 5th Grade Math Problems
Here you will find our selection of free 5th grade math word problems.
Each sheet is availabel in both standard and metric units (where applicable).
Each sheet comes complete with a separate answer sheet.
All the problems are based around ‘real life’ such as the planets, heights of mountains, or length of rivers.
Using these sheet will help your child to:
- apply their addition, subtraction, multiplication and division skills;
- apply their knowledge of rounding and place value;
- solve a range of ‘real life’ problems.
All the worksheets help to support Elementary math benchmarks.
-
5th Grade Math Problems
Finding all Possibilities Problems
This is our finding all possibilities area where all the worksheets involve finding many different answers to the problem posed.
The sheets here encourage systematic working and logical thinking.
The problems are different in that, there is typically only one problem per sheet, but the problem may take quite a while to solve!
Math Logic Problems
This is our logic problems area where all the worksheets involve using reasoning and logical thinking skills.
The sheets here are designed to get children thinking logically and puzzling the problems out.
There are a range of different logic problems from 1st through 5th grade!
Fraction Problems
Here you will find a range of fraction word problems to help your child apply their fraction learning.
The worksheets cover a range of fraction objectives, from adding and subtracting fractions to working out fractions of numbers.
The sheets support fraction learning from 2nd grade to 5th grade.
How to Print or Save these sheets
Need help with printing or saving?
Follow these 3 easy steps to get your worksheets printed out perfectly!
-
How to Print support
How to Print or Save these sheets
Need help with printing or saving?
Follow these 3 easy steps to get your worksheets printed out perfectly!
-
How to Print support
Math-Salamanders.com
The Math Salamanders hope you enjoy using these free printable Math worksheets
and all our other Math games and resources.
We welcome any comments about our site or worksheets on the Facebook comments box at the bottom of every page.
Lesson 9: Introduction to Word Problems
/en/algebra-topics/solving-equations/content/
What are word problems?
A word problem is a math problem written out as a short story or scenario. Basically, it describes a realistic problem and asks you to imagine how you would solve it using math. If you’ve ever taken a math class, you’ve probably solved a word problem. For instance, does this sound familiar?
Johnny has 12 apples. If he gives four to Susie, how many will he have left?
You could solve this problem by looking at the numbers and figuring out what the problem is asking you to do. In this case, you’re supposed to find out how many apples Johnny has left at the end of the problem. By reading the problem, you know Johnny starts out with 12 apples. By the end, he has 4 less because he gave them away. You could write this as:
12 — 4
12 — 4 = 8, so you know Johnny has 8 apples left.
Word problems in algebra
If you were able to solve this problem, you should also be able to solve algebra word problems. Yes, they involve more complicated math, but they use the same basic problem-solving skills as simpler word problems.
You can tackle any word problem by following these five steps:
- Read through the problem carefully, and figure out what it’s about.
- Represent unknown numbers with variables.
- Translate the rest of the problem into a mathematical expression.
- Solve the problem.
- Check your work.
We’ll work through an algebra word problem using these steps. Here’s a typical problem:
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took two days, and the van cost $360. How many miles did she drive?
It might seem complicated at first glance, but we already have all of the information we need to solve it. Let’s go through it step by step.
Step 1: Read through the problem carefully.
With any problem, start by reading through the problem. As you’re reading, consider:
- What question is the problem asking?
- What information do you already have?
Let’s take a look at our problem again. What question is the problem asking? In other words, what are you trying to find out?
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
There’s only one question here. We’re trying to find out how many miles Jada drove. Now we need to locate any information that will help us answer this question.
There are a few important things we know that will help us figure out the total mileage Jada drove:
- The van cost $30 per day.
- In addition to paying a daily charge, Jada paid $0.50 per mile.
- Jada had the van for 2 days.
- The total cost was $360.
Step 2: Represent unknown numbers with variables.
In algebra, you represent unknown numbers with letters called variables. (To learn more about variables, see our lesson on reading algebraic expressions.) You can use a variable in the place of any amount you don’t know. Looking at our problem, do you see a quantity we should represent with a variable? It’s often the number we’re trying to find out.
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
Since we’re trying to find the total number of miles Jada drove, we’ll represent that amount with a variable—at least until we know it. We’ll use the variable m for miles. Of course, we could use any variable, but m should be easy to remember.
Step 3: Translate the rest of the problem.
Let’s take another look at the problem, with the facts we’ll use to solve it highlighted.
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
We know the total cost of the van, and we know that it includes a fee for the number of days, plus another fee for the number of miles. It’s $30 per day, and $0.50 per mile. A simpler way to say this would be:
$30 per day plus $0.50 per mile is $360.
If you look at this sentence and the original problem, you can see that they basically say the same thing: It cost Jada $30 per day and $0.50 per mile, and her total cost was $360. The shorter version will be easier to translate into a mathematical expression.
Let’s start by translating $30 per day. To calculate the cost of something that costs a certain amount per day, you’d multiply the per-day cost by the number of days—in other words, 30 per day could be written as 30 ⋅days, or 30 times the number of days. (Not sure why you’d translate it this way? Check out our lesson on writing algebraic expressions.)
$30 per day and $.50 per mile is $360
$30 ⋅ day + $.50 ⋅ mile = $360
As you can see, there were a few other words we could translate into operators, so and $.50 became + $.50, $.50 per mile became $.50 ⋅ mile, and is became =.
Next, we’ll add in the numbers and variables we already know. We already know the number of days Jada drove, 2, so we can replace that. We’ve also already said we’ll use m to represent the number of miles, so we can replace that too. We should also take the dollar signs off of the money amounts to make them consistent with the other numbers.
$30 ⋅ day + $.50 ⋅ mile = $360
30 ⋅ 2 + .5 ⋅ m = 360
Now we have our expression. All that’s left to do is solve it.
Step 4: Solve the problem.
This problem will take a few steps to solve. (If you’re not sure how to do the math in this section, you might want to review our lesson on simplifying expressions.) First, let’s simplify the expression as much as possible. We can multiply 30 and 2, so let’s go ahead and do that. We can also write .5 ⋅ m as 0.5m.
30 ⋅ 2 + .5 ⋅ m = 360
60 + .5m = 360
Next, we need to do what we can to get the m alone on the left side of the equals sign. Once we do that, we’ll know what m is equal to—in other words, it will let us know the number of miles in our word problem.
We can start by getting rid of the 60 on the left side by subtracting it from both sides.
The only thing left to get rid of is .5. Since it’s being multiplied with m, we’ll do the reverse and divide both sides of the equation with it.
.5m / .5 is m and 300 / 0.50 is 600, so m = 600. In other words, the answer to our problem is 600—we now know Jada drove 600 miles.
Step 5: Check the problem.
To make sure we solved the problem correctly, we should check our work. To do this, we can use the answer we just got—600—and calculate backward to find another of the quantities in our problem. In other words, if our answer for Jada’s distance is correct, we should be able to use it to work backward and find another value, like the total cost. Let’s take another look at the problem.
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
According to the problem, the van costs $30 per day and $0.50 per mile. If Jada really did drive 600 miles in 2 days, she could calculate the cost like this:
$30 per day and $0.50 per mile
30 ⋅ day + .5 ⋅ mile
30 ⋅ 2 + .5 ⋅ 600
60 + 300
360
According to our math, the van would cost $360, which is exactly what the problem says. This means our solution was correct. We’re done!
While some word problems will be more complicated than others, you can use these basic steps to approach any word problem. On the next page, you can try it for yourself.
Practice!
Let’s practice with a couple more problems. You can solve these problems the same way we solved the first one—just follow the problem-solving steps we covered earlier. For your reference, these steps are:
- Read through the problem carefully, and figure out what it’s about.
- Represent unknown numbers with variables.
- Translate the rest of the problem into a mathematical expression.
- Solve the problem.
- Check your work.
If you get stuck, you might want to review the problem on page 1. You can also take a look at our lesson on writing algebraic expressions for some tips on translating written words into math.
Problem 1
Try completing this problem on your own. When you’re done, move on to the next page to check your answer and see an explanation of the steps.
A single ticket to the fair costs $8. A family pass costs $25 more than half of that. How much does a family pass cost?
Problem 2
Here’s another problem to do on your own. As with the last problem, you can find the answer and explanation to this one on the next page.
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
Problem 1 Answer
Here’s Problem 1:
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
Answer: $29
Let’s solve this problem step by step. We’ll solve it the same way we solved the problem on page 1.
Step 1: Read through the problem carefully
The first in solving any word problem is to find out what question the problem is asking you to solve and identify the information that will help you solve it. Let’s look at the problem again. The question is right there in plain sight:
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
So is the information we’ll need to answer the question:
- A single ticket costs $8.
- The family pass costs $25 more than half the price of the single ticket.
Step 2: Represent the unknown numbers with variables
The unknown number in this problem is the cost of the family pass. We’ll represent it with the variable f.
Step 3: Translate the rest of the problem
Let’s look at the problem again. This time, the important facts are highlighted.
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
In other words, we could say that the cost of a family pass equals half of $8, plus $25. To turn this into a problem we can solve, we’ll have to translate it into math. Here’s how:
- First, replace the cost of a family pass with our variable f.
- Next, take out the dollar signs and replace words like plus and equals with operators.
- Finally, translate the rest of the problem. Half of can be written as 1/2 times, or 1/2 ⋅ :
f equals half of $8 plus $25
f = half of 8 + 25
f = 1/2 ⋅ 8 + 25
Step 4: Solve the problem
Now all we have to do is solve our problem. Like with any problem, we can solve this one by following the order of operations.
- f is already alone on the left side of the equation, so all we have to do is calculate the right side.
- First, multiply 1/2 by 8. 1/2 ⋅ 8 is 4.
- Next, add 4 and 25. 4 + 25 equals 29 .
f = 1/2 ⋅ 8 + 25
f = 4 + 25
f = 29
That’s it! f is equal to 29. In other words, the cost of a family pass is $29.
Step 5: Check your work
Finally, let’s check our work by working backward from our answer. In this case, we should be able to correctly calculate the cost of a single ticket by using the cost we calculated for the family pass. Let’s look at the original problem again.
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
We calculated that a family pass costs $29. Our problem says the pass costs $25 more than half the cost of a single ticket. In other words, half the cost of a single ticket will be $25 less than $29.
- We could translate this into this equation, with s standing for the cost of a single ticket.
- Let’s work on the right side first. 29 — 25 is 4.
- To find the value of s, we have to get it alone on the left side of the equation. This means getting rid of 1/2. To do this, we’ll multiply each side by the inverse of 1/2: 2.
1/2s = 29 — 25
1/2s = 4
s = 8
According to our math, s = 8. In other words, if the family pass costs $29, the single ticket will cost $8. Looking at our original problem, that’s correct!
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
So now we’re sure about the answer to our problem: The cost of a family pass is $29.
Problem 2 Answer
Here’s Problem 2:
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
Answer: $70
Let’s go through this problem one step at a time.
Step 1: Read through the problem carefully
Start by asking what question the problem is asking you to solve and identifying the information that will help you solve it. What’s the question here?
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
To solve the problem, you’ll have to find out how much money Mo gave to charity. All the important information you need is in the problem:
- The amount Flor donated is three times as much the amount Mo donated
- Flor and Mo’s donations add up to $280 total
Step 2: Represent the unknown numbers with variables
The unknown number we’re trying to identify in this problem is Mo’s donation. We’ll represent it with the variable m.
Step 3: Translate the rest of the problem
Here’s the problem again. This time, the important facts are highlighted.
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
The important facts of the problem could also be expressed this way:
Mo’s donation plus Flor’s donation equals $280
Because we know that Flor’s donation is three times as much as Mo’s donation, we could go even further and say:
Mo’s donation plus three times Mo’s donation equals $280
We can translate this into a math problem in only a few steps. Here’s how:
- Because we’ve already said we’ll represent the amount of Mo’s donation with the variable m, let’s start by replacing Mo’s donation with m.
- Next, we can put in mathematical operators in place of certain words. We’ll also take out the dollar sign.
- Finally, let’s write three times mathematically. Three times m can also be written as 3 ⋅ m, or just 3m.
m plus three times m equals $280
m + three times m = 280
m + 3m = 280
Step 4: Solve the problem
It will only take a few steps to solve this problem.
- To get the correct answer, we’ll have to get m alone on one side of the equation.
- To start, let’s add m and 3m. That’s 4m.
- We can get rid of the 4 next to the m by dividing both sides by 4. 4m / 4 is m, and 280 / 4 is 70.
m + 3m = 280
4m = 280
m = 70.
We’ve got our answer: m = 70. In other words, Mo donated $70.
Step 5: Check your work
The answer to our problem is $70, but we should check just to be sure. Let’s look at our problem again.
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
If our answer is correct, $70 and three times $70 should add up to $280.
- We can write our new equation like this:
- The order of operations calls for us to multiply first. 3 ⋅ 70 is 210.
- The last step is to add 70 and 210. 70 plus 210 equals 280.
70 + 3 ⋅ 70 = 280
70 + 210 = 280
280 = 280
280 is the combined cost of the tickets in our original problem. Our answer is correct: Mo gave $70 to charity.
/en/algebra-topics/distance-word-problems/content/
Though the majority of ACT math problems use diagrams or simply ask you to solve given mathematical equations, you will also see approximately 15-18 word problems on any given ACT (between 25% and 30% of the total math section). This means that knowing how best to deal with word problems will help you significantly when taking the test. Though there are many different types of ACT word problems, most of them are not nearly as difficult or cumbersome as they may appear.
This post will be your complete guide to ACT word problems: how to translate your word problems into equations and diagrams, the different types of word problems you’ll see on the test, and how best to go about solving your word problems for test day.
What Are Word Problems?
A word problem is any problem that is based mostly or entirely on written description and does not provide you with an equation, diagram, or graph. You must use your reading skills to translate the words of the question into a workable math problem and then solve for your information.
Word problems will show up on the test for a variety of reasons. Most of the time, these types of questions act to test your reading and visualization skills, as well act as a medium to deliver questions that would otherwise be untestable. For instance, if you must determine the number of sides of an unknown polygon based on given information, a diagram would certainly give the game away!
Translating Word Problems Into Equations or Drawings
In order to translate your word problems into actionable math equations that you can solve, you’ll need to know and utilize some key math terms. Whenever you see these words, you can translate them into the proper action. For instance, the word “product” means “the value of two or more values that have been multiplied together,” so if you need to find “the product of a and b,” you’ll need to set up your equation with $a * b$.
|
Key Terms |
Mathematical Action |
|
Sum, increased by, added to, total of |
+ |
|
Difference, decreased by, subtracted from |
— |
|
Product, times |
* or x |
|
Divided by |
/ or ÷ |
|
Equals, is, are, equivalent, same |
= |
|
Is less than |
< |
|
Is greater than |
> |
|
Is less than or equal to |
≤ |
|
Is greater than or equal to |
≥ |
Let’s take a look at this in action with an example problem:
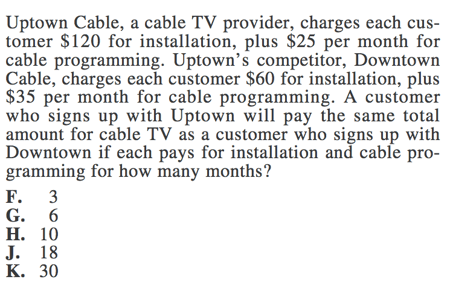
We have two different cable companies that each have different rates for installation and different monthly fees. We are asked to find out how many months it will take for the cost for each company to be the «same,» which means we must set the two rates equal.
Uptown Cable charges 120 dollars for installation plus 25 dollars a month. We do not know how many months we’re working with, so we will have:
$120 + 25x$
Downtown Cable charges 60 dollars for installation and 35 dollars per month. Again, we don’t know how many months we’re working with, but we know they will be the same, so we will have:
$60 + 35x$
And, again, because we are finding the amount of months when the cost is the «same,» we must set our rates equal.
$120 + 25x = 60 + 35x$
From here, we can solve for $x$, since it is a single variable equation.
[Note: the final answer is G, 6 months]
Learning the language of ACT word problems will help you to unravel much of the mystery of these types of questions.
Typical ACT Word Problems
ACT word problems can be grouped into two major categories: word problems where you must simply set up an equation and word problems in which you must solve for a specific piece of information.
Word Problem Type 1: Setting Up an Equation
This is the less common type of word problem on the test, but you’ll generally see it at least once or twice. You’ll also usually see this type of word problem first. For this type of question, you must use the given information to set up the equation, even though you don’t need to solve for the missing variable.
Almost always, you’ll see this type of question in the first ten questions on the test, meaning that the ACT test-makers consider them fairly “easy.” This is due to the fact that you only have to provide the set-up and not the execution.
We consider a “profit” to be any money that is gained, so we must always subtract our costs from our earnings. We know that Jones had to invest 10 million starting capital, so he is only making a profit if he has earned more than 10 million dollars. This means we can eliminate answer choices C, D, and E, as they do not account for this 10 million.
Now each boat costs Jones 7,000 dollars to make and he sells them for 20,000. This means that he earns a profit of:
$20,000 — 7,000$
$13,000$ per boat.
If $x$ represents our number of boats, then our final equation will be:
$13,000x — 10,000,000$
Our final answer is A, $13,000x — 10,000,000$
Word Problem Type 2: Solving for Your Information
Other than the few set-up word questions you’ll see, the rest of your ACT word problem questions will fall into this category. For these questions, you must both set up your equation and solve for a specific piece of information.
Most (though not all) word problem questions of this type will be scenarios or stories covering all sorts of ACT math topics, including averages, single variable equations, and probabilities, among others. You almost always must have a solid understanding of the math topic in question in order to solve the word problem on the topic.
This question is a rare example of a time in which not every piece of given information is needed to solve the problem. For most ACT word questions, all your given information will come into play at some point, but this is not the case here (though you can use all of your information, should you so choose).
For example, we are told that 25% of a given set of jelly beans are red. 25% translates to $1/4$ because 25% is the same as $25/100$ (or $1/4$). If we are being asked to find how many jelly beans are NOT red, then we know it would be $3/4$ because 100% is the same as 1, and 1 — $1/4$ = $3/4$.
So we didn’t need to know that there were 400 jellybeans to know that our final answer is H, $3/4$.
Alternatively, we could use all of our given information and find 25% of 400 in order to find the remaining jelly beans.
$400 * {1/4}$ or $400/4$
$100$
If 100 jellybeans are red, then 400 — 100 = 300 jelly beans are NOT red. This means that the not-red jelly beans make up,
$300/400$
$3/4$ of the total number of jelly beans.
Again, our final answer is H, $3/4$
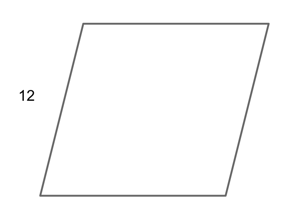
You might also be given a geometry problem as a word problem, which may or may not be set up with a scenario as well.
Geometry questions will be presented as word problems typically because the test-makers felt the problem would be too easy to solve had you been given a diagram.
The test-makers didn’t give us a diagram, so let’s make ourselves one and fill it in with what we know so far.
We know from our studies of parallelograms that opposite side pairs will be equal, so we know that the opposite side of our given will also be 12.
Now we can use this information to subtract from our total perimeter.
$72 — 12 — 12$
$48$
Again, opposite sides will be equal and we know that the sum of the two remaining sides will be 48. This means that each remaining side will be:
$48/2$
$24$
Now we have four sides in the pairings of 12 and 24.
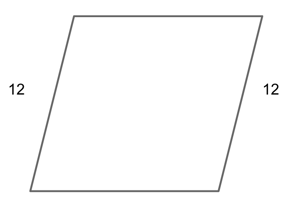
Our final answer is C, 12, 12, 24, 24.
Now, how do we put our knowledge to its best effect? Let’s take a look.
ACT Math Strategies for Your Word Problems
Though you’ll see word problems on a myriad of different types of ACT math topics, there are still a few techniques you can apply to solve your word problems as a whole.
#1: Draw It Out
Whether your problem is a geometry problem or an algebra problem, sometimes making a quick sketch of the scene can help you understand what, exactly, you’re working with. For instance, let’s look at how a picture can help you solve a ratio/division problem:
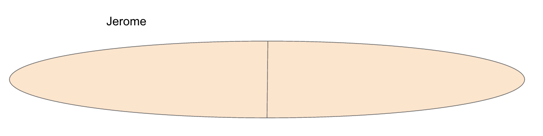
Let’s start by first drawing our sandwich and Jerome’s portion of it.
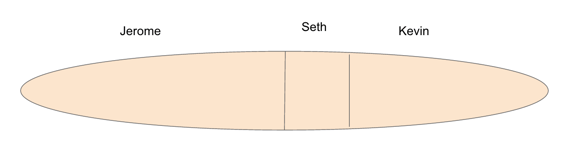
Now let’s divvy off Kevin’s portion and, by the remainder, Seth’s as well.
By seeing the problem visually, we can see that the ratio of Jerome’s share, to Kevin’s, to Seth’s will go in descending order of size. This let’s us eliminate answer choices A, B, and C, and leaves us with answer choices D and E.
Just by drawing it out and using process of elimination, and without knowing anything else about ratios, we have a 50-50 shot of guessing the right answer. And, again, without knowing anything else about fractions or ratios, we can make an educated guess between the two options. Since Jerome’s share doesn’t look twice as large as Kevin’s, our answer is probably not E.
This leaves us with our final answer D, 3:2:1.
[Note: for a breakdown on how to solve this problem using fractions and ratios instead of using a diagram and educated guessing, check out our guide to ACT fractions and ratios.]
As for geometry problems, remember—you’re often given a word problem as a word problem because it would be too simple to solve had you had a diagram to work with from the get-go. So take back the advantage and draw the picture yourself. Even a quick and dirty sketch can help you visualize the problem much easier than you can in your head and help keep all your information clear.
#2: Memorize Important Terms
If you’re not used to translating English words into mathematical equations, then ACT word problems can sound like so much nonsense and leave you floundering to set up the proper equation. Look to the chart and learn how to translate your keywords into their math equivalents. Doing so will help you to understand exactly what the problem is asking you to find.
There are free ACT math questions available online, so memorize your terms and then practice on real ACT word problems to make sure you’ve got your definitions down and can apply them to real problems.
#3: Underline and Write Out the Key Information
The key to solving a word problem is bringing together all the relevant pieces of given information and putting them in the right places. Make sure you write out all your givens on the diagram you’ve drawn (if the problem calls for a diagram) and that all your moving pieces are in order.
One of the best ways to keep all your pieces straight is to underline them in the problem and then write them out yourself before you set up your equation, so take a moment to perform this step.
#4: Pay Close Attention to Exactly What Is Being Asked of You
Little is more frustrating than solving for the wrong variable or writing in your given values in the wrong places. And yet this is entirely too easy to do when working with word problems.
Make sure you pay strict attention to exactly what you’re meant to be solving for and exactly what pieces of information go where. Are you looking for the area or the perimeter? The value of $x$ or $x + y$? Better to make sure before you start what you’re supposed to find than realize two minutes down the line that you have to solve the problem all over again.
#5: Brush Up on Any Specific Math Topic in Which You Feel Weak
You are likely to see both diagram/equation problems and word problems for any given ACT math topic on the test. Many of the topics can swing either way, which is why there are so many different types of word problems and why you’ll need to know the ins and outs of any particular math topic in order to solve its corresponding word problem. For example, if you don’t know how to properly set up a system of equations problem, you will have a difficult time of it when presented with a word problem on the topic.
So understand that solving a word problem is a two-step process: it requires you to both understand how word problems themselves work and to understand the math topic in question. If you have any areas of mathematical weakness, now is a good time to brush up on them, or else the word problem might be trickier than you were expecting.
All set? Time to shine!
Test Your Knowledge
Now to put your word problem know-how to the test with real ACT math problems.
Answers: K, C, A, E
Answer Explanations:
1) First, let us make a sketch of what we have, just so we can keep our measurements straight. We know we have two triangles, one smaller than the other, and the hypotenuse of the smaller triangle is 5.
Now our triangles are in a ratio of 2:5, so if the hypotenuse of the smaller triangle is 5, we can find the hypotenuse of the larger triangle by setting them up in a proportion.
$2/5 = 5/x$
$2x = 25$
$x = 12.5$
Our final answer is K, 12.5.
2) Because we are dealing with a hypothetical number that is increasing and decreasing based on percentage, we can solve this problem in one of two ways—by using algebra or by plugging in our own numbers.
Solving Method 1: Algebra
If we assign our hypothetical number as $x$, we can say that $x$ is increased by 25% by saying:
$x + 0.25x$
Which gives us:
$1.25x$
Now, we can decrease this value by 20% by saying:
$1.25x — (1.25x * 0.2)$
$1.25x — 0.25x$
This leaves us with:
$1x$ or 100% of our original number.
Our final answer is C, 100%.
Solving Method 2: Plugging in Numbers
Alternatively, we can use the same basic process, but make it a little simpler by using numbers instead of variables.
Let’s say our original number is 100. (Why 100? Why not! Our number can literally be anything and 100 is an easy number to work with.)
So if we need to increase 100 by 25%, we first need to find 25% of 100 and then add that to 100.
$100 + (0.25)100$
$100 + 25$
$125$
Now we need to decrease this value by 20%, so we would say:
$125 — (0.2)125$
$125 — 25$
$100$
We are left with the same number we started with, which means we are left with 100% of the number we started with.
Again, our final answer is C, 100%.
3) Let’s first begin by drawing a picture of our scene. We know that one vertex of the square is at (3, 0), so we can mark it on a coordinate plane.
Now, we are told that each side of the square is 3 cm long. To make life simple, we can start by marking all the possible vertexes attached to our known vertex at (3, 0) straight up, down, and side to side. If no answers match, we can then look to vertexes at different angles.
Our possible vertexes are:
(0, 0), (6, 0), 3, 3) and (3, -3)
One of our possible vertexes is at (6, 0 and this matches one of our answer choices, so we can stop here.
Our final answer is A, (6, 0).
4) We are told that Ms. Lopez throws out the lowest test score and then averages the remaining scores. Because Victor’s scores are already in ascending order, we can throw out the first score of 62.
Now to find the average of the remaining 4 scores, let us add them together and then divide by the number of scores.
$(78 + 83 + 84 + 93)/4$
$338/4$
$84.5$
Our final answer is E, 84.5.

Picture: John Morris/Flickr
The Take-Aways
Word problems comprise a significant portion of the ACT, so it’s a good idea to understand how they work and how to translate the words into a proper equation. But remember that translating your word problems is still only half the battle.
You must also supplement this knowledge of how to solve word problems with a solid understanding of the math topic in question. For example, it won’t do a lot of good if you can translate a probability word problem if you don’t understand exactly how probabilities work. So be sure to not only learn how to approach your word problems, but also hone your focus on any math topics you feel you need to improve upon. You can find links to all of our ACT math topic guides here to help your studies.
What’s Next?
Have friends who also need help with test prep? Share this article!
About the Author
Courtney scored in the 99th percentile on the SAT in high school and went on to graduate from Stanford University with a degree in Cultural and Social Anthropology. She is passionate about bringing education and the tools to succeed to students from all backgrounds and walks of life, as she believes open education is one of the great societal equalizers. She has years of tutoring experience and writes creative works in her free time.