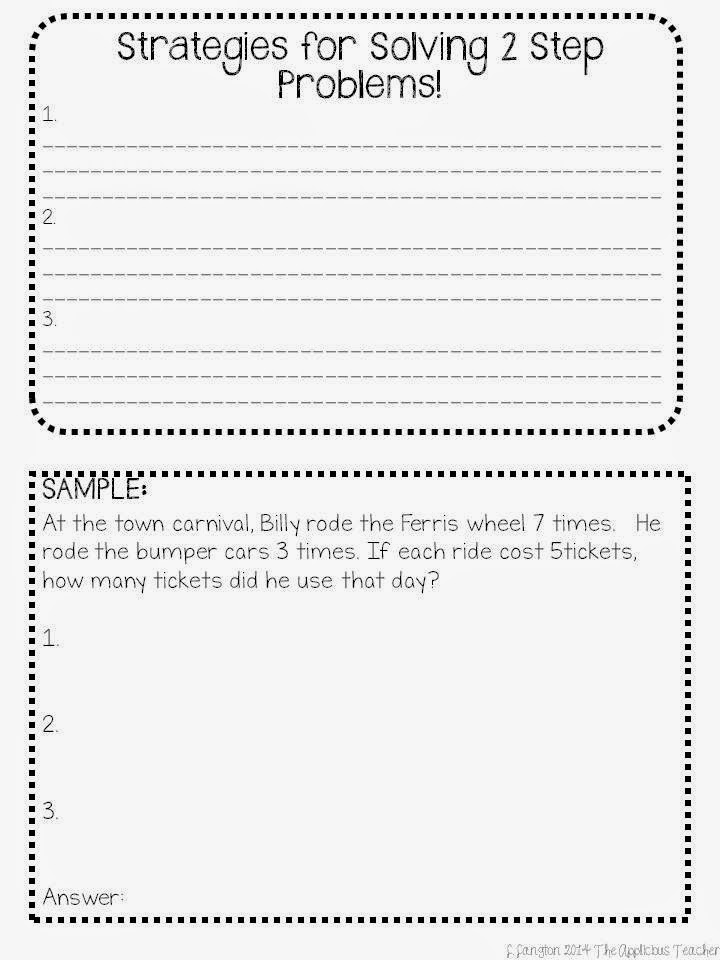
Word problems often confuse students simply because the question does not present itself in a ready-to-solve mathematical equation. You can answer even the most complex word problems, provided you understand the mathematical concepts addressed. While the degree of difficulty may change, the way to solve word problems involves a planned approach that requires identifying the problem, gathering the relevant information, creating the equation, solving and checking your work.
Identify the Problem
Begin by determining the scenario the problem wants you to solve. This might come as a question or a statement. Either way, the word problem provides you with all the information you need to solve it. Once you identify the problem, you can determine the unit of measurement for the final answer. In the following example, the question asks you to determine the total number of socks between the two sisters. The unit of measurement for this problem is pairs of socks.
«Suzy has eight pairs of red socks and six pairs of blue socks. Suzy’s brother Mark owns eight socks. If her little sister owns nine pairs of purple socks and loses two of Suzy’s pairs, how many pairs of socks do the sisters have left?»
Gather Information
Create a table, list, graph or chart that outlines the information you know, and leave blanks for any information you don’t yet know. Each word problem may require a different format, but a visual representation of the necessary information makes it easier to work with.
In the example, the question asks how many socks the sisters own together, so you can disregard the information about Mark. Also, the color of the socks doesn’t matter. This eliminates much of the information and leaves you with only the total number of socks that the sisters started with and how many the little sister lost.
Create an Equation
Translate any of the math terms into math symbols. For example, the words and phrases «sum,» «more than,» «increased» and «in addition to» all mean to add, so write in the «+» symbol over these words. Use a letter for the unknown variable, and create an algebraic equation that represents the problem.
In the example, take the total number of pairs of socks Suzy owns — eight plus six. Take the total number of pairs that her sister owns — nine. The total pairs of socks owned by both sisters is 8 + 6 + 9. Subtract the two missing pairs for a final equation of (8 + 6 + 9) — 2 = n, where n is the number of pairs of socks the sisters have left.
Solve the Problem
Using the equation, solve the problem by plugging in the values and solving for the unknown variable. Double-check your calculations along the way to prevent any mistakes. Multiply, divide and subtract in the correct order using the order of operations. Exponents and roots come first, then multiplication and division, and finally addition and subtraction.
In the example, after adding the numbers together and subtracting, you get an answer of n = 21 pairs of socks.
Verify the Answer
Check if your answer makes sense with what you know. Using common sense, estimate an answer and see if you come close to what you expected. If the answer seems absurdly large or too small, search through the problem to find where you went wrong.
In the example, you know by adding up all the numbers for the sisters that you have a maximum of 23 socks. Since the problem mentions that the little sister lost two pairs, the final answer must be less than 23. If you get a higher number, you did something wrong. Apply this logic to any word problem, regardless of the difficulty.
Download Article
Download Article
You can solve many real world problems with the help of math. In order to familiarize students with these kinds of problems, teachers include word problems in their math curriculum. However, word problems can present a real challenge if you don’t know how to break them down and find the numbers underneath the story. Solving word problems is an art of transforming the words and sentences into mathematical expressions and then applying conventional algebraic techniques to solve the problem.
-
1
Read the problem carefully.[1]
A common setback when trying to solve algebra word problems is assuming what the question is asking before you read the entire problem. In order to be successful in solving a word problem, you need to read the whole problem in order to assess what information is provided, and what information is missing.[2]
-
2
Determine what you are asked to find. In many problems, what you are asked to find is presented in the last sentence. This is not always true, however, so you need to read the entire problem carefully.[3]
Write down what you need to find, or else underline it in the problem, so that you do not forget what your final answer means.[4]
In an algebra word problem, you will likely be asked to find a certain value, or you may be asked to find an equation that represents a value.- For example, you might have the following problem: Jane went to a book shop and bought a book. While at the store Jane found a second interesting book and bought it for $80. The price of the second book was $10 less than three times the price of he first book. What was the price of the first book?
- In this problem, you are asked to find the price of the first book Jane purchased.
Advertisement
-
3
Summarize what you know, and what you need to know. Likely, the information you need to know is the same as what information you are asked to find. You also need to assess what information you already know. Again, underline or write out this information, so you can keep track of all the parts of the problem. For problems involving geometry, it is often helpful to draw a sketch at this point.[5]
- For example, you know that Jane bought two books. You know that the second book was $80. You also know that the second book cost $10 less than 3 times the price of the first book. You don’t know the price of the first book.
-
4
Assign variables to the unknown quantities. If you are being asked to find a certain value, you will likely only have one variable. If, however, you are asked to find an equation, you will likely have multiple variables. No matter how many variables you have, you should list each one, and indicate what they are equal to.[6]
-
5
Look for keywords.[7]
Word problems are full of keywords that give you clues about what operations to use. Locating and interpreting these keywords can help you translate the words into algebra.[8]
- Multiplication keywords include times, of, and factor.[9]
- Division keywords include per, out of, and percent.[10]
- Addition keywords include some, more, and together.[11]
- Subtraction keywords include difference, fewer, and decreased.[12]
- Multiplication keywords include times, of, and factor.[9]
Advertisement
-
1
Write an equation. Use the information you learn from the problem, including keywords, to write an algebraic description of the story.[13]
-
2
Solve an equation for one variable. If you have only one unknown in your word problem, isolate the variable in your equation and find which number it is equal to. Use the normal rules of algebra to isolate the variable. Remember that you need to keep the equation balanced. This means that whatever you do to one side of the equation, you must also do to the other side.[14]
-
3
Solve an equation with multiple variables. If you have more than one unknown in your word problem, you need to make sure you combine like terms to simplify your equation.
-
4
Interpret your answer. Look back to your list of variables and unknown information. This will remind you what you were trying to solve. Write a statement indicating what your answer means.[15]
Advertisement
-
1
Solve the following problem. This problem has more than one unknown value, so its equation will have multiple variables. This means you cannot solve for a specific numerical value of a variable. Instead, you will solve to find an equation that describes a variable.
- Robyn and Billy run a lemonade stand. They are giving all the money that they make to a cat shelter. They will combine their profits from selling lemonade with their tips. They sell cups of lemonade for 75 cents. Their mom and dad have agreed to double whatever amount they receive in tips. Write an equation that describes the amount of money Robyn and Billy will give to the shelter.
-
2
Read the problem carefully and determine what you are asked to find.[16]
You are asked to find how much money Robyn and Billy will give to the cat shelter. -
3
Summarize what you know, and what you need to know. You know that Robyn and Billy will make money from selling cups of lemonade and from getting tips. You know that they will sell each cup for 75 cents. You also know that their mom and dad will double the amount they make in tips. You don’t know how many cups of lemonade they sell, or how much tip money they get.
-
4
Assign variables to the unknown quantities. Since you have three unknowns, you will have three variables. Let
equal the amount of money they will give to the shelter. Let
equal the number of cups they sell. Let
equal the number of dollars they make in tips.
-
5
Look for keywords. Since they will “combine” their profits and tips, you know addition will be involved. Since their mom and dad will “double” their tips, you know you need to multiply their tips by a factor of 2.
-
6
Write an equation. Since you are writing an equation that describes the amount of money they will give to the shelter, the variable
will be alone on one side of the equation.
-
7
Interpret your answer. The variable
equals the amount of money Robyn and Billy will donate to the cat shelter. So, the amount they donate can be found by multiplying the number of cups of lemonade they sell by .75, and adding this product to the product of their tip money and 2.
Advertisement
Add New Question
-
Question
How do you solve an algebra word problem?
Daron Cam is an Academic Tutor and the Founder of Bay Area Tutors, Inc., a San Francisco Bay Area-based tutoring service that provides tutoring in mathematics, science, and overall academic confidence building. Daron has over eight years of teaching math in classrooms and over nine years of one-on-one tutoring experience. He teaches all levels of math including calculus, pre-algebra, algebra I, geometry, and SAT/ACT math prep. Daron holds a BA from the University of California, Berkeley and a math teaching credential from St. Mary’s College.
Academic Tutor
Expert Answer
Carefully read the problem and figure out what information you’re given and what that information should be used for. Once you know what you need to do with the values they’ve given you, the problem should be a lot easier to solve.
-
Question
If Deborah and Colin have $150 between them, and Deborah has $27 more than Colin, how much money does Deborah have?
Let x = Deborah’s money. Then (x — 27) = Colin’s money. That means that (x) + (x — 27) = 150. Combining terms: 2x — 27 = 150. Adding 27 to both sides: 2x = 177. So x = 88.50, and (x — 27) = 61.50. Deborah has $88.50, and Colin has $61.50, which together add up to $150.
-
Question
Karl is twice as old Bob. Nine years ago, Karl was three times as old as Bob. How old is each now?
Let x be Bob’s current age. Then Karl’s current age is 2x. Nine years ago Bob’s age was x-9, and Karl’s age was 2x-9. We’re told that nine years ago Karl’s age (2x-9) was three times Bob’s age (x-9). Therefore, 2x-9 = 3(x-9) = 3x-27. Subtract 2x from both sides, and add 27 to both sides: 18 = x. So Bob’s current age is 18, and Karl’s current age is 36, twice Bob’s current age. (Nine years ago Bob would have been 9, and Karl would have been 27, or three times Bob’s age then.)
See more answers
Ask a Question
200 characters left
Include your email address to get a message when this question is answered.
Submit
Advertisement
Video
-
Word problems can have more than one unknown and more the one variable.
-
The number of variables is always equal to the number of unknowns.
-
While solving word problems you should always read every sentence carefully and try to extract all the numerical information.
Show More Tips
Advertisement
References
About This Article
Article SummaryX
To solve word problems in algebra, start by reading the problem carefully and determining what you’re being asked to find. Next, summarize what information you know and what you need to know. Then, assign variables to the unknown quantities. For example, if you know that Jane bought 2 books, and the second book cost $80, which was $10 less than 3 times the price of the first book, assign x to the price of the 1st book. Use this information to write your equation, which is 80 = 3x — 10. To learn how to solve an equation with multiple variables, keep reading!
Did this summary help you?
Thanks to all authors for creating a page that has been read 56,462 times.
Reader Success Stories
-
James Carson
Sep 13, 2019
«I think this is amazing because it explains how and what you need to do.This helped me in algebra, and I recommend…» more
Did this article help you?
After you finish this lesson, view all of our Pre-Algebra lessons and practice problems. After you finish this lesson, view all of our Algebra 1 lessons and practice problems.
Solving Word Problems
To solve a word problem using a system of equations, it is important to;
– Identify what we don’t know
– Declare variables
– Use sentences to create equations
An example on how to do this:
Mary and Jose each bought plants from the same store. Mary spent $188 on 7 cherry trees and 11 rose bushes. Jose spent $236 on 13 cherry trees and 11 rose bushes. Find the cost of one cherry tree and the cost of one rose bush.
Cost of a cherry tree:
Cost of a rose bush:
7 cherry trees and 11 rose bushes = $188
The y-values cancel each other out, so now you are left with only x-values and real numbers.
Then, you plug in your x-value into an original equation in order to find the y-value.
Cost of a cherry tree: $8
Cost of a rose bush: $12
Example 1
Three coffees and a cupcake cost a total of dollars. Two coffees and four cupcake cost a total of
dollars. What is the individual price for a single coffee and a single cupcake?
Let’s solve this by following steps.
1. What we don’t know:
Cost of a single coffee
Cost of single cupcake
2. Declare variables:
Cost of a single coffee=
Cost of single cupcake=
3. Use sentences to create equations.
Three coffees and a cupcake cost a total of dollars.
Two coffees and four cupcake cost a total of dollars.
Now, we have a system of equations:
Let’s solve for one of the variables in one of the equations and then use that to substitute into the other.
Now, solve for the value of using the first equation.
Let’s solve the value of by substituting the value of
to the bottom equation.
Distribute to each terms inside the parenthesis
Combine like terms
Now, let’s isolate the by subtracting
on both sides.
Then divide both sides by ,
And we’ll have
Then, let’s plug the value of into one equation to get the value of
.
Cost of a single coffee=
Cost of single cupcake=
Example 2
The senior class at High School A rented and filled vans and
buses with
students. High School B rented and filled
vans and
bus with
students. Every van had the same number of students in it as did the buses. Find the number of students in each van and in each bus.
Let’s solve this by following steps.
1. What we don’t know:
Students in each van
Students in each bus
2. Declare variables:
Students in each van=
Students in each bus=
3. Use sentences to create equations.
High School A rented and filled 8 vans and 8 buses with 240 students.
High School B rented and filled 4 vans and 1 bus with 54 students.
Now, we have a system of equations:
Let’s solve for one of the variables in one of the equations and then use that to substitute into the other.
Now, solve for the value of using the second equation.
Let’s solve the value of by substituting the value of
to the bottom equation.
Distribute to each terms inside the parenthesis
Combine like terms
Now, let’s isolate the by subtracting
on both sides.
Then divide both sides by ,
And we’ll have
Then, let’s plug the value of into one equation to get the value of
.
Students in each van=
Students in each bus=
Video-Lesson Transcript
To solve a word problem using system of equations, it is important to:
1. Identify what we don’t know
2. Declare variables.
3. Use sentences to create equations.
Let’s have an example:
Mary and Jose each bought plants from the same store. Mary spent on
cherry trees and
rose bushes. Jose spent
on
cherry trees and
rose bushes. Find the cost of one cherry tree and the cost of one rose bush.
Let’s solve this by following steps above.
1. What we don’t know:
cost of a cherry tree
cost of a rose bush
2. Declare variables:
cost of a cherry tree :
cost of a rose bush :
3. Use sentences to create equations.
For Mary:
cherry trees and
rose bushes
For Jose:
cherry trees and
rose bushes
Now, we have a system of equations
We can solve this by process of substitution, elimination or fraction.
Since the value of is the same for both equations, let’s do the process of elimination.
First, let’s multiply the first equation by
Here we’ll have a negated equation
Let’s do the process of elimination now
We’ll have
Then, let’s isolate by dividing both sides by
Now, we have
Remember, our declared variable?
cost of a cherry tree :
Since
Now we can say that
cost of a cherry tree :
Now, let’s solve for the value of by getting one equation and plugging the value of
.
Let’s use the first equation to plug in
Let’s isolate by subtracting
on both sides of the equation
Then divide by
And we get
Now, we know that cost of a rose bush is .
Ah, word problems. They are SO important for our students to understand, yet one of the most challenging parts of math instruction. Word problems are unique in that they are not JUST about math – they require reading comprehension, as well. Therefore, even students who excel at math can often struggle with word problems. As teachers, it can be difficult to know which word problem strategies to teach.
In this post, I am going to share some of the common strategies for teaching word problems and why they are or aren’t effective. I would also highly encourage you to check out this post, where I share ideas for reaching all your students in your word problem instruction.
Math Word Problem Solving Strategies
There are many word problem strategies out there, but some are more effective than others. Let’s take a look at some of the different strategies and whether or not they are beneficial.

Teaching Keywords in Word Problem Instruction
Growing up, many of us were probably told that certain words in story problems indicated a specific operation. For example, “more” means addition, “fewer” means subtraction, “each” means multiplication. And while often this is the case, sometimes it is not. For example, take the following problem:
Katie picked 4 apples fewer than Marvin. If Katie picked 12 apples, how many did Marvin pick?
In this problem, the word “fewer” is used. Normally this would indicate subtraction, but if you really look at what the question is asking, you will find that we actually need to add to solve. Because of this, the keyword strategy is not effective.
That being said, I personally believe the words and language used in a problem are still worth noting. The word “fewer” is still significant; the problem lies in assuming it automatically means subtraction. As a teacher, I always taught my students to pay attention to important words, but never taught them that certain words always mean a certain operation.
Instead of using the term “keywords,” I refer to them as “important words” or “context clues”. Because reading comprehension is a major part of solving word problems, we cannot entirely ignore the language used. I do think it’s worth taking the time to encourage students to look for certain words in problems, such as the words listed above. The important thing is that we do not tell them what to do with those words – only that they are important. More on this later, but I thought it was worth noting here.
Using Attack Strategies
Another common strategy for teaching word problems is what’s known as an attack strategy. Attack strategies involve a series of steps (or “plan of attack”) to follow when solving word problems. Common attack strategies include:
- RDW (Read the problem, Draw a model, Write the equation)
- CUBES (Circle the numbers, Underline the question, Box the keywords/context clues, Evaluate the problem, Solve & check)
- RUN (Read the problem, Underline the question, Name the problem type)
- FOPS (Find the problem, Organize information with a diagram, Plan to solve the problem, Solve the problem)

There are many more – these are just a few examples. Attack strategies can certainly be helpful when used correctly. They are easy to remember and give students a clear plan for solving. Many attack strategies use fun acronyms like the ones listed above; however even strategies that do not spell out words can still be effective.
These strategies are effective largely because they focus on reading and understanding the problem first, and then solving. As we all know, many students like to simply pull out the numbers and start doing math instead of actually taking the time to read the problem. Attack strategies help solve that issue.
Numberless Word Problems
In 2014, Brian Bushart popularized the idea of removing the numbers from word problems. This is to help students understand what is actually happening in the problem. He details the process in this blog post, which is a GREAT read and I highly recommend checking it out (once you’re done reading this one!).

In short, numberless word problems are effective because they cause students to take a step back and really look at what the problem is asking. Eventually, of course, you’ll want to add the numbers back in. But starting out with the numbers removed and engaging in a discussion of what is actually happening in the problem is an effective first step in gaining comprehension.
Schema-Based Instruction
Of all the word problem strategies out there, schema-based instruction is the one with the most research backing it. Schema-based instruction (or SBI) involves categorizing word problems into particular types, or schemas, which will help you determine how to solve the problem.
Schemas can be additive or multiplicative. There are 3 main additive schemas: combine, compare, and change. Combine problems involve putting together two or more numbers to find a total. Compare involves finding the greater set, lesser set, or the difference. In change problems, an amount either increases or decreases over time.

Likewise, there are also 3 main multiplicative schemas: equal groups, comparison, and proportions. Equal groups involves a unit or group being multiplied by a specific number to find a product. In comparison problems, a set is multiplied a number of times for a product. The proportions schema focuses on the relationships among quantities.

The idea behind schema-based instruction is that all word problems fit into one of these schemas. Each schema has a unique graphic organizer and process for solving a problem that fits that schema. SBI is often accompanied with an attack strategy – such as RUN, for example. Students will first Read the problem, then Underline the questions, and finally Name the problem type (schema) before solving. This last step of identifying the schema will help students understand how to solve the problem.
Part-Part-Whole Instruction
This isn’t really a strategy, per se. But, it is an important concept for students to understand in order to be successful with word problems. Understanding part-part-whole relationships is a critical aspect of number sense. My approach to teaching word problems involves a major emphasis on part-part-whole. Granted – I taught second grade, where word problems were mostly solved by addition and subtraction. Part-part-whole works with multiplication and division, too, but looks a little bit different.
My process for walking students through word problems always included having students identify whether each number represented a part or the whole, and which (a part or the whole) was missing. This was helpful because my students knew that a missing part means subtraction, and a missing whole means addition.

Identifying what each number represented is where the “keywords” I mentioned above come in. Words like “more,” “fewer,” and “each” – while they do NOT tell us how to solve – are important context clues to help us decide whether each number given is a part or the whole.
I used the CUBES attack strategy to go with this. However, instead of boxing “keywords,” we boxed “context clues”. Again, those words are still important – they just don’t tell us how to solve. The words are important because they tell us which numbers are greater or less than other numbers, or if a certain number represents a group. All are important things to know in determining the parts and the whole.
Once students identify what each number represents, they are able to solve. I’ve found that students who were correctly able to determine the parts and the whole in a word problem were VERY successful in finding the answer.

Free Training for Word Problem Strategies
Want to learn more about how to effectively teach word problems? I’m hosting a FREE video training series that you won’t want to miss! It’s launching THIS MONDAY, 9/21, so make sure you join the waitlist so you don’t miss out. Upon signing up, you will receive my free 30-page e-book for how to differentiate word problem instruction in your classroom. CLICK HERE TO JOIN THE WAITLIST, or sign up using the form below.
How do you teach word problems?

Solving Word Problems in Mathematics
What Is a Word Problem? (And How to Solve It!)
Learn what word problems are and how to solve them in 7 easy steps.
Real life math problems don’t usually look as simple as 3 + 5 = ?. Instead, things are a bit more complex. To show this, sometimes, math curriculum creators use word problems to help students see what happens in the real world. Word problems often show math happening in a more natural way in real life circumstances.
As a teacher, you can share some tips with your students to show that in everyday life they actually solve such problems all the time, and it’s not as scary as it may seem.
As you know, word problems can involve just about any operation: from addition to subtraction and division, or even multiple operations simultaneously.
If you’re a teacher, you may sometimes wonder how to teach students to solve word problems. It may be helpful to introduce some basic steps of working through a word problem in order to guide students’ experience. So, what steps do students need for solving a word problem in math?
Steps of Solving a Word Problem
To work through any word problem, students should do the following:
1. Read the problem: first, students should read through the problem once.
2. Highlight facts: then, students should read through the problem again and highlight or underline important facts such as numbers or words that indicate an operation.
3. Visualize the problem: drawing a picture or creating a diagram can be helpful.
Students can start visualizing simple or more complex problems by creating relevant images, from concrete (like drawings of putting away cookies from a jar) to more abstract (like tape diagrams). It can also help students clarify the operations they need to carry out. (next step!)
4. Determine the operation(s): next, students should determine the operation or operations they need to perform. Is it addition, subtraction, multiplication, division? What needs to be done?
Drawing the picture can be a big help in figuring this out. However, they can also look for the clues in the words such as:
– Addition: add, more, total, altogether, and, plus, combine, in all;
– Subtraction: fewer, than, take away, subtract, left, difference;
– Multiplication: times, twice, triple, in all, total, groups;
– Division: each, equal pieces, split, share, per, out of, average.
These key words may be very helpful when learning how to determine the operation students need to perform, but we should still pay attention to the fact that in the end it all depends on the context of the wording. The same word can have different meanings in different word problems.
Another way to determine the operation is to search for certain situations, Jennifer Findley suggests. She has a great resource that lists various situations you might find in the most common word problems and the explanation of which operation applies to each situation.
5. Make a math sentence: next, students should try to translate the word problem and drawings into a math or number sentence. This means students might write a sentence such as 3 + 8 =.
Here they should learn to identify the steps they need to perform first to solve the problem, whether it’s a simple or a complex sentence.
6. Solve the problem: then, students can solve the number sentence and determine the solution. For example, 3 + 8 = 11.
7. Check the answer: finally, students should check their work to make sure that the answer is correct.
These 7 steps will help students get closer to mastering the skill of solving word problems. Of course, they still need plenty of practice. So, make sure to create enough opportunities for that!
At Happy Numbers, we gradually include word problems throughout the curriculum to ensure math flexibility and application of skills. Check out how easy it is to learn how to solve word problems with our visual exercises!
Word problems can be introduced in Kindergarten and be used through all grades as an important part of an educational process connecting mathematics to real life experience.
Happy Numbers introduces young students to the first math symbols by first building conceptual understanding of the operation through simple yet engaging visuals and key words. Once they understand the connection between these keywords and the actions they represent, they begin to substitute them with math symbols and translate word problems into number sentences. In this way, students gradually advance to the more abstract representations of these concepts.
For example, during the first steps, simple wording and animation help students realize what action the problem represents and find the connection between these actions and key words like “take away” and “left” that may signal them.
From the beginning, visualization helps the youngest students to understand the concepts of addition, subtraction, and even more complex operations. Even if they don’t draw the representations by themselves yet, students learn the connection between operations they need to perform in the problem and the real-world process this problem describes.
Next, students organize data from the word problem and pictures into a number sentence. To diversify the activity, you can ask students to match a word problem with the number sentence it represents.
Solving measurement problems is also a good way of mastering practical math skills. This is an example where students can see that math problems are closely related to real-world situations. Happy Numbers applies this by introducing more complicated forms of word problems as we help students advance to the next skill. By solving measurement word problems, students upgrade their vocabulary, learning such new terms as “difference” and “sum,” and continue mastering the connection between math operations and their word problem representations.
Later, students move to the next step, in which they learn how to create drawings and diagrams by themselves. They start by distributing light bulbs equally into boxes, which helps them to understand basic properties of division and multiplication. Eventually, with the help of Dino, they master tape diagrams!
To see the full exercise, follow this link.
The importance of working with diagrams and models becomes even more apparent when students move to more complex word problems. Pictorial representations help students master conceptual understanding by representing a challenging multi-step word problem in a visually simple and logical form. The ability to interact with a model helps students better understand logical patterns and motivates them to complete the task.
Having mentioned complex word problems, we have to show some of the examples that Happy Numbers uses in its curriculum. As the last step of mastering word problems, it is not the least important part of the journey. It’s crucial for students to learn how to solve the most challenging math problems without being intimidated by them. This only happens when their logical and algorithmic thinking skills are mastered perfectly, so they easily start talking in “math” language.
These are the common steps that may help students overcome initial feelings of anxiety and fear of difficulty of the task they are given. Together with a teacher, they can master these foundational skills and build their confidence toward solving word problems. And Happy Numbers can facilitate this growth, providing varieties of engaging exercises and challenging word problems!
Solving Word Problems Reminder
First, I reviewed with my class how to solve regular word problems. I’ve taught them the strategy, “Circle, Circle, Underline” (circle information and underline what they want them to do figure out) to help zero in on the important stuff. We solved the problem and we were ready to move on. Then, I added a second question to our previous. I showed the students how to use information gathered from our first step to solve the second question. Once we solved, we headed back to our seats to take notes on the three-step method.
You can read more about our simple chant to help with 1 step word problems here!
I used the gradual release process as teaching structure for this method and it worked really well.
Modeling Two-Step Word Problems
First, the kiddos wrote out the 3 steps to take to solve a multi-step word problem.
Practice With a Buddy
Then, it was their turn to practice with a buddy. I have my class in Kagan style groupings, so I had them turn to their shoulder partner for this part.
They talked it through with their buddy and then glued it in.
Independent Practice
Finally, they were ready to try the process on their own. They worked through the word problems just like they did with their buddy. And because their buddy was still sitting right by them, they could also double check if they had an issue.
I spot-checked each student’s notebook as they finished. This was a perfect chance for me to see who still needed help with multi-step problems.
My orange soda smelly marker check was their ticket to move on to math centers. If students were still struggling, I pulled them to my small group while the other students worked through their tubs for the week. The following day, we continued to use the 3 step process to solve multi-step problems. I wanted students to try the process more on their own again. So I passed out another half sheet and glue it into their notebooks.
I gave them 10 minutes to work through the problem. This was WAAYY more time then they would really actually need, but I wanted them to really work through the problem and it gave me a chance to spot check with the students who struggled from the previous day.
Number Talking our Answers
Once all the students were ready, we came back together as a class and shared every single person’s way of solving the problem. I wanted each student to take a moment and explain their thought process. Eventually, we’ll move to writing out the why’s and how’s but for now, talking is our first step!
I recorded everyone’s method on the whiteboard. (Yes… my new school has half chalkboard and half whiteboard… so sad!)
The results were powerful! If students used the exact same method, I wrote their names next to the work shown. It was a great start to explaining our thinking. Together our little class of 15 was able to come up with 9 different ways to solve this one problem! I loved the conversations that sparked afterward, “Why did you do that?” “Why did you do this?” “That would have been an easier way…”
This was one of the most effective strategies I’ve ever used for solving word problems! No more wondering… wait… did I do all the steps? Solved, completed, BAM! If you’re in need of some more multi-step word problem activities, be sure to check out these word problem task cards!
These differentiated cards are PERFECT for all learners in your 2nd or 3rd-grade classroom as they have word problems for 2-3 digit addition, subtraction, simple multiplication, and division. They also include multi-step problems for every section!
So do you have a little trick for solving multi-step problems? This way worked great with my students! You can grab the sheets I used here for FREE! Just confirm your email so I know you’re not a robot, and then you can download the freebie!
Word problems are a special kind of math challenge. Not only do they require computation skills, but they also test students’ reading comprehension and problem-solving abilities. This can be the perfect storm of frustration for many kids. But it doesn’t have to be so hard! Today, I’m sharing easy strategies for helping students master the word problem!
Start With Reading Comprehension
The first step in conquering word problems goes right back to good ol’ reading comprehension. Kids have a bad habit of reading word problems like they are just passing through words to get to the numbers. Blah, blah, blah. 127! Blah, blah, blah, 52! They search for numbers and then rush to DO something with those numbers.
If our students are going to find success with word problems, they need to use their good reading strategies! Teach your students to:
Reread: Slow down and reread as many times as needed
Visualize: Imagine what is taking place in the problem. What is the order of events?
Ask questions: What do I know? What do I need to find out?
Make predictions: What would be a reasonable result? Do I expect the answer to be more or less than the numbers I’m given?
Teach a Problem-Solving Routine
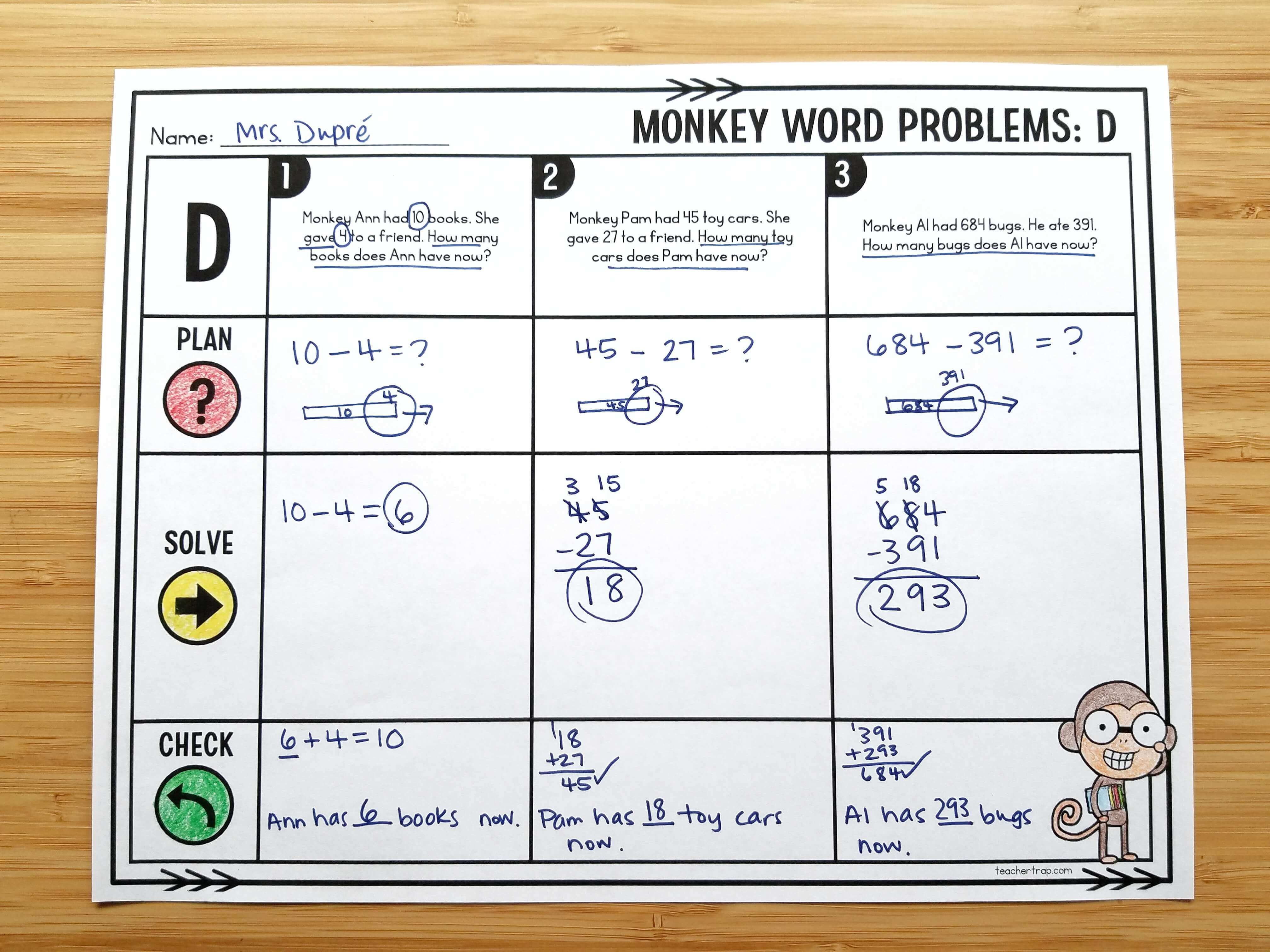
Help students form effective thinking habits by teaching and practicing a problem-solving process. I’m not talking about a rote set of steps like CUBES (Circle the numbers, Underline the question, Box key words, etc.). I’m talking about a THINKING routine that helps the child learn the critical thinking skills involved in solving real problems.
I’ve found a simple PLAN, SOLVE, CHECK format works best. With young children, the biggest issue I find is that they simply skip (or struggle with) one of these steps, which causes a breakdown in their ability to solve the problem. In fact, I form my guided math groups for word problems around those three categories (planning strategies, solving strategies, checking strategies.) **You can grab a FREE Word Problem Thinking Mat in the Preview File of my Tiered Word Problems Pack.
Compare Problem Structures
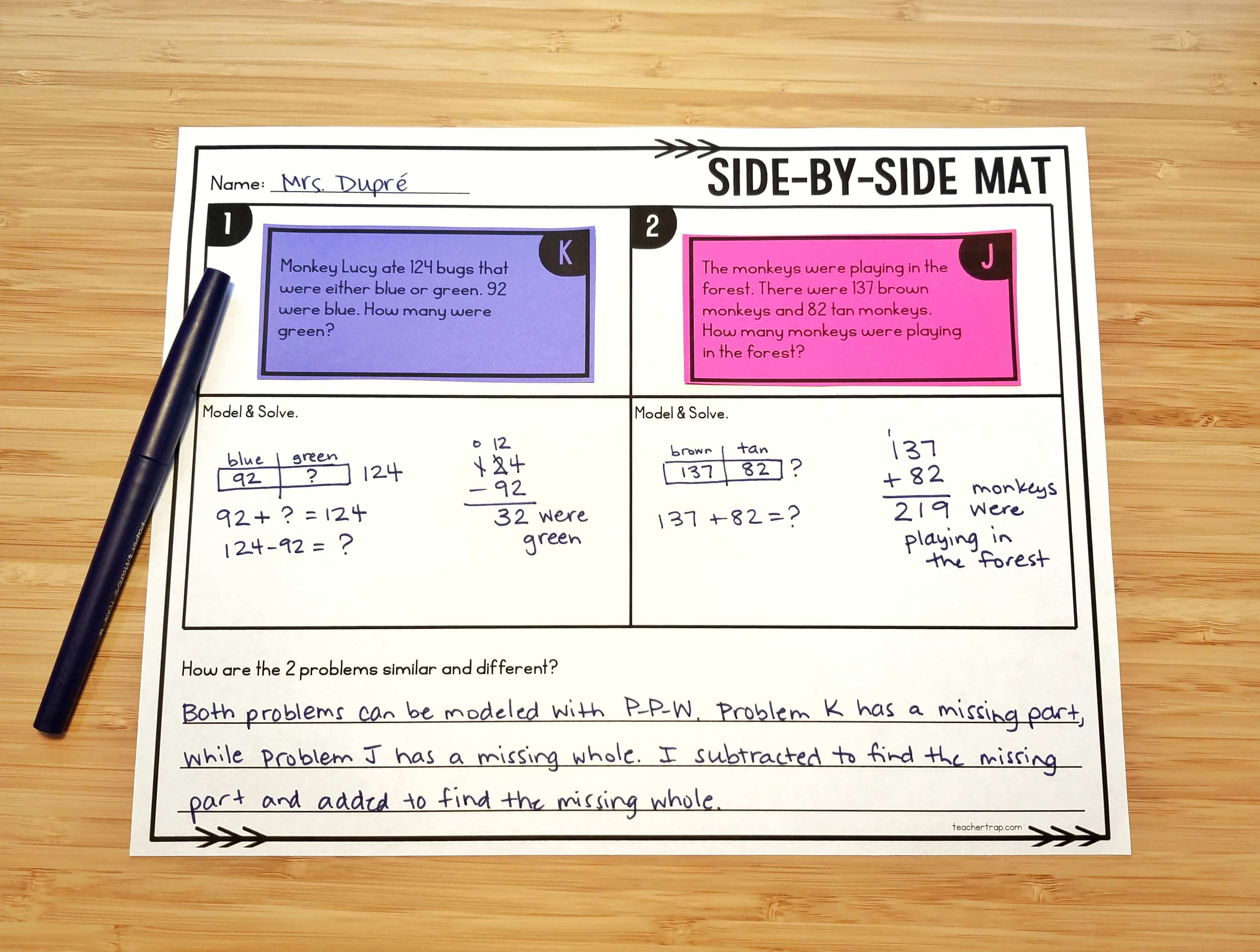
One of my favorite word problem teaching tools is something I call “Side-by-Sides.” This is simply where we solve two different problems and then compare the structure of the problem and the operation used.
When students model, solve, and discuss the different problem types, they grow a deeper understanding of how word problems work.
**You can grab FREE samples of side-by-side problems in the Preview File of my Side-by-Side Word Problems Pack or grab a FREE Side-by-Side Mat in the Preview File of my Tiered Word Problems Pack for 2nd Grade or 3rd Grade.
Start With the Easy Version
Differentiation isn’t just for reading! Many students get overwhelmed when they see large numbers in word problems, so why not meet them where they are? I use what I call “Tiered Word Problems” to help students understand word problem structures and grow confidence before tackling the on-level version.
In my Word Problem Solving Bundle, I provide all kinds of leveled word problems – Tiered Problem Pages, Task Cards, Exit Tickets, and more, that all increase in challenge from first through fifth grade. This allows me to scaffold instruction by student need, and throughout the school year.
**You can grab free goodies and sample pages from the Bundle in the Word Problem Solving Bundle Sampler Pack.
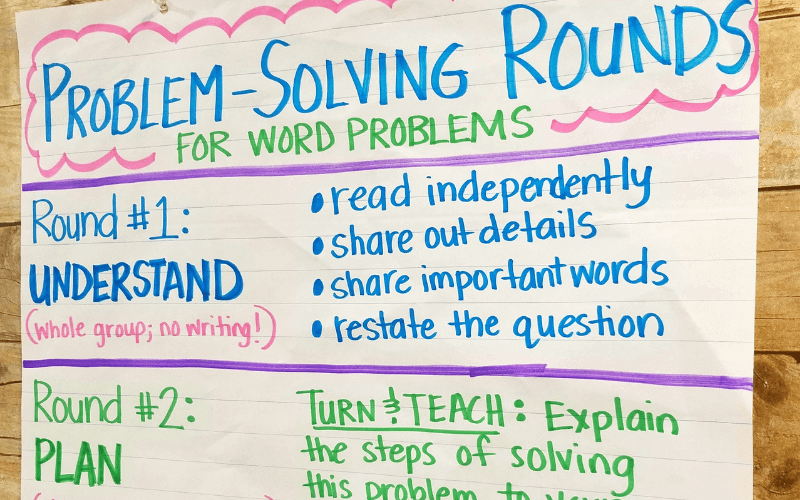
Use Problem-Solving Rounds
Problem-Solving Rounds changed math instruction in my classroom forever! This powerful routine encourages students to move through the problem-solving process I mentioned at the beginning of this post, to explain their thinking, and to reflect on other possible strategies and solutions.
Since using Problem-Solving Rounds, my students are more confident with word problems, have a deeper understanding of problem-types, and are better able to tackle new and challenging tasks.
The basic structure of the rounds follows the Problem-Solving Process I’ve shared. In Round 1, students read and discuss the problem with a focus on UNDERSTANDING. In Round 2, they turn to a partner and explain how they PLAN to solve the problem. They SOLVE the problem independently in Round 3, and in Round 4 they work with a partner and as a whole class to CHECK and reflect on their work. For a detailed guide to this process, check out my blog post all about Problem-Solving Rounds.
I’d love to hear from you! Comment below with your favorite word problem tips for teachers!
A Picture is Worth a Thousand Words
“Today, we are going to solve math word problems.” When students hear this from their math teacher, their faces drop, sweat starts to form on their foreheads and they refuse to make eye contact. As a math teacher, I understand. I understand the anxiety and want to make the learning process with word problems more enjoyable. This is where reading, writing and, math collide and the real world of math begins. Let’s talk about how to solve word problems with pictures.
When students struggle with word problems in school, they also have difficulty tackling them for homework. Most students want the “one-size-fits-all” formula for word problems but unfortunately, that does not exist. However, drawing a picture will help students visualize the problem and will start advancing their learning stage from the concrete to the abstract. Let’s take a look at how to solve a word problem using pictures:
Steps for Solving Word Problems using Pictures
- Read the entire problem: Get all the facts – Underline key word
- Answer the question: What am I looking for?
- Draw a picture or diagram: Visualize as a real world situation
- Solve the problem: Set up the equation and solve
- Check your solution: Is this answer reasonable?
Word Problem Examples – Example A
Cody has 6 pencils on his desk, Jonah has 4 more than Cody and Vinny has three less pencils than Jonah. How many pencils are there in all?
- Read the entire problem √
- What am I looking for? How many pencils do Cody, Jonah and Vinny have altogether? √
- Draw a picture or diagram √
- Solve the problem √
6 + 10 + 7 = 23 pencils
- Check your solution √
This answer is reasonable
Example B
There are 3 fish tanks labeled X, Y, and Z. Y weighs 6 times as much as X and twice as much as Z. If Z is 36 lbs. heavier than X, find the total weight of X, Y and Z.
- Read the entire problem √
- What am I looking for? What is thetotal weight of fish tanks X, Y and Z? √
- Draw a picture or diagram √
- Solve the problem √
18 + 108 + 54 = 180 pounds
- Check your solution √
This answer is reasonable
Visualizing a word problem with pictures is a strategy that will help motivate many students to begin the process of solving them. This works especially for students that may become bored by the excess amount of words instead of numbers or for those students who become overwhelmed by the information and want to break it down into a simpler form. Another benefit of using pictures when solving word problems involves communicating the results. The pictures act as justification for answers, make the problems easier to understand and therefore, secure the learning process.
Meet our Guest Blogger: Jan Rowe
Jan is one of Educational Connections’ top tutors. She has twelve years of classroom teaching experience and holds a Virginia and Florida teaching license in middle school mathematics and elementary education. Jan has been with Educational Connections for over a year, working with over twenty students. Her tutoring goal is to help each student understand their learning style so they can improve the speed and quality of that learning. When she is not tutoring or teaching, Jan loves to play scrabble, go hiking and play Frisbee with her new puppy.