In mathematics, an equation is a formula that expresses the equality of two expressions, by connecting them with the equals sign =.[2][3] The word equation and its cognates in other languages may have subtly different meanings; for example, in French an équation is defined as containing one or more variables, while in English, any well-formed formula consisting of two expressions related with an equals sign is an equation.[4]
Solving an equation containing variables consists of determining which values of the variables make the equality true. The variables for which the equation has to be solved are also called unknowns, and the values of the unknowns that satisfy the equality are called solutions of the equation. There are two kinds of equations: identities and conditional equations. An identity is true for all values of the variables. A conditional equation is only true for particular values of the variables.[5][6]
An equation is written as two expressions, connected by an equals sign («=»).[2] The expressions on the two sides of the equals sign are called the «left-hand side» and «right-hand side» of the equation. Very often the right-hand side of an equation is assumed to be zero. This does not reduce the generality, as this can be realized by subtracting the right-hand side from both sides.
The most common type of equation is a polynomial equation (commonly called also an algebraic equation) in which the two sides are polynomials.
The sides of a polynomial equation contain one or more terms. For example, the equation
has left-hand side 

An equation is analogous to a scale into which weights are placed. When equal weights of something (e.g., grain) are placed into the two pans, the two weights cause the scale to be in balance and are said to be equal. If a quantity of grain is removed from one pan of the balance, an equal amount of grain must be removed from the other pan to keep the scale in balance. More generally, an equation remains in balance if the same operation is performed on its both sides.
In Cartesian geometry, equations are used to describe geometric figures. As the equations that are considered, such as implicit equations or parametric equations, have infinitely many solutions, the objective is now different: instead of giving the solutions explicitly or counting them, which is impossible, one uses equations for studying properties of figures. This is the starting idea of algebraic geometry, an important area of mathematics.
Algebra studies two main families of equations: polynomial equations and, among them, the special case of linear equations. When there is only one variable, polynomial equations have the form P(x) = 0, where P is a polynomial, and linear equations have the form ax + b = 0, where a and b are parameters. To solve equations from either family, one uses algorithmic or geometric techniques that originate from linear algebra or mathematical analysis. Algebra also studies Diophantine equations where the coefficients and solutions are integers. The techniques used are different and come from number theory. These equations are difficult in general; one often searches just to find the existence or absence of a solution, and, if they exist, to count the number of solutions.
Differential equations are equations that involve one or more functions and their derivatives. They are solved by finding an expression for the function that does not involve derivatives. Differential equations are used to model processes that involve the rates of change of the variable, and are used in areas such as physics, chemistry, biology, and economics.
The «=» symbol, which appears in every equation, was invented in 1557 by Robert Recorde, who considered that nothing could be more equal than parallel straight lines with the same length.[1]
IntroductionEdit
Analogous illustrationEdit
Illustration of a simple equation; x, y, z are real numbers, analogous to weights.
An equation is analogous to a weighing scale, balance, or seesaw.
Each side of the equation corresponds to one side of the balance. Different quantities can be placed on each side: if the weights on the two sides are equal, the scale balances, and in analogy, the equality that represents the balance is also balanced (if not, then the lack of balance corresponds to an inequality represented by an inequation).
In the illustration, x, y and z are all different quantities (in this case real numbers) represented as circular weights, and each of x, y, and z has a different weight. Addition corresponds to adding weight, while subtraction corresponds to removing weight from what is already there. When equality holds, the total weight on each side is the same.
Parameters and unknownsEdit
Equations often contain terms other than the unknowns. These other terms, which are assumed to be known, are usually called constants, coefficients or parameters.
An example of an equation involving x and y as unknowns and the parameter R is
When R is chosen to have the value of 2 (R = 2), this equation would be recognized in Cartesian coordinates as the equation for the circle of radius of 2 around the origin. Hence, the equation with R unspecified is the general equation for the circle.
Usually, the unknowns are denoted by letters at the end of the alphabet, x, y, z, w, …, while coefficients (parameters) are denoted by letters at the beginning, a, b, c, d, … . For example, the general quadratic equation is usually written ax2 + bx + c = 0.
The process of finding the solutions, or, in case of parameters, expressing the unknowns in terms of the parameters, is called solving the equation. Such expressions of the solutions in terms of the parameters are also called solutions.
A system of equations is a set of simultaneous equations, usually in several unknowns for which the common solutions are sought. Thus, a solution to the system is a set of values for each of the unknowns, which together form a solution to each equation in the system. For example, the system
has the unique solution x = −1, y = 1.
IdentitiesEdit
An identity is an equation that is true for all possible values of the variable(s) it contains. Many identities are known in algebra and calculus. In the process of solving an equation, an identity is often used to simplify an equation, making it more easily solvable.
In algebra, an example of an identity is the difference of two squares:
which is true for all x and y.
Trigonometry is an area where many identities exist; these are useful in manipulating or solving trigonometric equations. Two of many that involve the sine and cosine functions are:
and
which are both true for all values of θ.
For example, to solve for the value of θ that satisfies the equation:
where θ is limited to between 0 and 45 degrees, one may use the above identity for the product to give:
yielding the following solution for θ:
Since the sine function is a periodic function, there are infinitely many solutions if there are no restrictions on θ. In this example, restricting θ to be between 0 and 45 degrees would restrict the solution to only one number.
PropertiesEdit
Two equations or two systems of equations are equivalent, if they have the same set of solutions. The following operations transform an equation or a system of equations into an equivalent one – provided that the operations are meaningful for the expressions they are applied to:
- Adding or subtracting the same quantity to both sides of an equation. This shows that every equation is equivalent to an equation in which the right-hand side is zero.
- Multiplying or dividing both sides of an equation by a non-zero quantity.
- Applying an identity to transform one side of the equation. For example, expanding a product or factoring a sum.
- For a system: adding to both sides of an equation the corresponding side of another equation, multiplied by the same quantity.
If some function is applied to both sides of an equation, the resulting equation has the solutions of the initial equation among its solutions, but may have further solutions called extraneous solutions. For example, the equation has the solution Raising both sides to the exponent of 2 (which means applying the function to both sides of the equation) changes the equation to , which not only has the previous solution but also introduces the extraneous solution, Moreover, if the function is not defined at some values (such as 1/x, which is not defined for x = 0), solutions existing at those values may be lost. Thus, caution must be exercised when applying such a transformation to an equation.
The above transformations are the basis of most elementary methods for equation solving, as well as some less elementary ones, like Gaussian elimination.
AlgebraEdit
Polynomial equationsEdit
The solutions –1 and 2 of the polynomial equation x2 – x + 2 = 0 are the points where the graph of the quadratic function y = x2 – x + 2 cuts the x-axis.
In general, an algebraic equation or polynomial equation is an equation of the form
- , or
- [a]
where P and Q are polynomials with coefficients in some field (e.g., rational numbers, real numbers, complex numbers). An algebraic equation is univariate if it involves only one variable. On the other hand, a polynomial equation may involve several variables, in which case it is called multivariate (multiple variables, x, y, z, etc.).
For example,
is a univariate algebraic (polynomial) equation with integer coefficients and
is a multivariate polynomial equation over the rational numbers.
Some polynomial equations with rational coefficients have a solution that is an algebraic expression, with a finite number of operations involving just those coefficients (i.e., can be solved algebraically). This can be done for all such equations of degree one, two, three, or four; but equations of degree five or more cannot always be solved in this way, as the Abel–Ruffini theorem demonstrates.
A large amount of research has been devoted to compute efficiently accurate approximations of the real or complex solutions of a univariate algebraic equation (see Root finding of polynomials) and of the common solutions of several multivariate polynomial equations (see System of polynomial equations).
Systems of linear equationsEdit
A system of linear equations (or linear system) is a collection of linear equations involving one or more variables.[b] For example,
is a system of three equations in the three variables x, y, z. A solution to a linear system is an assignment of numbers to the variables such that all the equations are simultaneously satisfied. A solution to the system above is given by
since it makes all three equations valid. The word «system» indicates that the equations are to be considered collectively, rather than individually.
In mathematics, the theory of linear systems is a fundamental part of linear algebra, a subject which is used in many parts of modern mathematics. Computational algorithms for finding the solutions are an important part of numerical linear algebra, and play a prominent role in physics, engineering, chemistry, computer science, and economics. A system of non-linear equations can often be approximated by a linear system (see linearization), a helpful technique when making a mathematical model or computer simulation of a relatively complex system.
GeometryEdit
Analytic geometryEdit
The blue and red line is the set of all points (x,y) such that x+y=5 and —x+2y=4, respectively. Their intersection point, (2,3), satisfies both equations.
A conic section is the intersection of a plane and a cone of revolution.
In Euclidean geometry, it is possible to associate a set of coordinates to each point in space, for example by an orthogonal grid. This method allows one to characterize geometric figures by equations. A plane in three-dimensional space can be expressed as the solution set of an equation of the form , where and are real numbers and are the unknowns that correspond to the coordinates of a point in the system given by the orthogonal grid. The values are the coordinates of a vector perpendicular to the plane defined by the equation. A line is expressed as the intersection of two planes, that is as the solution set of a single linear equation with values in or as the solution set of two linear equations with values in
A conic section is the intersection of a cone with equation and a plane. In other words, in space, all conics are defined as the solution set of an equation of a plane and of the equation of a cone just given. This formalism allows one to determine the positions and the properties of the focuses of a conic.
The use of equations allows one to call on a large area of mathematics to solve geometric questions. The Cartesian coordinate system transforms a geometric problem into an analysis problem, once the figures are transformed into equations; thus the name analytic geometry. This point of view, outlined by Descartes, enriches and modifies the type of geometry conceived of by the ancient Greek mathematicians.
Currently, analytic geometry designates an active branch of mathematics. Although it still uses equations to characterize figures, it also uses other sophisticated techniques such as functional analysis and linear algebra.
Cartesian equationsEdit
A Cartesian coordinate system is a coordinate system that specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, that are marked using the same unit of length.
One can use the same principle to specify the position of any point in three-dimensional space by the use of three Cartesian coordinates, which are the signed distances to three mutually perpendicular planes (or, equivalently, by its perpendicular projection onto three mutually perpendicular lines).
Cartesian coordinate system with a circle of radius 2 centered at the origin marked in red. The equation of a circle is (x − a)2 + (y − b)2 = r2 where a and b are the coordinates of the center (a, b) and r is the radius.
The invention of Cartesian coordinates in the 17th century by René Descartes (Latinized name: Cartesius) revolutionized mathematics by providing the first systematic link between Euclidean geometry and algebra. Using the Cartesian coordinate system, geometric shapes (such as curves) can be described by Cartesian equations: algebraic equations involving the coordinates of the points lying on the shape. For example, a circle of radius 2 in a plane, centered on a particular point called the origin, may be described as the set of all points whose coordinates x and y satisfy the equation x2 + y2 = 4.
Parametric equationsEdit
A parametric equation for a curve expresses the coordinates of the points of the curve as functions of a variable, called a parameter.[7][8] For example,
are parametric equations for the unit circle, where t is the parameter. Together, these equations are called a parametric representation of the curve.
The notion of parametric equation has been generalized to surfaces, manifolds and algebraic varieties of higher dimension, with the number of parameters being equal to the dimension of the manifold or variety, and the number of equations being equal to the dimension of the space in which the manifold or variety is considered (for curves the dimension is one and one parameter is used, for surfaces dimension two and two parameters, etc.).
Number theoryEdit
Diophantine equationsEdit
A Diophantine equation is a polynomial equation in two or more unknowns for which only the integer solutions are sought (an integer solution is a solution such that all the unknowns take integer values). A linear Diophantine equation is an equation between two sums of monomials of degree zero or one. An example of linear Diophantine equation is ax + by = c where a, b, and c are constants. An exponential Diophantine equation is one for which exponents of the terms of the equation can be unknowns.
Diophantine problems have fewer equations than unknown variables and involve finding integers that work correctly for all equations. In more technical language, they define an algebraic curve, algebraic surface, or more general object, and ask about the lattice points on it.
The word Diophantine refers to the Hellenistic mathematician of the 3rd century, Diophantus of Alexandria, who made a study of such equations and was one of the first mathematicians to introduce symbolism into algebra. The mathematical study of Diophantine problems that Diophantus initiated is now called Diophantine analysis.
Algebraic and transcendental numbersEdit
An algebraic number is a number that is a solution of a non-zero polynomial equation in one variable with rational coefficients (or equivalently — by clearing denominators — with integer coefficients). Numbers such as π that are not algebraic are said to be transcendental. Almost all real and complex numbers are transcendental.
Algebraic geometryEdit
Algebraic geometry is a branch of mathematics, classically studying solutions of polynomial equations. Modern algebraic geometry is based on more abstract techniques of abstract algebra, especially commutative algebra, with the language and the problems of geometry.
The fundamental objects of study in algebraic geometry are algebraic varieties, which are geometric manifestations of solutions of systems of polynomial equations. Examples of the most studied classes of algebraic varieties are: plane algebraic curves, which include lines, circles, parabolas, ellipses, hyperbolas, cubic curves like elliptic curves and quartic curves like lemniscates, and Cassini ovals. A point of the plane belongs to an algebraic curve if its coordinates satisfy a given polynomial equation. Basic questions involve the study of the points of special interest like the singular points, the inflection points and the points at infinity. More advanced questions involve the topology of the curve and relations between the curves given by different equations.
Differential equationsEdit
A differential equation is a mathematical equation that relates some function with its derivatives. In applications, the functions usually represent physical quantities, the derivatives represent their rates of change, and the equation defines a relationship between the two. Because such relations are extremely common, differential equations play a prominent role in many disciplines including physics, engineering, economics, and biology.
In pure mathematics, differential equations are studied from several different perspectives, mostly concerned with their solutions — the set of functions that satisfy the equation. Only the simplest differential equations are solvable by explicit formulas; however, some properties of solutions of a given differential equation may be determined without finding their exact form.
If a self-contained formula for the solution is not available, the solution may be numerically approximated using computers. The theory of dynamical systems puts emphasis on qualitative analysis of systems described by differential equations, while many numerical methods have been developed to determine solutions with a given degree of accuracy.
Ordinary differential equationsEdit
An ordinary differential equation or ODE is an equation containing a function of one independent variable and its derivatives. The term «ordinary» is used in contrast with the term partial differential equation, which may be with respect to more than one independent variable.
Linear differential equations, which have solutions that can be added and multiplied by coefficients, are well-defined and understood, and exact closed-form solutions are obtained. By contrast, ODEs that lack additive solutions are nonlinear, and solving them is far more intricate, as one can rarely represent them by elementary functions in closed form: Instead, exact and analytic solutions of ODEs are in series or integral form. Graphical and numerical methods, applied by hand or by computer, may approximate solutions of ODEs and perhaps yield useful information, often sufficing in the absence of exact, analytic solutions.
Partial differential equationsEdit
A partial differential equation (PDE) is a differential equation that contains unknown multivariable functions and their partial derivatives. (This is in contrast to ordinary differential equations, which deal with functions of a single variable and their derivatives.) PDEs are used to formulate problems involving functions of several variables, and are either solved by hand, or used to create a relevant computer model.
PDEs can be used to describe a wide variety of phenomena such as sound, heat, electrostatics, electrodynamics, fluid flow, elasticity, or quantum mechanics. These seemingly distinct physical phenomena can be formalised similarly in terms of PDEs. Just as ordinary differential equations often model one-dimensional dynamical systems, partial differential equations often model multidimensional systems. PDEs find their generalisation in stochastic partial differential equations.
Types of equationsEdit
Equations can be classified according to the types of operations and quantities involved. Important types include:
- An algebraic equation or polynomial equation is an equation in which both sides are polynomials (see also system of polynomial equations). These are further classified by degree:
- linear equation for degree one
- quadratic equation for degree two
- cubic equation for degree three
- quartic equation for degree four
- quintic equation for degree five
- sextic equation for degree six
- septic equation for degree seven
- octic equation for degree eight
- A Diophantine equation is an equation where the unknowns are required to be integers
- A transcendental equation is an equation involving a transcendental function of its unknowns
- A parametric equation is an equation in which the solutions for the variables are expressed as functions of some other variables, called parameters appearing in the equations
- A functional equation is an equation in which the unknowns are functions rather than simple quantities
- Equations involving derivatives, integrals and finite differences:
- A differential equation is a functional equation involving derivatives of the unknown functions, where the function and its derivatives are evaluated at the same point, such as . Differential equations are subdivided into ordinary differential equations for functions of a single variable and partial differential equations for functions of multiple variables
- An integral equation is a functional equation involving the antiderivatives of the unknown functions. For functions of one variable, such an equation differs from a differential equation primarily through a change of variable substituting the function by its derivative, however this is not the case when the integral is taken over an open surface
- An integro-differential equation is a functional equation involving both the derivatives and the antiderivatives of the unknown functions. For functions of one variable, such an equation differs from integral and differential equations through a similar change of variable.
- A functional differential equation of delay differential equation is a function equation involving derivatives of the unknown functions, evaluated at multiple points, such as
- A difference equation is an equation where the unknown is a function f that occurs in the equation through f(x), f(x−1), …, f(x−k), for some whole integer k called the order of the equation. If x is restricted to be an integer, a difference equation is the same as a recurrence relation
- A stochastic differential equation is a differential equation in which one or more of the terms is a stochastic process
See alsoEdit
- Formula
- History of algebra
- Indeterminate equation
- List of equations
- List of scientific equations named after people
- Term (logic)
- Theory of equations
- Cancelling out
NotesEdit
- ^ As such an equation can be rewritten P – Q = 0, many authors do not consider this case explicitly.
- ^ The subject of this article is basic in mathematics, and is treated in a lot of textbooks. Among them, Lay 2005, Meyer 2001, and Strang 2005 contain the material of this article.
ReferencesEdit
- ^ a b Recorde, Robert, The Whetstone of Witte … (London, England: Jhon Kyngstone, 1557), the third page of the chapter «The rule of equation, commonly called Algebers Rule.»
- ^ a b «Equation — Math Open Reference». www.mathopenref.com. Retrieved 2020-09-01.
- ^ «Equations and Formulas». www.mathsisfun.com. Retrieved 2020-09-01.
- ^ Marcus, Solomon; Watt, Stephen M. «What is an Equation?». Retrieved 2019-02-27.
- ^ Lachaud, Gilles. «Équation, mathématique». Encyclopædia Universalis (in French).
- ^ «A statement of equality between two expressions. Equations are of two types, identities and conditional equations (or usually simply «equations»)». « Equation », in Mathematics Dictionary, Glenn James [de] et Robert C. James (éd.), Van Nostrand, 1968, 3 ed. 1st ed. 1948, p. 131.
- ^ Thomas, George B., and Finney, Ross L., Calculus and Analytic Geometry, Addison Wesley Publishing Co., fifth edition, 1979, p. 91.
- ^ Weisstein, Eric W. «Parametric Equations.» From MathWorld—A Wolfram Web Resource. http://mathworld.wolfram.com/ParametricEquations.html
External linksEdit
- Winplot: General Purpose plotter that can draw and animate 2D and 3D mathematical equations.
- Equation plotter: A web page for producing and downloading pdf or postscript plots of the solution sets to equations and inequations in two variables (x and y).
An equation is a mathematical statement with an ‘equal to’ symbol between two expressions that have equal values. For example, 3x + 5 = 15. There are different types of equations like linear, quadratic, cubic, etc. Let us learn more about equations in math in this article.
| 1. | What are Equations? |
| 2. | Parts of an Equation |
| 3. | How to Solve an Equation? |
| 4. | Types of Equations |
| 5. | Equation vs Expression |
| 6. | FAQs on Equations |
What are Equations?
Equations are mathematical statements containing two algebraic expressions on both sides of an ‘equal to (=)’ sign. It shows the relationship of equality between the expression written on the left side with the expression written on the right side. In every equation in math, we have, L.H.S = R.H.S (left hand side = right hand side). Equations can be solved to find the value of an unknown variable representing an unknown quantity. If there is no ‘equal to’ symbol in the statement, it means it is not an equation. It will be considered as an expression. You will learn the difference between equation and expression in the later section of this article.
Look at the following examples. These will give you an idea of the meaning of an equation in math.
| Equations | Is it an equation? | |
|---|---|---|
| 1. | y = 8x — 9 | Yes |
| 2. | y + x2 — 7 | No, because there is no ‘equal to’ symbol. |
| 3. | 7 + 2 = 10 — 1 | Yes |
Now, let us move forward and learn about parts of an equation in math.
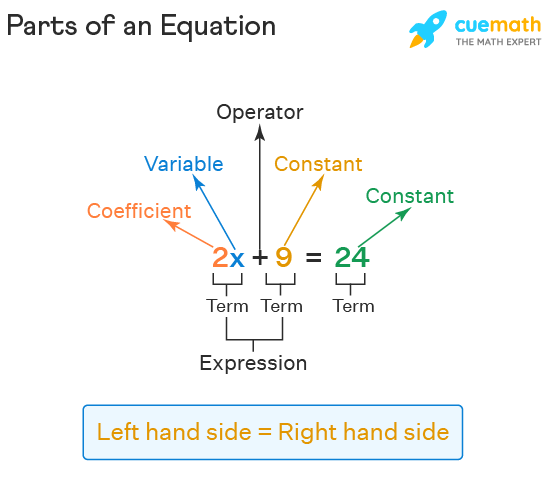
Parts of an Equation
There are different parts of an equation which include coefficients, variables, operators, constants, terms, expressions, and an equal to sign. When we write an equation, it is mandatory to have an «=» sign, and terms on both sides. Both sides should be equal to each other. An equation doesn’t need to have multiple terms on either of the sides, having variables, and operators. An equation can be formed without these as well, for example, 5 + 10 = 15. This is an arithmetic equation with no variables. As opposed to this, an equation with variables is an algebraic equation. Look at the image below to understand the parts of an equation.
How to Solve an Equation?
An equation is like a weighing balance with equal weights on both sides. If we add or subtract the same number from both sides of an equation, it still holds. Similarly, if we multiply or divide the same number into both sides of an equation, it still holds. Consider the equation of a line, 3x − 2 = 4. We will perform mathematical operations on the LHS and the RHS so that the balance is not disturbed. Let’s add 2 on both sides to reduce the LHS to 3x. This will not disturb the balance. The new LHS is 3x − 2 + 2 = 3x and the new RHS is 4 + 2 = 6. So, the equation becomes 3x = 6. Now, let’s divide both sides by 3 to reduce the LHS to x. Thus, the solution of the given equation of a line is x = 2.
The steps to solve a basic equation with one variable (linear) are given below:
- Step 1: Bring all the terms with variables on one side and all the constants on the other side of the equation by applying arithmetic operations on both sides.
- Step 2: Combine all like terms (terms containing the same variable with the same exponent) by adding/subtracting them.
- Step 3: Simplify it and get the answer.
Let us take one more example of a basic equation: 3x — 20 = 7. To bring all the constants on RHS, we have to add 20 to both sides. This implies, 3x — 20 + 20 = 7 + 20, which can be simplified as 3x = 27. Now, divide both sides by 3. This will get you x = 9 which is the required solution of the equation.
Types of Equations
Based on the degree, equations can be classified into three types. Following are the three types of equations in math:
- Linear Equations
- Quadratic Equations
- Cubic Equations
Linear Equation
Equations with 1 as the degree are known as linear equations in math. In such equations, 1 is the highest exponent of terms. These can be further classified into linear equations in one variable, two-variable linear equations, with three variables, etc. The standard form of a linear equation with variables X and Y are aX + bY — c = 0, where a and b are the coefficients of X and Y respectively and c is the constant.
Quadratic Equation
Equations with degree 2 are known as quadratic equations. The standard form of a quadratic equation with variable x is ax2 + bx + c = 0, where a ≠ 0. These equations can be solved by splitting the middle term, completing the square, or by the discriminant method.
Cubic Equations
Equations with degree 3 are known as cubic equations. Here, 3 is the highest exponent of at least one of the terms. The standard form of a cubic equation with variable x is ax3 + bx2 + cx + d = 0, where a ≠ 0.
Equation vs Expression
Expressions and equations in math are used simultaneously in algebra, but there is a major difference between the two terms. When 2x + 4 is an expression, 2x + 4 = 0 is considered an equation. Let us understand the basic difference between equation and expression through the table given below:
| Equation | Expression |
|---|---|
| When two expressions are equal in value and written together with an ‘equal to’ sign in between, it is known as an equation in math. | It is a mathematical statement having at least one term or multiple terms connected through operators in between. |
| It has an equal to «=» sign. | An expression does not contain an equal to «=» sign. |
| It can be solved to find the value of the unknown quantity. | It can be simplified to the lowest form. |
| Example: x — 8 = 16, 6y = 33, 3z — 7y = 9, etc. | Example: x — 8, 6y, 3z — 7y — 9, etc. |
Important Notes on Equations in Math:
- The values of the variable that makes an equation true are called the solution or root of the equation.
- The solution of an equation is unaffected if the same number is added, subtracted, multiplied, or divided into both sides of the equation.
- The graph of a linear equation in one or two variables is a straight line.
- The curve of the quadratic equation is in the form of a parabola.
☛ Related Topics:
Check these interesting articles related to the concept of equations in math.
- System of Equations
- Simple Equations and Its Applications
- Solve for x
FAQs on Equation
What is an Equation in Math?
An equation in math is an equality relationship between two expressions written on both sides of the equal to sign. For example, 3y = 16 is an equation.
What is a Linear Equation?
A linear equation is an equation with degree 1. It means the highest exponent of any term could be 1. An example of a linear equation in math is x + y = 24.
What is a Quadratic Equation?
A quadratic equation is an equation with degree 2. It can have any number of variables but the highest power of terms could be only 2. The standard form of a quadratic equation with variable y is ay2 + by + c = 0, where a ≠ 0.
How Equations are Used in Real Life?
In real life, there are many situations in which equations can be used. Whenever an unknown quantity has to be found, an equation can be formed and solved. For example, if the cost of 1 pencil is $1.2, and the total money spent by you on pencils is $9.6, the number of pencils bought can be found by forming an equation based on the given information. Let the number of pencils bought be x. Then, the equation will be 1.2x = 9.6, which can be solved as x = 8.
How to Solve Quadratic Equations?
Quadratic equations in one variable can be solved using the following methods:
- Factorization method
- Completing the square method
- Discriminant method
What are the 3 Types of Equation?
Based on the degree, equations can be classified into the following three types:
- Linear equation
- Quadratic equation
- Cubic equation
What Equation Has No Solution?
Equations of two parallel lines have no solutions as they do not intersect at any point. To identify the equations of parallel lines, we have to compare the coefficients of both the variables in the given two linear equations in two variables. If the ratio of coefficients is the same and unequal to the ratio of constants, it means those equations have no solution. For two equations ax + by + c = 0 and px + qy + r = 0, it will have no solution when a/p = b/q ≠ c/r.
What is the Equation of a Circle?
The equation of a circle with radius r and center (x1, y1) is (x − x1)2 + (y − y1)2 = r2.
e·qua·tion
(ĭ-kwā′zhən, -shən)
n.
1. The act or process of equating or of being equated.
2. The state of being equal.
3. Mathematics A statement asserting the equality of two expressions, usually written as a linear array of symbols that are separated into left and right sides and joined by an equal sign.
4. Chemistry A representation of a chemical reaction, usually written as a linear array in which the symbols and quantities of the reactants are separated from those of the products by an arrow or a set of opposing arrows.
5. A complex of variable elements or factors: «The world was full of equations … there must be an answer for everything, if only you knew how to set forth the questions» (Anne Tyler).
e·qua′tion·al adj.
e·qua′tion·al·ly adv.
American Heritage® Dictionary of the English Language, Fifth Edition. Copyright © 2016 by Houghton Mifflin Harcourt Publishing Company. Published by Houghton Mifflin Harcourt Publishing Company. All rights reserved.
equation
(ɪˈkweɪʒən; -ʃən)
n
1. (Mathematics) a mathematical statement that two expressions are equal: it is either an identity in which the variables can assume any value, or a conditional equation in which the variables have only certain values (roots)
2. the act of regarding as equal; equating
3. the act of making equal or balanced; equalization
4. a situation, esp one regarded as having a number of conflicting elements: what you want doesn’t come into the equation.
5. the state of being equal, equivalent, or equally balanced
6. a situation or problem in which a number of factors need to be considered
eˈquational adj
eˈquationally adv
Collins English Dictionary – Complete and Unabridged, 12th Edition 2014 © HarperCollins Publishers 1991, 1994, 1998, 2000, 2003, 2006, 2007, 2009, 2011, 2014
e•qua•tion
(ɪˈkweɪ ʒən, -ʃən)
n.
1. the act of equating or making equal.
2. the state of being equated or equal.
3. equally balanced state; equilibrium.
4. an expression or a proposition, often algebraic, asserting the equality of two quantities.
5. a symbolic representation in chemistry showing the kind and amount of the starting materials and products of a reaction.
[1350–1400; Middle English < Latin]
Random House Kernerman Webster’s College Dictionary, © 2010 K Dictionaries Ltd. Copyright 2005, 1997, 1991 by Random House, Inc. All rights reserved.
e·qua·tion
(ĭ-kwā′zhən)
1. Mathematics A written statement indicating the equality of two expressions. It consists of a sequence of symbols that is split into left and right sides joined by an equal sign. For example, 2 + 3 + 5 = 10 is an equation.
2. Chemistry A written representation of a chemical reaction, in which the symbols and amounts of the reactants are separated from those of the products by an equal sign, arrow, or a set of opposing arrows. For example, NaOH + HCl = NaCl + H2O is an equation.
The American Heritage® Student Science Dictionary, Second Edition. Copyright © 2014 by Houghton Mifflin Harcourt Publishing Company. Published by Houghton Mifflin Harcourt Publishing Company. All rights reserved.
ThesaurusAntonymsRelated WordsSynonymsLegend:
| Noun | 1. | math, mathematics, maths — a science (or group of related sciences) dealing with the logic of quantity and shape and arrangement regression equation, regression of y on x — the equation representing the relation between selected values of one variable (x) and observed values of the other (y); it permits the prediction of the most probable values of y simultaneous equations — a set of equations in two or more variables for which there are values that can satisfy all the equations simultaneously |
| 2. | equation — a state of being essentially equal or equivalent; equally balanced; «on a par with the best»
status, position — the relative position or standing of things or especially persons in a society; «he had the status of a minor»; «the novel attained the status of a classic»; «atheists do not enjoy a favorable position in American life» egalite, egality — social and political equality; «egality represents an extreme leveling of society» tie — equality of score in a contest |
|
| 3. | equation — the act of regarding as equal |
Based on WordNet 3.0, Farlex clipart collection. © 2003-2012 Princeton University, Farlex Inc.
equation
noun equating, match, agreement, balancing, pairing, comparison, parallel, equality, correspondence, likeness, equivalence, equalization the equation between higher spending and higher taxes
Collins Thesaurus of the English Language – Complete and Unabridged 2nd Edition. 2002 © HarperCollins Publishers 1995, 2002
equation
noun
The state of being equivalent:
The American Heritage® Roget’s Thesaurus. Copyright © 2013, 2014 by Houghton Mifflin Harcourt Publishing Company. Published by Houghton Mifflin Harcourt Publishing Company. All rights reserved.
Translations
rovnice
ligningregnestykke
yhtälö
jednadžba
egyenletkiegyenlítés
jafnaefnajafna
等しくすること
방정식
rovnica
enačba
ekvation
สมการ
phương trình
equation
[ɪˈkweɪʒən] N
2. (= linking) the equation of sth with sth → la identificación de algo con algo
Collins Spanish Dictionary — Complete and Unabridged 8th Edition 2005 © William Collins Sons & Co. Ltd. 1971, 1988 © HarperCollins Publishers 1992, 1993, 1996, 1997, 2000, 2003, 2005
Collins English/French Electronic Resource. © HarperCollins Publishers 2005
equation
Collins German Dictionary – Complete and Unabridged 7th Edition 2005. © William Collins Sons & Co. Ltd. 1980 © HarperCollins Publishers 1991, 1997, 1999, 2004, 2005, 2007
Collins Italian Dictionary 1st Edition © HarperCollins Publishers 1995
equate
(iˈkweit) verb
to regard as the same in some way. He equates money with happiness.
eˈquation (-ʒən) noun
1. a statement that two things are equal or the same. xy+xy=2xy is an equation.
2. a formula expressing the action of certain substances on others. 2H2 + O2 = 2 H2O is an equation.
Kernerman English Multilingual Dictionary © 2006-2013 K Dictionaries Ltd.
equation
→ مُعادَلة rovnice ligning Gleichung εξίσωση ecuación yhtälö équation jednadžba equazione 等しくすること 방정식 vergelijking likning równanie equação равенство ekvation สมการ eşitlik phương trình 等式
Multilingual Translator © HarperCollins Publishers 2009
- equation
- [ɪ’kweɪʒ(ə)n]
сущ.
1) приравнивание, уравнивание; отождествление
She emphatically rejects the automatic equation of anatomical gender with the corresponding sociocultural roles. — Она категорически отвергает автоматическое отождествление биологического пола с соответствующими социокультурными ролями.
That wailing was prescribed to send off a daughter suggests a symbolic equation of marriage and death. (G. Cooper, Life-Cycle Rituals in Dongyang County: Time, Affinity, and Exchange in Rural China) — Тот факт, что на проводах дочери полагалось рыдать, наводит на мысль о символическом отождествлении замужества со смертью.
Syn:
2) уравновешивание; равновесие, баланс
Balancing work and family — I’m forever trying to come up with the perfect equation in that regard. — Работа и семья — я всё время пытаюсь найти в этом вопросе золотую середину.
3)
The final variable in the equation is hype. To advertise or not to advertise, that is the question. — Последней переменной в этом уравнении является реклама. Рекламировать или не рекламировать — таков вопрос.
I want to ask you both about money, which is always a big part of the equation in Hollywood. — Я хочу спросить вас обоих про деньги, в Голливуде они всегда играют большую роль.
Neapolitans earned the right to claim pizza as their own by entering a tomato into the equation. — Неаполитанцы заслужили право называть пиццу своим изобретением, добавив в число ингредиентов помидор.
according to an equation — по уравнению
to formulate / state an equation — сформулировать уравнение
to solve / work an equation — решить уравнение
an equation in one unknown — уравнение с одним неизвестным
— simple equation
— integral equation
— linear equation
— quadratic equation
— equation of the first order5)
; = chemical equation уравнение
Англо-русский современный словарь.
2014.
Полезное
Смотреть что такое «equation» в других словарях:
-
Equation — Équation (mathématiques) Cet article concerne les équations mathématiques dans leur généralité. Pour une introduction au concept, voir Équation (mathématiques élémentaires). … Wikipédia en Français
-
équation — [ ekwasjɔ̃ ] n. f. • 1613; h. XIIIe « égalité »; lat. æquatio 1 ♦ (1637) Math. Relation conditionnelle existant entre deux quantités et dépendant de certaines variables (ou inconnues). Poser une équation. Mettre en équation un phénomène complexe … Encyclopédie Universelle
-
Equation — E*qua tion, n. [L. aequatio an equalizing: cf. F. [ e]quation equation. See {Equate}.] 1. A making equal; equal division; equality; equilibrium. [1913 Webster] Again the golden day resumed its right, And ruled in just equation with the night.… … The Collaborative International Dictionary of English
-
equation — e‧qua‧tion [ɪˈkweɪʒn] noun [countable] a statement in mathematics, showing that two quantities are equal acˈcounting eˌquation one of the relationships between assets and liabilities used in accounting: • The accounting equation here is: assets… … Financial and business terms
-
equation — [ē kwā′zhən, ikwā′zhən] n. [ME equacioun < L aequatio] 1. the act of equating; equalization 2. the state of being equated; equality, equivalence, or balance; also, identification or association 3. a) a complex whole [the human equation] b) an… … English World dictionary
-
equation — index balance (equality), comparison, parity Burton s Legal Thesaurus. William C. Burton. 2006 … Law dictionary
-
equation — late 14c., a term in astrology; meaning “action of making equal” is from 1650s; mathematical sense is from 1560s, on notion of equalizing the expressions; from L. aequationem (nom. aequatio) an equal distribution, community, from pp. stem of… … Etymology dictionary
-
equation — ► NOUN 1) the process of equating one thing with another. 2) Mathematics a statement that the values of two mathematical expressions are equal (indicated by the sign =). 3) Chemistry a symbolic representation of the changes which occur in a… … English terms dictionary
-
Équation — Cet article concerne les équations mathématiques dans leur généralité. Pour une introduction au concept, voir Équation (mathématiques élémentaires). … Wikipédia en Français
-
Equation — This article is about equations in mathematics. For the chemistry term, see chemical equation. The first use of an equals sign, equivalent to 14x+15=71 in modern notation. From The Whetstone of Witte by Robert Recorde (1557). An equation is a… … Wikipedia
-
equation — /i kway zheuhn, sheuhn/, n. 1. the act of equating or making equal; equalization: the symbolic equation of darkness with death. 2. equally balanced state; equilibrium. 3. Math. an expression or a proposition, often algebraic, asserting the… … Universalium
English[edit]
Alternative forms[edit]
- æquation (archaic)
Etymology[edit]
From Old French, from Latin aequātiō (“an equalizing”).
Morphologically equate + -ion
Pronunciation[edit]
- enPR: ĭkwā’zhən, IPA(key): /ɪˈkweɪʒən/; enPR: ĭkwā’shən, IPA(key): /ɪˈkweɪʃən/
- Rhymes: -eɪʒən
Noun[edit]
equation (plural equations)
- The act or process of equating two or more things, or the state of those things being equal (that is, identical).
- We need to bring the balance of power into equation
-
2013, Eva Illouz, Why Love Hurts: A Sociological Explanation:
-
The cultural equation of love with suffering is similar to the equation of love with an experience of both transcendence and consummation in which love is affirmed in an ostentatious display of self loss.
-
- (mathematics) An assertion that two expressions are equal, expressed by writing the two expressions separated by an equal sign; from which one is to determine a particular quantity.
- (astronomy) A small correction to observed values to remove the effects of systematic errors in an observation.
Derived terms[edit]
- absolute personal equation
- affected equation
- Airy equation
- algebraic equation
- Arrhenius equation
- Cassie equation
- Cauchy-Riemann equation
- Chazy equation
- chemical equation
- cubic equation
- delay differential equation
- difference equation
- differential equation
- Diophantine equation
- Dirac equation
- Drake equation
- Duffing equation
- Einstein field equation
- enter into the equation
- enter the equation
- equation division
- equation of motion
- equation of time
- Ernst equation
- Euler-Lagrange equation
- exponential equation
- Fermat equation
- Flory-Fox equation
- generalized estimating equation
- Hagen-Poiseuille equation
- half-equation
- Hartree equation
- Henderson-Hasselbalch equation
- integral equation
- Kepler’s equation
- Lagrange’s equations
- Laguerre’s equation
- Lanchester equation
- Laplace’s equation
- Legendre’s differential equation
- Lewin’s equation
- light equation
- linear equation
- Lotka-Volterra equation
- Manning’s equation
- MOJS equation
- Morison equation
- Navier-Stokes equation
- Nernst equation
- net ionic equation
- ordinary differential equation
- parametric equation
- partial differential equation
- Pell’s equation
- Penman equation
- personal equation
- polar equation
- polynomial equation
- Price equation
- Price’s equation
- quadratic equation
- rate equation
- Riccati equation
- Schrödinger equation
- Schrodinger equation
- Schrödinger’s equation
- Schrodinger’s equation
- Schrödinger’s wave equation
- Schroedinger equation
- Schroedinger’s equation
- Slutsky equation
- stochastic differential equation
- Sylvester equation
- time-independent Schrödinger equation
- Van der Waals equation
- Volterra integral equation
- wave equation
- Wheeler-DeWitt equation
- Young-Laplace equation
[edit]
- equality
- equational
- identity
- inequation
- inequality
Translations[edit]
mathematics: assertion
- Afrikaans: vergelyking
- Albanian: ekuacion (sq) m
- Arabic: مُعَادَلَة (ar) f (muʕādala)
- Armenian: հավասարում (hy) (havasarum)
- Azerbaijani: tənlik, təngləxmə
- Basque: ekuazio
- Belarusian: раўна́нне n (raŭnánnje)
- Bengali: সমীকরণ (bn) (śomikoron)
- Bulgarian: уравне́ние n (uravnénie)
- Burmese: ညီမျှခြင်း (my) (nyihmya.hkrang:)
- Catalan: equació f
- Chinese:
- Mandarin: 方程式 (zh) (fāngchéngshì), 方程 (zh) (fāngchéng)
- Czech: rovnice (cs) f
- Danish: ligning (da) c
- Dutch: vergelijking (nl)
- Esperanto: ekvacio (eo)
- Estonian: võrrand
- Faroese: líkning f
- Finnish: yhtälö (fi)
- French: équation (fr) f
- Georgian: გასწორება (gasc̣oreba)
- German: Gleichung (de) f
- Greek: εξίσωση (el) f (exísosi)
- Greenlandic: assigiissitaq
- Hebrew: משוואה מִשְׁוָאָה (he) f (mishva’á)
- Hindi: समीकरण (hi) m (samīkraṇ)
- Hungarian: egyenlet (hu)
- Icelandic: jafna f
- Ido: equaciono (io)
- Indonesian: persamaan (id)
- Irish: cothromóid (ga)
- Italian: equazione (it) f
- Japanese: 方程式 (ja) (ほうていしき, hōteishiki)
- Kazakh: теңдеу (teñdeu)
- Khmer: សមីការ (saʼməykaa)
- Korean: 방정식(方程式) (ko) (bangjeongsik)
- Kurdish:
- Central Kurdish: ھاوکێشە (ckb) (hawkêşe)
- Northern Kurdish: hevkêşe (ku)
- Kyrgyz: теңдеме (ky) (teŋdeme)
- Lao: ສົມຜົນ (lo) (som phon), ສົມມະການ (som ma kān), ສົມການ (som kān)
- Latin: aequatio f
- Latvian: vienādojums m
- Lithuanian: lygtis m
- Macedonian: равенка f (ravenka)
- Malay: persamaan (ms)
- Malayalam: സമവാക്യം (samavākyaṃ)
- Maori: whārite
- Marathi: समीकरण n (samīkraṇ)
- Mongolian:
- Cyrillic: тэгшитгэл (mn) (tegšitgel)
- Neapolitan: equazzione f
- Nepali: समीकरण (samīkaraṇ)
- Norwegian:
- Bokmål: likning (no) m or f
- Oromo: qixxaatoo
- Pashto: معادله f (ma’ādela)
- Persian: معادله (fa) (mo’âdele)
- Polish: równanie (pl) n
- Portuguese: equação (pt) f
- Quechua: paqtachani
- Romanian: ecuație (ro) f
- Russian: уравне́ние (ru) n (uravnénije)
- Serbo-Croatian:
- Cyrillic: (Bosnian, Serbian) једна̀чина f, (Croatian) једна̀џба f
- Roman: (Bosnian, Serbian) jednàčina (sh) f, (Croatian) jednàdžba (sh) f
- Slovak: rovnica f
- Slovene: enačba (sl) f
- Spanish: ecuación (es) f
- Swahili: mlinganyo
- Swedish: ekvation (sv) c
- Tagalog: tumbasan
- Tajik: муодила (tg) (muodila)
- Tamil: சமன்பாடு (ta) (camaṉpāṭu)
- Tatar: тигезләмә (tigezlämä)
- Thai: สมการ (th) (sà-má-gaan)
- Tibetan: please add this translation if you can
- Turkish: denklem (tr)
- Ukrainian: рівня́ння n (rivnjánnja)
- Urdu: مساوات f (musāvāt)
- Uyghur: تەڭلىمە (tenglime)
- Uzbek: tenglama (uz)
- Vietnamese: phương trình (vi) (方程)
- Yiddish: גלייכונג (gleykhung)