What is a word problem for addition

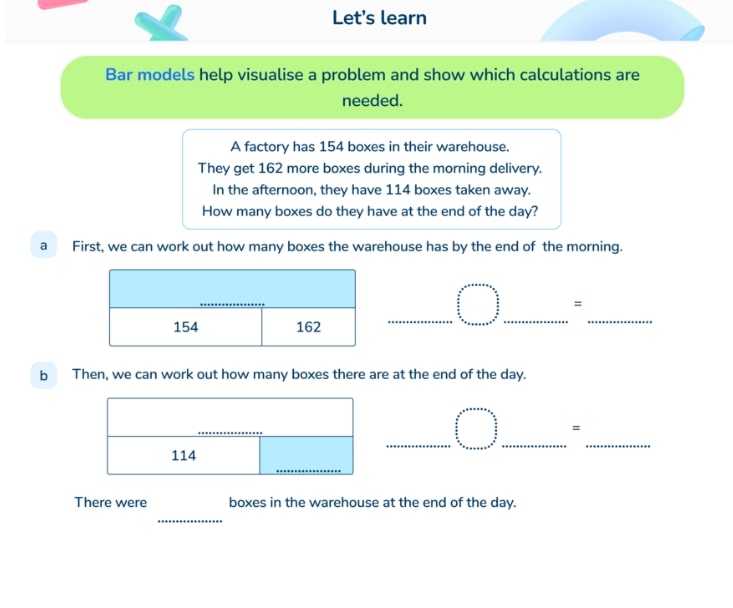
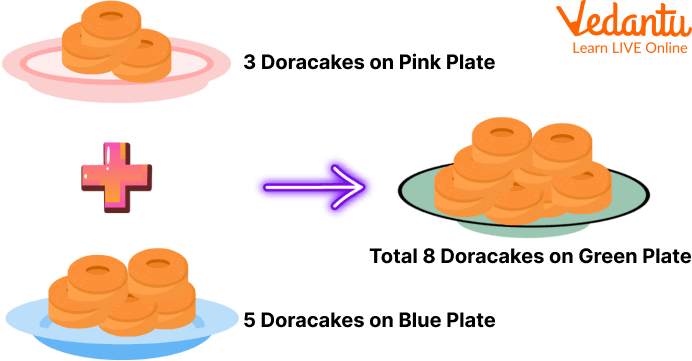
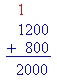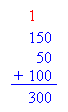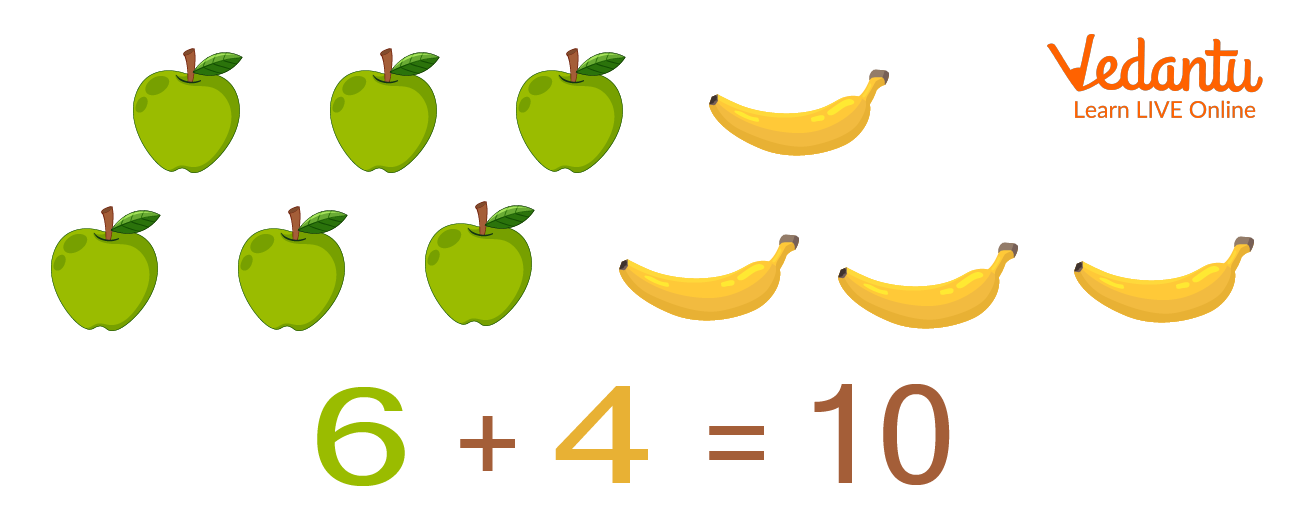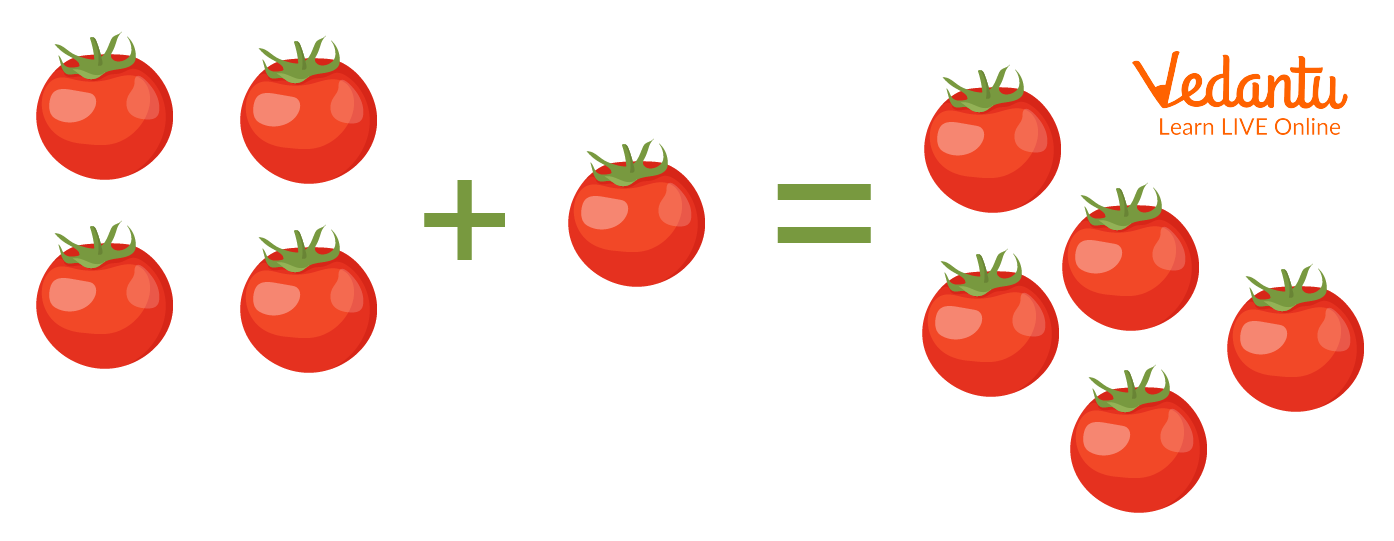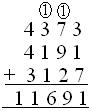 Addition word problems arise in any situations where there is a gain or an increase of something as a result of combining one or more numbers. Think of addition as combining parts to form a whole.

Problem #4:

Ana found a 15 dollar bill on the floor on Saturday.

Then on Sunday her parents gave her 155 dollars.
How much does Ana have all together?

CLUE:
Saturday~15 dollars
Sunday~155 dollars

Solution:
15+155 = 170 dollars all together

Addition word problems appear throughout KS1 and KS2. Children are introduced to simple addition and subtraction word problems in the early years. This knowledge is then built upon throughout primary school, right up to Year 6, when pupils work with complex multi-step word problems involving large whole numbers and decimal numbers.

In the early stages, in Key Stage one and lower Key Stage two, addition word problems are taught through the use of concrete resources and visual images. As pupils become more confident with the formal written methods, children progress to use these to help solve more complex addition and subtraction word problems.

Children benefit from regular exposure to word problems, alongside any fluency work they are doing. To help you with this, we have put together a collection of 25 addition word problems with answer keys, which can be used with pupils from Year 2 to Year 6.

Addition word problems in the National Curriculum

Addition word problems in Year 1

Pupils in Year 1 are introduced to simple one-step problems involving place value addition and subtraction word problems, through the use of concrete objects, pictorial representations and missing number problems. At this stage, children should be memorising and reasoning with number bonds to 10 and 20. They also need to understand the effect of adding zero.

Addition word problems in Year 2

In Year 2, pupils continue to use concrete objects and pictorial representations to solve addition problems, including those involving numbers, quantities, measures and simple money word problems. They are also expected to apply their increasing knowledge of mental and written methods,  using one and two-digit numbers. Pupils start working on basic written addition and are introduced to the concept of regrouping.

Addition word problems in Year 3

By Year 3, pupils are starting to tackle more complex addition word problems. They begin to use written methods to add 3-digit numbers, using formal column method for addition. Pupils also begin solving addition problems involving fractions, in addition to building on their mental addition skills to add a three-digit number and ones, tens and hundreds. 

Addition word problems in Year 4

Pupils in Year 4 progress to solving two-step problems in contexts, deciding which operations and methods to use and why. They are also beginning to work with larger numbers, adding up to four digits using the formal written method of column addition.

Addition word problems in Year 5

By Year 5, pupils are solving multi-step word problems in contexts. They add whole numbers with more than four digits and decimal numbers, using formal written methods. Children are also adding increasingly larger numbers mentally.

Third Space Learning’s online one-to-one tutoring programmes work with students to fill their learning gaps and build confidence in maths. Tailored to the needs of each student, Third Space Learning’s tutoring programmes offer students more opportunities to practice their maths skills and reach their year group standard.

Addition word problems in Year 6

Pupils in Year 6 continue to work with larger numbers and decimals, solving increasingly complex multi-step problems, deciding which operations to use and why. By this stage students should have a solid grasp of the written methods for all four operations.

Why are word problems important for children’s understanding of addition

Word problems help children to make sense of addition. In the Early Years, simple story problems aid understanding of what is being asked, alongside the use of concrete resources and visual images. Real-life word problems are an important element of the maths curriculum for children throughout the school, giving them the opportunity to put the addition skills they have learnt into context, to understand how they can be used in the outside world. 

How to teach addition word problem solving in primary school

Pupils need to be taught how to approach word problems, beginning by reading the questions carefully and making sure they fully understand what is being asked. The next step is to determine what calculation is needed and whether there are concrete resources or pictorial representations  they can use to help solve it, or if 

Here is an example:

There were 3,756 people on a cruise ship.

456 people get on after the first week, whilst 623 get off.

How many passengers are on board the ship now after the first week?

How to solve:

What do you already know?

• There are 3,756 on the cruise ship at the start of the journey.
• 456 people get on after the first week, therefore we need to add 456 to 3,746. This gives us a total of 4,212 people
• 623 people get off, so we need to subtract 623 from the total number of passengers now on the boat. When we subtract 623 from 4,212, we get a total of 3,589.

How can this be represented pictorially?

• We can draw 2 bar models to represent this problem.
• The number of passengers who got on the ship after the first week is added to the  total number of people on the boat at the start of the trip. 
• We then draw a second bar model to show how many passengers were left on the ship once the 623 passengers got off after the first week.

Addition word problems for year 2

Addition word problems in Year 2 require pupils to work with 1 and 2-digit numbers. At this stage it is important children are working with concrete resources to support their understanding. Children also begin to learn how to check their answers by finding the inverse of  calculations.

Addition word problems for year 3

Word problems for Year 3 require pupils to work with larger numbers. By this stage, children are becoming more confident with the formal written column  method and are able to use this to solve 2 and 3 digit addition word problems. Addition word problems can be incorporated into other areas of the maths curriculum, for example, through time word problems. 

Addition word problems for year 4

With word problems for Year 4, pupils progress onto 4 digit addition word problems. They are also beginning to solve more complex, two-step word problems. Children should be encouraged to estimate and find the inverse, to check the accuracy of their calculations. In Year 4, pupils also solve addition word problems involving  fractions and decimals.

Addition word problems for year 5

Word problems for Year 5 require pupils to solve addition word problems with larger numbers (more than 4 digits), using formal written methods and  mentally. Word problems become more complex multi-step problems, involving other operations. Pupils at this stage should continue to be encouraged to check their answers by finding the inverse.

Addition word problems for year 6

With word problems for Year 6, pupils need to be able to solve word problems using larger whole numbers of up to 6 or 7 digits, problems involving fraction word problems and problems involving decimals.  Addition word problems in SATs include one-step, two-step and more complex multi-step problems.

Looking for more word problems resources?

Take a look at our comprehensive collection of word problems practice questions covering a wide range of topics such as ratio word problems, percentages word problems, multiplication word problems, division word problems and more. 

Addition is the most basic mathematical operation that we can use for calculations. We need to practice using real-life situations and for that word problems are the best. Addition is based on certain properties, i.e. closure, commutative, and associative. Closure property is when two or more whole numbers are added to get a whole number. Commutative property is when two or more whole numbers are added but the result is not dependent on the order of addends. Associative property is when we add three or more numbers and the result obtained is the same and does not depend on the grouping of the addends.

Addition of Two Types of Fruits

Adding Dora Cakes

In the below examples, we will see some word problems for Class 2 with solutions.

Example 1. Let us take two numbers 30 and 68. What is the sum of those two numbers?

Ans: One number = 30

Other number = 68

Therefore, sum of those two numbers = 30 + 68 = 98

Example 2. If there are 45 boys and 34 girls. What is the total number of students present in the classroom?

Ans: Number of boys in the classroom = 45

Number of girls in the classroom = 34

Therefore, total number of students in the classroom = 45 + 34 = 79

Example 3. Prithvi scored a brave 88 runs in the first match of the Ranji Trophy and scored 62 runs in the second match of the Ranji Trophy group stage. Find the total number of runs scored by him in the two matches.

Ans: Runs scored in first match= 88

Runs scored in the second match= 62

Total runs scored in those two matches = 88 + 62 = 150

2 + 8 = 10 so we carry 1

Now, 8 + 6 = 14 and we add that carried 1 here making it 15

So, the total is 150.

Example 4. There are 58 books on a bookshelf in the central library. 18 more books are kept on the same bookshelf later on. What is the total number of books on that shelf now?

Ans: Books on the bookshelf before = 58

Books on the bookshelf after the addition of new books= 18

Total number of books within the bookshelf = 58 + 18 = 76

8 + 8 = 16 so we carry 1

Now, 5 + 1 = 6 and we add that carried 1 here making it 7

So, the total is 76.

Practice Problem Sums for Class 2

Q1. Shankar went to the local sweet shop and bought 31 Gulab Jamun. Her sister came home for Bhai Duj and brought 23 more Gulab jamun. How many Gulab jamun did Shankar have now altogether?

Ans: 54

Q2. Hema scored 43 marks out of 50 in a summative assessment. She scored 35 in marks on the final exam. How many marks does he get in all?

Ans: 78

Q3. Soham bought 31 pencils. His brother Ram has 46 pencils. How many pencils do they have altogether?

Ans: 77

The Sum of 4 Tomatoes with 1

Summary

In conclusion, we have learnt that addition is the most basic tool of calculation. This tool is the key to every other form of calculation. It is also a real-life skill that comes into use almost every day. Additional word problems are effective in making students understand the application of addition and clarify the concept by actual problems that they would face in life. These problems have everyday incidents regarding addition of objects such as clothes, food items, or toys. It can also relate to money where they calculate the total payable amount for the goods and items they purchase from a shop.

Addition and subtraction are the basic operations of Mathematics taught to primary school children as a fundamental part of their syllabus. These basic operations also help us in building a great base for higher-level maths. We start learning the process of addition and subtraction using an abacus at the initial level and move towards complicated world problems. As your academic level increases, you will understand the relevance and importance of addition and subtraction word problems in the world of Mathematics. Here is an exclusive blog discussing different methods of solving these questions!

What are Word Problems?

As the name suggests, word problems are comprehensive questions that are designed to give a real-life simulation of mathematical problems. In the case of addition and subtraction word problems, the question generally revolves around some quantity of an object, which increases and decreases as the question progresses. These questions are pretty direct and easy to solve, even without the use of paper and pen. These word problems can be of the following types, Single and Multiple operations. 

Before we move on to the Addition and Subtraction Word Problems,
here is a list of Seating Arrangement Questions for you!

Single Operation Word Problems

This type of word problem deals with a single operation per question. Here are some examples of single-operation addition and subtraction word problems:-

Q1: Mohit has 12 marbles. He buys 5 more. How many does he have now?

For this question, we will follow these simple steps:

Note the number of marbles Mohit has in the beginning(12)

As he buys more marbles, the total number of marbles will increase, so add the number of marbles he purchased(5) to the number of marbles he originally had(12)

The sum of both numbers(12+5) is the total number of marbles Mohit has now, i.e. 17 marbles.

The answer is 17 Marbles

Did you get these Addition and Subtraction word problems?
Great, try out some Algebra Questions as well!

Q2: Joe has 23 oranges. He eats 2 of them. How many does he have now?

For this question, we will follow these steps: 

Note the number of oranges he has in the beginning(23)

As he eats the oranges, the number of oranges he has will reduce. To get the new number of oranges he has now, we will subtract the number of oranges he ate(2) from the total number of oranges(23)

The difference between the two numbers(23-2) is the number of oranges he has now, i.e. 21 oranges

The answer is 21 Oranges

Multiple Operation Word Problems

Multiple operation word problems include more than one operation in a single addition and subtraction word problem. They are the most common type of addition and subtraction word problems as they include both operations in a single question. We just follow a step-wise approach to solve these word problems. Here’s an example-

Q1: Aman owns 24 pens. He decides to buy 5 more. When he comes home he loses 3 pens. How many pens does he have now?

For this question, we take the following steps:

Note the original number of pens Aman has (24)

Add the number of pens he buys(24+5=29)

Subtract the number of pens he loses(29-3=26)

The number of pens he has in the end = 26

The answer is 26 Pens

Try out these BODMAS Questions to improve your Mathematics!

Word Problems for Grade 2

Q1. 114 birds were sitting on a tree. 21 more birds flew up to the tree. How many birds were there altogether in the tree? 

No. of birds initially = 114

Birds flew up to the tree = 21 

Total no. of birds = 114 + 21 = 135

Hence, There were 135 birds in the tree 

Q2. 29 birds were sitting in a tree. Some more fly up to the tree. Then there would be 142 birds in the tree. How many flew up to the tree?

No. of birds sitting in a tree = 29 

Total no. of birds = 142 

Birds flew up to the tree = 142 – 29 = 113 

Hence, 113 birds flew up to the tree

Q3. Beth has 74 crayons. She gives 25 of them away to Jen. How many crayons does beth have left. 

Total no. of crayons of Beth = 74 

Beth gives = 25 

No. of crayons left = 74 – 25 = 49 

Hence, Beth has 49 crayons left 

Q4. Cindy’s mom baked 41 cookies. Paul’s dad baked 38 cookies. Cindy and Paul ate 6 cookies each and then brought them to school for a party. How many cookies did they bring to school altogether? 

Cindy’s baked cookies = 41 

Paul’s dad baked cookies = 38 

Cindy and Paul ate cookies = 6 each

Total no. of cookies baked = 41 + 38 = 79 

Total cookies they bring to school = 79 – 12 = 67 

Hence, 67 total cookies they bring to the school 

Word Problems for Grade 3 

Janina owns a catering service company. She was hired to cater for the mayor’s 50th birthday. 

Q1. For the appetizers, she needs to make 750 mini meat pies. She divided her crew into 3 teams. If the first team made 235, and the second made 275, how many pies should the third team make? 

Total Meat Pies needed = 750 

Meat pies made by first-team = 235

Meat pies made by the second team = 275 

235 + 275 = 510

Then, Meat Pies made by third-team = 750 – 510 = 240 

Hence, meat pies made by third-team = 240

Q2. She needs 280 cups of mushroom soup. If the first team made 90 cups in 60 mins, and the third team made 70 cups in 90 mins, how many cups should the second team prepare in order to meet the required amount of soup?

Total soup cups needed = 280 

Cups made by first-team = 90

Cups made by third-team = 70 

90+70 = 160

Then, cups made by second-team = 280 – 160 = 120

Hence, the second team should prepare 120 soup cups. 

Q3. For the first main dish, they were asked to cook steak. If the third and second-team cooked 240 plates of steak, and the first team cooked 75 plates less than what the second and third-team made, how many steaks did they cook altogether? 

Steak cooked by third and second-team = 240 

Steak cooked by first-team cooked = 75

Steak cooked by second and third-team = 240 – 75 = 165 

Total steaks cooked = 240 + 165 = 405

Hence, they cooked a total of 40 steaks.  

Q4. For the second main course, they made fish fillets for the 320 people at the party. The first team made 189 pieces, the second team made 131 pieces and the third team made 180 pieces, how many pieces were made altogether? 

Pieces made by first-team = 189

Pieces made by second-team = 131

Pieces made by third-team = 180 

Total pieces = 189 + 131 + 180 = 500

Hence, 500 pieces were made altogether.

Word Problems for Grade 4

The table shows the number of people visiting an art museum over 3 months. 

	January	February	March
Child	28	34	56
Adult	59	?	55
Senior	15	22	?
Total	?	139	?

Q1. What is the total number of people that visited the art museum in January? 

In Jan, 

Child = 28

Adult = 59

Senior = 15 

Total = 102

Hence, the total number visited the art museum in January = 102 

Q2. Compared to January, how many more children go to the museum in February? 

Children in January = 28

Children in February = 34 

34-28 = 6

Hence, 6 more children go to the museum in February. 

Q3. How many adults visited the museum in February? 

In February, 

Total People = 139 

Children = 34

Senior = 22

No. of Adults = 139 – 34 – 22 = 83

Hence, 83 adults visited the museum in February. 

Q4. 16 more seniors visited in March. Tell the total no. of seniors who visited the museum in March?

Seniors in Jan and Feb = 15 + 22 = 37

Seniors in March = 37 + 16 = 53 

Also, here we can calculate the total no. of visitors = 55 + 56 + 53 = 164

Hence, March has a total no. of 164 visitors in the three months. 

Common Errors

When attempting to solve addition and subtraction word problems, there are a few frequent errors. Some of these include:

• Calculation mistakes – You must be very careful when adding or subtracting numbers because it is frequently observed that students make errors when working carelessly.
• Right explanation – When working on word problems, it’s crucial to use correct statements in order to properly explain the actions conducted. Mentioning what is given, what needs to be calculated, and the formula envisioned for usage in this regard are among them.
• Understanding of the Problem – One of the most frequent mistakes is when a student is unable to determine if the issue requires an addition or a subtraction solution.

Tips for Solving Addition and Subtraction Word Problems

Here’s a little helpful tip to get through addition and subtraction word problems:

• Words like purchasing, winning, making, buying or getting something from someone are related to addition problems. If you spot such sentences, you should apply addition operations.
• Words like losing, eating, selling, and giving to someone are related to subtraction problems. If you spot such sentences, you should apply subtraction operations.

Now that you know how to solve addition and subtraction word problems,
you must try these simplification questions as well!

Practice Questions

Having explained the types of questions which can come for addition and subtraction word problems, here are some practice questions for you to sharpen your skills-

Q1: if Kishan has 2 balls and Shyam gives him 9 balls, how many balls does Kishan have now?

Q2: If Seeta has 4 apples and Geeta takes away 3 apples from her, how many apples does Seeta have now?

Q3: Matt has 10 watches. He sells 6 of them. How many watches are left with Matt?

Q4: Joseph has 20 cars. If his friends take away 12 of them, then how many cars are left with Joseph?

Q5: Raman has 25 shirts.12 got dirty and 3 are torn. How many good shirts does Raman have?

Q6: Fred has 50 Rupees as his pocket money. He spends 12 on a burger and 17 on a cold drink. How much money does he have now?

Q7: Bruce has 6 gold medals. He wins 3 more at racing. How many gold medals does he have now?

Q8: ABC company has 250 bikes in their store. They sell 153 of them. Then they make 46 new bikes. How many bikes do they have now?

Q9: Varun has 30 fans. Sonam sold him 40 more and Raju bought 22 fans from him. How many fans does Varun have now?

Q10: Radhika has 45 lipsticks. She bought 25 more and gave 17 to Rama. How many lipsticks does Radhika have now?

Q11: A company has 345 workers. They hire 123 more. 12 people leave the company. 23 workers are absent on Monday. How many workers are there in the company on Monday?

Q12: A person takes 14 minutes for a bath. He further takes 20 minutes to get ready and 20 minutes to drive to his office. How much time does it take to reach the office?

FAQs

We hope these questions helped you understand addition and subtraction word problems in a better way. Connect with us at Leverage Edu to browse through more such educational and informative blogs.

Different Types of Addition and Subtraction Situations

In our day to day situations, we come across different problems that relate to addition and subtraction in mathematics. For instance, if you buy a few items from a grocery store, you will need to check the bill raised by the store by adding the costs of the items purchased. Similarly, there might be a situation when you need to find the difference between the original and the discounted price of an item. Such situations make it necessary for students to have a strong grasp of the understanding of the concepts of addition and subtraction. However, this cannot be achieved without enough practice. 

Important Terms and Keywords in addition and subtraction

Below are some important terms and keywords that are associated with addition and subtraction –

how many	how much	leftover	still to go
additional	together	combined	add
both	sum	increase	difference
total	change	more	decrease
fewer	spent	reduce	remaining
less

Let us discuss some such situations which can be useful in the understanding of these terms.

Situations where we use the term “Add To”

Below are some situational problems where we make use of the term “ Add To ”–

1. There are three fishes in a pond. Four more fish are added to the pond.  How many fishes are there in the pond now?
   We have been given – 
   Number of fishes in the pond = 3
   Number of more fishes added to the pond = 4
   For obtaining the total number of fishes in the pond now, we will have to add the number of fishes added to the pond to the number of fishes that were present in the pond earlier. Therefore,
   3 + 4 = 7 this is the total number of fishes in the pond now

1. There are 2 tigers in a forest. Five more tigers are brought from the nearby forest. How many tigers are there in the forest now?
   We have been given – 
   Number of tigers in the forest = 2
   Number of more tigers brought in from nearby forest = 5
   For obtaining the total number of tigers in the forest now, we will have to add the number of tigers brought in from the nearby forest to the number of tigers that were present in the forest earlier. Therefore,
   2 + 5 = 7 this is the total number of tigers in the forest now

Situations where we use the term “Put Together”

We could have used the term put together as well in the above questions. For instance, the question in the example above could be rephrased as –

1. There are three fishes in a pond. Four more fish are added to the pond.  How many fishes are there in the pond put together?

Similarly, the second question could also be rephrased as – 

1. There are 2 tigers in a forest. Five more tigers are brought from the nearby forest. How many tigers are there in the forest put together?

Situations where we use the term “Take From”

Below are some situational problems where we make use of the term “Take From”–

1. On his birthday, James placed 12 burning candles on the cake. He blew nine of them. How many of the candles were burning on the cake now?
   We have been given – 
   Number of candles burning on the cake = 12
   Number of candles blown by James = 9
   For obtaining the total number of candles burning on the cake now, we will have to take the number of candles left burning on the cake from the number of candles that were burning on the cake earlier. Therefore,
   12 – 9 = 3 this is the total number of candles left burning on the cake

1. There were 16 mangoes on a tree. Samuel plucked 9 of them. How many mangoes were left on the tree?
   We have been given – 
   Number of mangoes on a tree = 15
   Number of mangoes plucked by Samuel = 9
   For obtaining the total number of mangoes left on the tree now, we will have to take the number of mangoes plucked by Samuel from the number of mangoes on a tree earlier. Therefore,
   15 – 9 = 6 this is the total number of mangoes left on the tree now

Situations where we use the term “Compare”

Below are some situational problems where we make use of the term “Compare”–

1. Jack scored 25 marks in a test while Peter scored 38 marks in the same test. How many more marks did Peter score as compared to Jack?
   We have been given – 
   Marks scored by Jack in a test= 25
   Marks scored by Peter in the same test = 38
   For obtaining additional marks scored by Peter, we shall compare his marks with that of Jack. We will have, 
     25 + ________ = 38
   = 13

Hence, Peter scored 13 more marks as compared to Jack.

1. Michael has 13 playing cards while Alice has 22 playing cards. How many more playing cards does Alice have as compared to Michael?
   We have been given – 
   Number of cards that Michael has = 13
   Number of cards that Alice has = 22
   For obtaining additional cards that Alice has as compared to Michael, we shall compare the number of cards that Alice has with that of Michael.  We will have, 
     13 + ________ = 22
   = 9

Hence, Alice has 9 more cards as compared to Michael. 

Does more always mean to add?

It is usually assumed that the term “ more “ means to add. However, that is not the case. At times, when we ask questions such as “ How much more is A than B “, this means that we need to find the difference between A and B or in other words subtract them. 

Common Errors 

There are some common mistakes when it comes to solving word problems on addition and subtraction. Some of these are – 

1. Calculation errors – You need to be extremely careful when adding or subtracting numbers as often it is seen that students make mistakes when working in a careless manner.
2. Proper explanation – When it comes to working on word problems, it is important to mention proper statements so as to explain the steps undertaken correctly. These include mentioning what is given, what needs to be calculated and the formula intended to be used for this purpose.
3. Understanding of the Problem – One of the most common errors is when a student fails to identify whether the problem needs a solution through addition or subtraction.

Addition and Subtraction Word problems Involving Money

We know that money is available in different forms at different places. For buying things we use coins and notes which are issued by the respective governments of the country we live in. These are called currency. Let us discuss some money-related situations where we make use of addition or subtraction 

Example

Suppose, Gracia purchased two items, one at the cost of £ 2.05 while another at the cost of £ 4.20. What is the total cost she needs to pay altogether?

Solution

We have been that given that Gracia purchased two items, one at the cost of £ 2.05 while another at the cost of £ 4.20. We need to find out how much Gracia needs to pay altogether. There are two ways of doing it. Either we add the money directly or first convert the given amounts in pence. We know that

£ 1 = 100 p

Therefore, we have,

£ 2.05 = 2.05 x 100 = 205 p

£ 4.20 = 4.20 x 100 = 420 p

Now we have two values 205 p and 420 p. we can add these values, in the same manner, we add two whole numbers. We will get,

205 p + 420 p = 625 p

Now we have obtained our result in pence. To convert it back into pounds, we will have to divide the result by 100. We will now have,

625 p = £ $frac{625}{100}$  = £ 6.25

Hence, we can say that the sum of £ 2.05 and £ 4.20 = £ 6.25

A direct way of solving this problem would have been to add the two amounts directly. We would then have,

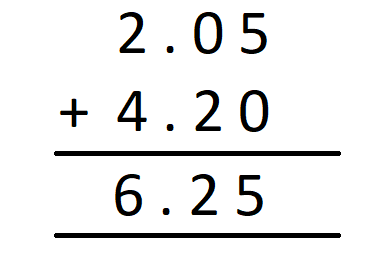
It is important to note here that irrespective of the method we use, we shall get the same result. 

Let us consider another example.

Example

Maria purchased a syrup for £ 36.00, a cookies box for  £ 29.50 and a hair oil bottle for  £ 32.50. If she gave the shopkeeper £ 100, how much money did the shopkeeper return as balance?

Solution

We have been given that Maria purchased a syrup for £ 36.00, a cookies box for £ 29.50 and a hair oil bottle for £ 32.50. She gave the shopkeeper £ 100. We are required to find the money returned by the shopkeeper as a balance. In order to do so, first, let us summarise the items purchased by Maria.

Cost of syrup purchased by Maria =  £ 36.00

Cost of cookies box purchased by Maria = £ 29.50

Cost of hair oil bottle purchased by Maria = £ 32.50

Total shopping by Maria = £ 36.00 + £ 29.50 + £ 32.50 = £ 98

Now, Maria gave £ 100 to the shopkeeper. Therefore

Change returned by the shopkeeper = £ 100 – £ 98 = £ 2.00 Change returned by the shopkeeper = £ 100 – £ 98 = £ 2.00

Hence, Maria got £ 2.00 from the shopkeeper as a change.

Addition and Subtraction Word problems Involving Decimals

We perform addition and subtraction of decimals in many situations in our day-to-day lives. For example, you may need to add up your grocery bill before paying at the counter. Have you ever noticed that most of the prices are in decimals? Let us discuss through an example where we use addition or subtraction of decimals in our daily life.

Example

Sophie had £ 305.80 in her bank account. She deposited £ 250.25 more and then withdrew £ 317.50 from her account. What is the balance now in her account?

Solution

Let us first define what is given and what needs to be calculated. We have been given that Sophie had £ 305.80 in her bank account. She deposited £ 250.25 more and then withdrew £ 317.50 from her account. We are required to find the balance in her account now. Therefore,

Initial amount in Sophie’s account = £ 305.80 …………………. ( 1 ) 

The amount deposited by Sophie = £ 250.25 …………………. ( 2 ) 

Both the decimal numbers are like terms; hence we can go ahead with the calculations.

Total amount in Sophie’s account = ( 1 ) + ( 2 )  

= £ 305.80 + £ 250.25 

= £ 556.05 …………………………… ( 3 ) 

Now, from this total amount, Sophie had withdrawn £ 317.50

Therefore, money left in her account = ( 3 ) – £ 317.50

Again, both the decimal numbers are like terms; hence we can go ahead with the calculations.

= £ 556.05 – £ 317.50

= £ 238.55

Hence, money left in Sophie’s account = £ 238.55

Let us take another example.

Example 

Sam, Peter and Henry bought 8.5 litres, 7.25 litres and 9.4 litres of milk respectively from a milk booth. How much milk did they buy in all? If there were 30 litres of milk in both, find the quantity of milk left.

Solution

Let us first define what is given and what needs to be calculated. We have been given that Sam, Peter and Henry bought 8.5 litres, 7.25 litres and 9.4 litres milk respectively from a milk booth. We need to find out – 

a) How much milk did they buy in all?

b) If there were 30 litres of milk in both, find the quantity of milk left.

Let us find the answers to the above problems one by one.

Amount of milk bought by Sam = 8.5 litres

Amount of milk bought by Peter = 7.25 litres

Amount of milk bought by Henry = 9.4 litres

The total amount of milk bought by them = 8.5 litres + 7.25 litres + 9.4 litres

Note here that the given decimals are not like terms. Hence, we will first need them to be converted into like terms. Therefore, we now have,

Amount of milk bought by Sam = 8.5 litres = 8.50 litres

Amount of milk bought by Peter = 7.25 litres = 7.25 litres

Amount of milk bought by Henry = 9.4 litres = 9.40 litres

Now that the terms are like terms, we can go ahead with the calculations.

Total milk bought by Sam, Peter and Henry = 8.50 litres + 7.25 litres + 9.40 litres = 25.15 litres

Hence, the total milk bought by Sam, Peter and Henry =25.15 litres

Now, let us solve the second part of the question. We have been given that there were 30 litres of milk in the booth.  How much milk is left after Sam, Peter and Henry bought the milk?

Therefore, 

Total milk in booth = 30 litres

Milk bought by Sam, Peter and Henry = 25.15 litres

Again, we can see here that the given decimals are not like terms. Hence, we will first need them to be converted into like terms. Therefore, we now have,

Total milk in booth = 30 litres = 30.00 litres

Milk bought by Sam, Peter and Henry = 25.15 litres

Now that the terms are like terms, we can go ahead with the calculations.

Milk left in the booth = 30.00 litres – 25.15 litres

= 4.85 litres

Hence, after Sam, Peter and Henry, 4.85 litres of milk was left in the booth.

Addition and Subtraction Word problems Involving Fractions

It is not just money or decimals, we find everyday situations where we need to solve word problems involving addition and subtraction. We are required to do so in the case of fractions as well. Let us look at some situations where we encounter addition and subtraction word problems involving fractions – 

Example 

A recipe needs 3/7 teaspoon black pepper and 1/4 teaspoon red pepper. How much more black pepper does the recipe need?

Solution

We have been given that a recipe needs 3/7 teaspoon black pepper and 1/4 teaspoon red pepper. We need to find out how much more black pepper is required in the recipe as compared to red pepper.

First of all, we will summarise the fractions given to us. We have,

Fraction of black pepper needed in the recipe = $frac{3}{7}$

Fraction of red pepper needed in the recipe = $frac{1}{4}$

In order to find out how much more black pepper is required in the recipe as compared to red pepper, we will need to find the difference between the black pepper and the red pepper used.

Therefore, we need to find out the value of $frac{3}{7} – frac{1}{4}$

The denominators of the above fractions are different; therefore, we will find their L.C.M first.

L.C.M of 7 and 4 = 28

Now, we will convert the given fractions into equivalent fractions with denominator 28.

$frac{3}{7} = frac{3 x 4}{7 x 4} = frac{12}{28}$

$frac{1}{4} = frac{1 x 7}{4 x 7} = frac{7}{28}$

Now, that the denominator of both the fractions is the same we will find the difference in their numerators. We have,

$frac{3}{7} – frac{1}{4} = frac{12}{28} – frac{7}{28} = frac{12- 7}{28} = frac{5}{28}$

Hence, the amount of more black pepper required in the recipe as compared to red pepper = $frac{5}{28}$

Recommended Worksheets

Fact Families for Addition and Subtraction (Christmas Themed) Math Worksheets
Problem Solving – Addition and Subtraction (World Teachers’ Day Themed) Math Worksheets
Solving Word Problems involving Addition and Subtraction of numbers within 120 1st Grade Math Worksheets

Word problems involving addition and subtraction are discussed here step by step.

There are no magic rules to make problem solving easy, but a systematic approach can help to the problems easily. 

For example:

sum; total; in all; all together.

(b) Reducing : Find out how much has been given away or how much remains.

(c) Comparison : More than / less than.

Tickets sold in the second week = 108

Tickets sold in the third week = 210

Total number of tickets sold = 75 + 108 + 210 = 393

Answer: 393 tickets were sold in all.

Step 1: Money spent for petrol on Thursday

450 + 575 = $1025

Examples on word problems on addition and subtraction:

Therefore, sum =11,691

2. What is the difference of 3867 and 1298?

Therefore, difference = 2569

Difference of 6956 and 4358

Therefore, 2598 is the answer.

4. Find the number, which is

(i) 1240 greater than 3267.

Therefore, the number

= 5292 – 1353 or

= 3939

= 10750 + 12650 = 23400

Therefore, number of workers coming in the third shift = 35675 — 23400 = 12275

Related Concept

● Addition

● Word
Problems on Addition

● Subtraction

● Check
for Subtraction and Addition

● Word
Problems Involving Addition and Subtraction

● Estimating
Sums and Differences

● Find the
Missing Digits

● Multiplication

● Multiply
a Number by a 2-Digit Number

● Multiplication
of a Number by a 3-Digit Number

● Multiply a Number

● Estimating Products

● Word
Problems on Multiplication

● Multiplication
and Division

● Terms Used in
Division

● Division
of Two-Digit by a One-Digit Numbers

● Division
of Four-Digit by a One-Digit Numbers

● Division
by 10 and 100 and 1000

● Dividing Numbers

● Estimating
the Quotient

● Division
by Two-Digit Numbers

● Word
Problems on Division

HOME PAGE

Addition and subtraction word problems are commonly taught in Year 2 (Key Stage 1 in the UK) or second grade (in the USA).

The strategy to solve word problems is to firstly, write out the numbers involved and secondly, to decide which operation to use by reading the keywords in the question.

To solve addition and subtraction word problems, we try to read the question and look for keywords. The keyword list below will help to identify whether we have an addition or subtraction word problem.

Some common addition keywords are:

• Add
• Plus
• More
• Total
• Increase
• Together / Altogether
• Combined
• Sum
• Grow

If we see these words, we likely have an addition word problem.

Some common subtraction keywords are:

• Subtract
• Minus
• Take away
• Less / Fewer than
• Difference
• Decrease
• How many are left / remain?
• Change – in money questions
• Words ending in ‘er’, such as shorter, longer, faster.

Here is our first example of a word problem.

William has 20 counters and is given 7 more.

How many does he have in total?

We can see that we have the two addition keywords which are: ‘more‘ and ‘total‘.

When teaching word problems, it is useful to first write out the numbers that are in the text of the question.

We have 20 and 7.

The words ‘more‘ and ‘total‘ tell us that this is an addition word problem. We start with 20 counters and add 7 more.

Once we know that we have an addition word problem, then we can add the numbers.

20 + 7 = 27

William has 27 counters in total.

Here is another word problem example.

Phoebe has 12 cm of ribbon and Jack has 23 cm.

How much do they have altogether?

Our strategy is to first write out the numbers involved in the question.

We write down 12 and then 23. We can write the numbers above each other and line up the digits in each number.

There is only one keyword in this question which is altogether.

This in an addition keyword which tells us that we want to combine the two amounts to make a total.

We want to add the numbers 12 and 23.

It is common for children to write down the units involved in the question at this stage. However, it is easiest to just write down the numbers themselves and then to put the units in at the end of the question as part of checking the working out.

Adding the units column, 2 + 3 = 5.

Adding the digits in the tens column, 1 + 2 = 3.

Therefore 12 + 23 = 35 and so, we have 35 cm of ribbon in total.

We are measuring the length of ribbon in cm and so, we write ‘cm’ at the end of our answer.

Here is another word problem example.

I buy 2 sweets that cost 43 pence each.

How much do they cost in total?

Each sweet costs 43 pence and there are two of them.

We write down 43 twice in this question.

The keyword ‘total‘ tells us that this is an addition word problem.

We will add the two 43 amounts by writing their digits directly above each other without writing ‘pence’ at the end.

Adding the units, 3 + 3 = 6.

Adding the tens, 4 + 4 = 8.

The two sweets cost 86 pence in total.

We can write the pence or ‘p’ on the final answer now that the calculation has been done.

In this worded question, we have only one number in the text itself. There is only one ’43’ written.

It can help to draw a diagram when teaching word problems to children to help picture the situation.

Here is another word problem involving money.

Matthew has 35 pence.

He spends 13 pence.

How much does he have left?

We write down the numbers involved, which are 35 and 13.

In this word problem, the keyword is left.

Finding how much is left is a keyword for a subtraction word problem.

This means that we subtract the smaller number from the larger number.

To subtract 13 from 35, we write the larger number above the smaller number and line up the digits.

Subtracting the units column, 5 – 3 = 2.

Subtracting the tens column, 3 – 1 = 2.

35 – 13 = 22

This was a problem involving finding change with money.

Spending money and then receiving change is also very likely to indicate that a word problem is a subtraction one.

In this next worded problem, Adam has 59 grams of chocolate.

He eats 49 grams.

How much does he have left?

The first step of the word problem strategy is to write out both of the numbers involved in the question.

We have 59 and 49.

The second step is to identify keywords. The word left is a subtraction keyword.

We want to see how much is left after 49 grams have been subtracted.

We write the subtraction with the larger number above the smaller number.

Subtracting the units column digits, 9 – 9 = 0.

Subtracting the tens column digits, 5 – 4 = 1.

59 – 49 = 10.

There are 10 grams of chocolate remaining.

Here is another word problem example.

I have a candle that is 38 cm long.

After I light it, 11 cm melts away.

How long is the candle now?

This word problem is trickier in that there are not any direct keywords in the question.

However the phrase ‘melts away tells us that we are removing, or subtracting.

Again, when teaching word problems, drawing a diagram is a useful technique.

If no diagram or picture is given, it helps to draw the situation at the start and also the situation at the end.

Subtracting the digits in the units column, 8 – 1 = 7.

Subtracting the digits in the tens column, 3 – 1 = 2.

38 – 11 = 27

27 cm of the candle remains.

In this example, the candle reduced in size because we were removing length as it melted.

The candle has become shorter. Shorter is a word which ends in ‘er’, which can also indicate that we have a subtraction word problem.

‘er’ words often look for a difference between two values and finding a difference is a subtraction.

Again, it can help to draw the situation with a diagram to help understand what type of word problem we have.

Word Problems: Word Problems are an important aspect of the primary curriculum because they require students to apply their understanding of various topics to “real-life” circumstances. They also assist students in becoming acquainted with mathematical terminology like more, fewer, subtract, difference, altogether, equal, share, multiply, reduced, etc.

A word problem consists of a description of a real-life scenario where a mathematical calculation is required to solve a problem. Students need to learn how to solve word problems as it enables them to apply mathematical concepts to various real-life scenarios. This means that to understand the word problem, students must be familiar with the terminology linked with the mathematical symbols they are used to. Let us learn in detail about Word Problems in this article.

What is a Word Problem?

In real life, mathematical problems do not usually present themselves as (2+3) or (6-4.) Instead, they are a bit more complex than we think. Authors of mathematical curriculum sometimes implement word problems to help students understand how they are related to the real world.

Learn 10th CBSE Exam Concepts

Word problems often show mathematics happening more naturally in the real world. Word problems can vary from simple to complex.

Examples:
Here are a few examples to get you some ideas:
1. Keerthi had (5) apples. Her mother gave her (7) more apples. Now, how many apples does Keerthi have altogether?
2. There were (18) pencils and (9) pens. How many more pencils than pens are there?
3. Spurti has one dozen eggs. Her friends ate (4) for breakfast. Now, how many eggs are left with Spurti?
4. There are (18) apples. Swetha, Priya, and Rachana want to eat them in equal share. How many apples will each of the friends get?

As you can notice, word problems involve addition, subtraction, multiplication, division, or even multiple operations.

Word Problems on Quadratic Equation

Handling Maths Word Problems in General

Steps for Solving a Word Problem:

To work out any word problem, follow the steps given below:

1. Read the Problem: First, read through the problem once.

2. Highlight Facts: Then, read through the problem again and underline or highlight important facts such as numbers or words that indicate an operation.

3. Draw a Picture: Drawing a picture sometimes help visualise the problem more clearly. It can also help understand clearly the algebraic operations that need to be carried out.

4. Determine the Operation(s): Next, determine the operation or operations that need to be performed. Is it addition, subtraction, multiplication, division? What needs to be done? Drawing the picture should be a big help in figuring this out. However, look for clues in words such as:
(i) Addition: add, total, brought, plus, altogether, and, combine, more, in all
(ii) Subtraction: subtract, fewer, take away, than, left
(iii) Multiplication: total, times, triple, twice, in all
(iv) Division: each, per, out of, equal pieces, split, average

Practice 10th CBSE Exam Questions

5. Make a Math Sentence: Next, translate the word problem and drawings into a math or number sentence. This means write a sentence such as (18+3=)

6. Solve the Problem: Then, solve the number sentence and find the solution—for example, (3+8=11.)

7. Check Your Answer: Finally, check the result obtained to ensure the correct answer.

With the above (7) steps, solving word problems in mathematics becomes easy.

Addition Word Problems

Addition word problems arise in situations where there is an increase of something or gain as a result of combining one or more numbers. Think of addition as combining parts to form a whole.
Example: Teena has (6) chocolates, her brother gives her (4) chocolates. How many chocolates in total does Teena have?
To find the total chocolates, we add the number of chocolates Teena has and the number of chocolates her brother gave her.

Thus, Teena has (10) chocolates now.

Attempt 10th CBSE Exam Mock Tests

Subtraction Word Problems

Subtraction word problems arise in situations where there is a decrease of something or loss as a result of deducting a number from another.
Example: Arya plucked (10) cherries from the tree, and her friend asked her to give (4) cherries to her. How many cherries are left with Arya?
Here we subtract the number of cherries given to Arya’s friend from the total cherries plucked by Arya.

Thus, the number of cherries left with Arya is (6.)

Multiplication Word Problems

Multiplication word problems are helpful for students to develop their multiplication knowledge by applying it to real-life situations. In this kind of multiplication word problem, one quantity is compared with another quantity, larger or smaller. Work out the multiplication problem using the clues provided.
Example: Ajay has two bunch of tomatoes. Each bunch has (4) tomatoes. What is the total number of tomatoes does Ajay have?
Here, we multiply the number of the bunch by the number of tomatoes in each bunch

Thus, Ajay has (8) tomatoes in total.

Division Word Problems

Division word problems are more confusing for students to understand. The words generally used in division word problems are “shared among” or “given to each” or similar phrases that imply total quantity to be split evenly into groups. We use division or multiplication when the problem involves equal parts of a whole. 

Example: He divides (12) apples evenly among (4) friends. How many apples did John give to each of his friends?
To find how many apples John gave to each of his friends, we divide the total number of apples by the number of friends.

Hence, each of John’s friends gets (3) apples.

Fraction Word Problems

Fraction word problems look more complex, but in reality, fraction word problems are just as easy as those involving whole numbers. Here, one extra step of simplification may be needed in some cases. Students should know the operations with fractions to solve word problems on fractions.

Example: Swaroop had half an apple, and his mother gave him another quarter of an apple. What portion of apple does Swaroop have?
The given example is the addition word problem that involves fractions.
Here, we add the portion of apple Swaroop initially had with the portion his mother gives. i.e., (frac{1}{2} + frac{1}{4} = frac{{2 + 1}}{4} = frac{3}{4}.)

Thus, Swaroop has (frac{3}{4})th of apple.

Solved Examples

Q.1. The male population of a village is (76138,) and the female population is (13776.) What is the total population of the village?
Ans: Male population (=76138)
Female population (=13776)
Total population of the village (=) male population (+) female population (=76138+13776 )
(=89914 )

Thus, the total population of the village is (89914.)

Q.2. Likith had collected (208) coins. He lost of (52) coins. How many coins are left with Likith?
Ans: Total coins Likith collected (=208)
The number of coins lost (=52)
Number of coins left (=208 – 52=156)

Thus, the number of coins left is (156.)

Q.3. The cost of one shirt is (₹206.) A retailer wants to buy (68) such shirts. How much should he pay?
Ans: Given that the cost of one shirt (=₹206)
Number of shirts a retailer wants to buy (=68)
Total amount to be paid (=₹206×68)

Therefore, the total amount to be paid is (₹14008.)

Q.4 If (4472,{rm{kg}}) of rice is packed in (52) bags, how much rice will each bag contain?
Ans: Since (52) bags contain rice of (4472,{rm{kg}})
Therefore, (1) bag contains rice ( = left( {frac{{4472}}{{52}}} right){rm{kg}})
( = 86,{rm{kg}})

Thus, each bag of rice contains ( = 86,{rm{kg}}.)

Q.5. One-half of the students in a school are boys, (frac{4}{5}) of these boys are studying in lower classes. What fraction of boys are studying in lower classes?
Ans: Fraction of boys studying in school ( = frac{1}{2})
Fraction of boys studying in lower classes ( = frac{4}{5}) of (frac{1}{2})
( = frac{4}{5} times frac{1}{2})
( = frac{2}{5})
Therefore, (frac{2}{5}) of boys studying in lower classes.

Q.6. Celena planned to interview some candidates for a position in her office. If she scheduled half an hour to meet each of them, how much time did she schedule for all (6) candidates?
Ans: Time spent to meet each candidate ( = frac{1}{2}) hour
Number of applicants (=6)
Time scheduled for all (6) candidates ( = frac{1}{2} times 6 = 3) hours
Thus, Celena scheduled (3) hours for the interview.

Summary

In this article, we studied the meaning and steps to solve word problems. Then we have discussed in detail taking an example of how to do the addition word problem, subtraction, multiplication, division and fraction word problem. 

We have solved word problem examples for students to understand the concept clearly.

Learn the Concepts on Unitary Method

Frequently Asked Questions on Word Problems

We have provided some frequently asked questions on Word Problems here:

Q.1. What are word problems in maths?
Ans: A word problem is a few sentences describing a real-life scenario where a problem needs to be solved by a mathematical calculation.

Q.2. What are numberless word problems? 
Ans: Numberless word problems are designed to provide scaffolding that allows students to understand the underlying structure of word problems.

Q.3. What is addition word problems?
Ans: Addition word problems appear when there is a gain or an increase of something due to combining one or more numbers. Think of addition as combining parts to form a whole.

Q.4. What are the steps in solving word problems?
Ans: Solving word problem requires the following steps: 
1. Read the problem
2. Highlight facts 
3. Draw a picture
4. Determine the operation/s.
(i) Addition
(ii) Subtraction
(iii) Multiplication
(iv) Division
5. Make a mathematical sentence
6. Solve the problem
7. Verify your answer

Q.5. What is an example of a word problem?
Ans: The typical examples of word problems in algebra are distance problems, age problems, percentage problems, work problems, mixtures problems and number problems.

Now you are provided with all the necessary information on Maths word problems and we hope this detailed article is helpful to you. If you have any queries regarding this article, please ping us through the comment section below and we will get back to you as soon as possible.

Related Pages
Addition & Subtraction Word
2-Step Word Problems and Bar Models
More Word Problems
More Singapore Math Lessons

Example:
There are 1030 books in the library. We bought 67 more books for the library. How many books are
there in the library now?

Solution:

1030 + 67 = 1097

There are 1097 books in the library now.

Example:
1085 girls and 531 boys took part in an art competition. How many students took part in the
competition altogether?

Solution:

1085 + 531 = 1616
1616 students took part in the competition altogether.

Example:
After giving $1085 to his wife, Simon had $746 left. How money had he at first?

Solution:

1085 + 746 = 1831

Simon had $1831 at first.

Example:
Margret sold 1392 meatballs on Friday. She sold 1940 more meatballs on Saturday than on Friday.
How many meatballs did she sell on Saturday?

Solution:
1392 + 1940 = 3332
She sold 3332 meatballs on Saturday.

A visual way to solve world problems using bar modeling
This type of word problem uses the part-whole model. Because the whole is missing, this is an addition problem.

Example:
Mr. Gray sold 64 drinks in the morning. Mr. Frank sold 25 drinks at night. How many drinks did they
sell altogether?

• Show Video Lesson

How to solve addition problem using part-whole model?

Example:
Maya had some stamps. She gave 7 stamps to her younger brothers. Maya then had 14 stamps. How many
stamps did Maya have at first?

• Show Video Lesson

Examples of 4th Grade word problems and bar models

Example:
Dad bought two hammers. One cost $18 and the other costs $28 more. What was his total bill?

• Show Video Lesson

Model Drawing — addition word problems

Example:
A girl jumps 42cm for her first jump in a high jump competition. Her second jump is 46cm.
How high did she jump in total?

• Show Video Lesson

Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.

We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.

My students had been struggling with how to solve addition and subtraction word problems for what seemed like forever. They could underline the question and they could find the numbers. Most of the time, my students just added the two numbers together without making sense of the problem.

Ugh.

Can you relate?

Below are five math problem-solving strategies to use when teaching word problems on addition and subtraction using any resource.

So, how do I teach word problems? It’s quite complex, but so much fun, once you get into it.

How to teach addition and subtraction word problems

The main components of teaching addition and subtraction word problems include:

1. Teaching the Relationship of the Numbers – As a teacher, know the problem type and help students solve for the action in the problem
2. Differentiate the Numbers – Give students just the right numbers so that they can read the problem without getting bogged down with the computation
3. Use Academic Vocabulary – And be consistent in what you use.
4. Stop Searching for the “Answer” – it’s not about the answer; it’s about the process
5. Differentiate between the Models and the Strategies – one has to do with the relationship between the numbers and the other has to do with how students “solve” or compute the problem.

I am a big proponent of NOT teaching keyword lists. It just doesn’t work consistently across all problems. It’s a shortcut that leads to breakdowns in mathematical thinking. Nor should you just give students word problem worksheets and have them look for word problem keywords. I talk more in-depth about why it doesn’t work in The Problem with Using Keywords to Solve Word Problems.

Teach the Relationship of the Numbers in Math Word Problems

One way to help your students solve word problems is to teach them the relationship of the numbers. In other words, help them understand that the numbers in the problem are related to each other in some way.

I teach word problems by removing the numbers. Sounds strange right?

Removing the distraction of the numbers helps students focus on the situation of the problem and understand the action or relationship of the numbers. It also keeps students from solving the problem before we talk about the relationship of the numbers.

When I teach word problems, I give students problems with blank spaces and no numbers. We first talk about the action in the problem. We identify whether something is being added to or taken from something else. That becomes our equation. We identify what we have to solve and set up the equation with blank spaces and a square for the unknown number

___ + ___ = unknown

Do you want a free sample of the word problems I use in my classroom?  Click the link or the image below. FREE Sample of Word Problems by Problem Type

Differentiate the numbers in the Word Problems

Only after we have discussed the problem do I give students numbers. I differentiate numbers based on student needs. At the beginning of the year, we all do the same numbers, so that I can make sure students understand the process.

After students are familiar with the process, I start to give different students different numbers, based on their level of mathematical thinking.

I also change numbers throughout the year, from one-digit to two-digit numbers. The beauty of the blank spaces is that I can put any numbers I want into the problem, to practice the strategies we have been working on in class.

At some point, we do create a list of words, but not a keyword list. We create a list of actions or verbs and determine whether those actions are joining or separating something. How many can you think of?

Here are a few ideas:

Join: put, got, picked up, bought, made
Separate: ate, lost, put down, dropped, used

Don’t be afraid to use academic vocabulary when teaching word problems

I teach my students to identify the start of the problem, the change in the problem, and the result of the problem. I teach them to look for the unknown.

These are all words we use when solving problems and we learn the structure of a word problem through the vocabulary and relationship of the numbers.

In fact, using the same vocabulary across problem types helps students see the relationship of the numbers at a deeper level.

Take these examples, can you identify the start, change and result in each problem? Hint: Look at the code used for the problem type in the lower right corner.

For compare problems, we use the terms, larger, smaller, more and less. Try out these problems and see if you can identify the components of the word problems.

Stop searching for “the answer” when solving word problems

This is the most difficult misconception to break.

Students are not solving a word problem to find “the answer”. Although the answer helps me, the teacher, understand whether or not the student understood the relationship of the numbers, I want students to be able to explain their process and understand the depth of word problems.

Okay, they’re first and second-graders. I know.

My students can still explain, after instruction, that they started with one number. The problem resulted in other another number. Students then know that they are searching for the change between those two numbers.

It’s all about the relationship.

Differentiate between the models and the strategies

A couple of years ago, I came across this article about the need to help students develop adequate models to understand the relationship of the numbers within the problem.

A light bulb went off in my head. I needed to make a distinction between the models students use to understand the relationship of the numbers in the problem and the strategies to solve the computation in the problem. Models and strategies work in tandem but are very different.

Models are the visual ways problems are represented. Strategies are the ways a student solves a problem, putting together and taking apart the numbers.

The most important thing about models is to move away from them. I know that sounds odd.

You spend so long teaching students how to use models and then you don’t want them to use a model. Well, actually, you want students to move toward efficiency.

Younger students will act out problems, draw out problems with representations, and draw out problems with circles or lines. Move students toward efficiency. As the numbers get larger, the model needs to represent the relationship of the numbers

This is a prime example of moving from an inverted-v model to a bar model.

Here is a student moving from drawing circles to using an inverted-v.

Students should be solidly using one model before transitioning to another.  They may even use two at the same time while they work out the similarities between the models.

Students should also be able to create their own models. You’ll see how I sometimes gave students copies of the model that they could glue into their notebooks and sometimes students drew their own model.  They need to be responsible for choosing what works best for them. Start your instruction with specific models and then allow students to choose one to use. Always move students toward more efficient models.

The same goes for strategies for computation. Teach the strategies first through the use of math fact practice, before applying it to word problems so that students understand the strategies and can quickly choose one to use. When teaching, focus on one or two strategies. Once students have some fluency in a few strategies, have them choose strategies that work for different problems.

Which numbers do you put in the blank spaces?

Be purposeful in the numbers that you choose for your word problems. Different number sets will lend themselves to different strategies and different models. Use number sets that students have already practiced computationally.

If you’ve been taught to make 10, use numbers that make 10. If you’re working on addition without regrouping, use those number sets. The more connections you can make between the computation and the problem-solving the better.

The examples above are mainly for join and separate problems. It’s no wonder our students have so much difficulty with compare problems since we don’t teach them to the same degree as join and separate problems.

Our students need even more practice with those types of problems because the relationship of the numbers is more abstract. I’m going to leave that for another blog post, though.

Do you want a FREE sample of the resource that I use to teach Addition & Subtraction Word Problems by Problem Type?  Click this link or the image below.

How to Purchase the Addition & Subtraction Word Problems

The full resource is also available in my store for purchase and on Teachers Pay Teachers.

More Ideas for Teaching Word Problems