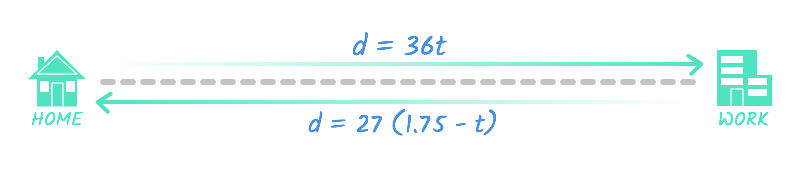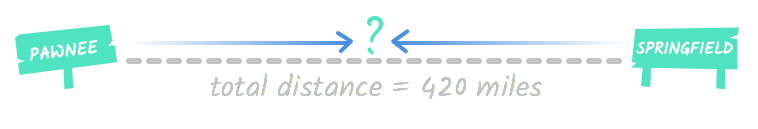Time it takes word problems

Microsoft Word is one of the popular text processing applications available. Though there are many alternate apps, Word is an ultimate document app due to universal use. However, slow performance of Microsoft Word is quite common on many computers due to various reasons. This may result in reduced productivity for business professionals and normal computer users. In this article let us explain how to fix slow Word when opening, typing and saving documents to speedup productivity.

Related: How to fix slow Microsoft Excel and speedup your productivity.

Fix Slow Microsoft Office Problems

Here are the fixes to solve the performance problem with Microsoft Word.

1. Deleting default template file
2. Disable graphics acceleration in Word settings
3. Use registry editor to disable graphics acceleration
4. Install updates
5. Update your OS
6. Reinstall Microsoft Office
7. Delete temporary files
8. Optimize the document
9. Open Word in safe mode
10. Check locked files
11. Disable add-ins

Method #1: Delete Normal.dot File

Microsoft Word uses template files to create documents. Normal.dot is the global template file that Word uses for creating a blank document file. This essentially means, the template file is always in use when Word is in use. Word also stores all style related settings, such as font size and font type in this template. So, problematic or corrupted ‘Normal.dot’ template can cause slow performance when opening or closing your documents.

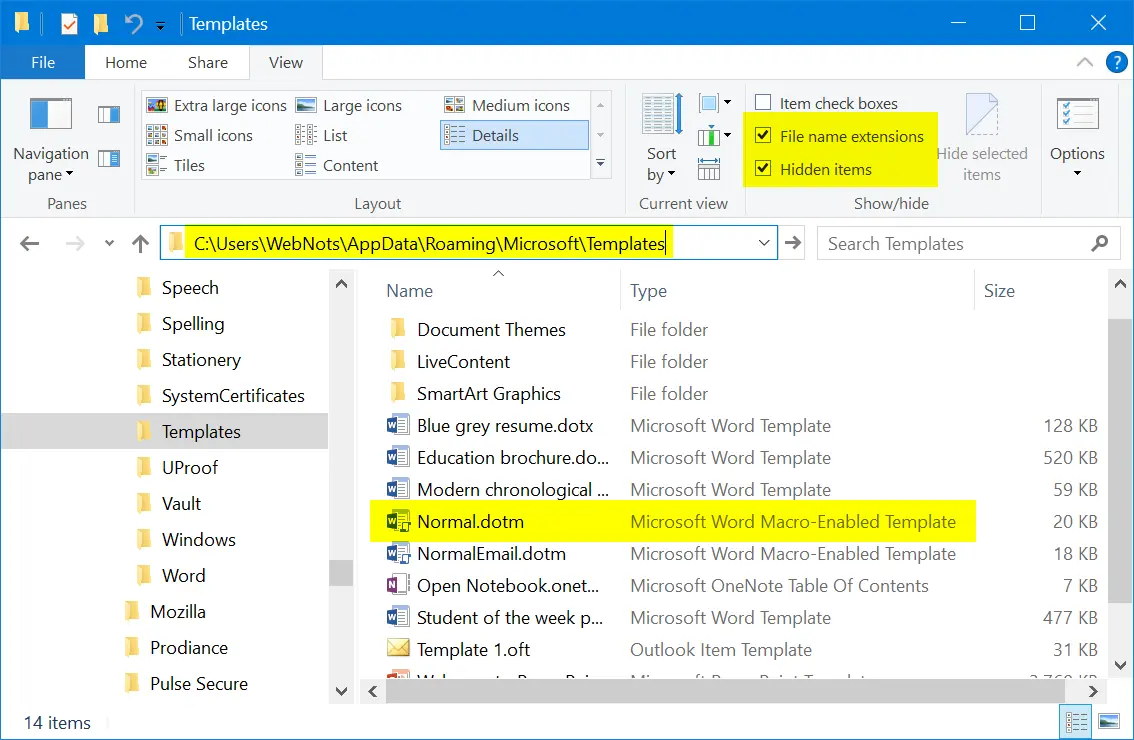
An effective solution is to delete the Nomal.dot or Normal.dotm template file. You don’t need to worry about deleting this file as Word will recreate a fresh Normal.dot file when you open the application. However, it is a bit tricky task, since Normal.dot or Normal.dotm file is a hidden file. Therefore, you need to first enable the option in Windows to show hidden files. Then save all your open documents and close Microsoft Word.

The file location may be different depending upon your Windows version. Generally you can find the Normal.dot (Word 2007) or Normal.dotm (Word 2007/10) or Normal.dotx (Word 2013 and above) file in the following location:

C:UsersUsernameappdataMicrosoftTemplates

In Windows 10, you can look for the file in the below path:

C:UsersYour User NameAppDataRoamingMicrosoftTemplates

If you use Office on Mac, go to Finder and press “Command + Shift + G” to open “Go to folder” box. Type the following location and press enter key.

~/Library/Application Support/Microsoft/Office/User Templates/

After locating the template file in your PC or Mac, simply delete it. Now, open Microsoft Word to create a new Normal.dot/dotm/dotx file. Check whether this helps to solve the slow processing issues in Microsoft Word.

Related: How to quickly insert dividers in Microsoft Word?

Method #2: Disable Hardware Graphics Acceleration

In some cases, when you type on a Word document, it will lag for few seconds or longer from the moment you press the key. This will badly affect your productivity by causing delay and diverting your concentration when doing important work. Newer Office versions use hardware graphics acceleration to speed up the performance. However, slow computers often have limited hardware resources, which make Word less responsive. Follow these steps to disable hardware acceleration in Word:

• Open Microsoft Word and create a blank document.
• Click “File” menu and choose “Options” in the left pane to go to backstage view.
• On the “Word Options” screen, choose “Advanced” in the left pane.
• Look for “Disable hardware graphics acceleration” option at the right pane under the “Display” section and enable it.
• Click the OK button to apply your changes.

Restart Microsoft Word and verify whether the problem has been resolved.

Method #3: Disable Hardware Acceleration in Registry Editor

If Microsoft Word still performs slowly, it may be a good idea to perform changes in the registry. Note that modifying registry is not recommended and you may not have access in the administered computers. If you have access to registry editor then backup your data and follow the below steps:

• Press “Win + R” keys to open Run prompt. Type the command “regedit” and hit enter. Alternatively, use Windows search box and type in “regedit” to open Registry Editor.
• Navigate to the entry HKEY_CURRENT_USERSoftwareMicrosoftOffice16.0common.
• Note that 16.0 is the version of Microsoft Office 2016, your version could be different.
• Right click on the right pane select “New > Key” to create a new key under the “common” folder.

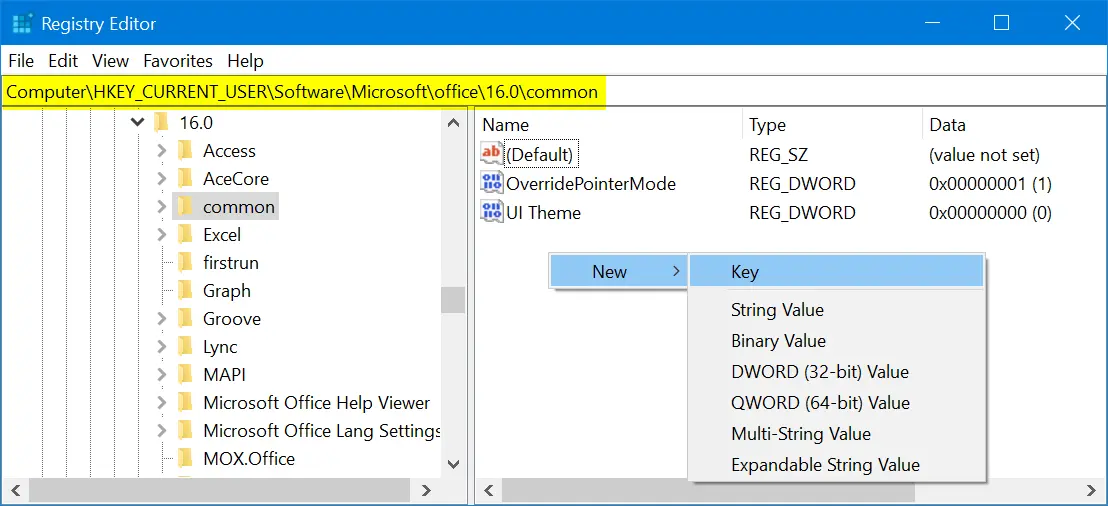
• Windows will create a key with the name as “New Key #1” with an option to edit. Type in “Graphics” for the name of the key.
• Click the newly created “Graphics” key and right click on the right pane. Select “New > DWORD (32-bit) Value” option.

• Type in “DisableHardwareAcceleration” as the name of the new DWORD value.
• Double click on the new DWORD and change its value from 0 to 1.

Close the registry editor and restart your computer. Check Word works smoother now.

Related: Fix slow Microsoft PowerPoint presentations.

Method #4: Install Latest Updates

If Microsoft Word seems to react poorly to any command for processing a task, you may need to look for updating to the latest service pack. The latest service pack may provide you with bug fixes and performance optimization. Microsoft releases service pack regularly, generally they are installed automatically if you have auto update enabled. Otherwise you need to check and update them manually. Often, by installing a new service pack, you can regain lost performance.

Note that Apple stopped supporting 32-bit applications from macOS Catalina. Hence the 32-bit Mac Word 2011 or 2016 apps will no more work on your Mac. Ensure to purchase Office 365 subscription to work with 64-bit compatible Office apps on your Mac.

Method #5: Update Your OS

Also ensure your Windows OS is getting automatic security updates to make sure that rest of the system is working properly. Microsoft updates Windows 10 automatically, however follow the below steps to check the status and manually update:

• Press “Win + I” keys to open Windows Settings app.
• Click on “Update & Security” section.
• Click on the “Check for updates” button to update your Windows 10 to the latest version.

If you have Mac, you can install the latest updates by following the below instructions:

• Go to “Apple menu” by clicking the Apple icon on top left corner of your Mac.
• Select “System Preferences…” and click on the “Software Update” option.
• Check any update is available and install the latest version.

Related: How to change embedded document name in Word?

Method #6: Reinstall Microsoft Office

If you use Microsoft Office regularly for creating complex documents, spreadsheets and presentations, there’s a possibility that it will become faulty or corrupted. You may remedy it easily by reinstalling Office package. Prepare your Microsoft Office DVD and product key to complete the re-installation process. Follow these steps to uninstall and re-install Office in Windows 10:

• Go to “Control Panel” and choose “Uninstall a program” under “Programs” section.
• Find Microsoft Office and click the “Change” button. Follow steps on the screen to remove the program.
• Use the installation disk or download from the Windows Store for re-installing again.

Note that Word 2000, 2002 and 2003 can be restored to original settings by choosing ‘”Detect and Repair” in the “Help” menu.

On Mac, you can simply drag the application file to trash bin and reinstall from the App Store.

Method #7: Delete “~.dot” or “~.doc” Files

When you open a document, Word will have a temporary file in the same folder indicated by the ~ (tilde) sign at the beginning. Generally, Word will delete them automatically when you save and close your document. however, if you accumulate lot of these temporary files, Word would become slow and sluggish. For example, if you zip the content when the document is open then the compressed folder will include the temporary file in addition to the original file. Follow these steps to cleanup the temporary files:

• Go to Windows Search box and type in ~*.dot.
• Hit enter and wait until you get all the files on your computer. Select all of them, right click and choose “Delete”.
• Repeat the process by typing in ~*.doc in the search box. Also select all of them, right click and choose “Delete”.
• You can also empty your Recycle Bin to save storage space on your PC.

Open Microsoft Word and make sure that the problem has been resolved

Method #8: Optimize Document Files

Often, less optimized document files cause slow performance. Check whether your document has plenty of embedded macros and remove any of them if possible. Many people also put high quality pictures with large resolution inside documents. You need to optimize the pictures by changing the dimension that matches the size of the page. You may also use Adobe Photoshop or Snagit to save these images in optimized format to reduce the file sizes without sacrificing the quality too much.

Method #9: Open Word in Safe Mode

One of the reasons for slow Word app is the corrupted documents. When you try to open corrupted document, Word will show a message like “Microsoft Word is not responding” indicating the problem. You can choose “Restart the program” option to cancel the current operation and try to reopen the file again.

If that does not help, then press “Win + R” keys and open Run Command box. Type “winword /safe” to open Word in safe mode. This will help you to speedup the document as Word will disable extensions and other unnecessary stuffs in safe mode. Sometimes, Word will also suggest to open a document in safe mode. Choose “Yes” to check whether the safe mode can help in improving the performance at least when processing that document.

Related: Fix slow Microsoft Outlook emails.

Method #10: Check File Lock

Some times people share the locked file in chat, emails or SharePoint. When you try to open locked file, Word will show you the “File in Use” warning and ask you to choose the action.

You may notice, slow response time in Word when you open and read locked documents. We recommend you to open the locked file in “Read Only” mode to open it faster. Otherwise, you can request the owner to share the file without edit lock.

Method #11: Disable Add-ins

If you use add-ins to enhance the functionalities then ensure to disable them when you have problem with Word.

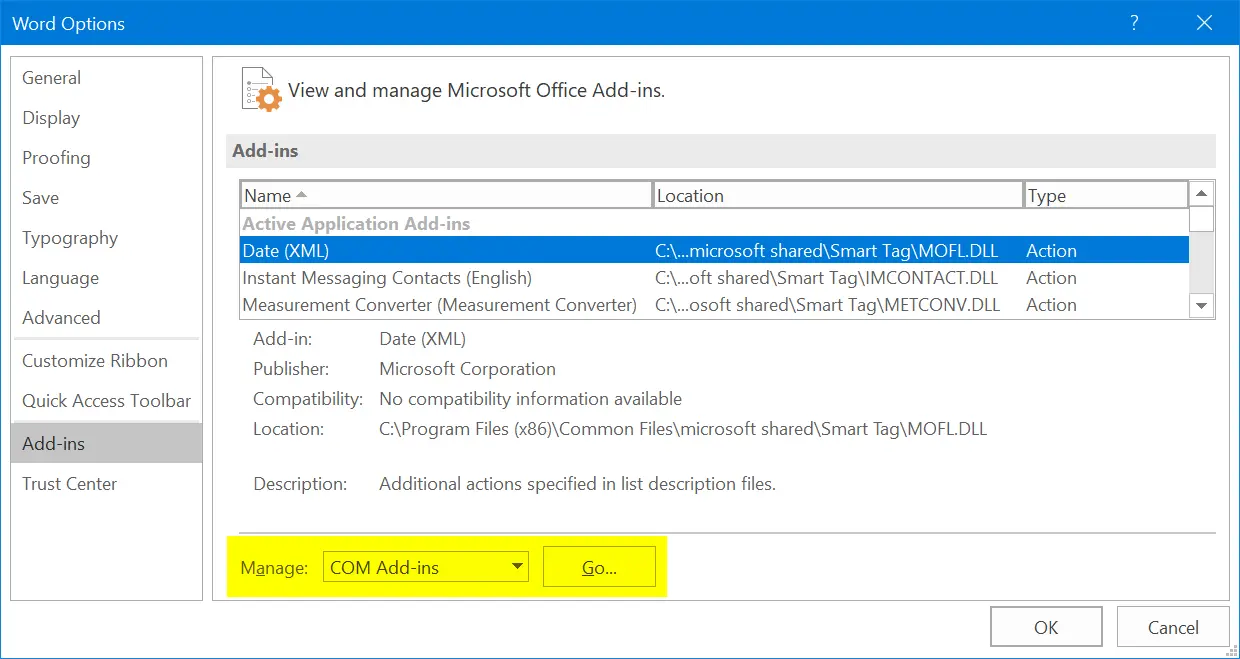
• Go to “File > Options” menu in Word.
• Choose “Add-ins” to view all installed on your Word installation. you can click each add-in to check the function it does.
• Select the add-ins type from the “Manage” drop-down and click on “Go…” button.
• Uncheck the add-ins to disable them.

Check whether this helps to resolve your problems. You can enable the add-ins back any time when needed.

Conclusion

Microsoft Word is almost an essential utility in office workers and many user’s daily life. So when you experience slow response, typing delay or any other problem, it is a good idea to check all the above steps. This will ensure to fix the problems and focus on creating documents with improved productivity.

/en/algebra-topics/introduction-to-word-problems/content/

What are distance word problems?

Distance word problems are a common type of algebra word problems. They involve a scenario in which you need to figure out how fast, how far, or how long one or more objects have traveled. These are often called train problems because one of the most famous types of distance problems involves finding out when two trains heading toward each other cross paths.

In this lesson, you’ll learn how to solve train problems and a few other common types of distance problems. But first, let’s look at some basic principles that apply to any distance problem.

The basics of distance problems

There are three basic aspects to movement and travel: distance, rate, and time. To understand the difference among these, think about the last time you drove somewhere.

The distance is how far you traveled. The rate is how fast you traveled. The time is how long the trip took.

The relationship among these things can be described by this formula:

distance = rate x time
d = rt

In other words, the distance you drove is equal to the rate at which you drove times the amount of time you drove. For an example of how this would work in real life, just imagine your last trip was like this:

• You drove 25 miles—that’s the distance.
• You drove an average of 50 mph—that’s the rate.
• The drive took you 30 minutes, or 0.5 hours—that’s the time.

According to the formula, if we multiply the rate and time, the product should be our distance.

And it is! We drove 50 mph for 0.5 hours—and 50 ⋅ 0.5 equals 25, which is our distance.

What if we drove 60 mph instead of 50? How far could we drive in 30 minutes? We could use the same formula to figure this out.

60 ⋅ 0.5 is 30, so our distance would be 30 miles.

Solving distance problems

When you solve any distance problem, you’ll have to do what we just did—use the formula to find distance, rate, or time. Let’s try another simple problem.

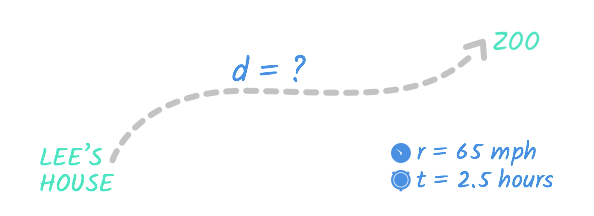
On his day off, Lee took a trip to the zoo. He drove an average speed of 65 mph, and it took him two-and-a-half hours to get from his house to the zoo. How far is the zoo from his house?

First, we should identify the information we know. Remember, we’re looking for any information about distance, rate, or time. According to the problem:

• The rate is 65 mph.
• The time is two-and-a-half hours, or 2.5 hours.
• The distance is unknown—it’s what we’re trying to find.

You could picture Lee’s trip with a diagram like this:

This diagram is a start to understanding this problem, but we still have to figure out what to do with the numbers for distance, rate, and time. To keep track of the information in the problem, we’ll set up a table. (This might seem excessive now, but it’s a good habit for even simple problems and can make solving complicated problems much easier.) Here’s what our table looks like:

distance	rate	time
d	65	2.5

We can put this information into our formula: distance = rate ⋅ time.

We can use the distance = rate ⋅ time formula to find the distance Lee traveled.

d = rt

The formula d = rt looks like this when we plug in the numbers from the problem. The unknown distance is represented with the variable d.

d = 65 ⋅ 2.5

To find d, all we have to do is multiply 65 and 2.5. 65 ⋅ 2.5 equals 162.5.

d = 162.5

We have an answer to our problem: d = 162.5. In other words, the distance Lee drove from his house to the zoo is 162.5 miles.

Be careful to use the same units of measurement for rate and time. It’s possible to multiply 65 miles per hour by 2.5 hours because they use the same unit: an hour. However, what if the time had been written in a different unit, like in minutes? In that case, you’d have to convert the time into hours so it would use the same unit as the rate.

Solving for rate and time

In the problem we just solved we calculated for distance, but you can use the d = rt formula to solve for rate and time too. For example, take a look at this problem:

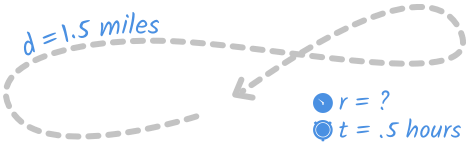
After work, Janae walked in her neighborhood for a half hour. She walked a mile-and-a-half total. What was her average speed in miles per hour?

We can picture Janae’s walk as something like this:

And we can set up the information from the problem we know like this:

distance	rate	time
1.5	r	0.5

The table is repeating the facts we already know from the problem. Janae walked one-and-a-half miles or 1.5 miles in a half hour, or 0.5 hours.

As always, we start with our formula. Next, we’ll fill in the formula with the information from our table.

d = rt

The rate is represented by r because we don’t yet know how fast Janae was walking. Since we’re solving for r, we’ll have to get it alone on one side of the equation.

1.5 = r ⋅ 0.5

Our equation calls for r to be multiplied by 0.5, so we can get r alone on one side of the equation by dividing both sides by 0.5:
1.5 / 0.5 = 3.

3 = r

r = 3, so 3 is the answer to our problem. Janae walked 3 miles per hour.

In the problems on this page, we solved for distance and rate of travel, but you can also use the travel equation to solve for time. You can even use it to solve certain problems where you’re trying to figure out the distance, rate, or time of two or more moving objects. We’ll look at problems like this on the next few pages.

Two-part and round-trip problems

Do you know how to solve this problem?

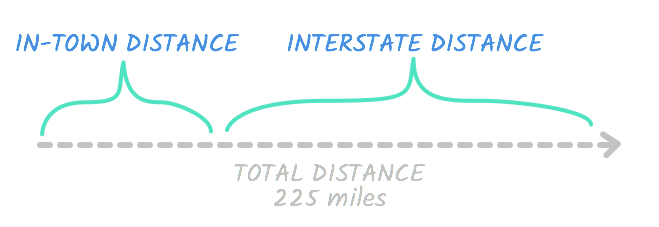
Bill took a trip to see a friend. His friend lives 225 miles away. He drove in town at an average of 30 mph, then he drove on the interstate at an average of 70 mph. The trip took three-and-a-half hours total. How far did Bill drive on the interstate?

This problem is a classic two-part trip problem because it’s asking you to find information about one part of a two-part trip. This problem might seem complicated, but don’t be intimidated!

You can solve it using the same tools we used to solve the simpler problems on the first page:

• The travel equation d = rt
• A table to keep track of important information

Let’s start with the table. Take another look at the problem. This time, the information relating to distance, rate, and time has been underlined.

Bill took a trip to see a friend. His friend lives 225 miles away. He drove in town at an average of 30 mph, then he drove on the interstate at an average of 70 mph. The trip took three-and-a-half hours total. How far did Bill drive on the interstate?

If you tried to fill in the table the way we did on the last page, you might have noticed a problem: There’s too much information. For instance, the problem contains two rates—30 mph and 70 mph. To include all of this information, let’s create a table with an extra row. The top row of numbers and variables will be labeled in town, and the bottom row will be labeled interstate.

	distance	rate	time
in town		30
interstate		70

We filled in the rates, but what about the distance and time? If you look back at the problem, you’ll see that these are the total figures, meaning they include both the time in town and on the interstate. So the total distance is 225. This means this is true:

Interstate distance + in-town distance = Total distance

Together, the interstate distance and in-town distance are equal to the total distance. See?

In any case, we’re trying to find out how far Bill drove on the interstate, so let’s represent this number with d. If the interstate distance is d, it means the in-town distance is a number that equals the total, 225, when added to d. In other words, it’s equal to 225 — d.

We can fill in our chart like this:

	distance	rate	time
in town	225 — d	30
interstate	d	70

We can use the same technique to fill in the time column. The total time is 3.5 hours. If we say the time on the interstate is t, then the remaining time in town is equal to 3.5 — t. We can fill in the rest of our chart.

	distance	rate	time
in town	225 — d	30	3.5 — t
interstate	d	70	t

Now we can work on solving the problem. The main difference between the problems on the first page and this problem is that this problem involves two equations. Here’s the one for in-town travel:

225 — d = 30 ⋅ (3.5 — t)

And here’s the one for interstate travel:

d = 70t

If you tried to solve either of these on its own, you might have found it impossible: since each equation contains two unknown variables, they can’t be solved on their own. Try for yourself. If you work either equation on its own, you won’t be able to find a numerical value for d. In order to find the value of d, we’ll also have to know the value of t.

We can find the value of t in both problems by combining them. Let’s take another look at our travel equation for interstate travel.

While we don’t know the numerical value of d, this equation does tell us that d is equal to 70t.

d = 70t

Since 70t and d are equal, we can replace d with 70t. Substituting 70t for d in our equation for interstate travel won’t help us find the value of t—all it tells us is that 70t is equal to itself, which we already knew.

70t = 70t

But what about our other equation, the one for in-town travel?

225 — d = 30 ⋅ (3.5 — t)

When we replace the d in that equation with 70t, the equation suddenly gets much easier to solve.

225 — 70t = 30 ⋅ (3.5 — t)

Our new equation might look more complicated, but it’s actually something we can solve. This is because it only has one variable: t. Once we find t, we can use it to calculate the value of d—and find the answer to our problem.

To simplify this equation and find the value of t, we’ll have to get the t alone on one side of the equals sign. We’ll also have to simplify the right side as much as possible.

225 — 70t = 30 ⋅ (3.5 — t)

Let’s start with the right side: 30 times (3.5 — t) is 105 — 30t.

225 — 70t = 105 — 30t

Next, let’s cancel out the 225 next to 70t. To do this, we’ll subtract 225 from both sides. On the right side, it means subtracting 225 from 105. 105 — 225 is -120.

— 70t = -120 — 30t

Our next step is to group like terms—remember, our eventual goal is to have t on the left side of the equals sign and a number on the right. We’ll cancel out the -30t on the right side by adding 30t to both sides. On the right side, we’ll add it to -70t. -70t + 30t is -40t.

— 40t = -120

Finally, to get t on its own, we’ll divide each side by its coefficient: -40. -120 / — 40 is 3.

t = 3

So t is equal to 3. In other words, the time Bill traveled on the interstate is equal to 3 hours. Remember, we’re ultimately trying to find the distance Bill traveled on the interstate. Let’s look at the interstate row of our chart again and see if we have enough information to find out.

	distance	rate	time
interstate	d	70	3

It looks like we do. Now that we’re only missing one variable, we should be able to find its value pretty quickly.

To find the distance, we’ll use the travel formula distance = rate ⋅ time.

d = rt

We now know that Bill traveled on the interstate for 3 hours at 70 mph, so we can fill in this information.

d = 3 ⋅ 70

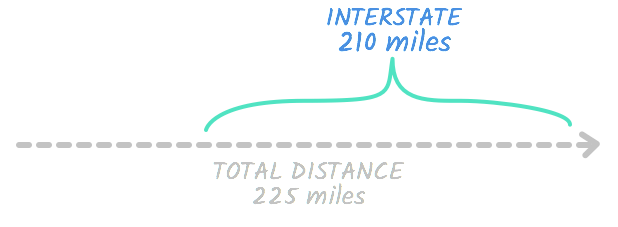
Finally, we finished simplifying the right side of the equation. 3 ⋅ 70 is 210.

d = 210

So d = 210. We have the answer to our problem! The distance is 210. In other words, Bill drove 210 miles on the interstate.

Solving a round-trip problem

It might have seemed like it took a long time to solve the first problem. The more practice you get with these problems, the quicker they’ll go. Let’s try a similar problem. This one is called a round-trip problem because it describes a round trip—a trip that includes a return journey. Even though the trip described in this problem is slightly different from the one in our first problem, you should be able to solve it the same way. Let’s take a look:

Eva drove to work at an average speed of 36 mph. On the way home, she hit traffic and only drove an average of 27 mph. Her total time in the car was 1 hour and 45 minutes, or 1.75 hours. How far does Eva live from work?

If you’re having trouble understanding this problem, you might want to visualize Eva’s commute like this:

As always, let’s start by filling in a table with the important information. We’ll make a row with information about her trip to work and from work.

1.75 — t to describe the trip from work. (Remember, the total travel time is 1.75 hours, so the time to work and from work should equal 1.75.)

From our table, we can write two equations:

• The trip to work can be represented as d = 36t.
• The trip from work can be represented as d = 27 (1.75 — t).

In both equations, d represents the total distance. From the diagram, you can see that these two equations are equal to each other—after all, Eva drives the same distance to and from work.

Just like with the last problem we solved, we can solve this one by combining the two equations.

We’ll start with our equation for the trip from work.

d = 27 (1.75 — t)

Next, we’ll substitute in the value of d from our to work equation, d = 36t. Since the value of d is 36t, we can replace any occurrence of d with 36t.

36t = 27 (1.75 — t)

Now, let’s simplify the right side. 27 ⋅(1.75 — t) is 47.25.

36t = 47.25 — 27t

Next, we’ll cancel out -27t by adding 27t to both sides of the equation. 36t + 27t is 63t.

63t = 47.25

Finally, we can get t on its own by dividing both sides by its coefficient: 63. 47.25 / 63 is .75.

t = .75

t is equal to .75. In other words, the time it took Eva to drive to work is .75 hours. Now that we know the value of t, we’ll be able to can find the distance to Eva’s work.

If you guessed that we were going to use the travel equation again, you were right. We now know the value of two out of the three variables, which means we know enough to solve our problem.

d = rt

First, let’s fill in the values we know. We’ll work with the numbers for the trip to work. We already knew the rate: 36. And we just learned the time: .75.

d = 36 ⋅ .75

Now all we have to do is simplify the equation: 36 ⋅ .75 = 27.

d = 27

d is equal to 27. In other words, the distance to Eva’s work is 27 miles. Our problem is solved.

Intersecting distance problems

An intersecting distance problem is one where two things are moving toward each other. Here’s a typical problem:

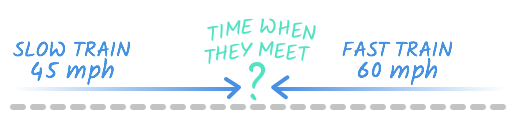
Pawnee and Springfield are 420 miles apart. A train leaves Pawnee heading to Springfield at the same time a train leaves Springfield heading to Pawnee. One train is moving at a speed of 45 mph, and the other is moving 60 mph. How long will they travel before they meet?

This problem is asking you to calculate how long it will take these two trains moving toward each other to cross paths. This might seem confusing at first. Even though it’s a real-world situation, it can be difficult to imagine distance and motion abstractly. This diagram might help you get a sense of what this situation looks like:

If you’re still confused, don’t worry! You can solve this problem the same way you solved the two-part problems on the last page. You’ll just need a chart and the travel formula.

Pawnee and Springfield are 420 miles apart. A train leaves Pawnee heading toward Springfield at the same time a train leaves Springfield heading toward Pawnee. One train is moving at a speed of 45 mph, and the other is moving 60 mph. How long will they travel before they meet?

Let’s start by filling in our chart. Here’s the problem again, this time with the important information underlined. We can start by filling in the most obvious information: rate. The problem gives us the speed of each train. We’ll label them fast train and slow train. The fast train goes 60 mph. The slow train goes only 45 mph.

We can also put this information into a table:

	distance	rate	time
fast train		60
slow train		45

We don’t know the distance each train travels to meet the other yet—we just know the total distance. In order to meet, the trains will cover a combined distance equal to the total distance. As you can see in this diagram, this is true no matter how far each train travels.

This means that—just like last time—we’ll represent the distance of one with d and the distance of the other with the total minus d. So the distance for the fast train will be d, and the distance for the slow train will be 420 — d.

	distance	rate	time
fast train	d	60
slow train	420 — d	45

Because we’re looking for the time both trains travel before they meet, the time will be the same for both trains. We can represent it with t.

	distance	rate	time
fast train	d	60	t
slow train	420 — d	45	t

The table gives us two equations: d = 60t and 420 — d = 45t. Just like we did with the two-part problems, we can combine these two equations.

The equation for the fast train isn’t solvable on its own, but it does tell us that d is equal to 60t.

d = 60t

The other equation, which describes the slow train, can’t be solved alone either. However, we can replace the d with its value from the first equation.

420 — d = 45t

Because we know that d is equal to 60t, we can replace the d in this equation with 60t. Now we have an equation we can solve.

420 — 60t = 45t

To solve this equation, we’ll need to get t and its coefficients on one side of the equals sign and any other numbers on the other. We can start by canceling out the -60t on the left by adding 60t to both sides. 45t + 60t is 105t.

420 = 105t

Now we just need to get rid of the coefficient next to t. We can do this by dividing both sides by 105. 420 / 105 is 4.

4 = t

t = 4. In other words, the time it takes the trains to meet is 4 hours. Our problem is solved!

Practice problem 1

Here’s another intersecting distance problem. It’s similar to the one we just solved. See if you can solve it on your own. When you’re finished, scroll down to see the answer and an explanation.

Jon and Dani live 270 miles apart. One day, they decided to drive toward each other and hang out wherever they met. Jon drove an average of 65 mph, and Dani drove an average of 70 mph. How long did they drive before they met up?

Problem 1 answer

Here’s practice problem 1:

Jon and Dani live 270 miles apart. One day, they decided to drive toward each other and hang out wherever they met. Jon drove an average of 65 mph, and Dani drove 70 mph. How long did they drive before they met up?

Answer: 2 hours.

Let’s solve this problem like we solved the others. First, try making the chart. It should look like this:

	distance	rate	time
Jon	d	65	t
Dani	270 — d	70	t

Here’s how we filled in the chart:

• Distance: Together, Dani and Jon will have covered the total distance between them by the time they meet up. That’s 270. Jon’s distance is represented by d, so Dani’s distance is 270 — d.
• Rate: The problem tells us Dani and Jon’s speeds. Dani drives 65 mph, and Jon drives 70 mph.
• Time: Because Jon and Dani drive the same amount of time before they meet up, both of their travel times are represented by t.

Now we have two equations. The equation for Jon’s travel is d = 65t. The equation for Dani’s travel is 270 — d = 70t. To solve this problem, we’ll need to combine them.

The equation for Jon tells us that d is equal to 65t. This means we can combine the two equations by replacing the d in Dani’s equation with 65t.

270 — 65t = 70t

Let’s get t on one side of the equation and a number on the other. The first step to doing this is to get rid of -65t on the left side. We’ll cancel it out by adding 65t to both sides: 70t + 65t is 135t.

270 = 135t

All that’s left to do is to get rid of the 135 next to the t. We can do this by dividing both sides by 135: 270 / 135 is 2.

2 = t

That’s it. t is equal to 2. We have the answer to our problem: Dani and Jon drove 2 hours before they met up.

Overtaking distance problems

The final type of distance problem we’ll discuss in this lesson is a problem in which one moving object overtakes—or passes—another. Here’s a typical overtaking problem:

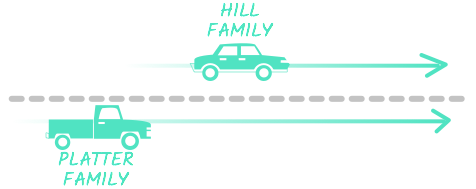
The Hill family and the Platter family are going on a road trip. The Hills left 3 hours before the Platters, but the Platters drive an average of 15 mph faster. If it takes the Platter family 13 hours to catch up with the Hill family, how fast are the Hills driving?

You can picture the moment the Platter family left for the road trip a little like this:

The problem tells us that the Platter family will catch up with the Hill family in 13 hours and asks us to use this information to find the Hill family’s rate. Like some of the other problems we’ve solved in this lesson, it might not seem like we have enough information to solve this problem—but we do. Let’s start making our chart. The distance can be d for both the Hills and the Platters—when the Platters catch up with the Hills, both families will have driven the exact same distance.

	distance	rate	time
the Hills	d
the Platters	d

Filling in the rate and time will require a little more thought. We don’t know the rate for either family—remember, that’s what we’re trying to find out. However, we do know that the Platters drove 15 mph faster than the Hills. This means if the Hill family’s rate is r, the Platter family’s rate would be r + 15.

	distance	rate	time
the Hills	d	r
the Platters	d	r + 15

Now all that’s left is the time. We know it took the Platters 13 hours to catch up with the Hills. However, remember that the Hills left 3 hours earlier than the Platters—which means when the Platters caught up, they’d been driving 3 hours more than the Platters. 13 + 3 is 16, so we know the Hills had been driving 16 hours by the time the Platters caught up with them.

	distance	rate	time
the Hills	d	r	16
the Platters	d	r + 15	13

Our chart gives us two equations. The Hill family’s trip can be described by d = r ⋅ 16. The equation for the Platter family’s trip is d = (r + 15) ⋅ 13. Just like with our other problems, we can combine these equations by replacing a variable in one of them.

The Hill family equation already has the value of d equal to r ⋅ 16. So we’ll replace the d in the Platter equation with r ⋅ 16. This way, it will be an equation we can solve.

r ⋅ 16 = (r + 15) ⋅ 13

First, let’s simplify the right side: r ⋅ 16 is 16r.

16r = (r + 15) ⋅ 13

Next, we’ll simplify the right side and multiply (r + 15) by 13.

16r = 13r + 195

We can get both r and their coefficients on the left side by subtracting 13r from 16r : 16r — 13r is 3r.

3r = 195

Now all that’s left to do is get rid of the 3 next to the r. To do this, we’ll divide both sides by 3: 195 / 3 is 65.

r = 65

So there’s our answer: r = 65. The Hill family drove an average of 65 mph.

You can solve any overtaking problem the same way we solved this one. Just remember to pay special attention when you’re setting up your chart. Just like the Hill family did in this problem, the person or vehicle who started moving first will always have a greater travel time.

Practice problem 2

Try solving this problem. It’s similar to the problem we just solved. When you’re finished, scroll down to see the answer and an explanation.

A train moving 60 mph leaves the station at noon. An hour later, a train moving 80 mph leaves heading the same direction on a parallel track. What time does the second train catch up to the first?

Problem 2 answer

Here’s practice problem 2:

A train moving 60 mph leaves the station at noon. An hour later, a train moving 80 mph leaves heading the same direction on a parallel track. What time does the second train catch up to the first?

Answer: 4 p.m.

To solve this problem, start by making a chart. Here’s how it should look:

	distance	rate	time
fast train	d	80	t
slow train	d	60	t + 1

Here’s an explanation of the chart:

• Distance: Both trains will have traveled the same distance by the time the fast train catches up with the slow one, so the distance for both is d.
• Rate: The problem tells us how fast each train was going. The fast train has a rate of 80 mph, and the slow train has a rate of 60 mph.
• Time: We’ll use t to represent the fast train’s travel time before it catches up. Because the slow train started an hour before the fast one, it will have been traveling one hour more by the time the fast train catches up. It’s t + 1.

Now we have two equations. The equation for the fast train is d = 80t. The equation for the slow train is d = 60 (t + 1). To solve this problem, we’ll need to combine the equations.

The equation for the fast train says d is equal to 80t. This means we can combine the two equations by replacing the d in the slow train’s equation with 80t.

80t = 60 (t + 1)

First, let’s simplify the right side of the equation: 60 ⋅ (t + 1) is 60t + 60.

80t = 60t + 60

To solve the equation, we’ll have to get t on one side of the equals sign and a number on the other. We can get rid of 60t on the right side by subtracting 60t from both sides: 80t — 60t is 20t.

20t = 60

Finally, we can get rid of the 20 next to t by dividing both sides by 20. 60 divided by 20 is 3.

t = 3

So t is equal to 3. The fast train traveled for 3 hours. However, it’s not the answer to our problem. Let’s look at the original problem again. Pay attention to the last sentence, which is the question we’re trying to answer.

A train moving 60 mph leaves the station at noon. An hour later, a train moving 80 mph leaves heading the same direction on a parallel track. What time does the second train catch up to the first?

Our problem doesn’t ask how long either of the trains traveled. It asks what time the second train catches up with the first.

The problem tells us that the slow train left at noon and the fast one left an hour later. This means the fast train left at 1 p.m. From our equations, we know the fast train traveled 3 hours. 1 + 3 is 4, so the fast train caught up with the slow one at 4 p.m. The answer to the problem is 4 p.m.

Updated on October 22, 2018

Elapsed time is the amount of time that passes between the beginning and the end of an event. The concept of elapsed time fits nicely in the elementary school curriculum. Beginning in third grade, students should be able to tell and write time to the nearest minute and solve word problems involving addition and subtraction of time. Reinforce these essential skills with the following elapsed time word problems and games.

Elapsed Time Word Problems

These quick and easy elapsed time word problems are perfect for parents and teachers who want to help students practice elapsed time to the nearest minute with simple mental math problems. Answers are listed below.

1. Sam and his mom arrive at the doctor’s office at 2:30 p.m. They see the doctor at 3:10 p.m. How long was their wait?
2. Dad says dinner will be ready in 35 minutes. It’s 5:30 p.m. now. What time will dinner be ready?
3. Becky is meeting her friend at the library at 12:45 p.m. It takes her 25 minutes to get to the library. What time will she need to leave her house to arrive on time?
4. Ethan’s birthday party started at 4:30 p.m. The last guest left at 6:32 p.m. How long did Ethan’s party last?
5. Kayla put cupcakes in the oven at 3:41 p.m. The directions say that the cupcakes need to bake for 38 minutes. What time will Kayla need to take them out of the oven?
6. Dakota arrived at school at 7:59 a.m. He left at 2:33 p.m. How long was Dakota at school?
7. Dylan started working on homework at 5:45 p.m. It took him 1 hour and 57 minutes to complete it. What time did Dylan complete his homework?
8. Dad arrives home at 4:50 p.m. He left work 40 minutes ago. What time did Dad get off work? 
9. Jessica’s family is traveling from Atlanta, Georgia to New York by plane. Their flight leaves at 11:15 a.m. and should take 2 hours and 15 minutes. What time will their plane arrive in New York?
10. Jordan got to football practice at 7:05 p.m. Steve showed up 11 minutes later. What time did Steve get to practice?
11. Jack ran a marathon in 2 hours and 17 minutes. He crossed the finish line at 10:33 a.m. What time did the race start?
12. Marci was babysitting for her cousin. Her cousin was gone for 3 hours and 40 minutes. Marci left at 9:57 p.m. What time did she start babysitting? 
13. Caleb and his friends went to see a movie at 7:35 p.m. They left at 10:05 p.m. How long was the movie?
14. Francine got to work at 8:10 a.m. She left at 3:45 p.m. How long did Francine work?
15. Brandon went to bed at 9:15 p.m. It took him 23 minutes to fall asleep. What time did Brandon fall asleep?
16. Kelli had to wait in a long, slow-moving line to purchase a popular new video game that was just released. She got in line at 9:15 a.m. She left with the game at 11:07 a.m. How long did Kelli wait in line?
17. Jaydon went to batting practice Saturday morning at 8:30 a.m. He left at 11:42 a.m. How long was he at batting practice?
18. Ashton got behind on her reading assignment, so she had to read four chapters last night. She started at 8:05 p.m. and finished at 9:15 p.m. How long did it take Ashton to catch up on her assignment?
19. Natasha has a dentist appointment at 10:40 a.m. It should last 35 minutes. What time will she finish?
20. Mrs. Kennedy’s 3rd-grade class is going to the aquarium on a field trip. They are scheduled to arrive at 9:10 a.m. and leave at 1:40 p.m. How long will they spend at the aquarium?

Elapsed Time Games

Try these games and activities at home to help your children practice elapsed time.

Daily Schedule

Let your children keep track of their schedule and ask them to figure the elapsed time for each activity. For example, how long did your child spend eating breakfast, reading, taking a bath, or playing video games?

How Long Will It Take?

Give your kids practice with elapsed time by encouraging them to figure out how long daily activities take. For example, the next time you order a pizza online or by phone, you’ll probably be given an estimated delivery time. Use that information to create a word problem that’s relevant to your child’s life, such as, «It’s 5:40 p.m. now and the pizza shop says the pizza will be here at 6:20 p.m. How long will it take for the pizza to arrive?»

Time Dice

Order a set of time dice from online retailers or teacher supply stores. The set contains two twelve-sided dice, one with numbers representing the hours and the other with numbers representing minutes. Take turns rolling the time dice with your child. Each player should roll twice, then calculate the elapsed time between the two resulting dice times. (A pencil and paper will come in handy, as you’ll want to jot down the time of the first roll.)

Elapsed Time Word Problem Answers

1. 40 minutes
2. 6:05 p.m.
3. 12:20 p.m.
4.  2 hours and 2 minutes
5. 4:19 p.m.
6. 6 hours and 34 minutes
7. 7:42 p.m.
8. 4:10 p.m.
9. 1:30 p.m.
10. 7:16 p.m.
11. 8:16 a.m.
12. 6:17 p.m.
13. 2 hours and 30 minutes
14. 7 hours and 35 minutes
15. 9:38 p.m.
16. 1 hour and 52 minutes
17. 3 hours and 12 minutes
18. 1 hour and 10 minutes
19. 11:15 a.m.
20. 4 hours and 30 minutes

Before you get into distance, time and speed word problems, take a few minutes to read this first and understand: How to build your credit score in USA as an international student.

Problems involving Time, Distance and Speed are solved based on one simple formula.

Distance = Speed * Time

Which implies →

Speed = Distance / Time   and

Time = Distance / Speed

Solution

Solution

Solution

Distance covered = 4*40 = 160 miles

Example 4. If a person walks at 4 mph, he covers a certain distance. If he walks at 9 mph, he covers 7.5 miles more. How much distance did he actually cover?

Now we can see that the direct application of our usual formula Distance = Speed * Time or its variations cannot be done in this case and we need to put in extra effort to calculate the given parameters.

Let us see how this question can be solved.

Solution

For these kinds of questions, a table like this might make it easier to solve.

Distance	Speed	Time
d	4	t
d+7.5	9	t

 
Let the distance covered by that person be ‘d’.

Walking at 4 mph and covering a distance ‘d’ is done in a time of ‘d/4’

IF he walks at 9 mph, he covers 7.5 miles more than the actual distance d, which is ‘d+7.5’.

He does this in a time of (d+7.5)/9.

Since the time is same in both the cases →

d/4 = (d+7.5)/9            →        9d = 4(d+7.5)   →        9d=4d+30        →        d = 6.

Solution

Here, we see that the distance is same.

Let us assume that its usual speed is ‘s’ and time is ‘t’, then

Distance	Speed	Time
d	s	t min
d	S+1/3	t+30 min

 
s*t = (1/3)s*(t+30)      →        t = t/3 + 10      →        t = 15.

So the actual time taken to cover the distance is 15 minutes.

Note: Note the time is expressed in terms of ‘minutes’. When we express distance in terms of miles or kilometers, time is expressed in terms of hours and has to be converted into appropriate units of measurement.

Solved Questions on Trains

Example 1. X and Y are two stations which are 320 miles apart. A train starts at a certain time from X and travels towards Y at 70 mph. After 2 hours, another train starts from Y and travels towards X at 20 mph. At what time do they meet?

Solution

Let the time after which they meet be ‘t’ hours.

Then the time travelled by second train becomes ‘t-2’.

Now,

Distance covered by first train+Distance covered by second train = 320 miles

70t+20(t-2) = 320

Solving this gives t = 4.

Solution

Let the speed of the first train be ‘s’.

Distance covered by the first train in (2+4) hours = Distance covered by second train in 4 hours

Therefore, 6s = 60*4

Solving which gives s=40.

So the slower train is moving at the rate of 40 mph.
 

Questions on Boats/Airplanes

For problems with boats and streams,

Speed of the boat upstream (against the current) = Speed of the boat in still water – speed of the stream

[As the stream obstructs the speed of the boat in still water, its speed has to be subtracted from the usual speed of the boat]

Speed of the boat downstream (along with the current) = Speed of the boat in still water + speed of the stream

[As the stream pushes the boat and makes it easier for the boat to reach the destination faster, speed of the stream has to be added]

Similarly, for airplanes travelling with/against the wind,

Speed of the plane with the wind = speed of the plane + speed of the wind

Speed of the plane against the wind = speed of the plane – speed of the wind

Let us look at some examples.

Example 1. A man travels at 3 mph in still water. If the current’s velocity is 1 mph, it takes 3 hours to row to a place and come back. How far is the place?

Solution

Let the distance be ‘d’ miles.

Time taken to cover the distance upstream + Time taken to cover the distance downstream = 3

Speed upstream = 3-1 = 2 mph

Speed downstream = 3+1 = 4 mph

Solution

Let the speed of the plane be ‘a’ and that of the wind be ‘w’.

Our table looks like this:
 

	Distance	Speed	Time
With the wind	2400	a+w	4
Against the wind	2400	a-w	6

 
4(a+w) = 2400 and 6(a-w) = 2400

Expressing one unknown variable in terms of the other makes it easier to solve, which means

a+w = 600 → w=600-a

Substituting the value of w in the second equation,

a-w = 400

a-(600-a) = 400 → a = 500

The speed of the plane is 500 kmph and that of the wind is 100 kmph.
 

More solved examples on Speed, Distance and Time

Example 1. A boy travelled by train which moved at the speed of 30 mph. He then boarded a bus which moved at the speed of 40 mph and reached his destination. The entire distance covered was 100 miles and the entire duration of the journey was 3 hours. Find the distance he travelled by bus.

Solution

	Distance	Speed	Time
Train	d	30	t
Bus	100-d	40	3-t

Let the time taken by the train be ‘t’. Then that of bus is ‘3-t’.

The entire distance covered was 100 miles

So, 30t + 40(3-t) = 100

Solving which gives t=2.

Substituting the value of t in 40(3-t), we get the distance travelled by bus is 40 miles.

Alternatively, we can add the time and equate it to 3 hours, which directly gives the distance.

d/30 + (100-d)/40 = 3

Solution

Our table looks like this.

	Distance	Speed	Time
1st part of journey	d	100	t
2nd part of journey	630-d	110	6-t

Assuming the distance covered in the 1^st part of journey to be ‘d’, the distance covered in the second half becomes ‘630-d’.

Assuming the time taken for the first part of the journey to be ‘t’, the time taken for the second half becomes ‘6-t’.

From the first equation, d=100t

The second equation is 630-d = 110(6-t).

Substituting the value of d from the first equation, we get

630-100t = 110(6-t)

Solving this gives t=3.

Solution

	Distance	Speed	Time
First person	d	3	t
Second person	20-d	4	t

 
Assuming the distance travelled by the first person to be ‘d’, the distance travelled by the second person is ’20-d’.

The time is ‘t’ for both of them because when they meet, they would have walked for the same time.

Since time is same, we can equate as

d/3 = (20-d)/4

Solving this gives d=60/7 miles (8.5 miles approximately)

Then t = 20/7 hours

So the two persons meet after 2 6/7 hours.
 

Practice Questions for you to solve

---

Problem 1: Click here

Answer 1: Click here

---

Problem 2: Click here

Answer 2: Click here

---

Click here to see ALL problems on Miscellaneous Word Problems

Question 299092: This word problem is from the section of the chapter dealing with: Inverse and Joint Variation (Please solve showing steps) The time it takes to get a sunburn varies inversely as the UV rating. At a UV rating of 6 the time it takes to obtain a sunburn can be as little as 15 minutes. Determine the time it will take to get a sunburn when the UV rating is 10. (Scroll Down for Answer!)

Did you know that Algebra.Com has hundreds of free volunteer tutors who help people with math homework? Anyone can ask a math question, and most questions get answers! OR get immediate PAID help on:

Answer by stanbon(75887)   (Show Source):

You can put this solution on YOUR website!
The time it takes to get a sunburn varies inversely as the UV rating. At a UV rating of 6 the time it takes to obtain a sunburn can be as little as 15 minutes. Determine the time it will take to get a sunburn when the UV rating is 10.
——————
t = k/»uv»
—-
Find «k» if t = 15 when uv= 6
15 = k/6
k = 90
——

General Equation for this problem;
t = 90/»uv»
—
Determine the time it will take to get a sunburn when the UV rating is 10.
t(10) = 90/10
time = 9 minutes
=======================
Cheers,
Stan H.

---