If you are looking for tips and ideas for how to teach word problems to your elementary students, then you’ve found the right place! We know that teaching elementary students how to solve word problems is important for math concept and skill application, but it sure can feel like a daunting charge without knowing about the different types, the best practices for teaching them, and common misconceptions to plan in advance for, as well as having the resources you need. All this information will make you feel confident about how to teach addition, subtraction, multiplication, and division word problems! Teaching students how to solve word problems will be so much easier!
This blog post will address the following questions:
- What is a word problem?
- What is a multi-step word problem?
- Why are elementary math word problems important?
- Why are math word problems so hard for elementary students?
- What are the types of word problems?
- How do I teach math word problems in a systematic way?
- What are the best elementary math word problem strategies I can teach my students and what are some tips for how to teach math word problems strategies?
- Do you have any helpful tips for how to teach word problems?
- What are the common mistakes I should look for that my students may make?
- How do I address my students’ common misconceptions surrounding elementary math word problems?
What is a Word Problem?
A word problem is a math situation that calls for an equation to be solved. Students must apply their critical thinking skills to determine how to solve the problem. Word problems give students the opportunity to practice turning situations into numbers. This is critical as students progress in their education, as well as in their day-to-day life. By teaching students how to solve word problems in a strategic way, you are setting them up for future success!
What is a Multi-Step Word Problem?
A multi-step word problem, also known as a two-step word problem or two-step equation word problem, is a math situation that involves more than one equation having to be answered in order to solve the ultimate question. This requires students to apply their problem solving skills to determine which operation or operations to use to tackle the problem and find the necessary information. In some cases, the situation may call for mixed operations, and in others the operations will be the same. Multi-step word problems offer students the opportunity to practice the skill of applying different math concepts with a given problem.
Why are Word Problems Important in Math?
Word problems are essential in math because they give students the opportunity to apply what they have learned to a real life situation. In addition, it facilitates students in developing their higher order thinking and critical thinking skills, creativity, positive mindset toward persevering while problem solving, and confidence in their math abilities. Word problems are an effective tool for teachers to determine whether or not students understand and can apply the concepts and skills they learned to a real life situation.
Why do Students Struggle with Math Word Problems?
Knowing why students have trouble with word problems will help you better understand how to teach them. The reason why math word problems are difficult for your students is because of a few different reasons. First, students need to be able to fluently read and comprehend the text. Second, they need to be able to identify which operations and steps are needed to find the answer. Finally, they need to be able to accurately calculate the answer. If you have students who struggle with reading or English is their second language (ESL), they may not be able to accurately show what they know and can do because of language and literacy barriers. In these cases, it is appropriate to read the text aloud to them or have it translated into their native language for assignments and assessments.
Types of Word Problems
Knowing the different types of word problems will help you better understand how to teach math word problems. Read below to learn about the four types of basic one-step addition and subtraction word problems, the subcategories within each of them, and specific examples for all of them. Two-step equation word problems can encompass two of the same type or two separate types (also known as mixed operation word problems).
1. Join
This type of word problem involves an action that increases the original amount. There are three kinds: Result unknown, change unknown, and initial quantity unknown.
Result Unknown
Example: There were 7 kids swimming in the pool. 3 more kids jumped in. How many kids are in the pool now? (7 + 3 = ?)
Change Unknown
Example: There were 8 kids swimming in the pool. More kids jumped in. Now there are 15 kids in the pool. How many kids jumped in? (8 + ? = 15)
Initial Quantity Unknown
Example: There were kids swimming in the pool. 2 kids jumped in. Now there are 6 kids in the pool. How many kids were swimming in the pool at first? (? + 2 = 6)
2. Separate
This type of word problem involves an action that decreases the original amount. There are three kinds: Result unknown, change unknown, and initial quantity unknown.
Result Unknown
Example: There were 12 kids swimming in the pool. 6 of the kids got out of the pool. How many kids are in the pool now? (12 – 6 = ?)
Change Unknown
Example: There were 9 kids swimming in the pool. Some of the kids got out of the pool. Now there are 4 kids in the pool. How many kids got out of the pool? (9 – ? = 4)
Initial Quantity Unknown
Example: There were kids swimming in the pool. 3 of the kids got out of the pool. Now there are 2 kids in the pool. How many kids were in the pool at first? (? – 3 = 2)
 |
 |
 |
 |
 |
 |
3. Part-Part-Whole
This type of word problem does not involve an action like the join and separate types. Instead, it is about defining relationships among a whole and two parts. There are two kinds: result unknown and part unknown.
Result Unknown
Example: There are 5 boys and 9 girls swimming in the pool. How many kids are in the pool? (5 + 9 = ?)
Part Unknown
Example: There are 12 kids swimming in the pool. 8 of them are girls and the rest of them are boys. How many boys are swimming in the pool? (8 + ? = 12)
4. Compare
This type of word problem does not involve an action or relationship like the three other types. Instead, it is about comparing two different unrelated items. There are two kinds: Difference unknown and quantity unknown.
Difference Unknown
Example: There are 2 kids in the pool. There are 7 kids in the yard. How many more kids are in the yard than in the pool? (2 + ? = 7 or 7 – 2 = ?)
Quantity Unknown
Example 1: There are 5 kids in the pool. There are 3 fewer kids playing in the yard. How many kids are playing in the yard? (5 – 3 = ?)
Example 2: There are 2 kids in the pool. There are 10 more kids playing in the yard than in the pool. How many kids are playing in the yard? (2 + 10 = ?)
How to Solve Word Problems in 5 Easy Steps
Here are 5 steps that will help you teach word problems to your 1st, 2nd, 3rd, 4th or 5th grade students:
- Read the problem.
- Read the problem a second time and make meaning of it by visualizing, drawing pictures, and highlighting important information (numbers, phrases, and questions).
- Plan how you will solve the problem by organizing information in a graphic organizer and writing down equations and formulas that you will need to solve.
- Implement the plan and determine answer.
- Reflect on your answer and determine if it is reasonable. If not, check your work and start back at step one if needed. If the answer is reasonable, check your answer and be prepared to explain how you solved it and why you chose the strategies you did.
5 Math Word Problem Strategies
Here are 5 strategies for how to teach elementary word problems:
Visualize
Understand the math situation and what the question is asking by picturing what you read in your head while you are reading.
Draw Pictures
Make meaning of what the word problem is asking by drawing a picture of the math situation.
Make Models
Use math tools like base-ten blocks to model what is happening in the math situation.
Highlight Important Information
Underline or highlight important numbers, phrases, and questions.
Engage in Word Study
Look for key words and phrases like “less” or “in all.” Check out this blog post if you are interested in learning more about math word problem keywords and their limitations.
10 Tips for Teaching Students How to Solve Math Word Problems
Here are 10 tips for how to teach math word problems:
- Model a positive attitude toward word problems and math.
- Embody a growth mindset.
- Model! Provide plenty of direct instruction.
- Give lots of opportunities to practice.
- Explicitly teach strategies and post anchor charts so students can access them and remember prior learning.
- Celebrate the strategies and process rather than the correct answer.
- Encourage students to continue persevering when they get stuck.
- Invite students to act as peer tutors.
- Provide opportunities for students to write their own word problems.
- Engage in whole-group discussions when solving word problems as a class.
Common Misconceptions and Errors When Students Learn How to Solve Math Word Problems
Here are 5 common misconceptions or errors elementary students have or make surrounding math word problems:
1. Use the Incorrect Operation
Elementary students often apply the incorrect operations because they pull the numbers from a word problem and add them without considering what the question is asking them or they misunderstand what the problem is asking. Early in their experience with word problems, this strategy may work most of the time; however, its effectiveness will cease as the math gets more complex. It is important to instruct students to develop and apply problem-solving strategies.
Although helpful in determining the meaning, elementary students rely solely on key words and phrases in a word problem to determine what operation is being called for. Again, this may be an effective strategy early on in their math career, but it should not be the only strategy students use to determine what their plan of attack is.
2. Get Stuck in a Fixed Mindset
Some elementary students give up before starting a word problem because they think all word problems are too hard. It is essential to instill a positive mindset towards math in students. The best way to do that is through modeling. If you portray an excitement for math, many of your students will share that same feeling.
3. Struggle with Reading Skills Component
For first and second graders (as well as struggling readers and ESL students), it is common for students to decode the text incorrectly. Along the same lines, some elementary students think they can’t solve word problems because they do not know how to read yet. The purpose of word problems is not to assess whether a child can read or not. Instead, the purpose is to assess their critical thinking and problem-solving skills. As a result, it is appropriate to read word problems to elementary students.
4. Calculate Incorrectly
You’ll find instances where students will understand what the question is asking, but they will calculate the addends or the subtrahend from the minuend incorrectly. This type of error is important to note when analyzing student responses because it gives you valuable information for when you plan your instruction.
5. Encode Response Incorrectly
Another error that is important to note when analyzing student responses is when you find that they encode their solution in writing incorrectly. This means they understand what the problem is asking, they solve the operations correctly, document their work meticulously, but then write the incorrect answer on the line.
How to Address Common Misconceptions Surrounding Math Word Problems
You might be wondering, “What can I do in response to some of these misconceptions and errors?” After collecting and analyzing the data, forming groups based on the results, and planning differentiated instruction, you may want to consider trying out these prompts:
- Can you reread the question aloud to me?
- What is the question asking us to do?
- How can we represent the information and question?
- Can we represent the information and question with an equation?
- What is our first step?
- What is our next step?
- Can you think of any strategies we use to help us solve?
- How did you find your answer?
- Can you walk me through how you found your answer step by step?
- What do we need to remember when recording our answer?
Now that you have all these tips and ideas for how to teach word problems, we would love for you to try these word problem resources with your students. They offer students opportunities to practice solving word problems after having learned how to solve word problems. You can download word problem worksheets specific to your grade level (along with lots of other math freebies) in our free printable math resources bundle using this link: free printable math activities for elementary teachers.
Check out my monthly word problem resources!
- 1st Grade Word Problems
- 2nd Grade Word Problems
- 3rd Grade Word Problems
- 4th Grade Word Problems
- 5th Grade Word Problems


It’s one thing to solve a math equation when all of the numbers are given to you but with word problems, when you start adding reading to the mix, that’s when it gets especially tricky.
The simple addition of those words ramps up the difficulty (and sometimes the math anxiety) by about 100!
How can you help your students become confident word problem solvers? By teaching your students to solve word problems in a step by step, organized way, you will give them the tools they need to solve word problems in a much more effective way.
Here are the seven strategies I use to help students solve word problems.
1. Read the Entire Word Problem
Before students look for keywords and try to figure out what to do, they need to slow down a bit and read the whole word problem once (and even better, twice). This helps kids get the bigger picture to be able to understand it a little better too.
2. Think About the Word Problem
Students need to ask themselves three questions every time they are faced with a word problem. These questions will help them to set up a plan for solving the problem.
Here are the questions:
A. What exactly is the question?
What is the problem asking? Often times, curriculum writers include extra information in the problem for seemingly no good reason, except maybe to train kids to ignore that extraneous information (grrrr!). Students need to be able to stay focused, ignore those extra details, and find out what the real question is in a particular problem.
B. What do I need in order to find the answer?
Students need to narrow it down, even more, to figure out what is needed to solve the problem, whether it’s adding, subtracting, multiplying, dividing, or some combination of those. They’ll need a general idea of which information will be used (or not used) and what they’ll be doing.
This is where key words become very helpful. When students learn to recognize that certain words mean to add (like in all, altogether, combined), while others mean to subtract, multiply, or to divide, it helps them decide how to proceed a little better
Here’s a Key Words Chart I like to use for teaching word problems. The handout could be copied at a smaller size and glued into interactive math notebooks. It could be placed in math folders or in binders under the math section if your students use binders.
One year I made huge math signs (addition, subtraction, multiplication, and divide symbols) and wrote the keywords around the symbols. These served as a permanent reminder of keywords for word problems in the classroom.
If you’d like to download this FREE Key Words handout, click here:

C. What information do I already have?
This is where students will focus in on the numbers which will be used to solve the problem.
3. Write on the Word Problem
This step reinforces the thinking which took place in step number two. Students use a pencil or colored pencils to notate information on worksheets (not books of course, unless they’re consumable). There are lots of ways to do this, but here’s what I like to do:
- Circle any numbers you’ll use.
- Lightly cross out any information you don’t need.
- Underline the phrase or sentence which tells exactly what you’ll need to find.
4. Draw a Simple Picture and Label It
Drawing pictures using simple shapes like squares, circles, and rectangles help students visualize problems. Adding numbers or names as labels help too.
For example, if the word problem says that there were five boxes and each box had 4 apples in it, kids can draw five squares with the number four in each square. Instantly, kids can see the answer so much more easily!
5. Estimate the Answer Before Solving
Having a general idea of a ballpark answer for the problem lets students know if their actual answer is reasonable or not. This quick, rough estimate is a good math habit to get into. It helps students really think about their answer’s accuracy when the problem is finally solved.
6. Check Your Work When Done
This strategy goes along with the fifth strategy. One of the phrases I constantly use during math time is, Is your answer reasonable? I want students to do more than to be number crunchers but to really think about what those numbers mean.
Also, when students get into the habit of checking work, they are more apt to catch careless mistakes, which are often the root of incorrect answers.
7. Practice Word Problems Often
Just like it takes practice to learn to play the clarinet, to dribble a ball in soccer, and to draw realistically, it takes practice to become a master word problem solver.
When students practice word problems, often several things happen. Word problems become less scary (no, really).
They start to notice similarities in types of problems and are able to more quickly understand how to solve them. They will gain confidence even when dealing with new types of word problems, knowing that they have successfully solved many word problems in the past.
If you’re looking for some word problem task cards, I have quite a few of them for 3rd – 5th graders.
This 3rd Grade Math Task Cards Bundle has word problems in almost every one of its 30 task card sets.
There are also specific sets that are dedicated to word problems and two-step word problems too. I love these because there’s a task card set for every standard.
CLICK HERE to take a look at 3rd grade:

This 4th Grade Math Task Cards Bundle also has lots of word problems in almost every single of its 30 task card sets. These cards are perfect for centers, whole class, and for one on one.
CLICK HERE to see 4th grade:

This 5th Grade Math Task Cards Bundle is also loaded with word problems to give your students focused practice.
CLICK HERE to take a look at 5th grade:

Want to try a FREE set of math task cards to see what you think?
3rd Grade: Rounding Whole Numbers Task Cards
4th Grade: Convert Fractions and Decimals Task Cards
5th Grade: Read, Write, and Compare Decimals Task Cards
Thanks so much for stopping by!

Ah, word problems. They are SO important for our students to understand, yet one of the most challenging parts of math instruction. Word problems are unique in that they are not JUST about math – they require reading comprehension, as well. Therefore, even students who excel at math can often struggle with word problems. As teachers, it can be difficult to know which word problem strategies to teach.
In this post, I am going to share some of the common strategies for teaching word problems and why they are or aren’t effective. I would also highly encourage you to check out this post, where I share ideas for reaching all your students in your word problem instruction.
Math Word Problem Solving Strategies
There are many word problem strategies out there, but some are more effective than others. Let’s take a look at some of the different strategies and whether or not they are beneficial.

Teaching Keywords in Word Problem Instruction
Growing up, many of us were probably told that certain words in story problems indicated a specific operation. For example, “more” means addition, “fewer” means subtraction, “each” means multiplication. And while often this is the case, sometimes it is not. For example, take the following problem:
Katie picked 4 apples fewer than Marvin. If Katie picked 12 apples, how many did Marvin pick?
In this problem, the word “fewer” is used. Normally this would indicate subtraction, but if you really look at what the question is asking, you will find that we actually need to add to solve. Because of this, the keyword strategy is not effective.
That being said, I personally believe the words and language used in a problem are still worth noting. The word “fewer” is still significant; the problem lies in assuming it automatically means subtraction. As a teacher, I always taught my students to pay attention to important words, but never taught them that certain words always mean a certain operation.
Instead of using the term “keywords,” I refer to them as “important words” or “context clues”. Because reading comprehension is a major part of solving word problems, we cannot entirely ignore the language used. I do think it’s worth taking the time to encourage students to look for certain words in problems, such as the words listed above. The important thing is that we do not tell them what to do with those words – only that they are important. More on this later, but I thought it was worth noting here.
Using Attack Strategies
Another common strategy for teaching word problems is what’s known as an attack strategy. Attack strategies involve a series of steps (or “plan of attack”) to follow when solving word problems. Common attack strategies include:
- RDW (Read the problem, Draw a model, Write the equation)
- CUBES (Circle the numbers, Underline the question, Box the keywords/context clues, Evaluate the problem, Solve & check)
- RUN (Read the problem, Underline the question, Name the problem type)
- FOPS (Find the problem, Organize information with a diagram, Plan to solve the problem, Solve the problem)

There are many more – these are just a few examples. Attack strategies can certainly be helpful when used correctly. They are easy to remember and give students a clear plan for solving. Many attack strategies use fun acronyms like the ones listed above; however even strategies that do not spell out words can still be effective.
These strategies are effective largely because they focus on reading and understanding the problem first, and then solving. As we all know, many students like to simply pull out the numbers and start doing math instead of actually taking the time to read the problem. Attack strategies help solve that issue.
Numberless Word Problems
In 2014, Brian Bushart popularized the idea of removing the numbers from word problems. This is to help students understand what is actually happening in the problem. He details the process in this blog post, which is a GREAT read and I highly recommend checking it out (once you’re done reading this one!).

In short, numberless word problems are effective because they cause students to take a step back and really look at what the problem is asking. Eventually, of course, you’ll want to add the numbers back in. But starting out with the numbers removed and engaging in a discussion of what is actually happening in the problem is an effective first step in gaining comprehension.
Schema-Based Instruction
Of all the word problem strategies out there, schema-based instruction is the one with the most research backing it. Schema-based instruction (or SBI) involves categorizing word problems into particular types, or schemas, which will help you determine how to solve the problem.
Schemas can be additive or multiplicative. There are 3 main additive schemas: combine, compare, and change. Combine problems involve putting together two or more numbers to find a total. Compare involves finding the greater set, lesser set, or the difference. In change problems, an amount either increases or decreases over time.

Likewise, there are also 3 main multiplicative schemas: equal groups, comparison, and proportions. Equal groups involves a unit or group being multiplied by a specific number to find a product. In comparison problems, a set is multiplied a number of times for a product. The proportions schema focuses on the relationships among quantities.

The idea behind schema-based instruction is that all word problems fit into one of these schemas. Each schema has a unique graphic organizer and process for solving a problem that fits that schema. SBI is often accompanied with an attack strategy – such as RUN, for example. Students will first Read the problem, then Underline the questions, and finally Name the problem type (schema) before solving. This last step of identifying the schema will help students understand how to solve the problem.
Part-Part-Whole Instruction
This isn’t really a strategy, per se. But, it is an important concept for students to understand in order to be successful with word problems. Understanding part-part-whole relationships is a critical aspect of number sense. My approach to teaching word problems involves a major emphasis on part-part-whole. Granted – I taught second grade, where word problems were mostly solved by addition and subtraction. Part-part-whole works with multiplication and division, too, but looks a little bit different.
My process for walking students through word problems always included having students identify whether each number represented a part or the whole, and which (a part or the whole) was missing. This was helpful because my students knew that a missing part means subtraction, and a missing whole means addition.

Identifying what each number represented is where the “keywords” I mentioned above come in. Words like “more,” “fewer,” and “each” – while they do NOT tell us how to solve – are important context clues to help us decide whether each number given is a part or the whole.
I used the CUBES attack strategy to go with this. However, instead of boxing “keywords,” we boxed “context clues”. Again, those words are still important – they just don’t tell us how to solve. The words are important because they tell us which numbers are greater or less than other numbers, or if a certain number represents a group. All are important things to know in determining the parts and the whole.
Once students identify what each number represents, they are able to solve. I’ve found that students who were correctly able to determine the parts and the whole in a word problem were VERY successful in finding the answer.

Free Training for Word Problem Strategies
Want to learn more about how to effectively teach word problems? I’m hosting a FREE video training series that you won’t want to miss! It’s launching THIS MONDAY, 9/21, so make sure you join the waitlist so you don’t miss out. Upon signing up, you will receive my free 30-page e-book for how to differentiate word problem instruction in your classroom. CLICK HERE TO JOIN THE WAITLIST, or sign up using the form below.
How do you teach word problems?

Word problems are a special kind of math challenge. Not only do they require computation skills, but they also test students’ reading comprehension and problem-solving abilities. This can be the perfect storm of frustration for many kids. But it doesn’t have to be so hard! Today, I’m sharing easy strategies for helping students master the word problem!
Start With Reading Comprehension
The first step in conquering word problems goes right back to good ol’ reading comprehension. Kids have a bad habit of reading word problems like they are just passing through words to get to the numbers. Blah, blah, blah. 127! Blah, blah, blah, 52! They search for numbers and then rush to DO something with those numbers.
If our students are going to find success with word problems, they need to use their good reading strategies! Teach your students to:
Reread: Slow down and reread as many times as needed
Visualize: Imagine what is taking place in the problem. What is the order of events?
Ask questions: What do I know? What do I need to find out?
Make predictions: What would be a reasonable result? Do I expect the answer to be more or less than the numbers I’m given?
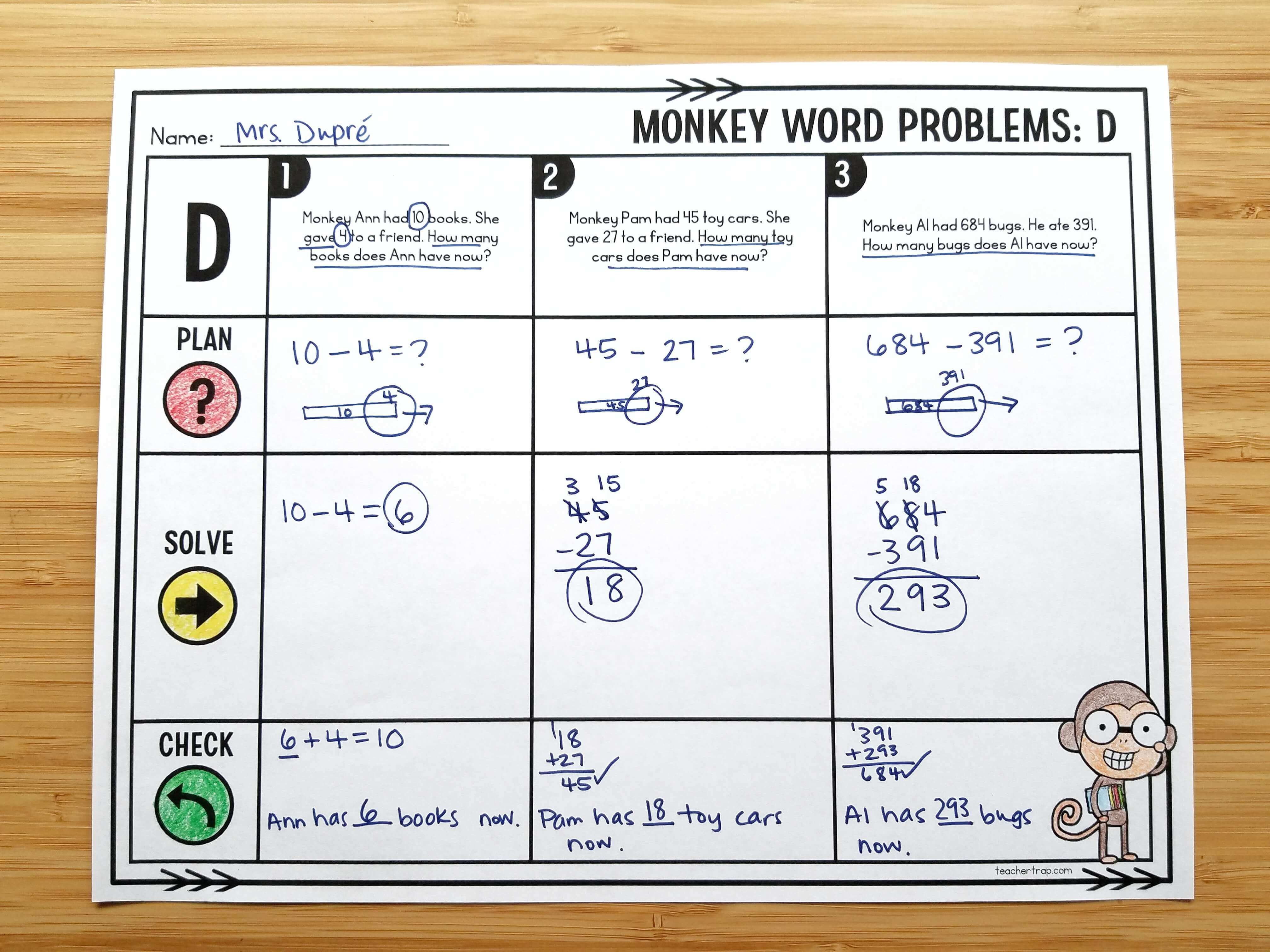
Teach a Problem-Solving Routine
Help students form effective thinking habits by teaching and practicing a problem-solving process. I’m not talking about a rote set of steps like CUBES (Circle the numbers, Underline the question, Box key words, etc.). I’m talking about a THINKING routine that helps the child learn the critical thinking skills involved in solving real problems.
I’ve found a simple PLAN, SOLVE, CHECK format works best. With young children, the biggest issue I find is that they simply skip (or struggle with) one of these steps, which causes a breakdown in their ability to solve the problem. In fact, I form my guided math groups for word problems around those three categories (planning strategies, solving strategies, checking strategies.) **You can grab a FREE Word Problem Thinking Mat in the Preview File of my Tiered Word Problems Pack.
Compare Problem Structures
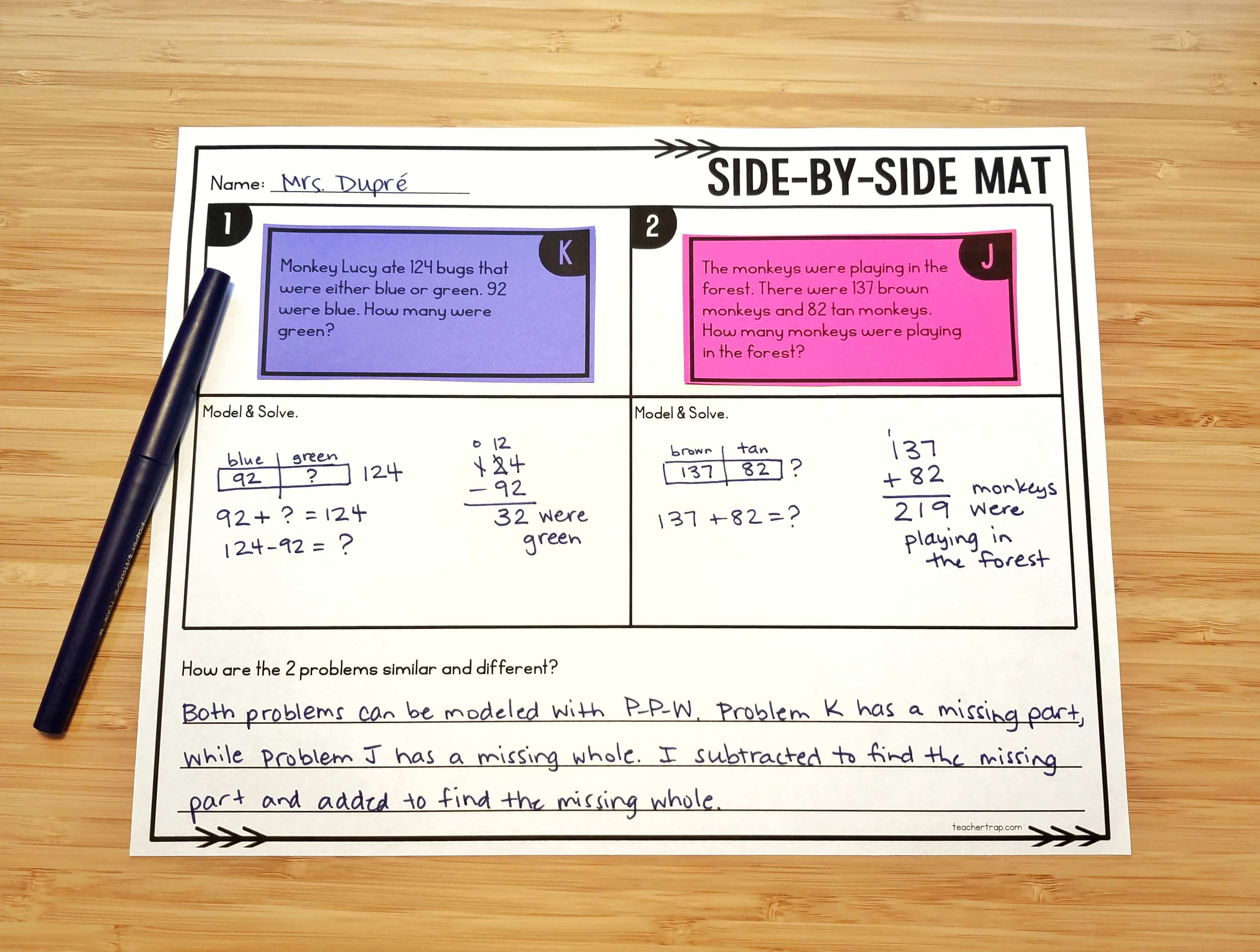
One of my favorite word problem teaching tools is something I call “Side-by-Sides.” This is simply where we solve two different problems and then compare the structure of the problem and the operation used.
When students model, solve, and discuss the different problem types, they grow a deeper understanding of how word problems work.
**You can grab FREE samples of side-by-side problems in the Preview File of my Side-by-Side Word Problems Pack or grab a FREE Side-by-Side Mat in the Preview File of my Tiered Word Problems Pack for 2nd Grade or 3rd Grade.
Start With the Easy Version
Differentiation isn’t just for reading! Many students get overwhelmed when they see large numbers in word problems, so why not meet them where they are? I use what I call “Tiered Word Problems” to help students understand word problem structures and grow confidence before tackling the on-level version.
In my Word Problem Solving Bundle, I provide all kinds of leveled word problems – Tiered Problem Pages, Task Cards, Exit Tickets, and more, that all increase in challenge from first through fifth grade. This allows me to scaffold instruction by student need, and throughout the school year.
**You can grab free goodies and sample pages from the Bundle in the Word Problem Solving Bundle Sampler Pack.
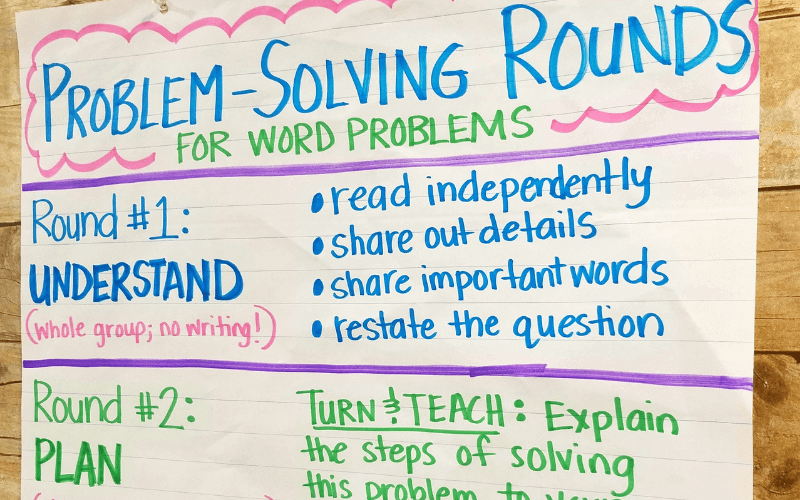
Use Problem-Solving Rounds
Problem-Solving Rounds changed math instruction in my classroom forever! This powerful routine encourages students to move through the problem-solving process I mentioned at the beginning of this post, to explain their thinking, and to reflect on other possible strategies and solutions.
Since using Problem-Solving Rounds, my students are more confident with word problems, have a deeper understanding of problem-types, and are better able to tackle new and challenging tasks.
The basic structure of the rounds follows the Problem-Solving Process I’ve shared. In Round 1, students read and discuss the problem with a focus on UNDERSTANDING. In Round 2, they turn to a partner and explain how they PLAN to solve the problem. They SOLVE the problem independently in Round 3, and in Round 4 they work with a partner and as a whole class to CHECK and reflect on their work. For a detailed guide to this process, check out my blog post all about Problem-Solving Rounds.
I’d love to hear from you! Comment below with your favorite word problem tips for teachers!

3-Step System
1. Read: Read the problem and decide what the question is asking.
- Read the problem 2 times or more.
- Underline or circle key words, phrases, and numbers. Draw a line through irrelevant information.
2. Plan: Think about what the story is asking you to do. What information are you given, and what do you need to find out?
- Draw a picture.
- Circle or underline key words. (Use highlighters or crayons to color-code key numbers and phrases.)
- Write out the question in your own words.
3. Solve: What strategy could you use to find the missing information: addition, subtraction, multiplication, or division?
- Write a number sentence and solve.
- Use counters.
- Create charts.
Check your work by explaining your reasoning. Does your answer make sense?
Download this free strategy checklist from Math Fundamentals to help your child solve word problems.
Different Strategies to Solve Word Problems
Everyone learns in a different way. What makes sense to one individual often isn’t the easiest option for another. Incorporating different strategies to solve word problems can help your child discover what strategy works best for him or her. A few tips to use are:
1. Circle numbers in a story and underline key phrases.
Color coding is a fun method to incorporate to help children decide what operation the question is asking for. Assign a color to each operation and highlight the phrase that identifies it. For example, red links to addition and blue links to subtraction.
2. Incorporate a key word list.
Key word lists are best used for teaching younger children how to solve word problems. As math curriculum advances, children should not be dependent on a key word list to solve a problem. The questions get trickier.
Addition
In all
Together
Total
Altogether
Combine
Sum
Join
Subtraction
Difference
Fewer
How many more
How much more
Left
Remain
Less
3. Visuals
If your child is a visual learner, drawing a picture or using counters can help him or her understand what the problem is asking. Use number lines, charts, or counters or draw a picture.
4. Write your own word problem.
Knowing what is needed to write a word problem is the first step in identifying key words to solve a story. Take turns writing your own word problems with your child and exchange them to solve.
5. Stay organized.
It is important to write clearly and keep work space neat so children can read and follow their own computations. Many children need a separate piece of paper to allow them enough space to solve and understand their answer. Graphing paper is a great option to help students record neat work.
Download this free sample word problem from Math Fundamentals, grade 1.
How to solve a two-step word problem
In a two-step word problem children are being asking to solve two related equations. These can get tricky for children to understand when they transition from one-step to two-step problems. Help your child understand his or her relationships within two-step word problems with these strategies:
1. Circle important information.
Circle numbers and important phrases that ask questions. The number sentences needed to solve these equations are hidden in those asking questions. Identify the first and second questions needed to solve.
2. Distinguish the two parts of the problem.
First identify the first step of the first part of the word problem. Write a number sentence and solve.
3. Use the answer from the first-step solution to the whole problem.
Use the answer from the first question to help you solve the next equation. What operation does the second question require?
Check your work by explaining your reasoning. What was the question answered? Is the answer reasonable for the question being asked?
Download this free sample two-strategy word problem from Math Fundamentals, grade 2
Download this free sample multi-strategy word problem from Math Fundamentals, grade 4
Evan-Moor’s Math Fundamentals is a great resource for training students how to solve word problems in 3 simple steps. It provides step-by-step directions for solving questions and guides children with helpful visuals and key phrases.
Check out Daily Word Problems for consistent practice solving word problems.
For more fun math tips and strategies check out our Math- Ideas, Activities and Lessons Pinterest Board.
Save these tips and Pin It now!