How to Create Rigorous Math Word Problems
Usually, we focus on breaking down math word problems to make them more simple for students to solve. But in this blog post, I want to talk about doing the opposite. We know we want to help students dig deeper, think analytically and creatively, and learn multiple problem-solving strategies. One way to do this is by using rigorous math word problems with our 3rd, 4th, and 5th grade students.

Why Should We Use Challenging Math Word Problems?
Maybe you have a handful of students who solve math problems in a second and ask for more. Maybe you need more complex questions to match the rigor of your end-of-year tests. Or maybe you see that the types of problems your students have been solving aren’t making them think critically. There’s plenty of reasons why we want to use more rigorous math tasks.
But the idea isn’t to make word problems more difficult just for the sake of it. It’s not about adding lots of extra information to trick kids or using larger numbers that will take them a lot longer to compute. It’s about helping them develop the higher-order thinking skills involved in solving a problem.
So let’s dive into some easy ways to make math word problems more challenging in the upper elementary classroom!
1. Vary the language.
Try using lots of different words and phrases to frame the problem so students are exposed to a variety of questions. “How much does money does he have in all?” can be asked in a lot of ways. This is a strategy I like to use with daily word problems so students aren’t seeing the same thing over and over.
2. Change up what you’re asking for.
What information are you providing and what do students need to determine? For example, a multiplication word problem might require students to determine the product or one of the factors. For an elapsed time word problem, students might figure out the starting time, ending time, or how much time has passed.
3. Include charts, tables, graphs, and pictures.
Require students to use information from tables and other visuals to answer the problem. This is a great real life skill!
4. Make it a multistep word problem.
Two-step word problems are great practice for students and much more challenging to think through.
5. Go beyond the four operations.
Plenty of math content can be adapted for word problems. I like giving students word problems to practice rounding, measurement, elapsed time, geometry, and even probability! Moving past the traditional addition, subtraction, multiplication, and division word problems helps expose students to different types of thinking by looking at math in different situations.
6. Use open-ended questions.
Try using word problems that allow for multiple ways of solving and multiple correct answer possibilities.
7. Add patterns to the word problem.
This is a good one to encourage students to use manipulatives, models, and drawings to solve the problem. Instead of “Emma makes seven donuts each day; how many donuts would Emma have after one week?,” you could try “Emma made three donuts on Sunday. She doubled the amount the next day. If she keeps increasing the number of donuts she makes by the same amount, how many donuts will she have made after one week?”
8. Use real-world math problems!
Get kids thinking about how the word problem would play out in real life. This is especially true for division problems in which there is a remainder! What happens to it?
9. Try using an “If, then…” sentence frame.
Give them the solution and have them figure out what the rule could be (either open-ended or having one correct answer). “If Rory walked 64 feet around the perimeter of the baseball diamond, then the distance from the first base to the second base was ____.”
10. Start with the solution.
Give students the answer and have them come up with the question. This is a good way to encourage creative math thinking. You can also do a sorting activity where they match up answers to questions.
11. Write the question as a poem or riddle.
Having to read a poem for clues instead of reading a tradition 3-line story problem will really get them thinking!
12 . Have students create their own word problems.
There’s no better way to increase engagement than to have students make up word problems for each other to solve!
All of these strategies are great ways to add a little more rigor to basic 3rd, 4th, and 5th grade math word problems and help your students develop those critical thinking skills.
Got another trick up your sleeve? Let me know in the comments!
Word problems are a great way for students to identify real world applications for the information they are learning in the classroom — while helping them develop critical thinking skills. To write a word problem, analyze the way you would solve it yourself, and decide on the best method for your students to use.
-
Introduce students to the concept of a word problem within the context of what they are learning. Doing a group example will help them better understand the material.
Identify the math skills you would like students to work on. For example, if you want students to focus on adding and using images to help solve problems, your focus will be on writing a paragraph that accomplishes that goal.
Choose a main character for your word problem. Pick the number of objects and type of object that he has. The best objects are easy visuals for children to identify and draw, if needed. For example, your first sentence can be «George has 6 red balloons.»
Choose a secondary character and identify the number of objects he possesses. For example, «Alexa has 7 purple balloons.»
Write a question at the end to the word problem. An example of end questions is the question, «What is the total number of balloons?» Use math vocabulary that is age-appropriate for the students.
Add further instructions to help students achieve the result you desire. For the sample problem, you would want to tell students to draw the balloons to help them solve it. Also, encourage students to write the number sentence that goes along with the problem.
Read through your word problem and try it. If you are not clear in your instructions or you decide that the problem is too complicated, revise it to suit your students.
Ask students to write their own word problems as an assignment to turn in. This will allow you to see if they grasp the concept. Use these student-created examples as practice problems in the future.
Tips
Related Pages
More Math Word Problems
Algebra Word Problems
How to write algebra word problems into systems of linear equations and solve systems of linear equations
using elimination and substitution methods?
Grade 8 Algebra Word Problems
How to solve algebra word problems using systems of linear equations?
Example:
Devon is going to make 3 shelves for her father. He has a piece of lumber 12 feet long.
She wants the top shelf to be half a foot shorter than the middle shelf, and the bottom
shelf to be half a foot shorter than twice the length of the top shelf. How long will
each shelf be if she uses the entire 12 feet of wood?
- Show Video Lesson
Grade 8 Algebra Word Problems — Line Segments
Example:
If JK = 7x + 9, JL = 114 and KL = 9x + 9. Find KL.
- Show Video Lesson
Grade 8 number word problems — common core
How to write word problems into systems of linear equations and solve systems of linear equations
using elimination and substitution methods?
Example 1:
The sum of two numbers is 361 and the difference between the two numbers is 173. What are the
two numbers?
Example 2:
There are 356 Grade 8 students at Euclid’s Middle School. Thirty-four more than four times the
number of girls is equal to half the number of boys. How many boys are in Grade 8 at Euclid’s
Middle School? How many girls?
Example 3:
A family member has some five-dollar bills and one-dollar bills in their wallet. Altogether
she has 18 bills and a total of $62. How many of each bill does she have?
Example 1:
A friend bought 2 boxes of pencils and 8 notebooks for school and it cost him $11. He went back
to the store the same day to buy school supplies for his younger brother. He spent $11.25 on 3
boxes of pencils and 5 notebooks. How much would notebooks cost?
Exercises:
- A farm raises cows and chickens. The farmer has a total of 42 animals. One day he counts the
legs of all of his animals and realizes he has a total of 114. How many cows does the farmer
have? How many chickens? - The length of a rectangle is 4 times the width. The perimeter of the rectangle is 45 inches.
What is the area of the rectangle? - The sum of the measures of angles x and y is 127″. If the measure of angle x is 34° more
than half the measure of angle y, what is the measure of each angle?
- Show Video Lesson
Try the free Mathway calculator and
problem solver below to practice various math topics. Try the given examples, or type in your own
problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.

It’s pretty common for a math word problem worksheet to get pretty boring pretty fast.
Consider this question from a typical word problems worksheet:
Harry has 4 bags. Each bag has 3 balls in it. How many balls did Harry have all together?
The problem is, unless you’re a football coach or a fertility specialist, nobody cares about counting balls in bags! And who is Harry anyway?
Jokes aside, word problems are part of the common core for 3rd grade math, but all to often, they’re too abstract and detached for 3rd grade students to enjoy.
In this post I’m going to share some tips I found for making math word problems more engaging.
If you’ve got to teach a math lesson with task cards involving multiplication or division word problems – read on! you’re in the right place.
I’ve focused on making multiplication word problems for 3rd grade and 4th grade, but really these tips will be helpful for the design of any math worksheet or math activity.
Tip 1 – Use a Story/Theme
One of the easiest ways I’ve found to make word problems more interesting is to simply embed them within a more interesting narrative context.
If the math skills are embedded in a human context, it gives the problem much more immediacy and relevance.
I’ve been wanting to make a word problems section of Math Maze 2 for some time now, and one of the ideas I had was for the word problems to all be a part of the overall story world of the game. Ideally, each word problem wouldn’t exist in isolation, but would be a meaningful part of a larger story.
With this in mind, my first goal was to make a PowerPoint quiz with a stronger narrative focus and see how students and teachers found it.
Instead of random balls and boxes, I made each multiplication word problem fit within with the unifying theme of a fantasy village. Instead of solving totally disconnected math questions about balls and boxes, players get mini conversations with villagers who each need help with their specific set of challenges.
Like all my projects, this ended up blowing out and taking much longer than I thought it would to implement… Ideally, a more specific narrative could tie the lesson together and take it to the next level. I quickly found PowerPoint was not really designed for this kind of thing, and had to settle for a theme-based approach.
Tip 2 – Add graphics!
It’s a fairly small thing, but simply adding a character, background and speech bubble really makes the experience more engaging.
You can see here I’ve used the same characters and backgrounds multiple times, but it’s quite remarkable how much more engaging it is just with a background, clip art and speech buble.
I picked up the fantasy backgrounds from stock images, but there are also plenty of decent ones to be found for free on Pixabay.

Tip 3 – Make it challenging
A veteran math tutor (BravoMath) showed me how important it was to interleave math work in a way that forces students to think at every step. The key is to avoid a math lesson where mindless memorisation or rote multiplying procedures are rewarded:

“Especially bad are worksheets that say “Multiplication Word Problems” in which students just mindlessly skim the text for digits and then multiply. They never have to read it and recognize that there are -or are not- equal groups embedded in the story.”
– BravoMath, 2021
This really got me thinking and has changed the way I think about math instruction as a whole. I really like the emphasis on engaging students with a challenging problem, and designing the learning sequence in a way that encourages engagement instead of falling back on memorised formulas.
This was a tension I felt myself in high school where the temptation was simply to memorise a formula rather than understand why the formula worked in the first place.
BravoMath also shared some practical advice which is super helpful for transforming boring word problems.
“…make sure you mix up the knowns and unknowns. Traditionally, worksheets and textbooks have entire sections and sequences called “Multiplication”. Students then stop thinking because they know they have to multiply. The next section is called “Division” which instantly shuts off student brains because students know they just have to divide. We know from studies on interleaving that this is likely the worst possible sequence of content.
– BravoMath, 2021
The secret is to mix up the task so that students are always kept on their toes, and always forced to engage with the terms of this particular question.
…mix [questions] up like this, so students become fluent in being given any two of the following and finding the third:
• the number of sets
• the number of objects per set
• the total number of objects
e.g. 12 pencils per box, 3 boxes. How many pencils?
e.g. 12 pencils, 3 boxes. How many pencils per box?
e.g. 12 pencils, 3 pencils per box. How many boxes get pencils?
– BravoMath, 2021

I found this piece of advice to be incredibly helpful, and I could instantly see the difference it made to the word problems I was making.
Making word problems more challenging in this way took a bit of extra thinking and writing work, but ultimately I think it was well worth it. Mixing up the knowns and unknowns like this forces the player to constantly re-examine the numbers and their relationships to each other – no more mindless multiplication!
Tip 3 – Make it relevant
With BravoMath’s pedagogical advice and my theming idea combined, I set about creating some characters multiplication problem scenarios to go with each one.
I tried to make sure the characters were asking the player a multiplication or division problem that would believable.
Ok, Ok, these villagers do sometimes seem to have the mental math skill of a 2nd grade student, but it’s nice to feel needed right? ;P
You can see how mixing the multiplication equation like this transforms these simple multiplication word problems into something much more challenging and believable. It’s a much more robust way for kids to exercise their multiplication skills because they have to really think, and it also highlights how useful this kind of skill is in every day life.
Overall, I’m pretty happy with how these PowerPoint quizzes turned out! I think they are pretty unique and pack quite punch in terms of bang for buck.
I would have liked to have a stronger story and a reward system baked in, but in the end I decided that would be best achieved in Math Maze 2, and that I should stop trying to make PowerPoint do things it wasn’t designed to do ;P
They are one of the simplest products I’ve made so far, but I think they are going to be useful task card products in terms of usability and feedback (students are sent forward on a correct answer and backward on an incorrect answer) and that’s becoming increasingly important during these days of mixed/distance learning!
And safe to say, they’re much more interesting than the typical word problem worksheet! You can get an individual times table PowerPoint (and Google Slides) or get the lot in a bundle here.
So there you have it, some simple tips to make your next set of word problems more engaging for your 3rd grade students!
-Thanks for reading!
For Discussion:
Do you have any tips for making word problems more engaging?
We recommend reading any word problem at least three times. By the conclusion of the third reading, you should be magically whisked away to your home in Kansas.
Read it once…
…to get a general idea of what’s going on. Is the problem about money? Height and width? Distances? Money? Yeah, we already said money, but it’s important. Can you draw a picture in the margin that helps you visualize the problem?
Twice…
…to translate from English into math. Read the problem carefully this time, figuring out which pieces of information are important and which ones aren’t. Then ignore the unnecessary bits. This may sound a bit harsh, but they’re big boys. They’ll get over it.
Three times…
…a lady. Wait, that’s not it.
…to make sure you answered the right question. To be sure, read the question one last time before drawing a box around your final answer. Even if this method of double-checking saves you only once out of every 100 times, it’ll help you in the long run. One out of 100 is better than zero out of 100, but now we’re moving into some advanced mathematics.
Sample Problem
The local department store was having a sale. Holla! Gabe bought a pair of shoes for $21, although they would’ve been cheaper if he’d bought penny loafers. He also bought some shirts that were on sale for 25% off their normal retail price of $18 each. Gabe, always the bargain hunter, spent $75 total. How many shirts did he buy? Also, does he really think any of them will go with those shoes?
Read the problem:
Once…
…for a general idea of what’s going on: Gabe went shopping and spent money. Sounds like an old familiar story. Time for an intervention, friends of Gabe. Preferably before he maxes out his Diner’s Club card.
Now we need to figure out how many shirts he bought, so we read the problem:
Twice…
…to translate from English into math. Remember, we can translate a bit at a time, sort of like how Gabe pays for some of his major purchases when he has them on layaway. Sheesh, Gabe. Get a hold of yourself.
(total amount Gabe spent) = (amount he spent on shoes) + (amount he spent on shirts)
We know he spent $75 total, of which $21 was spent on shoes. This gives us the equation:
$75 = 21 + (amount he spent on shirts)
The problem has gotten smaller. It’s depressing when that happens with cake, but great when it happens to a word problem. Now all we need to do is come up with a symbolic expression for how much Gabe spent on shirts, or the cost per shirt times the number of shirts:
(amount he spent on shirts) = (cost per shirt)(number of shirts)
Since the shirts are 25% off their normal price of $18, they cost $18 – 0.25(18) = $13.50 each. What a deal, and real polyester, too!
We need to introduce a variable for the number of shirts; s will do the trick nicely.
(amount he spent on shirts) = 13.5s
When we plug this into the earlier equation, we find that:
75 = 21 + 13.5s
Finally, we’ve reduced this thing to a super-simple-looking equation! Good riddance, vestiges of language! Begone, nouns and verbs!
Things look much nicer now, right? No worrying about shirts or shoes or prices or Gabe’s uncontrollable shopping addiction. For the moment, we can forget about the word problem and solve the equation. The answer is s = 4, by the way. In case you were interested.
Three times…
…to make sure we’re answering the right question. We want to know how many shirts Gabe bought. Is that the answer we arrived at? We found that s = 4, and s was the number of shirts Gabe bought, so we’re all done. Four new shirts for Gabe, and three of them feature a Hawaiian pattern. Gabe, if you insist on buying far more clothes than you need, can’t you at least have a decent fashion sense?
When translating from English into math, some information can be ignored. We don’t care that «the local department store was having a sale.» Gabe might, but we certainly don’t. We care about statements that tell us numbers, and statements that tell us what the question is. Any extraneous information has been placed there simply as a decoy. We’re not going to fall for that. Wait a second…two for one? We’ll grab our jacket and meet you there.
Some people find it helpful to underline the important pieces of information in a word problem. You’re like an actor highlighting in a script the lines that are important for him to remember. Unlike an actor, however, you can always look back at the original problem if you draw a blank. Also, you don’t need to wear any stage makeup.
In the problem we just did, the important bits might look something like this:
The local department store was having a sale. Gabe bought a pair of shoes for $21 and some shirts that were on sale for 25% off their normal retail price of $18 each. If Gabe spent $75 total, how many shirts did he buy?
As you practice, you’ll become better at figuring out which parts of the word problem you can ignore and which parts are important.
SDI Productions / Getty Images
Updated on January 07, 2020
When you take a real-world situation and translate it into math, you are actually ‘expressing’ it; hence the mathematical term ‘expression’. Everything that is left of the equal sign is considered to be something you are expressing. Everything to the right of the equal sign (or inequality) is yet another expression. Simply stated, an expression is a combination of numbers, variables (letters) and operations. Expressions have a numerical value. Equations are sometimes confused with expressions. To keep these two terms separate, simply ask yourself if you can answer with a true/false. If so, you have an equation, not an expression that would have a numerical value. When simplifying equations, one often drops expressions such as 7-7 that equal 0.
A few samples:
| Word Expression | Algebraic Expression |
| x plus 5 10 times x y — 12 |
x 5 5x y — 12 |
Getting Started
Word problems consist of sentences. You will need to read the problem through carefully to ensure you have some understanding of what you are being asked to solve. Pay close attention to the problem to determine the key clues. Focus on the final question of the word problem. Read the problem again to make sure you understand what you’re being asked for. Then, jot down the expression.
Let’s get started:
1. On my last birthday, I weighed 125 pounds. One year later I have put on x pounds. Which expression gives my weight one year later?
a) x 125 b) 125 — x c) x 125 d) 125x
2. If you multiply the square of a number n by 6 and then added 3 to the product, the sum is equal to 57. One of the expressions equals 57, which one is it?
a) (6n)2 3 b) (n 3)2 c) 6(n2 3)d) 6n2 3
Answer for 1 is a) x 125
Answer for 2 is d) 6n2 3
Word Problems to Try
Sample 1
The price of a new radio is p dollars. The radio is on sale for 30% off. What expression will you write that will tell the savings that are being offered on the radio?
Answer: 0.p3
Sample 2
Your friend Doug has given you the following algebraic expression: «Subtract 15 times a number n from twice the square of the number. What is the expression that your friend is saying?
Answer: 2b2-15b
Sample 3
Jane and her three college friends are going to be sharing the cost of a 3 bedroom apartment. The cost of rent is n dollars. What expression can you write that will tell you what Jane’s share is?
Answer: n/5
Ultimately, becoming quite familiar with the use of algebraic expressions is an important skill for learning and conquering algebra.
Word problems are a special kind of math challenge. Not only do they require computation skills, but they also test students’ reading comprehension and problem-solving abilities. This can be the perfect storm of frustration for many kids. But it doesn’t have to be so hard! Today, I’m sharing easy strategies for helping students master the word problem!
Start With Reading Comprehension
The first step in conquering word problems goes right back to good ol’ reading comprehension. Kids have a bad habit of reading word problems like they are just passing through words to get to the numbers. Blah, blah, blah. 127! Blah, blah, blah, 52! They search for numbers and then rush to DO something with those numbers.
If our students are going to find success with word problems, they need to use their good reading strategies! Teach your students to:
Reread: Slow down and reread as many times as needed
Visualize: Imagine what is taking place in the problem. What is the order of events?
Ask questions: What do I know? What do I need to find out?
Make predictions: What would be a reasonable result? Do I expect the answer to be more or less than the numbers I’m given?
Teach a Problem-Solving Routine
Help students form effective thinking habits by teaching and practicing a problem-solving process. I’m not talking about a rote set of steps like CUBES (Circle the numbers, Underline the question, Box key words, etc.). I’m talking about a THINKING routine that helps the child learn the critical thinking skills involved in solving real problems.
I’ve found a simple PLAN, SOLVE, CHECK format works best. With young children, the biggest issue I find is that they simply skip (or struggle with) one of these steps, which causes a breakdown in their ability to solve the problem. In fact, I form my guided math groups for word problems around those three categories (planning strategies, solving strategies, checking strategies.) **You can grab a FREE Word Problem Thinking Mat in the Preview File of my Tiered Word Problems Pack.
Compare Problem Structures
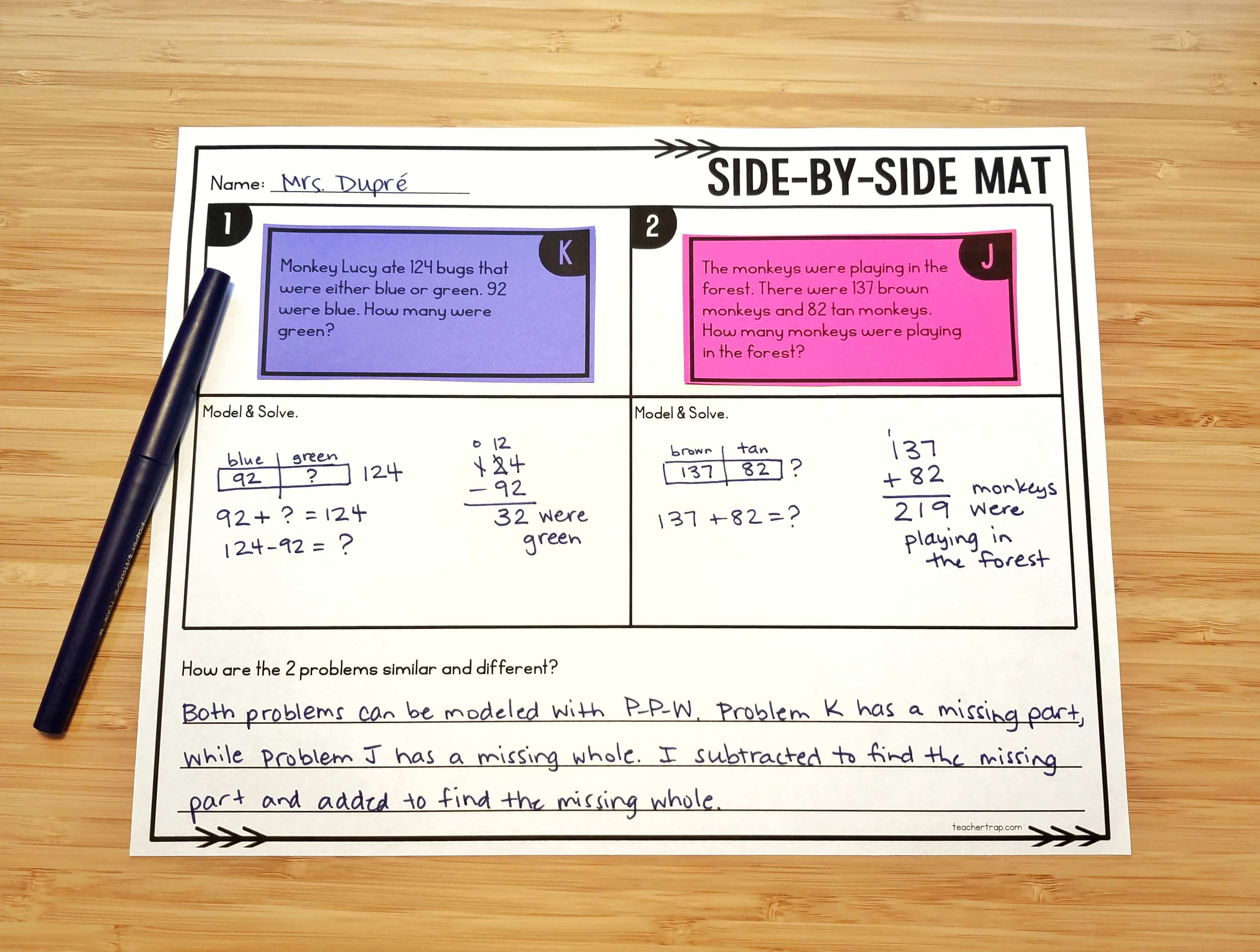
One of my favorite word problem teaching tools is something I call “Side-by-Sides.” This is simply where we solve two different problems and then compare the structure of the problem and the operation used.
When students model, solve, and discuss the different problem types, they grow a deeper understanding of how word problems work.
**You can grab FREE samples of side-by-side problems in the Preview File of my Side-by-Side Word Problems Pack or grab a FREE Side-by-Side Mat in the Preview File of my Tiered Word Problems Pack for 2nd Grade or 3rd Grade.
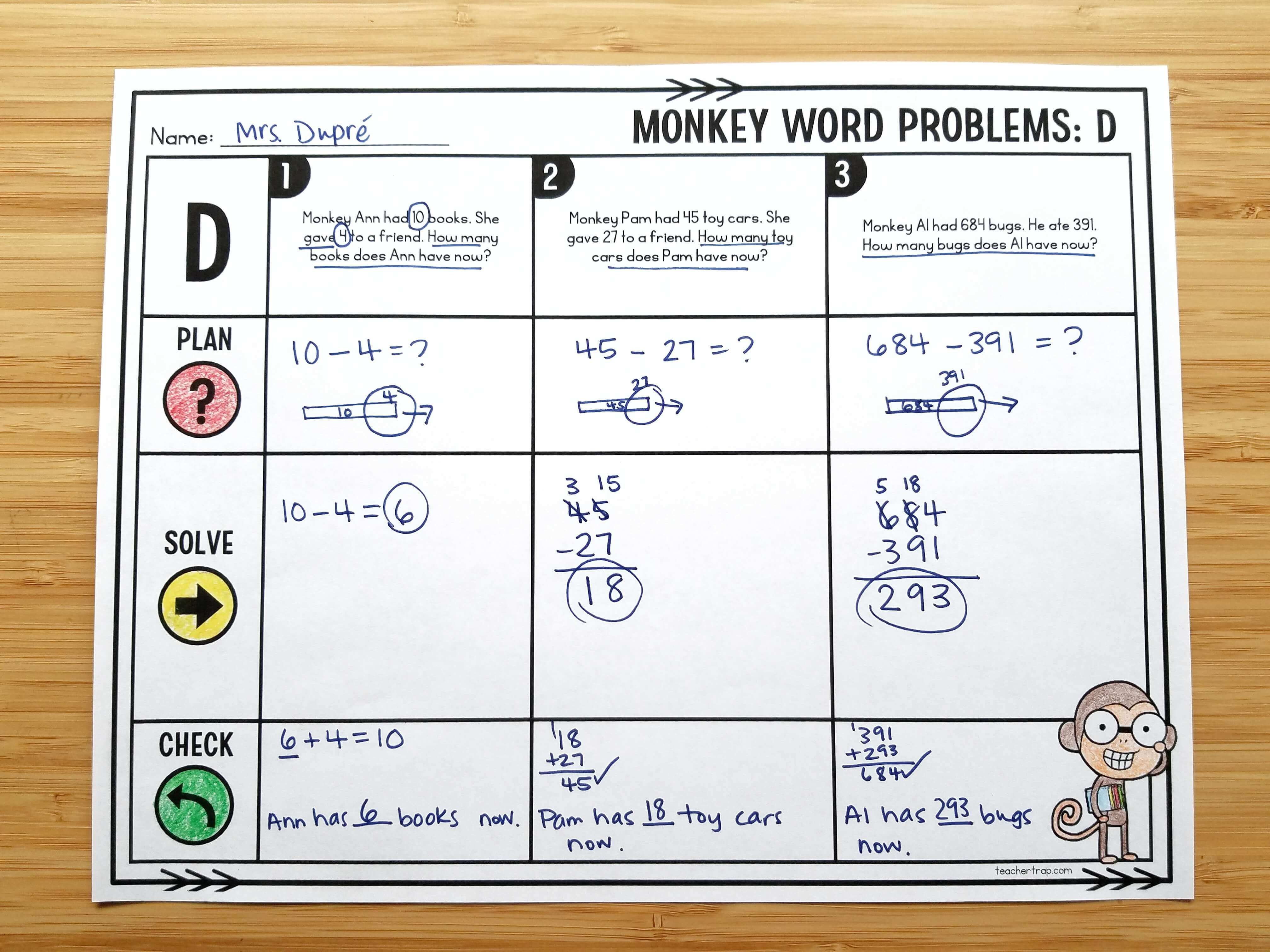
Start With the Easy Version
Differentiation isn’t just for reading! Many students get overwhelmed when they see large numbers in word problems, so why not meet them where they are? I use what I call “Tiered Word Problems” to help students understand word problem structures and grow confidence before tackling the on-level version.
In my Word Problem Solving Bundle, I provide all kinds of leveled word problems – Tiered Problem Pages, Task Cards, Exit Tickets, and more, that all increase in challenge from first through fifth grade. This allows me to scaffold instruction by student need, and throughout the school year.
**You can grab free goodies and sample pages from the Bundle in the Word Problem Solving Bundle Sampler Pack.
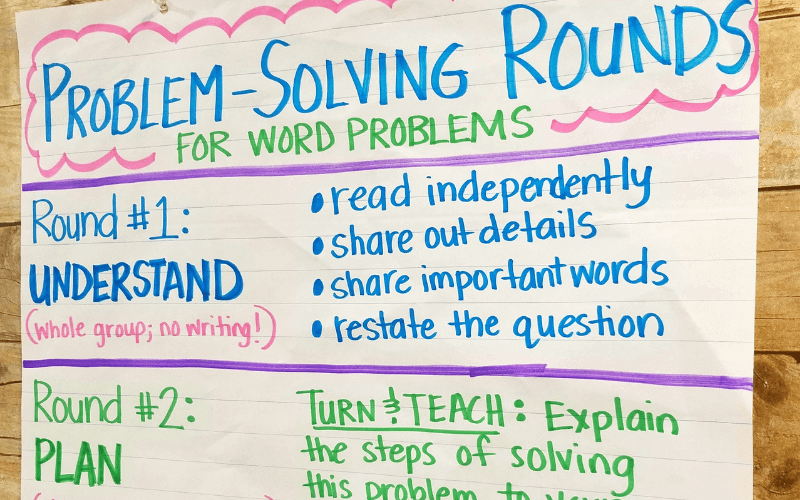
Use Problem-Solving Rounds
Problem-Solving Rounds changed math instruction in my classroom forever! This powerful routine encourages students to move through the problem-solving process I mentioned at the beginning of this post, to explain their thinking, and to reflect on other possible strategies and solutions.
Since using Problem-Solving Rounds, my students are more confident with word problems, have a deeper understanding of problem-types, and are better able to tackle new and challenging tasks.
The basic structure of the rounds follows the Problem-Solving Process I’ve shared. In Round 1, students read and discuss the problem with a focus on UNDERSTANDING. In Round 2, they turn to a partner and explain how they PLAN to solve the problem. They SOLVE the problem independently in Round 3, and in Round 4 they work with a partner and as a whole class to CHECK and reflect on their work. For a detailed guide to this process, check out my blog post all about Problem-Solving Rounds.
I’d love to hear from you! Comment below with your favorite word problem tips for teachers!