Repeal – To repeal something — usually a law, ordinance or public policy — is to take it back. For example, dog lovers might want the town council to repeal the law that says residents can have no more than four dogs.
The verb repeal comes from the Anglo-French word repeler, “to call back. ” Repeal is almost always used in the context of law: When a government decides to get rid of an ordinance or law, that ordinance or law is repealed. That means it is no longer in effect, like if the weather becomes unseasonably hot, the schools might repeal the part of the dress code to permit students to wear shorts.
Clarification in the spirit of helpful support…..
[FAQ]
What is an example of a law in science?
An example of a scientific law is Newton’s Aecond Law of Motion which states that acceleration (a) happens when a force (F) acts on an object’s mass (m). The equation for this law is F = ma.
What is a law in science kid definition?
A scientific law describes the relationship between two or more things we can observe in nature under certain conditions. Scientists like Newton and Kepler discovered many laws to do with gravity and motion that are very predictable with mathematical formulas. A law is different from a theory because it doesn’t change.
How is law used in science?
Scientific laws summarize the results of experiments or observations, usually within a certain range of application. … Laws differ from hypotheses and postulates, which are proposed during the scientific process before and during validation by experiment and observation.
How many laws are there in science?
What are the five scientific laws? The five most popular scientific laws are Hooke’s Law of Elasticity, Archimedes’ Principle of Buoyancy, Dalton’s Law of Partial Pressures, Bernoulli’s Law of Fluid Dynamics and Fourier’s Law of Heat Conduction.
Is a scientific law a fact?
Both scientific laws and theories are considered scientific fact. However, theories and laws can be disproven when new evidence emerges. Certain accepted truths of Newtonian physics were partially disproven by Albert Einstein’s theory of relativity.
Scientific laws or laws of science are statements, based on repeated experiments or observations, that describe or predict a range of natural phenomena.[1] The term law has diverse usage in many cases (approximate, accurate, broad, or narrow) across all fields of natural science (physics, chemistry, astronomy, geoscience, biology). Laws are developed from data and can be further developed through mathematics; in all cases they are directly or indirectly based on empirical evidence. It is generally understood that they implicitly reflect, though they do not explicitly assert, causal relationships fundamental to reality, and are discovered rather than invented.[2]
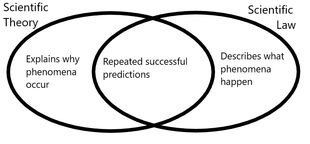
Scientific theories explain why something happens, whereas scientific law describes what happens.
Scientific laws summarize the results of experiments or observations, usually within a certain range of application. In general, the accuracy of a law does not change when a new theory of the relevant phenomenon is worked out, but rather the scope of the law’s application, since the mathematics or statement representing the law does not change. As with other kinds of scientific knowledge, scientific laws do not express absolute certainty, as mathematical theorems or identities do. A scientific law may be contradicted, restricted, or extended by future observations.
A law can often be formulated as one or several statements or equations, so that it can predict the outcome of an experiment. Laws differ from hypotheses and postulates, which are proposed during the scientific process before and during validation by experiment and observation. Hypotheses and postulates are not laws, since they have not been verified to the same degree, although they may lead to the formulation of laws. Laws are narrower in scope than scientific theories, which may entail one or several laws.[3] Science distinguishes a law or theory from facts.[4] Calling a law a fact is ambiguous, an overstatement, or an equivocation.[5] The nature of scientific laws has been much discussed in philosophy, but in essence scientific laws are simply empirical conclusions reached by scientific method; they are intended to be neither laden with ontological commitments nor statements of logical absolutes.
OverviewEdit
A scientific law always applies to a physical system under repeated conditions, and it implies that there is a causal relationship involving the elements of the system. Factual and well-confirmed statements like «Mercury is liquid at standard temperature and pressure» are considered too specific to qualify as scientific laws. A central problem in the philosophy of science, going back to David Hume, is that of distinguishing causal relationships (such as those implied by laws) from principles that arise due to constant conjunction.[6]
Laws differ from scientific theories in that they do not posit a mechanism or explanation of phenomena: they are merely distillations of the results of repeated observation. As such, the applicability of a law is limited to circumstances resembling those already observed, and the law may be found to be false when extrapolated. Ohm’s law only applies to linear networks; Newton’s law of universal gravitation only applies in weak gravitational fields; the early laws of aerodynamics, such as Bernoulli’s principle, do not apply in the case of compressible flow such as occurs in transonic and supersonic flight; Hooke’s law only applies to strain below the elastic limit; Boyle’s law applies with perfect accuracy only to the ideal gas, etc. These laws remain useful, but only under the specified conditions where they apply.
Many laws take mathematical forms, and thus can be stated as an equation; for example, the law of conservation of energy can be written as , where is the total amount of energy in the universe. Similarly, the first law of thermodynamics can be written as , and Newton’s second law can be written as dp⁄dt. While these scientific laws explain what our senses perceive, they are still empirical (acquired by observation or scientific experiment) and so are not like mathematical theorems which can be proved purely by mathematics.
Like theories and hypotheses, laws make predictions; specifically, they predict that new observations will conform to the given law. Laws can be falsified if they are found in contradiction with new data.
Some laws are only approximations of other more general laws, and are good approximations with a restricted domain of applicability. For example, Newtonian dynamics (which is based on Galilean transformations) is the low-speed limit of special relativity (since the Galilean transformation is the low-speed approximation to the Lorentz transformation). Similarly, the Newtonian gravitation law is a low-mass approximation of general relativity, and Coulomb’s law is an approximation to quantum electrodynamics at large distances (compared to the range of weak interactions). In such cases it is common to use the simpler, approximate versions of the laws, instead of the more accurate general laws.
Laws are constantly being tested experimentally to increasing degrees of precision, which is one of the main goals of science. The fact that laws have never been observed to be violated does not preclude testing them at increased accuracy or in new kinds of conditions to confirm whether they continue to hold, or whether they break, and what can be discovered in the process. It is always possible for laws to be invalidated or proven to have limitations, by repeatable experimental evidence, should any be observed. Well-established laws have indeed been invalidated in some special cases, but the new formulations created to explain the discrepancies generalize upon, rather than overthrow, the originals. That is, the invalidated laws have been found to be only close approximations, to which other terms or factors must be added to cover previously unaccounted-for conditions, e.g. very large or very small scales of time or space, enormous speeds or masses, etc. Thus, rather than unchanging knowledge, physical laws are better viewed as a series of improving and more precise generalizations.
PropertiesEdit
Scientific laws are typically conclusions based on repeated scientific experiments and observations over many years and which have become accepted universally within the scientific community. A scientific law is «inferred from particular facts, applicable to a defined group or class of phenomena, and expressible by the statement that a particular phenomenon always occurs if certain conditions be present.»[7] The production of a summary description of our environment in the form of such laws is a fundamental aim of science.
Several general properties of scientific laws, particularly when referring to laws in physics, have been identified. Scientific laws are:
- True, at least within their regime of validity. By definition, there have never been repeatable contradicting observations.
- Universal. They appear to apply everywhere in the universe.[8]: 82
- Simple. They are typically expressed in terms of a single mathematical equation.
- Absolute. Nothing in the universe appears to affect them.[8]: 82
- Stable. Unchanged since first discovered (although they may have been shown to be approximations of more accurate laws),
- All-encompassing. Everything in the universe apparently must comply with them (according to observations).
- Generally conservative of quantity.[9]: 59
- Often expressions of existing homogeneities (symmetries) of space and time.[9]
- Typically theoretically reversible in time (if non-quantum), although time itself is irreversible.[9]
- Broad. In physics, laws exclusively refer to the broad domain of matter, motion, energy, and force itself, rather than more specific systems in the universe, such as living systems, i.e. the mechanics of the human body.[10]
The term «scientific law» is traditionally associated with the natural sciences, though the social sciences also contain laws.[11] For example, Zipf’s law is a law in the social sciences which is based on mathematical statistics. In these cases, laws may describe general trends or expected behaviors rather than being absolutes.
In natural science, impossibility assertions come to be widely accepted as overwhelmingly probable rather than considered proved to the point of being unchallengeable. The basis for this strong acceptance is a combination of extensive evidence of something not occurring, combined with an underlying theory, very successful in making predictions, whose assumptions lead logically to the conclusion that something is impossible. While an impossibility assertion in natural science can never be absolutely proved, it could be refuted by the observation of a single counterexample. Such a counterexample would require that the assumptions underlying the theory that implied the impossibility be re-examined.
Some examples of widely accepted impossibilities in physics are perpetual motion machines, which violate the law of conservation of energy, exceeding the speed of light, which violates the implications of special relativity, the uncertainty principle of quantum mechanics, which asserts the impossibility of simultaneously knowing both the position and the momentum of a particle, and Bell’s theorem: no physical theory of local hidden variables can ever reproduce all of the predictions of quantum mechanics.
Laws as consequences of mathematical symmetriesEdit
Some laws reflect mathematical symmetries found in Nature (e.g. the Pauli exclusion principle reflects identity of electrons, conservation laws reflect homogeneity of space, time, and Lorentz transformations reflect rotational symmetry of spacetime). Many fundamental physical laws are mathematical consequences of various symmetries of space, time, or other aspects of nature. Specifically, Noether’s theorem connects some conservation laws to certain symmetries. For example, conservation of energy is a consequence of the shift symmetry of time (no moment of time is different from any other), while conservation of momentum is a consequence of the symmetry (homogeneity) of space (no place in space is special, or different than any other). The indistinguishability of all particles of each fundamental type (say, electrons, or photons) results in the Dirac and Bose quantum statistics which in turn result in the Pauli exclusion principle for fermions and in Bose–Einstein condensation for bosons. The rotational symmetry between time and space coordinate axes (when one is taken as imaginary, another as real) results in Lorentz transformations which in turn result in special relativity theory. Symmetry between inertial and gravitational mass results in general relativity.
The inverse square law of interactions mediated by massless bosons is the mathematical consequence of the 3-dimensionality of space.
One strategy in the search for the most fundamental laws of nature is to search for the most general mathematical symmetry group that can be applied to the fundamental interactions.
Laws of physicsEdit
Conservation lawsEdit
Conservation and symmetryEdit
Conservation laws are fundamental laws that follow from the homogeneity of space, time and phase, in other words symmetry.
- Noether’s theorem: Any quantity with a continuously differentiable symmetry in the action has an associated conservation law.
- Conservation of mass was the first law to be understood since most macroscopic physical processes involving masses, for example, collisions of massive particles or fluid flow, provide the apparent belief that mass is conserved. Mass conservation was observed to be true for all chemical reactions. In general, this is only approximative because with the advent of relativity and experiments in nuclear and particle physics: mass can be transformed into energy and vice versa, so mass is not always conserved but part of the more general conservation of mass-energy.
- Conservation of energy, momentum and angular momentum for isolated systems can be found to be symmetries in time, translation, and rotation.
- Conservation of charge was also realized since charge has never been observed to be created or destroyed and only found to move from place to place.
Continuity and transferEdit
Conservation laws can be expressed using the general continuity equation (for a conserved quantity) can be written in differential form as:
where ρ is some quantity per unit volume, J is the flux of that quantity (change in quantity per unit time per unit area). Intuitively, the divergence (denoted ∇•) of a vector field is a measure of flux diverging radially outwards from a point, so the negative is the amount piling up at a point; hence the rate of change of density in a region of space must be the amount of flux leaving or collecting in some region (see the main article for details). In the table below, the fluxes flows for various physical quantities in transport, and their associated continuity equations, are collected for comparison.
-
Physics, conserved quantity Conserved quantity q Volume density ρ (of q) Flux J (of q) Equation Hydrodynamics, fluids m = mass (kg) ρ = volume mass density (kg m−3) ρ u, where u = velocity field of fluid (m s−1)
Electromagnetism, electric charge q = electric charge (C) ρ = volume electric charge density (C m−3) J = electric current density (A m−2) Thermodynamics, energy E = energy (J) u = volume energy density (J m−3) q = heat flux (W m−2) Quantum mechanics, probability P = (r, t) = ∫|Ψ|2d3r = probability distribution ρ = ρ(r, t) = |Ψ|2 = probability density function (m−3), Ψ = wavefunction of quantum system
j = probability current/flux
More general equations are the convection–diffusion equation and Boltzmann transport equation, which have their roots in the continuity equation.
Laws of classical mechanicsEdit
Principle of least actionEdit
Classical mechanics, including Newton’s laws, Lagrange’s equations, Hamilton’s equations, etc., can be derived from the following principle:
where is the action; the integral of the Lagrangian
of the physical system between two times t1 and t2. The kinetic energy of the system is T (a function of the rate of change of the configuration of the system), and potential energy is V (a function of the configuration and its rate of change). The configuration of a system which has N degrees of freedom is defined by generalized coordinates q = (q1, q2, … qN).
There are generalized momenta conjugate to these coordinates, p = (p1, p2, …, pN), where:
The action and Lagrangian both contain the dynamics of the system for all times. The term «path» simply refers to a curve traced out by the system in terms of the generalized coordinates in the configuration space, i.e. the curve q(t), parameterized by time (see also parametric equation for this concept).
The action is a functional rather than a function, since it depends on the Lagrangian, and the Lagrangian depends on the path q(t), so the action depends on the entire «shape» of the path for all times (in the time interval from t1 to t2). Between two instants of time, there are infinitely many paths, but one for which the action is stationary (to the first order) is the true path. The stationary value for the entire continuum of Lagrangian values corresponding to some path, not just one value of the Lagrangian, is required (in other words it is not as simple as «differentiating a function and setting it to zero, then solving the equations to find the points of maxima and minima etc», rather this idea is applied to the entire «shape» of the function, see calculus of variations for more details on this procedure).[12]
Notice L is not the total energy E of the system due to the difference, rather than the sum:
The following[13][14] general approaches to classical mechanics are summarized below in the order of establishment. They are equivalent formulations. Newton’s is commonly used due to simplicity, but Hamilton’s and Lagrange’s equations are more general, and their range can extend into other branches of physics with suitable modifications.
-
Laws of motion Principle of least action: The Euler–Lagrange equations are: Using the definition of generalized momentum, there is the symmetry:
Hamilton’s equations The Hamiltonian as a function of generalized coordinates and momenta has the general form:
Hamilton–Jacobi equation Newton’s laws Newton’s laws of motion
They are low-limit solutions to relativity. Alternative formulations of Newtonian mechanics are Lagrangian and Hamiltonian mechanics.
The laws can be summarized by two equations (since the 1st is a special case of the 2nd, zero resultant acceleration):
where p = momentum of body, Fij = force on body i by body j, Fji = force on body j by body i.
For a dynamical system the two equations (effectively) combine into one:
in which FE = resultant external force (due to any agent not part of system). Body i does not exert a force on itself.
From the above, any equation of motion in classical mechanics can be derived.
- Corollaries in mechanics
- Euler’s laws of motion
- Euler’s equations (rigid body dynamics)
- Corollaries in fluid mechanics
Equations describing fluid flow in various situations can be derived, using the above classical equations of motion and often conservation of mass, energy and momentum. Some elementary examples follow.
- Archimedes’ principle
- Bernoulli’s principle
- Poiseuille’s law
- Stokes’s law
- Navier–Stokes equations
- Faxén’s law
Laws of gravitation and relativityEdit
Some of the more famous laws of nature are found in Isaac Newton’s theories of (now) classical mechanics, presented in his Philosophiae Naturalis Principia Mathematica, and in Albert Einstein’s theory of relativity.
Modern lawsEdit
- Special relativity
The two postulates of special relativity are not «laws» in themselves, but assumptions of their nature in terms of relative motion.
They can be stated as «the laws of physics are the same in all inertial frames» and «the speed of light is constant and has the same value in all inertial frames».
The said postulates lead to the Lorentz transformations – the transformation law between two frame of references moving relative to each other. For any 4-vector
this replaces the Galilean transformation law from classical mechanics. The Lorentz transformations reduce to the Galilean transformations for low velocities much less than the speed of light c.
The magnitudes of 4-vectors are invariants — not «conserved», but the same for all inertial frames (i.e. every observer in an inertial frame will agree on the same value), in particular if A is the four-momentum, the magnitude can derive the famous invariant equation for mass-energy and momentum conservation (see invariant mass):
in which the (more famous) mass-energy equivalence E = mc2 is a special case.
- General relativity
General relativity is governed by the Einstein field equations, which describe the curvature of space-time due to mass-energy equivalent to the gravitational field. Solving the equation for the geometry of space warped due to the mass distribution gives the metric tensor. Using the geodesic equation, the motion of masses falling along the geodesics can be calculated.
- Gravitomagnetism
In a relatively flat spacetime due to weak gravitational fields, gravitational analogues of Maxwell’s equations can be found; the GEM equations, to describe an analogous gravitomagnetic field. They are well established by the theory, and experimental tests form ongoing research.[15]
Classical lawsEdit
Kepler’s Laws, though originally discovered from planetary observations (also due to Tycho Brahe), are true for any central forces.[16]
-
Newton’s law of universal gravitation: For two point masses:
For a non uniform mass distribution of local mass density ρ (r) of body of Volume V, this becomes:
Gauss’ law for gravity: An equivalent statement to Newton’s law is:
Kepler’s 1st Law: Planets move in an ellipse, with the star at a focus where
is the eccentricity of the elliptic orbit, of semi-major axis a and semi-minor axis b, and l is the semi-latus rectum. This equation in itself is nothing physically fundamental; simply the polar equation of an ellipse in which the pole (origin of polar coordinate system) is positioned at a focus of the ellipse, where the orbited star is.
Kepler’s 2nd Law: equal areas are swept out in equal times (area bounded by two radial distances and the orbital circumference): where L is the orbital angular momentum of the particle (i.e. planet) of mass m about the focus of orbit,
Kepler’s 3rd Law: The square of the orbital time period T is proportional to the cube of the semi-major axis a: where M is the mass of the central body (i.e. star).
ThermodynamicsEdit
-
Laws of thermodynamics First law of thermodynamics: The change in internal energy dU in a closed system is accounted for entirely by the heat δQ absorbed by the system and the work δW done by the system: Second law of thermodynamics: There are many statements of this law, perhaps the simplest is «the entropy of isolated systems never decreases»,
meaning reversible changes have zero entropy change, irreversible process are positive, and impossible process are negative.
Zeroth law of thermodynamics: If two systems are in thermal equilibrium with a third system, then they are in thermal equilibrium with one another. Third law of thermodynamics:
- As the temperature T of a system approaches absolute zero, the entropy S approaches a minimum value C: as T → 0, S → C.
For homogeneous systems the first and second law can be combined into the Fundamental thermodynamic relation: Onsager reciprocal relations: sometimes called the Fourth Law of Thermodynamics - ;
- .
- Newton’s law of cooling
- Fourier’s law
- Ideal gas law, combines a number of separately developed gas laws;
- Boyle’s law
- Charles’s law
- Gay-Lussac’s law
- Avogadro’s law, into one
- now improved by other equations of state
- Dalton’s law (of partial pressures)
- Boltzmann equation
- Carnot’s theorem
- Kopp’s law
ElectromagnetismEdit
Maxwell’s equations give the time-evolution of the electric and magnetic fields due to electric charge and current distributions. Given the fields, the Lorentz force law is the equation of motion for charges in the fields.
These equations can be modified to include magnetic monopoles, and are consistent with our observations of monopoles either existing or not existing; if they do not exist, the generalized equations reduce to the ones above, if they do, the equations become fully symmetric in electric and magnetic charges and currents. Indeed, there is a duality transformation where electric and magnetic charges can be «rotated into one another», and still satisfy Maxwell’s equations.
- Pre-Maxwell laws
These laws were found before the formulation of Maxwell’s equations. They are not fundamental, since they can be derived from Maxwell’s Equations. Coulomb’s Law can be found from Gauss’ Law (electrostatic form) and the Biot–Savart Law can be deduced from Ampere’s Law (magnetostatic form). Lenz’ Law and Faraday’s Law can be incorporated into the Maxwell-Faraday equation. Nonetheless they are still very effective for simple calculations.
- Lenz’s law
- Coulomb’s law
- Biot–Savart law
- Other laws
- Ohm’s law
- Kirchhoff’s laws
- Joule’s law
PhotonicsEdit
Classically, optics is based on a variational principle: light travels from one point in space to another in the shortest time.
- Fermat’s principle
In geometric optics laws are based on approximations in Euclidean geometry (such as the paraxial approximation).
- Law of reflection
- Law of refraction, Snell’s law
In physical optics, laws are based on physical properties of materials.
- Brewster’s angle
- Malus’s law
- Beer–Lambert law
In actuality, optical properties of matter are significantly more complex and require quantum mechanics.
Laws of quantum mechanicsEdit
Quantum mechanics has its roots in postulates. This leads to results which are not usually called «laws», but hold the same status, in that all of quantum mechanics follows from them.
One postulate that a particle (or a system of many particles) is described by a wavefunction, and this satisfies a quantum wave equation: namely the Schrödinger equation (which can be written as a non-relativistic wave equation, or a relativistic wave equation). Solving this wave equation predicts the time-evolution of the system’s behaviour, analogous to solving Newton’s laws in classical mechanics.
Other postulates change the idea of physical observables; using quantum operators; some measurements can’t be made at the same instant of time (Uncertainty principles), particles are fundamentally indistinguishable. Another postulate; the wavefunction collapse postulate, counters the usual idea of a measurement in science.
-
Quantum mechanics, Quantum field theory Schrödinger equation (general form): Describes the time dependence of a quantum mechanical system.
The Hamiltonian (in quantum mechanics) H is a self-adjoint operator acting on the state space, (see Dirac notation) is the instantaneous quantum state vector at time t, position r, i is the unit imaginary number, ħ = h/2π is the reduced Planck’s constant.
Wave–particle duality Planck–Einstein law: the energy of photons is proportional to the frequency of the light (the constant is Planck’s constant, h).
De Broglie wavelength: this laid the foundations of wave–particle duality, and was the key concept in the Schrödinger equation,
Heisenberg uncertainty principle: Uncertainty in position multiplied by uncertainty in momentum is at least half of the reduced Planck constant, similarly for time and energy;
The uncertainty principle can be generalized to any pair of observables — see main article.
Wave mechanics Schrödinger equation (original form):
Pauli exclusion principle: No two identical fermions can occupy the same quantum state (bosons can). Mathematically, if two particles are interchanged, fermionic wavefunctions are anti-symmetric, while bosonic wavefunctions are symmetric: where ri is the position of particle i, and s is the spin of the particle. There is no way to keep track of particles physically, labels are only used mathematically to prevent confusion.
Radiation lawsEdit
Applying electromagnetism, thermodynamics, and quantum mechanics, to atoms and molecules, some laws of electromagnetic radiation and light are as follows.
- Stefan–Boltzmann law
- Planck’s law of black-body radiation
- Wien’s displacement law
- Radioactive decay law
Laws of chemistryEdit
Chemical laws are those laws of nature relevant to chemistry. Historically, observations led to many empirical laws, though now it is known that chemistry has its foundations in quantum mechanics.
- Quantitative analysis
The most fundamental concept in chemistry is the law of conservation of mass, which states that there is no detectable change in the quantity of matter during an ordinary chemical reaction. Modern physics shows that it is actually energy that is conserved, and that energy and mass are related; a concept which becomes important in nuclear chemistry. Conservation of energy leads to the important concepts of equilibrium, thermodynamics, and kinetics.
Additional laws of chemistry elaborate on the law of conservation of mass. Joseph Proust’s law of definite composition says that pure chemicals are composed of elements in a definite formulation; we now know that the structural arrangement of these elements is also important.
Dalton’s law of multiple proportions says that these chemicals will present themselves in proportions that are small whole numbers; although in many systems (notably biomacromolecules and minerals) the ratios tend to require large numbers, and are frequently represented as a fraction.
The law of definite composition and the law of multiple proportions are the first two of the three laws of stoichiometry, the proportions by which the chemical elements combine to form chemical compounds. The third law of stoichiometry is the law of reciprocal proportions, which provides the basis for establishing equivalent weights for each chemical element. Elemental equivalent weights can then be used to derive atomic weights for each element.
More modern laws of chemistry define the relationship between energy and its transformations.
- Reaction kinetics and equilibria
- In equilibrium, molecules exist in mixture defined by the transformations possible on the timescale of the equilibrium, and are in a ratio defined by the intrinsic energy of the molecules—the lower the intrinsic energy, the more abundant the molecule. Le Chatelier’s principle states that the system opposes changes in conditions from equilibrium states, i.e. there is an opposition to change the state of an equilibrium reaction.
- Transforming one structure to another requires the input of energy to cross an energy barrier; this can come from the intrinsic energy of the molecules themselves, or from an external source which will generally accelerate transformations. The higher the energy barrier, the slower the transformation occurs.
- There is a hypothetical intermediate, or transition structure, that corresponds to the structure at the top of the energy barrier. The Hammond–Leffler postulate states that this structure looks most similar to the product or starting material which has intrinsic energy closest to that of the energy barrier. Stabilizing this hypothetical intermediate through chemical interaction is one way to achieve catalysis.
- All chemical processes are reversible (law of microscopic reversibility) although some processes have such an energy bias, they are essentially irreversible.
- The reaction rate has the mathematical parameter known as the rate constant. The Arrhenius equation gives the temperature and activation energy dependence of the rate constant, an empirical law.
- Thermochemistry
- Dulong–Petit law
- Gibbs–Helmholtz equation
- Hess’s law
- Gas laws
- Raoult’s law
- Henry’s law
- Chemical transport
- Fick’s laws of diffusion
- Graham’s law
- Lamm equation
Laws of biologyEdit
EcologyEdit
- Competitive exclusion principle or Gause’s law
GeneticsEdit
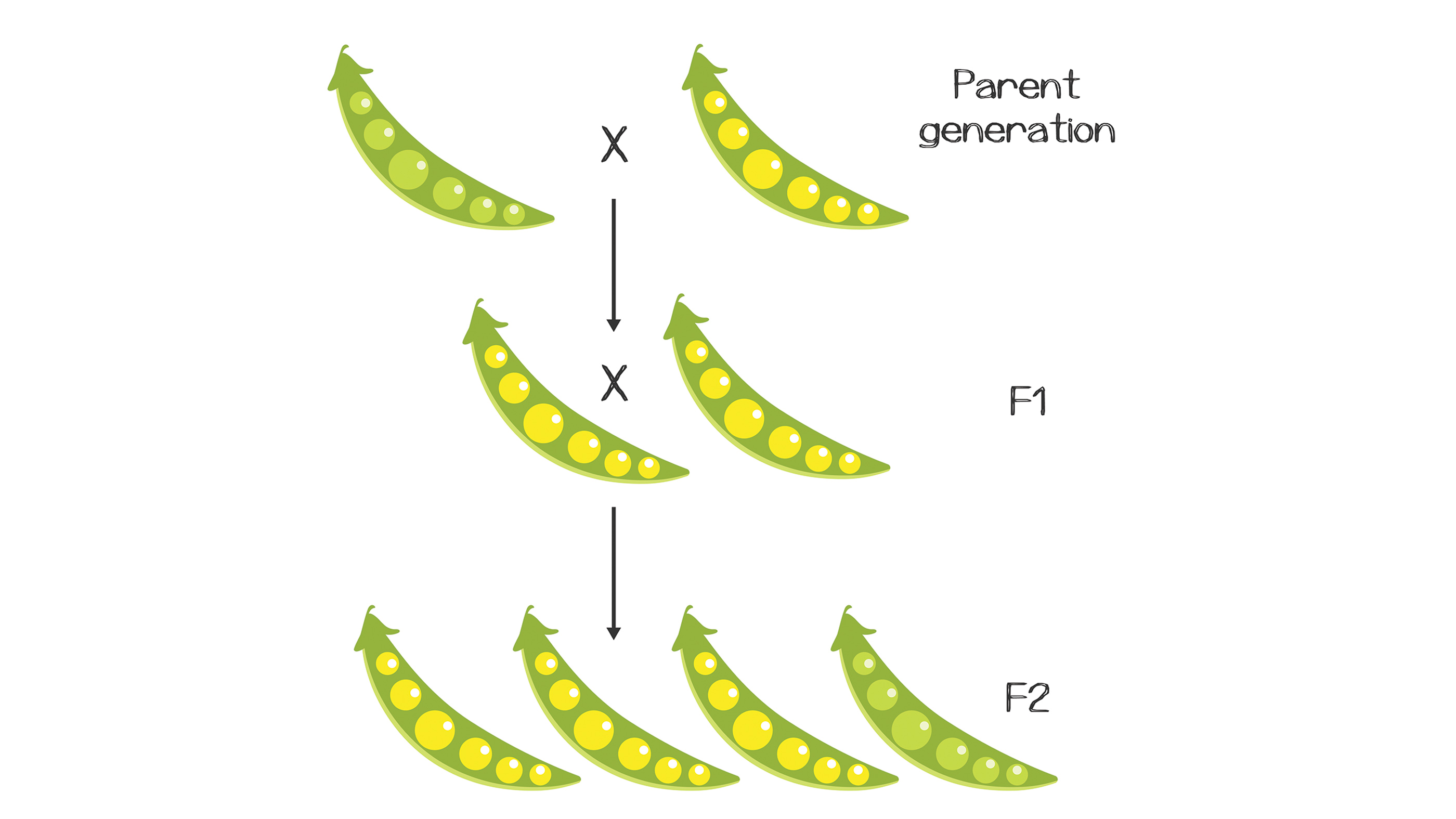
- Mendelian laws (Dominance and Uniformity, segregation of genes, and Independent Assortment)
- Hardy–Weinberg principle
Natural selectionEdit
Whether or not Natural Selection is a “law of nature” is controversial among biologists.[17][18] Henry Byerly, an American philosopher known for his work on evolutionary theory, discussed the problem of interpreting a principle of natural selection as a law. He suggested a formulation of natural selection as a framework principle that can contribute to a better understanding of evolutionary theory.[18] His approach was to express relative fitness, the propensity of a genotype to increase in proportionate representation in a competitive environment, as a function of adaptedness (adaptive design) of the organism.
Laws of Earth SciencesEdit
GeographyEdit
- Arbia’s law of geography
- Tobler’s first law of geography
- Tobler’s second law of geography
GeologyEdit
- Archie’s law
- Buys-Ballot’s law
- Birch’s law
- Byerlee’s law
- Principle of original horizontality
- Law of superposition
- Principle of lateral continuity
- Principle of cross-cutting relationships
- Principle of faunal succession
- Principle of inclusions and components
- Walther’s law
Other fieldsEdit
Some mathematical theorems and axioms are referred to as laws because they provide logical foundation to empirical laws.
Examples of other observed phenomena sometimes described as laws include the Titius–Bode law of planetary positions, Zipf’s law of linguistics, and Moore’s law of technological growth. Many of these laws fall within the scope of uncomfortable science. Other laws are pragmatic and observational, such as the law of unintended consequences. By analogy, principles in other fields of study are sometimes loosely referred to as «laws». These include Occam’s razor as a principle of philosophy and the Pareto principle of economics.
HistoryEdit
The observation and detection of underlying regularities in nature date from prehistoric times — the recognition of cause-and-effect relationships implicitly recognises the existence of laws of nature. The recognition of such regularities as independent scientific laws per se, though, was limited by their entanglement in animism, and by the attribution of many effects that do not have readily obvious causes—such as physical phenomena—to the actions of gods, spirits, supernatural beings, etc. Observation and speculation about nature were intimately bound up with metaphysics and morality.
In Europe, systematic theorizing about nature (physis) began with the early Greek philosophers and scientists and continued into the Hellenistic and Roman imperial periods, during which times the intellectual influence of Roman law increasingly became paramount.
The formula «law of nature» first appears as «a live metaphor» favored by Latin poets Lucretius, Virgil, Ovid, Manilius, in time gaining a firm theoretical presence in the prose treatises of Seneca and Pliny. Why this Roman origin? According to [historian and classicist Daryn] Lehoux’s persuasive narrative,[19] the idea was made possible by the pivotal role of codified law and forensic argument in Roman life and culture.
For the Romans . . . the place par excellence where ethics, law, nature, religion and politics overlap is the law court. When we read Seneca’s Natural Questions, and watch again and again just how he applies standards of evidence, witness evaluation, argument and proof, we can recognize that we are reading one of the great Roman rhetoricians of the age, thoroughly immersed in forensic method. And not Seneca alone. Legal models of scientific judgment turn up all over the place, and for example prove equally integral to Ptolemy’s approach to verification, where the mind is assigned the role of magistrate, the senses that of disclosure of evidence, and dialectical reason that of the law itself.[20]
The precise formulation of what are now recognized as modern and valid statements of the laws of nature dates from the 17th century in Europe, with the beginning of accurate experimentation and the development of advanced forms of mathematics. During this period, natural philosophers such as Isaac Newton (1642-1727) were influenced by a religious view — stemming from medieval concepts of divine law — which held that God had instituted absolute, universal and immutable physical laws.[21][22] In chapter 7 of The World, René Descartes (1596-1650) described «nature» as matter itself, unchanging as created by God, thus changes in parts «are to be attributed to nature. The rules according to which these changes take place I call the ‘laws of nature’.»[23] The modern scientific method which took shape at this time (with Francis Bacon (1561-1626) and Galileo (1564-1642)) contributed to a trend of separating science from theology, with minimal speculation about metaphysics and ethics. (Natural law in the political sense, conceived as universal (i.e., divorced from sectarian religion and accidents of place), was also elaborated in this period by scholars such as Grotius (1583-1645), Spinoza (1632-1677), and Hobbes (1588-1679).)
The distinction between natural law in the political-legal sense and law of nature or physical law in the scientific sense is a modern one, both concepts being equally derived from physis, the Greek word (translated into Latin as natura) for nature.[24]
See alsoEdit
- Empirical research
- Empirical statistical laws
- Formula
- List of laws
- Law (principle)
- Nomology
- Philosophy of science
- Physical constant
- Scientific laws named after people
- Theory
ReferencesEdit
- ^ «law of nature». Oxford English Dictionary (Online ed.). Oxford University Press. (Subscription or participating institution membership required.)
- ^ William F. McComas (30 December 2013). The Language of Science Education: An Expanded Glossary of Key Terms and Concepts in Science Teaching and Learning. Springer Science & Business Media. p. 58. ISBN 978-94-6209-497-0.
- ^ «Definitions from». the NCSE. Retrieved 2019-03-18.
- ^ «The Role of Theory in Advancing 21st Century Biology: Catalyzing Transformative Research» (PDF). Report in Brief. The National Academy of Sciences. 2007.
- ^ Gould, Stephen Jay (1981-05-01). «Evolution as Fact and Theory» (PDF). Discover. 2 (5): 34–37.
- ^ Honderich, Bike, ed. (1995), «Laws, natural or scientific», Oxford Companion to Philosophy, Oxford: Oxford University Press, pp. 474–476, ISBN 0-19-866132-0
- ^ «Law of nature». Oxford English Dictionary (Online ed.). Oxford University Press. (Subscription or participating institution membership required.)
- ^ a b Davies, Paul (2005). The mind of God : the scientific basis for a rational world (1st Simon & Schuster pbk. ed.). New York: Simon & Schuster. ISBN 978-0-671-79718-8.
- ^ a b c Feynman, Richard (1994). The character of physical law (Modern Library ed.). New York: Modern Library. ISBN 978-0-679-60127-2.
- ^ Frisch, Mathias (May 2014). «Laws in Physics | European Review | Cambridge Core». European Review. 22 (S1): S33–S49. doi:10.1017/S1062798713000768. S2CID 122262641.
- ^ Andrew S. C. Ehrenberg (1993), «Even the Social Sciences Have Laws», Nature, 365:6445 (30), page 385.(subscription required)
- ^ Feynman Lectures on Physics: Volume 2, R.P. Feynman, R.B. Leighton, M. Sands, Addison-Wesley, 1964, ISBN 0-201-02117-X
- ^ Encyclopaedia of Physics (2nd Edition), R.G. Lerner, G.L. Trigg, VHC Publishers, 1991, ISBN (Verlagsgesellschaft) 3-527-26954-1 (VHC Inc.) 0-89573-752-3
- ^ Classical Mechanics, T.W.B. Kibble, European Physics Series, McGraw-Hill (UK), 1973, ISBN 0-07-084018-0
- ^ Gravitation and Inertia, I. Ciufolini and J.A. Wheeler, Princeton Physics Series, 1995, ISBN 0-691-03323-4
- ^ 2.^ Classical Mechanics, T.W.B. Kibble, European Physics Series, McGraw-Hill (UK), 1973, ISBN 0-07-084018-0
- ^ Reed ES: The lawfulness of natural selection. Am Nat. 1981; 118(1): 61–71.
- ^ a b Byerly HC: Natural selection as a law: Principles and processes. Am Nat. 1983; 121(5): 739–745.
- ^ in Daryn Lehoux, What Did the Romans Know? An Inquiry into Science and Worldmaking (Chicago: University of Chicago Press, 2012), reviewed by David Sedley, «When Nature Got its Laws», Times Literary Supplement (12 October 2012).
- ^ Sedley, «When Nature Got Its Laws», Times Literary Supplement (12 October 2012).
- ^ Davies, Paul (2007-11-24). «Taking Science on Faith». The New York Times. ISSN 0362-4331. Retrieved 2016-10-07.
Isaac Newton first got the idea of absolute, universal, perfect, immutable laws from the Christian doctrine that God created the world and ordered it in a rational way.
- ^ Harrison, Peter (8 May 2012). «Christianity and the rise of western science». ABC.
Individuals such as Galileo, Johannes Kepler, Rene Descartes and Isaac Newton were convinced that mathematical truths were not the products of human minds, but of the divine mind. God was the source of mathematical relations that were evident in the new laws of the universe.
- ^ «Cosmological Revolution V: Descartes and Newton». bertie.ccsu.edu. Retrieved 2016-11-17.
- ^
Some modern philosophers, e.g. Norman Swartz, use «physical law» to mean the laws of nature as they truly are and not as they are inferred by scientists. See Norman Swartz, The Concept of Physical Law (New York: Cambridge University Press), 1985. Second edition available online [1].
Further readingEdit
- John Barrow (1991). Theories of Everything: The Quest for Ultimate Explanations. (ISBN 0-449-90738-4)
- Dilworth, Craig (2007). «Appendix IV. On the nature of scientific laws and theories». Scientific progress : a study concerning the nature of the relation between successive scientific theories (4th ed.). Dordrecht: Springer Verlag. ISBN 978-1-4020-6353-4.
- Francis Bacon (1620). Novum Organum.
- Hanzel, Igor (1999). The concept of scientific law in the philosophy of science and epistemology : a study of theoretical reason. Dordrecht [u.a.]: Kluwer. ISBN 978-0-7923-5852-7.
- Daryn Lehoux (2012). What Did the Romans Know? An Inquiry into Science and Worldmaking. University of Chicago Press. (ISBN 9780226471143)
- Nagel, Ernest (1984). «5. Experimental laws and theories». The structure of science problems in the logic of scientific explanation (2nd ed.). Indianapolis: Hackett. ISBN 978-0-915144-71-6.
- R. Penrose (2007). The Road to Reality. Vintage books. ISBN 978-0-679-77631-4.
- Swartz, Norman (20 February 2009). «Laws of Nature». Internet encyclopedia of philosophy. Retrieved 7 May 2012.
External linksEdit
- Physics Formulary, a useful book in different formats containing many or the physical laws and formulae.
- Eformulae.com, website containing most of the formulae in different disciplines.
- Stanford Encyclopedia of Philosophy: «Laws of Nature» by John W. Carroll.
- Baaquie, Belal E. «Laws of Physics : A Primer». Core Curriculum, National University of Singapore.
- Francis, Erik Max. «The laws list».. Physics. Alcyone Systems
- Pazameta, Zoran. «The laws of nature». Committee for the scientific investigation of Claims of the Paranormal.
- The Internet Encyclopedia of Philosophy. «Laws of Nature» – By Norman Swartz
- «Laws of Nature», In Our Time, BBC Radio 4 discussion with Mark Buchanan, Frank Close and Nancy Cartwright (Oct. 19, 2000)

In general, a scientific law is the description of an observed phenomenon. It doesn’t explain why the phenomenon exists or what causes it. The explanation for a phenomenon is called a scientific theory. It is a misconception that theories turn into laws with enough research.
«In science, laws are a starting place,» said Peter Coppinger, an associate professor of biology and biomedical engineering at the Rose-Hulman Institute of Technology in India. «From there, scientists can then ask the questions, ‘Why and how?'»
Difference between a scientific theory and a scientific law
Many people think that if scientists find evidence that supports a hypothesis, the hypothesis is upgraded to a theory, and if the theory is found to be correct, it is upgraded to a law. That is not how it works, though. Facts, theories and laws — as well as hypotheses — are separate elements of the scientific method. Though they may evolve, they aren’t upgraded to something else.
«Hypotheses, theories and laws are rather like apples, oranges and kumquats: One cannot grow into another, no matter how much fertilizer and water are offered,» according to the University of California, Berkeley (opens in new tab). A hypothesis is a potential explanation of a narrow phenomenon; a scientific theory is an in-depth explanation that applies to a wide range of phenomena. A law is a statement about an observed phenomenon or a unifying concept, according to Kennesaw State University (opens in new tab).
«There are four major concepts in science: facts, hypotheses, laws and theories,» Coppinger told Live Science.
Though scientific laws and theories are supported by a large body of empirical evidence that is accepted by the majority of scientists within that area of scientific study, and help to unify that body of data, they are not the same thing.
«Laws are descriptions — often mathematical descriptions — of natural phenomena for example, Newton’s Law of Gravity or Mendel’s Law of Independent Assortment. These laws simply describe the observation. Not how or why they work,» Coppinger said.
Coppinger pointed out that the law of gravity was discovered by Isaac Newton in the 17th century. This law mathematically describes how two different bodies in the universe interact with each other. However, Newton’s law doesn’t explain what gravity is or how it works. It wasn’t until three centuries later, when Albert Einstein developed the theory of Relativity, that scientists began to understand what gravity is and how it works.
(opens in new tab)
«Newton’s law is useful to scientists in that astrophysicists can use this centuries-old law to land robots on Mars. But it doesn’t explain how gravity works, or what it is. Similarly, Mendel’s Law of Independent Assortment describes how different traits are passed from parent to offspring, not how or why it happens,» Coppinger said. Gregor Mendel discovered that two different genetic traits would appear independently of each other in different offspring. «Yet, Mendel knew nothing of DNA or chromosomes. It wasn’t until a century later that scientists discovered DNA and chromosomes — the biochemical explanation of Mendel’s laws. It was only then that scientists, such as T.H. Morgan, working with fruit flies, explained the Law of Independent Assortment using the theory of chromosomal inheritance. Still today, this is the universally accepted explanation (theory) for Mendel’s Law,» Coppinger said.
The difference between scientific laws and scientific facts is a bit harder to define, though the definition is important. Facts are simple, one-off observations that have been shown to be true. Laws are generalized observations about a relationship between two or more things in the natural world based on a variety of facts and empirical evidence, often framed as a mathematical statement, according to NASA.
For example, «Apples fall down from this apple tree» is considered a fact because it is a simple statement that can be proven. «The strength of gravity between any two objects (like an apple and the Earth) depends on the masses of the objects and the distance between them» is a law because it describes the behavior of two objects in a certain circumstance. If the circumstance changes, then the implications of the law would change. For example, if the apple and the Earth shrank to a subatomic size, they would behave differently.
Scientific laws and mathematics
(opens in new tab)
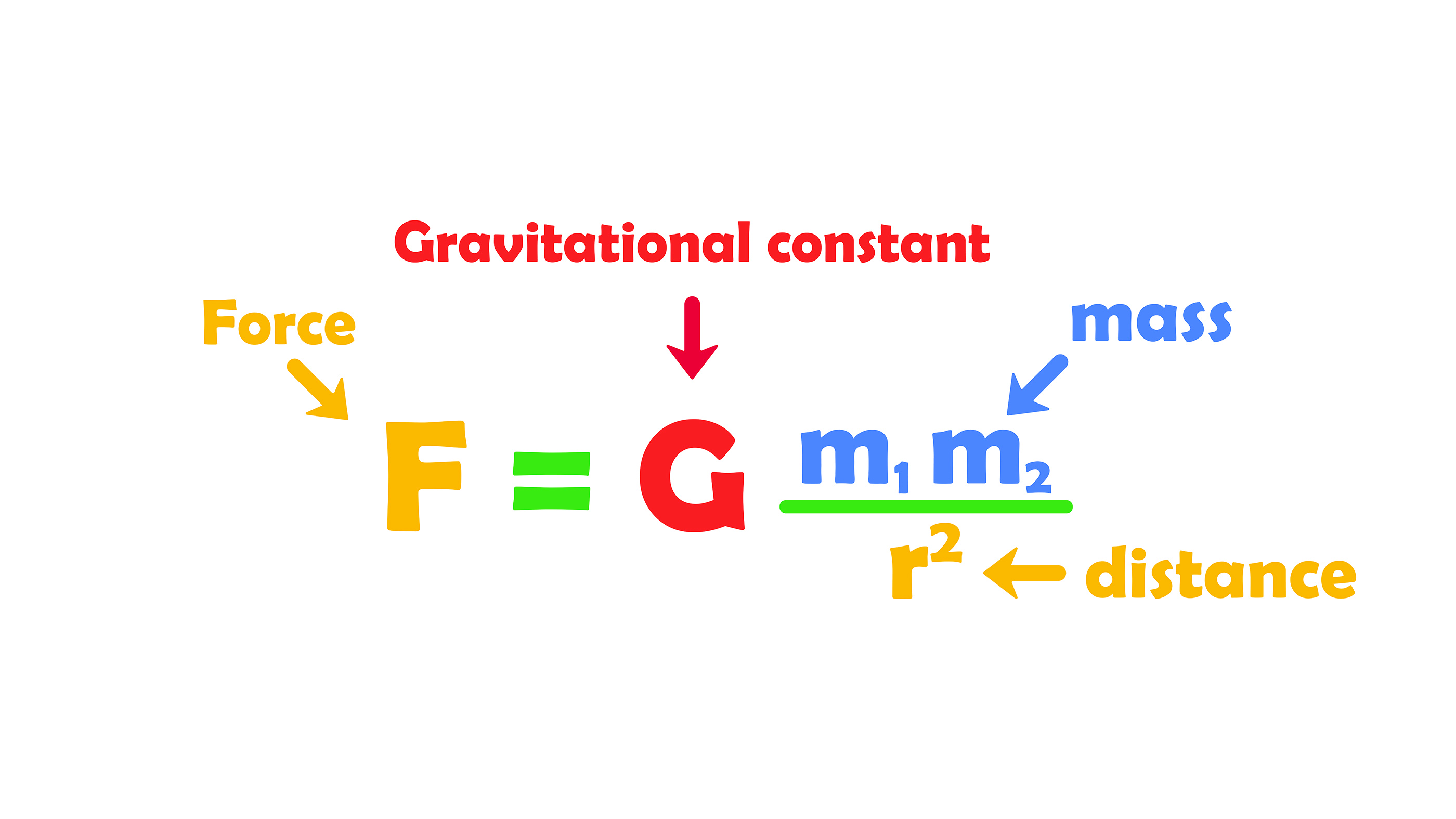
Many scientific laws can be boiled down to a mathematical equation. For example, Newton’s Law of Universal Gravitation states:
Fg = G (m1 ∙ m2) / d2
Fg is the force of gravity; G is the universal gravitational constant, which can be measured; m1 and m2 are the masses of the two objects, and d is the distance between them, according to The Ohio State University (opens in new tab).
Scientific laws are also often governed by the mathematics of probability. «With large numbers, probability always works. The house always wins,» said Sylvia Wassertheil-Smoller, a professor at Albert Einstein College of Medicine in New York. «We can calculate the probability of an event and we can determine how certain we are of our estimate, but there is always a trade-off between precision and certainty. This is known as the confidence interval. For example, we can be 95% certain that what we are trying to estimate lies within a certain range or we can be more certain, say 99% certain, that it lies within a wider range. Just like in life in general, we must accept that there is a trade-off.»
Do laws change?
Just because an idea becomes a law doesn’t mean that it can’t be changed through scientific research in the future. The use of the word «law» by laymen and scientists differs. When most people talk about a law, they mean something that is absolute. A scientific law is much more flexible. It can have exceptions, be proven wrong or evolve over time, according to the University of California, Berkeley.
«A good scientist is one who always asks the question, ‘How can I show myself wrong?'» Coppinger said. «In regards to the Law of Gravity or the Law of Independent Assortment, continual testing and observations have ‘tweaked’ these laws. Exceptions have been found. For example, Newton’s Law of Gravity breaks down when looking at the quantum (subatomic) level. Mendel’s Law of Independent Assortment breaks down when traits are «linked» on the same chromosome.»
Examples of scientific laws
- The law of conservation of energy, which says that the total energy in an isolated system remains constant. In other words, energy cannot be created or destroyed, according to Britannica (opens in new tab).
- The laws of thermodynamics, which deal with the relationships between heat and other forms of energy
- Newton’s universal law of gravitation, which says that any two objects exert a gravitational force upon each other, according to the University of Winnipeg (opens in new tab)
- Hubble’s law of cosmic expansion, which defines a relationship between a galaxy’s distance and how fast it’s moving away from us, according to astrophysicist Neta A. Bahcall
- The Archimedes Principle, which states that the buoyant force on an object submerged in a fluid is equal to the weight of the fluid displaced by that object.
Additional resources
- This resource from the New South Wales Education Standards Authority (opens in new tab) has an in-depth explanation of scientific theories and laws.
- Find out why a theory can’t evolve into a law in this article from Indiana Public Media (opens in new tab).
- Watch a video about the difference between a scientific law and a scientific theory from TEDEd. (opens in new tab)
Bibliography
University of California, Berkeley, «Misconceptions about science.» https://undsci.berkeley.edu/teaching/misconceptions.php
NASA IMAGE Education Center, «Teacher’s Guide: Theories, Hypothesis, Laws, Facts & Beliefs.» https://www.nasa.gov/pdf/371711main_SMII_Problem23.pdf
The Ohio State University, «Lecture 18: The Apple and the Moon: Newtonian Gravity.» https://www.astronomy.ohio-state.edu/pogge.1/Ast161/Unit4/gravity.html
Encyclopedia Britannica, «Conservation of energy.» November 16, 2021. https://www.britannica.com/science/conservation-of-energy
University of Winnipeg, «Newton’s Law of Gravitation.» 1997. https://theory.uwinnipeg.ca/physics/circ/node7.html
Neta A. Bahcall, «Hubble’s Law and the expanding universe,» Proceedings of the National Academy of Sciences, Volume 112, March 2015, https://doi.org/10.1073/pnas.1424299112
Alina Bradford is a contributing writer for Live Science. Over the past 16 years, Alina has covered everything from Ebola to androids while writing health, science and tech articles for major publications. She has multiple health, safety and lifesaving certifications from Oklahoma State University. Alina’s goal in life is to try as many experiences as possible. To date, she has been a volunteer firefighter, a dispatcher, substitute teacher, artist, janitor, children’s book author, pizza maker, event coordinator and much more.
Most Popular
Words have precise meanings in science. For example, «theory,» «law,» and «hypothesis» don’t all mean the same thing. Outside of science, you might say something is «just a theory,» meaning it’s a supposition that may or may not be true. In science, however, a theory is an explanation that generally is accepted to be true. Here’s a closer look at these important, commonly misused terms.
Hypothesis
A hypothesis is an educated guess, based on observation. It’s a prediction of cause and effect. Usually, a hypothesis can be supported or refuted through experimentation or more observation. A hypothesis can be disproven but not proven to be true.
Example: If you see no difference in the cleaning ability of various laundry detergents, you might hypothesize that cleaning effectiveness is not affected by which detergent you use. This hypothesis can be disproven if you observe a stain is removed by one detergent and not another. On the other hand, you cannot prove the hypothesis. Even if you never see a difference in the cleanliness of your clothes after trying 1,000 detergents, there might be one more you haven’t tried that could be different.
Model
Scientists often construct models to help explain complex concepts. These can be physical models like a model volcano or atom or conceptual models like predictive weather algorithms. A model doesn’t contain all the details of the real deal, but it should include observations known to be valid.
Example: The Bohr model shows electrons orbiting the atomic nucleus, much the same way as the way planets revolve around the sun. In reality, the movement of electrons is complicated but the model makes it clear that protons and neutrons form a nucleus and electrons tend to move around outside the nucleus.
Theory
A scientific theory summarizes a hypothesis or group of hypotheses that have been supported with repeated testing. A theory is valid as long as there is no evidence to dispute it. Therefore, theories can be disproven. Basically, if evidence accumulates to support a hypothesis, then the hypothesis can become accepted as a good explanation of a phenomenon. One definition of a theory is to say that it’s an accepted hypothesis.
Example: It is known that on June 30, 1908, in Tunguska, Siberia, there was an explosion equivalent to the detonation of about 15 million tons of TNT. Many hypotheses have been proposed for what caused the explosion. It was theorized that the explosion was caused by a natural extraterrestrial phenomenon, and was not caused by man. Is this theory a fact? No. The event is a recorded fact. Is this theory, generally accepted to be true, based on evidence to-date? Yes. Can this theory be shown to be false and be discarded? Yes.
Law
A scientific law generalizes a body of observations. At the time it’s made, no exceptions have been found to a law. Scientific laws explain things but they do not describe them. One way to tell a law and a theory apart is to ask if the description gives you the means to explain «why.» The word «law» is used less and less in science, as many laws are only true under limited circumstances.
Example: Consider Newton’s Law of Gravity. Newton could use this law to predict the behavior of a dropped object but he couldn’t explain why it happened.
As you can see, there is no «proof» or absolute «truth» in science. The closest we get are facts, which are indisputable observations. Note, however, if you define proof as arriving at a logical conclusion, based on the evidence, then there is «proof» in science. Some work under the definition that to prove something implies it can never be wrong, which is different. If you’re asked to define the terms hypothesis, theory, and law, keep in mind the definitions of proof and of these words can vary slightly depending on the scientific discipline. What’s important is to realize they don’t all mean the same thing and cannot be used interchangeably.
- Apply Apply UG PG
- FEESPAY FEES ONLINE
B.Des Phase — 2 Admissions 2023 is Open
What is the Definition of Laws in Science?
I have noticed that many students use the word laws in a variety of ways in class
For example, a student may ask a question, “What is the definition of laws in science?” When a student asks a question of this type, it means something different to each student. In this article, I will try to explain this term and the meaning that students should understand.
The first portion of the answer to the question of”what may be the definition of legislation in science?” Is that this is of regulation depends upon various factors.
To begin with, term paper writing service political science, sociology, economics, and history play an significant part . Students can see the effect of the facets through lecture or simply by doing analysis on subject or the topic.
Many students believe the term’s meaning is simple users.drew.edu and easy to understand. This is an erroneous perception also it must be corrected to attain their full potential. The most important cause that college students cannot attain their complete potential is that they don’t comprehend the approach through the word”legislation” was characterized.
In order to specify a law, it is critical to do a lot more than just employ a word which appears to clarify some thing. In fact, even in textbooks, definitions of phrases are usually quick descriptions of this behavior of events or things. It’s essential to bear in mind an event may be clarified as”legislation” though at the same time that it is clarified with respect to the behavior of the household item or the activity of your human mind. Therefore, in textbooks, the word”legislation” is a category that we associate with specific behaviours or functions.
When we implemented the term”legislation” to individual minds, for example, then we’d be making a new category which would need to become understood. It would end up just like including a note to a dictionary that supposed,”something that’s https://papernow.org/ two sides”
“Law” could only mean that an individual has some kind of power over a biological entity or a physical object. If we attempted to add the word “law” to the dictionary, it would just mean that the group of individuals that were affected had some control over the physical object. Therefore, when students ask what is the definition of laws in science, they are really asking, “What is the definition of the power that people have over organisms or objects?”
As a way to define a law, then it’s imperative to comprehend that the characteristics of the physical object that we’re studying, for example its own nature, arrangement, and how it behaves, the degree in which the bodily thing is capable of affecting the person’s thoughts and behavior, and the speed at which the average person may answer fluctuations in the scenario. A regular case can be considered by us in biology, where we need to know what the connection amongst a larva and the adult form of the receptor is. What size is your larva?
Just how much does the larva travel to accomplish the type? May be the lady able reproduce and also to consume herself? How much time does it require for the larva expand into the shape and to reach maturity?
As we have answered these questionswe are able to move ahead to an even definition of this definition of which could remedy the terms about all of the different aspects of the biology question. The alternative is to know how to answer the different questions relating to 1 component of the level of maturity of this larva, or even this physics query, such as the traits of the organism or perhaps the larva, how it interacts with additional entities.
Once we understand how the biological entities interact, we can also begin to see how these entities affect the human beings that interact with them, or observe them. We can begin to understand how these biological entities can be studied, studied with scientific instruments, and used to control some physical structures.
This information is utilised to control the human beings working together with these biological methods. It may be used to influence the activities of the human beings that study these biological approaches. Systems and how they are manipulated and used.
Scroll to top

A Talk by Mrs. Barkha Kedia Agrawal
Ms. Barkha Kedia Agrawal is an International Long Distance Swimmer. She is recipient of the “Tenzing Norgay National Adventure Award” (Equivalent to Arjuna Award) at the hands of Honourable Prime Minister Shri. Atal Bihari Vajpai, on 20th May 2003 in New Delhi. She was felicitated by Honourable President Dr. APJ Abdul Kalam, in Rashtrapati Bhavan New Delhi, for achieving excellence in the field of swimming. Barkha has successfully crossed the Gibraltar Strait from Spain to Morocco, a distance of 22.2 km in 3 Hrs. 50min, establishing the record of “Second Fastest Woman in the World” in crossing of Gibraltar Strait on 14th September 2001. At that time the waves in the sea were 19-20 feet high and the water temperature was 19⁰ C. She has successfully crossed the most prestigious and challenging of all the channels in the world, The English Channel from England to France, a distance of 39km in 17Hrs. 12Min. on 25th September 2001.
She addressed the students, faculty and staff of DJAD and DJAME on 25th March 2019, on her journey as a sports woman. She concluded on the inspiring note that the students need to develop endurance and focus in order to achieve their goals in life.

Venkat Rao
Red Dot Design Award – Winner 2012
Half dose
Reddot design award winner 2012
Venkat Rao completed his UG Diploma in 2010 in Industrial Design from DJAD. His entry “Half Dose” was selected for the award in August 2012.
His solution aims at offering a very easy method to break tablets into two.
Often splitting a tablet can get difficult and inaccurate for the elderly, visually impaired and single-handed people. Contamination due to touch is a high risk with the current design. Half dose offers a smart design solution to this problem. The Unique shape of the tablet lets the user split it using a single finger without removing it from the pack into two equal halves.

Shiva Nallaperumal
Winner – SOTA Catalyst Award 2015
Shiva Nallaperumal who completed his UG Diploma in Communication Design in 2013 from DJAD was the first alumni of DJAD to receive the SOTA Catalyst Award in the year 2015.

Jayashree.V
Finalist – Taiwan International Student Design Competition
Jayashree Venkat adorns the space in DJAD’s ‘Wall of Fame’ by achieving final position in Taiwan International Student Design Competition in Visual Design Category. She had completed the UG Diploma in Communication Design in the year 2017 from DJAD.
Jayashree created a couple of visuals of Mother and Child expressing their feelings for each other in the journey of the child’s career. ‘Ladder’ is a subject of these visuals which shows bonding between mother and child and it creates opportunities for both of them to reach out with each other.

Anagha Narayanan
Winner – SOTA Catalyst Award 2020
Anagha is the third alumni of DJAD to have won the SOTA Catalyst Award. The Society of Typographic Aficionados (SOTA) is an international not-for-profit organisation dedicated to the promotion, study and support of type, its history and development, its use in the world of print and digital imagery, its designers, and its admirers. SOTA is incorporated in the State of New York, USA. She was selected for this award in August 2020.
Anagha Narayanan, a Communication Designer of the DJAD 2018 batch had interned at Black [Foundry] in Paris. Her father owned an offset printing press and this background created an interest in her in type and all things connected. After completing her Graduate Diploma, Anagha joined Universal Thirst Type-foundry where she has been able to contribute to a diverse range of projects and scripts. She is currently working on her first typeface release Ilai. Ilai, one of the first variable Tamil typefaces, is a modern interpretation of 60’s psychedelia. It takes Tamil into new territory by offering nine styles of uncompromisingly quirky forms. It is designed for display uses, including branding, subheadings, and posters.
Final Tiles Gallery id=4 does not exist

Vatsal Shah
2nd & 3rd round Winner – D’Source Corona Design Challenge Award [Category- Game Design]
2nd Round Winner
Vatsal Shah completed his UG Diploma in 2019 in Industrial Design from DJAD. He participated in the D’source Coronavirus Design Challenge competition (organised by IIT Bombay) in the second round under the Game Design stream. His entry was selected for the award in August 2020.
Vatsal created the board game ‘Wreath’. Inspiration of this game was Snakes & Ladders. The game ‘Wreath’ was designed after conducting a brief study on various parts of the design process, which included the etymology. This game is all about how the virus spreads quickly and it also educates people on safety measures to end the spread of virus and at the same time engage people in an entertaining way.
3rd Round Winner
He decorates the Wall of Fame by becoming the winner of the 3rd Round also in the Winners’ Category. Vatsal has designed a card game “Precaution”, which is suitable for children of around 5 years. This will help them to increase their level of concentration, help them to learn the value of time along with creating an awareness about hygiene related issues. Can be played solo or with 2 to 4 partners. The design submitted will also be published in a book which will be handed over to all the winners along with the trophy and certificate.

Kiran S
Winner – D’Source Corona Design Challenge Award [Category- Book Design]
COVID 19 threw up challenges of various nature. Platform for one such challenge was offered by IIT Bombay. They organised D’source Corona Design Challenge seeking creative, innovative, out-of-the-box, resourceful, appropriate design solutions to current challenges thrown to us by the corona virus. This was open to students, recent graduates and young designers from around the world. It was a free design challenge where the solutions will be made available on an open platform for anyone to use.
Kiran S, currently a III Year Communication Design Post Graduate student of DJAD, took part in this international competition and came out successful. There were more than 2,000 entries from 1,242 participants from 52 countries. Her work, an illustrated book titled ‘HOPE’ was selected for the winner’s trophy in May 2020. The style throughout the book is inspired by Kalamkari art form, which is hand painted. The same is used traditionally for block printing on sarees. Kalamkari would give a very different view to a children’s book as the style has hand painting effect and the same carries throughout the book.

Ramkrishna Saiteja
Winner – SOTA Catalyst Award 2017
Ramakrishna completed his UG Diploma in Communication Design In 2015 from DJAD. He is the Second DJADian to have won the prestigious SOTA Catalyst Award. (The Society of Typographic Aficionados) He had achieved this accomplishment in 2017. Created in 2010, the award recognizes a person 25 years of age or younger who demonstrates significant achievement and future promise in the field of typography.
Ramakrishna designed a few Indic scripts, including Coorg Kannada, Coorg Kannada Sans, and a Latin type design. In collaboration with ITF colleagues Jonny Pinhorn and Nikhil Ranganathan, he worked on the Telugu and Kannada extensions for the ITF typeface Akhand.
A panel of judges comprised of notable and experienced professionals selected Ramakrishna Saiteja from among numerous talented and brilliant young creative people from all over the world.

Yashwanthi B S
1st round Winner – D’Source Corona Design Challenge Award [Category- Game Design]
Yashwanthi who completed her UG Diploma in Communication Design in 2019 from DJAD, won the 1st round in the D’source Corona Design Challenge Award [Category- Game Design] in the competition conducted by IIT Bombay in August 2020.
Yashwanthi created an innovative children’s game ‘VIRAL’! The objective of this board game was to spread awareness about the measures to protect one and all to prevent the spread of Covid 19 virus. This game is designed for 2-4 numbers of players with age group of 8 years and above. She implemented Design process including Research, Concept ideation, Mind mapping, Content preparation, Prototyping + Game Testing, Final Design + Execution in chronological order.

Inauguration of Cafeteria
In order to accommodate the long pending requirement of availability of titbits on campus, a cafeteria is now functional. Keeping in mind health hazards of fast food, only healthy snacks are available here. This will be functional in the forenoon from 10am to 12pm and in the afternoon from 3pm to 10pm.

International recognition for D J Academy of Design student – Ms. Kiran.S
COVID 19 has thrown up challenges of various nature. IIT Bombay recently organisedD’source Corona Design Challenge in seeking creative, innovative, out-of-the-box, design solutions to current challenges incurred by the coronavirus threat.
Kiran.S, a second Year Post Graduate student of D J Academy of Design, had taken part and not very surprisingly came out successful in this International competition. There were more than 2,000 entries from 1,242 participants from 52 countries. Her work, an illustrated book titled ‘HOPE’ has been selected to be awarded with the winner’s trophy in an event during May 2021 at IIT Bombay.
The style throughout the book is inspired by Kalamkari art form, which is hand painted. The same is used traditionally for block printing on sarees. Kalamkari would give a very different view to a children’s book as the style has hand painting effect and the same carries throughout the book, says Kiran.S.

Collaboration with Alagappa University
Alagappa University is located at Karaikudi in Tamil Nadu, and was brought into existence by a Special Act of the Government of Tamil Nadu in May 1985 with the objective of fostering research, development and dissemination of knowledge in various branches of learning. Alagappa University is recognized by the University Grants Commission (UGC) of India.
National Assessment and Accreditation Council (NAAC) of UGC accredited Alagappa University with A+ Category with 3.64/4.0 Cumulative Grade Point Average (CGPA). Thus the University became eligible to collaborate with Institutions.
Now, DJAD shall offer B.DES (Bachelor of Design) and M.DES (Master of Design) degrees effective from 2019-20, in collaboration with and under the approval of Alagappa University.

Niels Schoenfelder
Member
Niels Schoenfelder’s education and travel led him to establish Mancini enterprises in 2004, a firm for architecture, interiors, objects, furniture and landscapes in India and abroad. Based on his experience in Europe and thorough understanding of Indian ground realities he has become a specialist in designing and guiding the technical implementation of ambitious projects in India. He heads a team of 28 professionals catering to a wide variety of projects. It is the challenge of diversity which has proven to be the driving force for the firm’s understanding of the different building realities and their potential within the framework of contemporary architecture.

Michael Foley
Member
Michael Foley, Managing Director & Chief Designer, Foley Designs, India, is a National Institute of Design (NID) graduate who headed Titan Design Studio for many years. He is often recognized as one of India’s top 10 product designers. As Titan’s design head, Foley created watches, sunglasses and lifestyle products and was behind some of the company’s most talked-about brands, such as Titan Edge, the world’s slimmest watch, and Fastrack, the youth brand that became a best-seller across all age groups. He set up his own design studio in Bangalore, a couple of years ago. Foley Designs created the Commonwealth Games baton for the 2010 games and is currently ideating on successful waste disposal, which it will execute with Bangalore’s civic agencies.

Rahul Mehrotra
Member
Rahul Mehrotra is an architect and urban designer trained at the School of Architecture, Ahmedabad, and the Graduate School of Design at Harvard University. He has been in private practice since 1990, and works on architecture, urban design and conservation projects. He has built extensively in India, and besides several single family houses, his projects include the Laxmi Machine Works Corporate Office in Coimbatore, an Extension to the Prince of Wales Museum in Bombay, an Institute for Rural Development in Tulzapur, and the Restoration of the Chowmahalla Palace in Hyderabad; he is currently developing (with the Taj Mahal Conservation Collaborative) the Master Plan for the Taj Mahal and its surroundings. Professor Mehrotra is Executive Director of the Urban Design Research Institute, which promotes awareness and research on the city of Bombay. He has also written several books on Bombay, including “Bombay, the Cities Within” and has lectured extensively on urban design, conservation and architecture in India. His most recent book is “The Architecture of the 20th Century in the South Asian Region”. He also serves on several government committees that are responsible for historic preservation and the conservation as well as creation of public spaces in Bombay. Rahul Mehrotra teaches at the University of Michigan, Ann Arbor, where he is an associate professor.

Dr. Lalitha Devi Sanjay Jayavarthanavelu
Trustee
Dr Lalitha Devi Sanjay Jayavarthanavelu is a qualified medical professional. Besides the experience in health care management,she has expertise in general management and administration.She serves on the board of number of companies.She is a Trustee of GKD charity Trust, which manages the GKD Institute for Technological Resources, GKDITR – Tooling centre, D J Academy for Managerial Excellence and D J Academy of Design. She is a member of the Governing Council and Secretary/Correspondent of D J Academy for Managerial Excellence.
Sanjay Jayavarthanavelu
Chairman
Mr. Sanjay Jayavarthanavelu is the Chairman and Managing Director of Lakshmi Machine Works Limited (LMW), Coimbatore. LMW is one of the three leading Textile Machinery Manufacturers in the world, manufacturing the complete range textile spinning machinery.
He was the Chairman of the India-ITME Exhibition Society, Mumbai and is presently the Chairman of its Sub-Committee. He is a Member of the Textiles Committee appointed by the Ministry of Textiles, Govt. of India. He is a Member of the Development Council for Textile Machinery Industry and Machine Tool Industry constituted by the Govt. of India. He is the Member of Steering Committee on Industries & Minerals for the 12th Five Year plan constituted by Govt. of Tamil Nadu. He is an Executive Committee member of the Federation of Indian Chambers of Commerce & Industry (FICCI), New Delhi. He is a Member of the Southern Regional Council of Administration of the Southern India Textile Research Association. He is the Member of the Board of Governors of Sardar Vallabhai Patel International School of Textiles & Management, Coimbatore.
He is a Trustee of the G. Kuppusamy Naidu Charity Trust which runs a 600-bed multi-speciality hospital, a higher secondary school and a feeder school in Coimbatore and an Arts college at Kovilpatti in Tamilnadu. He is a Trustee of the Coimbatore Masonic Charity Trust, which runs a Children’s Hospital and a Working Women’s Hostel. He is a Trustee of GKD Charity Trust, which runs D J Academy for Managerial Excellence, D J Academy of Design and GKD Institute for Technological Resources. He is also a Member of the Coimbatore Golf Club Trust.
An active supporter of Sports, he is the Vice-President of the Tamil Nadu Shooting Association, Chennai and the Hon Secretary of the Rifle Club, Coimbatore.

Atul Kedia
Member
He has 30+ years of rich experience in Design, Development, Engineering, Research and allied fields working in industry as well as academics. For many years he was holding a key position in VIP Industries Ltd. Nasik. Under his leadership the company could establish its position as one of the top design driven companies in the country.In academics, he was holding a top position in SoDS – School of Design studies, University of Petroleum at Dehradun. It was a greenfield project, which was established by him from scratch in record time of 2 years. He was also holding the position of Director at Symbiosis Institute of Design in Pune.He has track record of hundreds of successful, global and national product launches of innovative products developed Art-2- Part to his credit. He has contributed immensely in establishing innovative image to brands like VIP & Princeware.In industry, he held key positions in the design & research functions in India at Tata Autocomp, VIP Industries, Blowplast Ergo, Prince Plastics, Gujarat Narmada Auto Ltd. etc.
He is an excellent trainer and has been nurturing creativity & innovations over the years of his vast field experience. Hundreds of successful designers and engineers today have been trained under him over the period of his professional working. This has become possible as over the years he himself has received professional training in various disciplines and topics ranging from Costing to Creativity. Many of them have been successfully practiced & implemented in day to day working.He has 8 patents on his name today as innovator & his professional work has received ‘Engineering Achievement Award –1997’ from Institute of Engineers, India & Prestigious National level ‘Golden Peacock Innovation Award –2004’ on behalf of VIP.
Presentation Skills Training Session Conducted for students, by Ms. Vineeta Chopra
To help students effectively organize their internship work in a form a presentation a workshop was conducted by Ms. Vineeta Chopra. The workshop had the following topics:i. Techniques of structuring a Presentation, ii. Methods of organizing content, iii. Best Practices in the delivery of presentations The workshop concluded with 10-min final presentations by students. The presentations were attended by Prof. Subramanya and Prof. Gopi Chandran along with instructor, to provide areas of improvements to students.
HEEK Educational Expo Visit (Kochi)
A team from DJ Academy of Design visited the HEEK Educational Expo at Kochi, Kerala between the 19th to 21st of July 2019. The objective of the visit was to enhance the brand awareness of DJ Academy of Design among the prospective parents and students visiting the expo. The team comprised of Mr. Nilesh, Mr. Supratik, Ms. Kothai, and Ms. Venugopal (student).
A total three workshops, which included a Calligraphy, Rapid Sketching and Kirigami Workshop, were conducted during the expo. Favourable feedback has been received for both the workshops.
Corporate Training Workshop for Venus Home Appliances, EXPO ID and CD
A 2-day in-house workshop on Exposure to Industrial Design and Communication Design was conducted on the 9th and 10th of July 2019 for Venus Home Appliances (P) Ltd. The experiential workshop conducted by DJAD faculty members covered areas such as Product Design and Development, Product Communication and Aesthetics, Semantics, Design and Innovation, Product Sketching, Visual Communication, and key areas of Sustainable Design.
The participants have expressed that they found the topics relevant and interesting and they also found the learning environment provided by DJAD to be inspiring.

Presentation on Sound and Design
On 1st July 2019, Prof. Tridibesh Sanyal discussed his unique work using sound mixing and composition in a session called Soundscapes. The session explored sound as form and also the link between sound, color and design.

Student Life Cycle (SLM) Management Software
A SLM software has been finalized for implementation which consists of the following modules: Institute Information, Student Information, Faculty Information, Course Management, Attendance Management, Time Table Generation, Feedback System, Mentor–Mentee System, Reporting Module Including TNG (Term Not Granted) and CNG (Course Not Granted) reports and Visiting Faculty Module.
This software will help DJAD in maintaining all student data on server, thereby reducing the use of paper to a large extent. Also, it will provide detailed information to parents, faculty, staff and students.
Student’s data will be available and processed in digital format right from admission, registration, till placements and alumni.

India Design Mission, Finland
On behalf DJ Academy of Design, Prof. Atul Kedia (Dean, DJ Academy of Design) participated in India Design Mission to Finland which was organised by CII between the 13th & 17th May 2019.The aim was to expose a team of select Indian design practitioners and industries to global design best practices. This meticulously planned mission had design firms ranging from product design, branding and service design sharing their processes and important projects.
On the academic front, visit to Aalto University Design department; PDP Design factory and Product design Gala Day (student Exhibition) were the highlights.

Order In Space: Understanding Geometry
The foundation students, during February 2017, had a module with Prof. A.G. Rao, a pioneer in Design Education from IDC, IIT Bombay, along with in-house co-faculty Mr. Subramanya. The students were introduced to 3D geometry and basics of 3D structures. The students used the learning to build Geodesic domes and Tensegrity Structures.DJAD is stepping into a new academic year, 2019-20. This is going to be a milestone in its history with courses of B.Des and M.Des on offer from this year. We start with lessons learnt in the past one and half decades and new ideas that are sweeping the world of design.

Stone Craft Development
The 2nd year Industrial Design students had a course on Craft Development and this included a visit to Thirumurugan Poondi in Thirupur district and meeting the master craftsman Mr.Radhakrishnan. They did a study on understanding stone, their types, manufacturing process and current trends. With these insights each of the 15 students conceptualized ideas using stone as a material. Students explored lighting, jewellery, accessories using stone. The students then went on to developing the products using the actual materials. Many students worked on the model themselves using the tools, and were supported by the craftsmen and their team. On 27th March 2019, students displayed their products at the campus. Craft Council of Tamil Nadu members visited D J Academy of Design Campus to see the finished products and expressed their appreciation for the students’ work.

Pana Olai Pinnal – A Craft Study
The 3rd year Communication Design students of D J Academy of Design for their craft study course visited the Pana Olai Pinnal Craft at Kolathur, Bodipalayam, Pollachi. This course was done in collaboration with the Craft Council of Tamil Nadu. The students during their visit interacted with the craftsmen Krishna Swamy and his wife, Karuppatha. It is important to document the crafts, because most of them are going extinct due to the rapid growth of technology and the mass manufacturing methods.
Pana Olai Pinnal is a craft that deals with weaving of palm leaves and Krishna Swamy has developed his own unique weaving style. He has developed weaving patterns to make little ornamentations and these ornamentations are found in different forms such as parrot, fish, deer, flower etc. These ornamentations are used to decorate temples or houses during festivals and are now being used at weddings as well.
Following the visit, the students have come up with a publication and a documentary film on Olai Craft weaving. As designers, the visit not only made students understand the craft but also brought a level of understanding and empathy in them. It also made them more aware of the possibilities to work with such artisans and also realise the importance of bringing the craft to the forefront.

Clay Embark
Clay is an extremely pliable and versatile material that is extensively used in the ceramic industry for sculpting and model making purposes. Not only is Clay Modelling a very useful skill to learn in the design field but it is also a very joyous one as it is a skill that requires a combination of both cognitive and physical involvement. As a beginner, ‘Hand Building’ is the asset of clay working techniques that one needs to learn and master as it is a crucial part of working with clay. In March 2019, the DJAD students had open elective with DJAD alumni Sushmita for Clay Embark. The students explored the material and displayed their work in an exhibition.

Design Movements – Learning History with Fun
The Design Movement course is a course which deals with the design history. The actual course is very theoretical but by adopting this method it becomes more interesting to the students. Using this approach the students learn the nuances of the movement, its philosophies, designers etc. During March 2019, the 2nd year students of Industrial and Communication Design worked on a dance musical. The whole idea of a performance helped students in grasping the core idea of each movement in a creative way. It is very interesting way of learning about the movements and breaking monotony.

Jury/Diploma Jury for 2018-19
Juries for 1st, 2nd and 3rd years were held in the month of April. The Jury panel Included external as well as internal members. Pre-jury for final year diploma project was held in the last week of May, where the students were given feedback about their final presentation so that the same can be fine-tuned before final jury, which was held in the first week of June.

DJAD Re-opening 2019-20 (for 2nd and 3rd years)
DJAD reopened for the year 2019-20 on 10th June 2019 for the second and third year students and for the final year students, the classes commenced on the 1st July 2019. During the re-opening day meeting, Prof. Atul Kedia, Dean and Capt. K Senthil Kumar, Chief Executive, interacted with the parents and shared the overall vision for the upcoming year and also took the opportunity to clarify specific questions on academics and other topics. Faculty members who were available on the day also could participate.
Undergraduate
| Age | Up to 23 years |
| Course | Bachelor of Design (B.Des) Undergraduate Programme in Industrial Design (IND) / Communication Design (CND) |
| Duration | 4 Years |
| Eligibility | 10 + 2 from any stream |
The Bachelor of Design (B.Des) undergraduate programme at DJAD starts with a one year Design Foundation Programme that concentrates on learning basic concepts of design involving courses that build the strong foundation for design thinking and practice. The students need to select a discipline at the time of applying to DJAD.
The learning is interdisciplinary as some of the courses offered to Industrial, Communication Design students are common encouraging them to work across disciplines. In the fourth year they specialize in one of the following areas by choosing design projects from their area of interest. Fourth year also consists of internship, colloquium paper and a six-month degree / diploma project.
Postgraduate
| Course | Master of Design (M.Des) Postgraduate Programme in Industrial Design / Communication Design / Service Design / Furniture & Space Design / User Experience Design |
| Duration | 2 Years |
| Age | 20 – 27 Years |
| Eligibility | Degree in Any Discipline |
Uniqueness of the DJAD program
Here at DJAD, each individual is groomed to be an independent thinker and doer. The Design programs strive to create individuals who can play a leadership role in the industry. The Course emphasizes a strong foundational focus on design fundamentals and principles, encourages experimentation, and inculcates an innovation mind-set. All courses are designed to inculcate a keen sense for the value parameters in design, where design becomes a key differentiator to a product or a service.