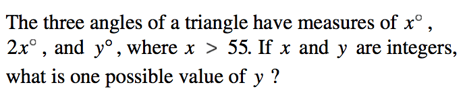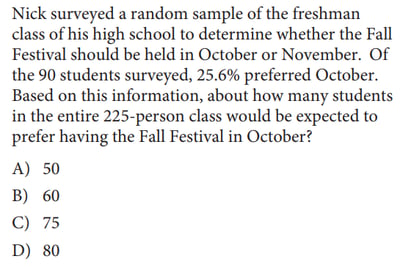6,738 Answered Questions for the topic Word Problem
The question is in the description.
There are cards in a box. Fifteen of these cards are red, the others are blue, and the rests are white cards. If the probability of drawing a blue card is 0.125 and the probability of drawing a…
more
need answered asap please
A truck enters a highway driving 60 mph. A car enters the highway at the same place 5 minutes later and drives 69 mph in the same direction. From the time the car enters the highway, how long will…
more
Middle school math finding the distance
You and your friend are standing back to back. Your friend runs 13 feet forward and 3 feet right. At the same time, you run 11 feet forward and 7 feet right. You stop and throw a baseball to your…
more
System of equations question!
Afternoon. I’m struggling to understand where to really go with this question! It reads as follows:========================»A lot of people have taken to baking as a pandemic interest. You’ve…
more
word problem Algebra 1
Adam and Jill each improved their yards by planting daylilies and geraniums. They bought theirsupplies from the same store. Adam spent $56 on 7 daylilies and 7 geraniums. Jill spent $94 on14…
more
Word problem!!!!!
Jen has in price speed past train fairs for a group trip to New York. Three adults and four children must pay $129. Two adults and three children must pay $92. Find the price of the adult ticket…
more
algebra word problem
Justin sells tickets at the theatre. At the end of the evening, he had $524.00 in toonies and $5 bills. The total number of coins and bills was 145. How many toonies and $5 bills does he have?
i need help with this word problem
Susan sells beaded necklaces. Each large necklace sells for $5.40 and each small necklace sells for $4.50. How much will she earn from selling 3 large necklaces and 1 small necklace?
i need help with this word problem
Aldo bought 2 CDs that were each the same price. Including sales tax, he paid a total of $25.60. Of that total, $2.40 was tax. What was the price of each CD before tax?
i need help on this word problem
Joe made 4 identical necklaces, each having beads and a pendant. The total cost of the beads and pendants for all 4 necklaces was $23.60. If the beads cost $3.10 for each necklace, how much did…
more
Pre Calc Probability Word Problem
You enter a lottery buying one ticket. There are 45 numbers and you try to choose 5 of them. Then, there are 30 red balls numbered 1-30 and you try to get that number right. How many possible…
more
Pre Calc Probability Word Problem
there are 5 red balls, 30 black balls, and 7 pink balls in a jar. You pull one out, don’t replace it, and pull another one out. Find the probability of getting two of the same color? Please include…
more
Still looking for help? Get the right answer, fast.
OR
Find an Online Tutor Now
Choose an expert and meet online.
No packages or subscriptions, pay only for the time you need.

3-Step System
1. Read: Read the problem and decide what the question is asking.
- Read the problem 2 times or more.
- Underline or circle key words, phrases, and numbers. Draw a line through irrelevant information.
2. Plan: Think about what the story is asking you to do. What information are you given, and what do you need to find out?
- Draw a picture.
- Circle or underline key words. (Use highlighters or crayons to color-code key numbers and phrases.)
- Write out the question in your own words.
3. Solve: What strategy could you use to find the missing information: addition, subtraction, multiplication, or division?
- Write a number sentence and solve.
- Use counters.
- Create charts.
Check your work by explaining your reasoning. Does your answer make sense?
Download this free strategy checklist from Math Fundamentals to help your child solve word problems.
Different Strategies to Solve Word Problems
Everyone learns in a different way. What makes sense to one individual often isn’t the easiest option for another. Incorporating different strategies to solve word problems can help your child discover what strategy works best for him or her. A few tips to use are:
1. Circle numbers in a story and underline key phrases.
Color coding is a fun method to incorporate to help children decide what operation the question is asking for. Assign a color to each operation and highlight the phrase that identifies it. For example, red links to addition and blue links to subtraction.
2. Incorporate a key word list.
Key word lists are best used for teaching younger children how to solve word problems. As math curriculum advances, children should not be dependent on a key word list to solve a problem. The questions get trickier.
Addition
In all
Together
Total
Altogether
Combine
Sum
Join
Subtraction
Difference
Fewer
How many more
How much more
Left
Remain
Less
3. Visuals
If your child is a visual learner, drawing a picture or using counters can help him or her understand what the problem is asking. Use number lines, charts, or counters or draw a picture.
4. Write your own word problem.
Knowing what is needed to write a word problem is the first step in identifying key words to solve a story. Take turns writing your own word problems with your child and exchange them to solve.
5. Stay organized.
It is important to write clearly and keep work space neat so children can read and follow their own computations. Many children need a separate piece of paper to allow them enough space to solve and understand their answer. Graphing paper is a great option to help students record neat work.
Download this free sample word problem from Math Fundamentals, grade 1.
How to solve a two-step word problem
In a two-step word problem children are being asking to solve two related equations. These can get tricky for children to understand when they transition from one-step to two-step problems. Help your child understand his or her relationships within two-step word problems with these strategies:
1. Circle important information.
Circle numbers and important phrases that ask questions. The number sentences needed to solve these equations are hidden in those asking questions. Identify the first and second questions needed to solve.
2. Distinguish the two parts of the problem.
First identify the first step of the first part of the word problem. Write a number sentence and solve.
3. Use the answer from the first-step solution to the whole problem.
Use the answer from the first question to help you solve the next equation. What operation does the second question require?
Check your work by explaining your reasoning. What was the question answered? Is the answer reasonable for the question being asked?
Download this free sample two-strategy word problem from Math Fundamentals, grade 2
Download this free sample multi-strategy word problem from Math Fundamentals, grade 4
Evan-Moor’s Math Fundamentals is a great resource for training students how to solve word problems in 3 simple steps. It provides step-by-step directions for solving questions and guides children with helpful visuals and key phrases.
Check out Daily Word Problems for consistent practice solving word problems.
For more fun math tips and strategies check out our Math- Ideas, Activities and Lessons Pinterest Board.
Save these tips and Pin It now!


It’s one thing to solve a math equation when all of the numbers are given to you but with word problems, when you start adding reading to the mix, that’s when it gets especially tricky.
The simple addition of those words ramps up the difficulty (and sometimes the math anxiety) by about 100!
How can you help your students become confident word problem solvers? By teaching your students to solve word problems in a step by step, organized way, you will give them the tools they need to solve word problems in a much more effective way.
Here are the seven strategies I use to help students solve word problems.
1. Read the Entire Word Problem
Before students look for keywords and try to figure out what to do, they need to slow down a bit and read the whole word problem once (and even better, twice). This helps kids get the bigger picture to be able to understand it a little better too.
2. Think About the Word Problem
Students need to ask themselves three questions every time they are faced with a word problem. These questions will help them to set up a plan for solving the problem.
Here are the questions:
A. What exactly is the question?
What is the problem asking? Often times, curriculum writers include extra information in the problem for seemingly no good reason, except maybe to train kids to ignore that extraneous information (grrrr!). Students need to be able to stay focused, ignore those extra details, and find out what the real question is in a particular problem.
B. What do I need in order to find the answer?
Students need to narrow it down, even more, to figure out what is needed to solve the problem, whether it’s adding, subtracting, multiplying, dividing, or some combination of those. They’ll need a general idea of which information will be used (or not used) and what they’ll be doing.
This is where key words become very helpful. When students learn to recognize that certain words mean to add (like in all, altogether, combined), while others mean to subtract, multiply, or to divide, it helps them decide how to proceed a little better
Here’s a Key Words Chart I like to use for teaching word problems. The handout could be copied at a smaller size and glued into interactive math notebooks. It could be placed in math folders or in binders under the math section if your students use binders.
One year I made huge math signs (addition, subtraction, multiplication, and divide symbols) and wrote the keywords around the symbols. These served as a permanent reminder of keywords for word problems in the classroom.
If you’d like to download this FREE Key Words handout, click here:

C. What information do I already have?
This is where students will focus in on the numbers which will be used to solve the problem.
3. Write on the Word Problem
This step reinforces the thinking which took place in step number two. Students use a pencil or colored pencils to notate information on worksheets (not books of course, unless they’re consumable). There are lots of ways to do this, but here’s what I like to do:
- Circle any numbers you’ll use.
- Lightly cross out any information you don’t need.
- Underline the phrase or sentence which tells exactly what you’ll need to find.
4. Draw a Simple Picture and Label It
Drawing pictures using simple shapes like squares, circles, and rectangles help students visualize problems. Adding numbers or names as labels help too.
For example, if the word problem says that there were five boxes and each box had 4 apples in it, kids can draw five squares with the number four in each square. Instantly, kids can see the answer so much more easily!
5. Estimate the Answer Before Solving
Having a general idea of a ballpark answer for the problem lets students know if their actual answer is reasonable or not. This quick, rough estimate is a good math habit to get into. It helps students really think about their answer’s accuracy when the problem is finally solved.
6. Check Your Work When Done
This strategy goes along with the fifth strategy. One of the phrases I constantly use during math time is, Is your answer reasonable? I want students to do more than to be number crunchers but to really think about what those numbers mean.
Also, when students get into the habit of checking work, they are more apt to catch careless mistakes, which are often the root of incorrect answers.
7. Practice Word Problems Often
Just like it takes practice to learn to play the clarinet, to dribble a ball in soccer, and to draw realistically, it takes practice to become a master word problem solver.
When students practice word problems, often several things happen. Word problems become less scary (no, really).
They start to notice similarities in types of problems and are able to more quickly understand how to solve them. They will gain confidence even when dealing with new types of word problems, knowing that they have successfully solved many word problems in the past.
If you’re looking for some word problem task cards, I have quite a few of them for 3rd – 5th graders.
This 3rd Grade Math Task Cards Bundle has word problems in almost every one of its 30 task card sets.
There are also specific sets that are dedicated to word problems and two-step word problems too. I love these because there’s a task card set for every standard.
CLICK HERE to take a look at 3rd grade:

This 4th Grade Math Task Cards Bundle also has lots of word problems in almost every single of its 30 task card sets. These cards are perfect for centers, whole class, and for one on one.
CLICK HERE to see 4th grade:

This 5th Grade Math Task Cards Bundle is also loaded with word problems to give your students focused practice.
CLICK HERE to take a look at 5th grade:

Want to try a FREE set of math task cards to see what you think?
3rd Grade: Rounding Whole Numbers Task Cards
4th Grade: Convert Fractions and Decimals Task Cards
5th Grade: Read, Write, and Compare Decimals Task Cards
Thanks so much for stopping by!

Solving Word Problems in Mathematics
What Is a Word Problem? (And How to Solve It!)
Learn what word problems are and how to solve them in 7 easy steps.
Real life math problems don’t usually look as simple as 3 + 5 = ?. Instead, things are a bit more complex. To show this, sometimes, math curriculum creators use word problems to help students see what happens in the real world. Word problems often show math happening in a more natural way in real life circumstances.
As a teacher, you can share some tips with your students to show that in everyday life they actually solve such problems all the time, and it’s not as scary as it may seem.
As you know, word problems can involve just about any operation: from addition to subtraction and division, or even multiple operations simultaneously.
If you’re a teacher, you may sometimes wonder how to teach students to solve word problems. It may be helpful to introduce some basic steps of working through a word problem in order to guide students’ experience. So, what steps do students need for solving a word problem in math?
Steps of Solving a Word Problem
To work through any word problem, students should do the following:
1. Read the problem: first, students should read through the problem once.
2. Highlight facts: then, students should read through the problem again and highlight or underline important facts such as numbers or words that indicate an operation.
3. Visualize the problem: drawing a picture or creating a diagram can be helpful.
Students can start visualizing simple or more complex problems by creating relevant images, from concrete (like drawings of putting away cookies from a jar) to more abstract (like tape diagrams). It can also help students clarify the operations they need to carry out. (next step!)
4. Determine the operation(s): next, students should determine the operation or operations they need to perform. Is it addition, subtraction, multiplication, division? What needs to be done?
Drawing the picture can be a big help in figuring this out. However, they can also look for the clues in the words such as:
– Addition: add, more, total, altogether, and, plus, combine, in all;
– Subtraction: fewer, than, take away, subtract, left, difference;
– Multiplication: times, twice, triple, in all, total, groups;
– Division: each, equal pieces, split, share, per, out of, average.
These key words may be very helpful when learning how to determine the operation students need to perform, but we should still pay attention to the fact that in the end it all depends on the context of the wording. The same word can have different meanings in different word problems.
Another way to determine the operation is to search for certain situations, Jennifer Findley suggests. She has a great resource that lists various situations you might find in the most common word problems and the explanation of which operation applies to each situation.
5. Make a math sentence: next, students should try to translate the word problem and drawings into a math or number sentence. This means students might write a sentence such as 3 + 8 =.
Here they should learn to identify the steps they need to perform first to solve the problem, whether it’s a simple or a complex sentence.
6. Solve the problem: then, students can solve the number sentence and determine the solution. For example, 3 + 8 = 11.
7. Check the answer: finally, students should check their work to make sure that the answer is correct.
These 7 steps will help students get closer to mastering the skill of solving word problems. Of course, they still need plenty of practice. So, make sure to create enough opportunities for that!
At Happy Numbers, we gradually include word problems throughout the curriculum to ensure math flexibility and application of skills. Check out how easy it is to learn how to solve word problems with our visual exercises!
Word problems can be introduced in Kindergarten and be used through all grades as an important part of an educational process connecting mathematics to real life experience.
Happy Numbers introduces young students to the first math symbols by first building conceptual understanding of the operation through simple yet engaging visuals and key words. Once they understand the connection between these keywords and the actions they represent, they begin to substitute them with math symbols and translate word problems into number sentences. In this way, students gradually advance to the more abstract representations of these concepts.
For example, during the first steps, simple wording and animation help students realize what action the problem represents and find the connection between these actions and key words like “take away” and “left” that may signal them.
From the beginning, visualization helps the youngest students to understand the concepts of addition, subtraction, and even more complex operations. Even if they don’t draw the representations by themselves yet, students learn the connection between operations they need to perform in the problem and the real-world process this problem describes.
Next, students organize data from the word problem and pictures into a number sentence. To diversify the activity, you can ask students to match a word problem with the number sentence it represents.
Solving measurement problems is also a good way of mastering practical math skills. This is an example where students can see that math problems are closely related to real-world situations. Happy Numbers applies this by introducing more complicated forms of word problems as we help students advance to the next skill. By solving measurement word problems, students upgrade their vocabulary, learning such new terms as “difference” and “sum,” and continue mastering the connection between math operations and their word problem representations.
Later, students move to the next step, in which they learn how to create drawings and diagrams by themselves. They start by distributing light bulbs equally into boxes, which helps them to understand basic properties of division and multiplication. Eventually, with the help of Dino, they master tape diagrams!
To see the full exercise, follow this link.
The importance of working with diagrams and models becomes even more apparent when students move to more complex word problems. Pictorial representations help students master conceptual understanding by representing a challenging multi-step word problem in a visually simple and logical form. The ability to interact with a model helps students better understand logical patterns and motivates them to complete the task.
Having mentioned complex word problems, we have to show some of the examples that Happy Numbers uses in its curriculum. As the last step of mastering word problems, it is not the least important part of the journey. It’s crucial for students to learn how to solve the most challenging math problems without being intimidated by them. This only happens when their logical and algorithmic thinking skills are mastered perfectly, so they easily start talking in “math” language.
These are the common steps that may help students overcome initial feelings of anxiety and fear of difficulty of the task they are given. Together with a teacher, they can master these foundational skills and build their confidence toward solving word problems. And Happy Numbers can facilitate this growth, providing varieties of engaging exercises and challenging word problems!
1. A
The ratio between black and blue pens is 7 to 28 or 7:28. Bring to the lowest terms by dividing both sides by 7 gives 1:4.
2. A
At 100% efficiency 1 machine produces 1450/10 = 145 m of cloth.
At 95% efficiency, 4 machines produce 4 * 145 * 95/100 = 551 m of cloth.
At 90% efficiency, 6 machines produce 6 * 145 * 90/100 = 783 m of cloth.
Total cloth produced by all 10 machines = 551 + 783 = 1334 m
Since the information provided and the question are based on 8 hours, we did not need to use time to reach the answer.
3. D
The turnout at polling station A is 945 out of 1270 registered voters. So, the percentage turnout at station A is:
(945/1270) × 100% = 74.41%
The turnout at polling station B is 860 out of 1050 registered voters. So, the percentage turnout at station B is:
(860/1050) × 100% = 81.90%
The turnout at polling station C is 1210 out of 1440 registered voters. So, the percentage turnout at station C is:
(1210/1440) × 100% = 84.03%
To find the total turnout from all three polling stations, we need to add up the total number of voters and the total number of registered voters from all three stations:
Total number of voters = 945 + 860 + 1210 = 3015
Total number of registered voters = 1270 + 1050 + 1440 = 3760
The overall percentage turnout is:
(3015/3760) × 100% = 80.12%
Therefore, the total turnout from all three polling stations is 80.12% — rounding to 80%.
4. D
This is a simple direct proportion problem:
If Lynn can type 1 page in p minutes, then she can type x pages in 5 minutes
We do cross multiplication: x * p = 5 * 1
Then,
x = 5/p
5. A
This is an inverse ratio problem.
1/x = 1/a + 1/b where a is the time Sally can paint a house, b is the time John can paint a house, x is the time Sally and John can together paint a house.
So,
1/x = 1/4 + 1/6 … We use the least common multiple in the denominator that is 24:
1/x = 6/24 + 4/24
1/x = 10/24
x = 24/10
x = 2.4 hours.
In other words; 2 hours + 0.4 hours = 2 hours + 0.4•60 minutes
= 2 hours 24 minutes
6. D
The original price of the dishwasher is $450. During a 15% off sale, the price of the dishwasher will be reduced by:
15% of $450 = 0.15 x $450 = $67.50
So the sale price of the dishwasher will be:
$450 – $67.50 = $382.50
As an employee, the person receives an additional 20% off the lowest price, which is $382.50. We can calculate the additional discount as:
20% of $382.50 = 0.20 x $382.50 = $76.50
So the final price that the employee will pay for the dishwasher is:
$382.50 – $76.50 = $306.00
Therefore, the employee will pay $306.00 for the dishwasher.
7. D
Original price = x,
80/100 = 12590/X,
80X = 1259000,
X = 15,737.50.
8. D
We are given that each of the n employees earns s amount of salary weekly. This means that one employee earns s salary weekly. So; Richard has ‘ns’ amount of money to employ n employees for a week.
We are asked to find the number of days n employees can be employed with x amount of money. We can do simple direct proportion:
If Richard can employ n employees for 7 days with ‘ns’ amount of money,
Richard can employ n employees for y days with x amount of money … y is the number of days we need to find.
We can do cross multiplication:
y = (x * 7)/(ns)
y = 7x/ns
9. B
The distribution is done at three different rates and in three different amounts:
$6.4 per 20 kilograms to 15 shops … 20•15 = 300 kilograms distributed
$3.4 per 10 kilograms to 12 shops … 10•12 = 120 kilograms distributed
550 – (300 + 120) = 550 – 420 = 130 kilograms left. This 50
amount is distributed in 5 kilogram portions. So, this means that there are 130/5 = 26 shops.
$1.8 per 130 kilograms.
We need to find the amount he earned overall these distributions.
$6.4 per 20 kilograms : 6.4•15 = $96 for 300 kilograms
$3.4 per 10 kilograms : 3.4 *12 = $40.8 for 120 kilograms
$1.8 per 5 kilograms : 1.8 * 26 = $46.8 for 130 kilograms
So, he earned 96 + 40.8 + 46.8 = $ 183.6
The total distribution cost is given as $10
The profit is found by: Money earned – money spent … It is important to remember that he bought 550 kilograms of potatoes for $165 at the beginning:
Profit = 183.6 – 10 – 165 = $8.6
10. B
We check the fractions taking place in the question. We see that there is a “half” (that is 1/2) and 3/7. So, we multiply the denominators of these fractions to decide how to name the total money. We say that Mr. Johnson has 14x at the beginning; he gives half of this, meaning 7x, to his family. $250 to his landlord. He has 3/7 of his money left. 3/7 of 14x is equal to:
14x * (3/7) = 6x
So,
Spent money is: 7x + 250
Unspent money is: 6×51
Total money is: 14x
Write an equation: total money = spent money + unspent money
14x = 7x + 250 + 6x
14x – 7x – 6x = 250
x = 250
We are asked to find the total money that is 14x:
14x = 14 * 250 = $3500
11. D
First calculate total square feet, which is 15 * 24 = 360 ft2. Next, convert this value to square yards, (1 yards2 = 9 ft2) which is 360/9 = 40 yards2. At $0.50 per square yard, the total cost is 40 * 0.50 = $20.
About 25% of your total SAT Math section will be word problems, meaning you will have to create your own visuals and equations to solve for your answers. Though the actual math topics can vary, SAT word problems share a few commonalities, and we’re here to walk you through how to best solve them.
This post will be your complete guide to SAT Math word problems. We’ll cover how to translate word problems into equations and diagrams, the different types of math word problems you’ll see on the test, and how to go about solving your word problems on test day.
Feature Image: Antonio Litterio/Wikimedia
What Are SAT Math Word Problems?
A word problem is any math problem based mostly or entirely on a written description. You will not be provided with an equation, diagram, or graph on a word problem and must instead use your reading skills to translate the words of the question into a workable math problem. Once you do this, you can then solve it.
You will be given word problems on the SAT Math section for a variety of reasons. For one, word problems test your reading comprehension and your ability to visualize information.
Secondly, these types of questions allow test makers to ask questions that’d be impossible to ask with just a diagram or an equation. For instance, if a math question asks you to fit as many small objects into a larger one as is possible, it’d be difficult to demonstrate and ask this with only a diagram.
Translating Math Word Problems Into Equations or Drawings
In order to translate your SAT word problems into actionable math equations you can solve, you’ll need to understand and know how to utilize some key math terms. Whenever you see these words, you can translate them into the proper mathematical action.
For instance, the word «sum» means the value when two or more items are added together. So if you need to find the sum of a and b, you’ll need to set up your equation like this: a+b.
Also, note that many mathematical actions have more than one term attached, which can be used interchangeably.
Here is a chart with all the key terms and symbols you should know for SAT Math word problems:
| Key Terms | Mathematical Action |
| Sum, increased by, added to, more than, total of | + |
| Difference, decreased by, less than, subtracted from | − |
| Product, times, __ times as much, __ times as many (a number, e.g., “three times as many”) | * or x |
| Divided by, per, __ as many, __ as much (a fraction, e.g., “one-third as much”) | / or ÷ |
| Equals, is, are, equivalent | = |
| Is less than | < |
| Is greater than | > |
| Is less than or equal to | ≤ |
| Is greater than or equal to | ≥ |
Now, let’s look at these math terms in action using a few official examples:
We can solve this problem by translating the information we’re given into algebra. We know the individual price of each salad and drink, and the total revenue made from selling 209 salads and drinks combined. So let’s write this out in algebraic form.
We’ll say that the number of salads sold = S, and the number of drinks sold = D. The problem tells us that 209 salads and drinks have been sold, which we can think of as this:
S + D = 209
Finally, we’ve been told that a certain number of S and D have been sold and have brought in a total revenue of 836 dollars and 50 cents. We don’t know the exact numbers of S and D, but we do know how much each unit costs. Therefore, we can write this equation:
6.50S + 2D = 836.5
We now have two equations with the same variables (S and D). Since we want to know how many salads were sold, we’ll need to solve for D so that we can use this information to solve for S. The first equation tells us what S and D equal when added together, but we can rearrange this to tell us what just D equals in terms of S:
S + D = 209
Now, just subtract S from both sides to get what D equals:
D = 209 − S
Finally, plug this expression in for D into our other equation, and then solve for S:
6.50S + 2(209 − S) = 836.5
6.50S + 418 − 2S = 836.5
6.50S − 2S = 418.5
4.5S = 418.5
S = 93
The correct answer choice is (B) 93.
This word problem asks us to solve for one possible solution (it asks for «a possible amount»), so we know right away that there will be multiple correct answers.
Wyatt can husk at least 12 dozen ears of corn and at most 18 dozen ears of corn per hour. If he husks 72 dozen at a rate of 12 dozen an hour, this is equal to 72 / 12 = 6 hours. You could therefore write 6 as your final answer.
If Wyatt husks 72 dozen at a rate of 18 dozen an hour (the highest rate possible he can do), this comes out to 72 / 18 = 4 hours. You could write 4 as your final answer.
Since the minimum time it takes Wyatt is 4 hours and the maximum time is 6 hours, any number from 4 to 6 would be correct.
Though the hardest SAT word problems might look like Latin to you right now, practice and study will soon have you translating them into workable questions.
Typical SAT Word Problems
Word problems on the SAT can be grouped into three major categories:
- Word problems for which you must simply set up an equation
- Word problems for which you must solve for a specific value
- Word problems for which you must define the meaning of a value or variable
Below, we look at each world problem type and give you examples.
Word Problem Type 1: Setting Up an Equation
This is a fairly uncommon type of SAT word problem, but you’ll generally see it at least once on the Math section. You’ll also most likely see it first on the section.
For these problems, you must use the information you’re given and then set up the equation. No need to solve for the missing variable—this is as far as you need to go.
Almost always, you’ll see this type of question in the first four questions on the SAT Math section, meaning that the College Board consider these questions easy. This is due to the fact that you only have to provide the setup and not the execution.
To solve this problem, we’ll need to know both Armand’s and Tyrone’s situations, so let’s look at them separately:
Armand: Armand sent m text messages each hour for 5 hours, so we can write this as 5m—the number of texts he sent per hour multiplied by the total number of hours he texted.
Tyrone: Tyrone sent p text messages each hour for 4 hours, so we can write this as 4p—the number of texts he sent per hour multiplied by the total number of hours he texted.
We now know that Armand’s situation can be written algebraically as 5m, and Tyrone’s can be written as 4p. Since we’re being asked for the expression that represents the total number of texts sent by Armand and Tyrone, we must add together the two expressions:
5m + 4p
The correct answer is choice (C) 5m + 4p
Word Problem Type 2: Solving for a Missing Value
The vast majority of SAT Math word problem questions will fall into this category. For these questions, you must both set up your equation and solve for a specific piece of information.
Most (though not all) word problem questions of this type will be scenarios or stories covering all sorts of SAT Math topics, such as averages, single-variable equations, and ratios. You almost always must have a solid understanding of the math topic in question in order to solve the word problem on the topic.
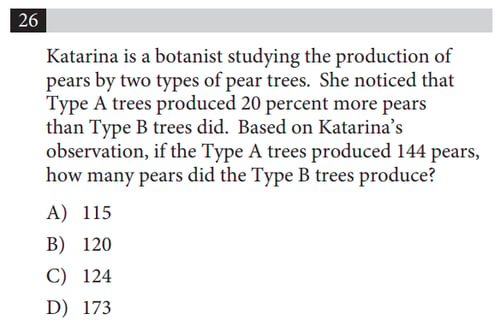
Let’s try to think about this problem in terms of x. If Type A trees produced 20% more pears than Type B did, we can write this as an expression:
x + 0.2x = 1.2x = # of pears produced by Type A
In this equation, x is the number of pears produced by Type B trees. If we add 20% of x (0.2x) to x, we get the number of pears produced by Type A trees.
The problem tells us that Type A trees produced a total of 144 pears. Since we know that 1.2x is equal to the number of pears produced by Type A, we can write the following equation:
1.2x = 144
Now, all we have to do is divide both sides by 1.2 to find the number of pears produced by Type B trees:
x = 144 / 1.2
x = 120
The correct answer choice is (B) 120.
You might also get a geometry problem as a word problem, which might or might not be set up with a scenario, too. Geometry questions will be presented as word problems typically because the test makers felt the problem would be too easy to solve had you been given a diagram, or because the problem would be impossible to show with a diagram. (Note that geometry makes up a very small percentage of SAT Math.)
This is a case of a problem that is difficult to show visually, since x is not a set degree value but rather a value greater than 55; thus, it must be presented as a word problem.
Since we know that x must be an integer degree value greater than 55, let us assign it a value. In this case, let us call x 56°. (Why 56? There are other values x could be, but 56 is guaranteed to work since it’s the smallest integer larger than 55. Basically, it’s a safe bet!)
Now, because x = 56, the next angle in the triangle—2x—must measure the following:
56*2 = 112
Let’s make a rough (not to scale) sketch of what we know so far:
Now, we know that there are 180° in a triangle, so we can find the value of y by saying this:
y = 180 − 112 − 56
y = 12
One possible value for y is 12. (Other possible values are 3, 6, and 9.)
Word Problem Type 3: Explaining the Meaning of a Variable or Value
This type of problem will show up at least once. It asks you to define part of an equation provided by the word problem—generally the meaning of a specific variable or number.
This question might sound tricky at first, but it’s actually quite simple.
We know that P is the number of phones Kathy has left to fix, and d is the number of days she has worked in a week. If she’s worked 0 days (i.e., if we plug 0 into the equation), here’s what we get:
P = 108 − 23(0)
P = 108
This means that, without working any days of the week, Kathy has 108 phones to repair. The correct answer choice, therefore, is (B) Kathy starts each week with 108 phones to fix.
To help juggle all the various SAT word problems, let’s look at the math strategies and tips at our disposal.
Ready to go beyond just reading about the SAT? Then you’ll love the free five-day trial for our SAT Complete Prep program. Designed and written by PrepScholar SAT experts, our SAT program customizes to your skill level in over 40 subskills so that you can focus your studying on what will get you the biggest score gains.
Click on the button below to try it out!
SAT Math Strategies for Word Problems
Though you’ll see word problems on the SAT Math section on a variety of math topics, there are still a few techniques you can apply to solve word problems as a whole.
#1: Draw It Out
Whether your problem is a geometry problem or an algebra problem, sometimes making a quick sketch of the scene can help you understand what exactly you’re working with. For instance, let’s look at how a picture can help you solve a word problem about a circle (specifically, a pizza):
If you often have trouble visualizing problems such as these, draw it out. We know that we’re dealing with a circle since our focus is a pizza. We also know that the pizza weighs 3 pounds.
Because we’ll need to solve the weight of each slice in ounces, let’s first convert the total weight of our pizza from pounds into ounces. We’re given the conversion (1 pound = 16 ounces), so all we have to do is multiply our 3-pound pizza by 16 to get our answer:
3 * 16 = 48 ounces (for whole pizza)
Now, let’s draw a picture. First, the pizza is divided in half (not drawn to scale):
We now have two equal-sized pieces. Let’s continue drawing. The problem then says that we divide each half into three equal pieces (again, not drawn to scale):
This gives us a total of six equal-sized pieces. Since we know the total weight of the pizza is 48 ounces, all we have to do is divide by 6 (the number of pieces) to get the weight (in ounces) per piece of pizza:
48 / 6 = 8 ounces per piece
The correct answer choice is (C) 8.
As for geometry problems, remember that you might get a geometry word problem written as a word problem. In this case, make your own drawing of the scene. Even a rough sketch can help you visualize the math problem and keep all your information in order.
#2: Memorize Key Terms
If you’re not used to translating English words and descriptions into mathematical equations, then SAT word problems might be difficult to wrap your head around at first. Look at the chart we gave you above so you can learn how to translate keywords into their math equivalents. This way, you can understand exactly what a problem is asking you to find and how you’re supposed to find it.
There are free SAT Math questions available online, so memorize your terms and then practice on realistic SAT word problems to make sure you’ve got your definitions down and can apply them to the actual test.
#3: Underline and/or Write Out Important Information
The key to solving a word problem is to bring together all the key pieces of given information and put them in the right places. Make sure you write out all these givens on the diagram you’ve drawn (if the problem calls for a diagram) so that all your moving pieces are in order.
One of the best ways to keep all your pieces straight is to underline your key information in the problem, and then write them out yourself before you set up your equation. So take a moment to perform this step before you zero in on solving the question.
#4: Pay Close Attention to What’s Being Asked
It can be infuriating to find yourself solving for the wrong variable or writing in your given values in the wrong places. And yet this is entirely too easy to do when working with math word problems.
Make sure you pay strict attention to exactly what you’re meant to be solving for and exactly what pieces of information go where. Are you looking for the area or the perimeter? The value of x, 2x, or y?
It’s always better to double-check what you’re supposed to find before you start than to realize two minutes down the line that you have to begin solving the problem all over again.
#5: Brush Up on Any Specific Math Topic You Feel Weak In
You’re likely to see both a diagram/equation problem and a word problem for almost every SAT Math topic on the test. This is why there are so many different types of word problems and why you’ll need to know the ins and outs of every SAT Math topic in order to be able to solve a word problem about it.
For example, if you don’t know how to find an average given a set of numbers, you certainly won’t know how to solve a word problem that deals with averages!
Understand that solving an SAT Math word problem is a two-step process: it requires you to both understand how word problems work and to understand the math topic in question. If you have any areas of mathematical weakness, now’s a good time to brush up on them—or else SAT word problems might be trickier than you were expecting!
All set? Let’s go!
Test Your SAT Math Word Problem Knowledge
Finally, it’s time to test your word problem know-how against real SAT Math problems:
Word Problems
1. No Calculator
2. Calculator OK
3. Calculator OK
4. Calculator OK
Answers: C, B, A, 1160
Answer Explanations
1. For this problem, we have to use the information we’re given to set up an equation.
We know that Ken spent x dollars, and Paul spent 1 dollar more than Ken did. Therefore, we can write the following equation for Paul:
x + 1
Ken and Paul split the bill evenly. This means that we’ll have to solve for the total amount of both their sandwiches and then divide it by 2. Since Ken’s sandwich cost x dollars and Paul’s cost x + 1, here’s what our equation looks like when we combine the two expressions:
x + x + 1
2x + 1
Now, we can divide this expression by 2 to get the price each person paid:
(2x + 1) / 2
x + 0.5
But we’re not finished yet. We know that both Ken and Paul also paid a 20% tip on their bills. As a result, we have to multiply the total cost of one bill by 0.2, and then add this tip to the bill. Algebraically, this looks like this:
(x + 0.5) + 0.2(x + 0.5)
x + 0.5 + 0.2x + 0.1
1.2x + 0.6
The correct answer choice is (C) 1.2x + 0.6
2. You’ll have to be familiar with statistics in order to understand what this question is asking.
Since Nick surveyed a random sample of his freshman class, we can say that this sample will accurately reflect the opinion (and thus the same percentages) as the entire freshman class.
Of the 90 freshmen sampled, 25.6% said that they wanted the Fall Festival held in October. All we have to do now is find this percentage of the entire freshmen class (which consists of 225 students) to determine how many total freshmen would prefer an October festival:
225 * 0.256 = 57.6
Since the question is asking «about how many students»—and since we obviously can’t have a fraction of a person!—we’ll have to round this number to the nearest answer choice available, which is 60, or answer choice (B).
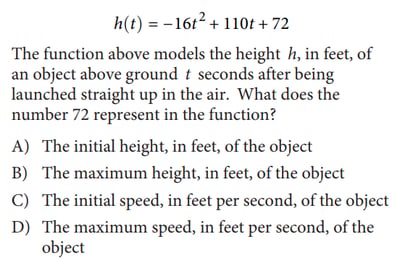
3. This is one of those problems that is asking you to define a value in the equation given. It might look confusing, but don’t be scared—it’s actually not as difficult as it appears!
First off, we know that t represents the number of seconds passed after an object is launched upward. But what if no time has passed yet? This would mean that t = 0. Here’s what happens to the equation when we plug in 0 for t:
h(0) = -16(0)2 + 110(0) + 72
h(0) = 0 + 0 + 72
h(0) = 72
As we can see, before the object is even launched, it has a height of 72 feet. This means that 72 must represent the initial height, in feet, of the object, or answer choice (A).
4. You might be tempted to draw a diagram for this problem since it’s talking about a pool (rectangle), but it’s actually quicker to just look at the numbers given and work from there.
We know that the pool currently holds 600 gallons of water and that water has been hosed into it at a rate of 8 gallons a minute for a total of 70 minutes.
To find the amount of water in the pool now, we’ll have to first solve for the amount of water added to the pool by hose. We know that 8 gallons were added each minute for 70 minutes, so all we have to do is multiply 8 by 70:
8 * 70 = 560 gallons
This tells us that 560 gallons of water were added to our already-filled, 600-gallon pool. To find the total amount of water, then, we simply add these two numbers together:
560 + 600 = 1160
The correct answer is 1160.
Aaaaaaaaaaand time for a nap.
Key Takeaways: Making Sense of SAT Math Word Problems
Word problems make up a significant portion of the SAT Math section, so it’s a good idea to understand how they work and how to translate the words on the page into a proper expression or equation. But this is still only half the battle.
Though you won’t know how to solve a word problem if you don’t know what a product is or how to draw a right triangle, you also won’t know how to solve a word problem about ratios if you don’t know how ratios work.
Therefore, be sure to learn not only how to approach math word problems as a whole, but also how to narrow your focus on any SAT Math topics you need help with. You can find links to all of our SAT Math topic guides here to help you in your studies.
What’s Next?
Want to brush up on SAT Math topics? Check out our individual math guides to get an overview of each and every topic on SAT Math. From polygons and slopes to probabilities and sequences, we’ve got you covered!
Running out of time on the SAT Math section? We have the know-how to help you beat the clock and maximize your score.
Been procrastinating on your SAT studying? Learn how you can overcome your desire to procrastinate and make a well-balanced prep plan.
Trying to get a perfect SAT score? Take a look at our guide to getting a perfect 800 on SAT Math, written by a perfect scorer.
Want to improve your SAT score by 160 points?
Check out our best-in-class online SAT prep program. We guarantee your money back if you don’t improve your SAT score by 160 points or more.
Our program is entirely online, and it customizes what you study to your strengths and weaknesses. If you liked this Math strategy guide, you’ll love our program. Along with more detailed lessons, you’ll get thousands of practice problems organized by individual skills so you learn most effectively. We’ll also give you a step-by-step program to follow so you’ll never be confused about what to study next.
Check out our 5-day free trial:
Have friends who also need help with test prep? Share this article!
About the Author
Courtney scored in the 99th percentile on the SAT in high school and went on to graduate from Stanford University with a degree in Cultural and Social Anthropology. She is passionate about bringing education and the tools to succeed to students from all backgrounds and walks of life, as she believes open education is one of the great societal equalizers. She has years of tutoring experience and writes creative works in her free time.
Get ready to practice word problems with your elementary math class! In our February blog post, we’re featuring a whole-class activity you can do with your students to practice solving word problems together. This activity’s a great way to get your entire class involved in math practice. Read on to find out more.
Assemble and Solve Word Problems as a Class
Looking for a way to build your students’ confidence and proficiency with basic word problems? Practice word problems together as a class! You can create word problems to practice a variety of foundational math skills, including the four operations. Use word problems to work on a specific skill, such as addition. Or help students learn to identify which operation they need to use to solve the word problem. Practice solving single-step word problems, multiple-step word problems, or a mix of both. The following activity is ideal for first grade, second grade, third grade, fourth grade, or fifth grade students.
Create a Word Problem Together…

This easy word problem activity is comprised of two parts. First, you and your class will create a word problem together. Then, your students will solve it.
First, decide what kind of word problems your class will practice:
- Addition Word Problems
- Subtraction Word Problems
- Multiplication Word Problems
- Division Word Problems
- Choose the Operation Word Problems
- Single-Step Word Problems
- Multiple-Step Word Problems
Next, write or print out a variety of objects on little slips of paper. Add the slips of paper to a container, like a shoebox, plastic tub, or small jar that can be passed around the classroom. Here are some ideas:
- Puppies
- Kittens
- Pencils
- Pennies
- Tickets
- People
- Books
- Flowers
- One Dollar Bills
- Shirts

If you wish, you can include a short descriptive phrase with each item written on the slips of paper to help create context for the word problem students will make:
- Puppies (at an animal shelter)
- Kittens (at a farm)
- Pencils (in the classroom supply closet)
- Pennies (in your piggy bank)
- Tickets (sold at the box office)
- People (in line for a ride at the theme park)
- Books (on a bookshelf)
- Flowers (in Grandma’s garden)
- One Dollar Bills (in your wallet)
- Shirts (at the dry cleaner’s)
Then, write or print out a variety of numbers on little slips of paper. Add these slips of paper to a separate container that can be passed around the classroom. You could use consecutive numbers, like the numbers 1 to 50, for example.

Here’s how to create word problems as a class:
Give the «Word Problem Items» jar to a student at one end of the classroom and give the «Word Problem Numbers» jar to a student at the opposite end of the classroom. The student with the «Word Problem Items» jar gets to be the subject of the first word problem. He or she will pick an item at random from the jar and read it out loud to the class. The student with the «Word Problem Numbers» jar will pick two numbers at random and read them out loud to the class.

You can write the first student’s name, the item name, and the two numbers on your whiteboard for the whole class to view.
For example, Rosie is the student with the «Word Problem Items» jar. Rosie’s name will be the subject of the first word problem. Rosie picks an item at random from her jar: «Puppies (at an animal shelter)».
Aaron is the student with the «Word Problem Numbers» jar. He picks two numbers at random from his jar. He reads the numbers out loud to the class: «16» and «11».
Now you and your students have the information you need to create a word problem.
Ask your students for ideas about how to put the information together in a word problem. For example, «What happens to puppies at an animal shelter?» Students can offer suggestions: «They get adopted!» «Families come to meet them and take them home,» etc.
Guide your students through the assembly of all the important pieces of information into a word problem: «Rosie and her family went to see the puppies at the animal shelter on Saturday morning. There were 16 puppies available for adoption. When they came back later in the afternoon, there were 11 puppies left. How many puppies were adopted on Saturday?»

… And Solve!
Once you create a word problem together as a class, work together to solve it. Ask your students what operation is needed to solve the word problem. Then write the problem on the board. You can invite students at random to come to the whiteboard to solve the problem.
After solving the word problem, the students with the jars will pass them to the student sitting next to, or behind them. Continue to create and solve word problems together until all students have had a chance to pick at least one of the word problem components from a jar. Consider switching up the word problem skills you practice throughout the activity for variety, too.
How do you like to practice word problems with your class? We’d love to hear from you! Share your ideas with our teaching community in the comment section below.
Looking for more fun, engaging word problem activities? Visit iKnowIt.com for lots of interactive word problem games!
Comments
Leave a comment
Thank you! Your comment has been submitted.
Lesson 9: Introduction to Word Problems
/en/algebra-topics/solving-equations/content/
What are word problems?
A word problem is a math problem written out as a short story or scenario. Basically, it describes a realistic problem and asks you to imagine how you would solve it using math. If you’ve ever taken a math class, you’ve probably solved a word problem. For instance, does this sound familiar?
Johnny has 12 apples. If he gives four to Susie, how many will he have left?
You could solve this problem by looking at the numbers and figuring out what the problem is asking you to do. In this case, you’re supposed to find out how many apples Johnny has left at the end of the problem. By reading the problem, you know Johnny starts out with 12 apples. By the end, he has 4 less because he gave them away. You could write this as:
12 — 4
12 — 4 = 8, so you know Johnny has 8 apples left.
Word problems in algebra
If you were able to solve this problem, you should also be able to solve algebra word problems. Yes, they involve more complicated math, but they use the same basic problem-solving skills as simpler word problems.
You can tackle any word problem by following these five steps:
- Read through the problem carefully, and figure out what it’s about.
- Represent unknown numbers with variables.
- Translate the rest of the problem into a mathematical expression.
- Solve the problem.
- Check your work.
We’ll work through an algebra word problem using these steps. Here’s a typical problem:
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took two days, and the van cost $360. How many miles did she drive?
It might seem complicated at first glance, but we already have all of the information we need to solve it. Let’s go through it step by step.
Step 1: Read through the problem carefully.
With any problem, start by reading through the problem. As you’re reading, consider:
- What question is the problem asking?
- What information do you already have?
Let’s take a look at our problem again. What question is the problem asking? In other words, what are you trying to find out?
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
There’s only one question here. We’re trying to find out how many miles Jada drove. Now we need to locate any information that will help us answer this question.
There are a few important things we know that will help us figure out the total mileage Jada drove:
- The van cost $30 per day.
- In addition to paying a daily charge, Jada paid $0.50 per mile.
- Jada had the van for 2 days.
- The total cost was $360.
Step 2: Represent unknown numbers with variables.
In algebra, you represent unknown numbers with letters called variables. (To learn more about variables, see our lesson on reading algebraic expressions.) You can use a variable in the place of any amount you don’t know. Looking at our problem, do you see a quantity we should represent with a variable? It’s often the number we’re trying to find out.
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
Since we’re trying to find the total number of miles Jada drove, we’ll represent that amount with a variable—at least until we know it. We’ll use the variable m for miles. Of course, we could use any variable, but m should be easy to remember.
Step 3: Translate the rest of the problem.
Let’s take another look at the problem, with the facts we’ll use to solve it highlighted.
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
We know the total cost of the van, and we know that it includes a fee for the number of days, plus another fee for the number of miles. It’s $30 per day, and $0.50 per mile. A simpler way to say this would be:
$30 per day plus $0.50 per mile is $360.
If you look at this sentence and the original problem, you can see that they basically say the same thing: It cost Jada $30 per day and $0.50 per mile, and her total cost was $360. The shorter version will be easier to translate into a mathematical expression.
Let’s start by translating $30 per day. To calculate the cost of something that costs a certain amount per day, you’d multiply the per-day cost by the number of days—in other words, 30 per day could be written as 30 ⋅days, or 30 times the number of days. (Not sure why you’d translate it this way? Check out our lesson on writing algebraic expressions.)
$30 per day and $.50 per mile is $360
$30 ⋅ day + $.50 ⋅ mile = $360
As you can see, there were a few other words we could translate into operators, so and $.50 became + $.50, $.50 per mile became $.50 ⋅ mile, and is became =.
Next, we’ll add in the numbers and variables we already know. We already know the number of days Jada drove, 2, so we can replace that. We’ve also already said we’ll use m to represent the number of miles, so we can replace that too. We should also take the dollar signs off of the money amounts to make them consistent with the other numbers.
$30 ⋅ day + $.50 ⋅ mile = $360
30 ⋅ 2 + .5 ⋅ m = 360
Now we have our expression. All that’s left to do is solve it.
Step 4: Solve the problem.
This problem will take a few steps to solve. (If you’re not sure how to do the math in this section, you might want to review our lesson on simplifying expressions.) First, let’s simplify the expression as much as possible. We can multiply 30 and 2, so let’s go ahead and do that. We can also write .5 ⋅ m as 0.5m.
30 ⋅ 2 + .5 ⋅ m = 360
60 + .5m = 360
Next, we need to do what we can to get the m alone on the left side of the equals sign. Once we do that, we’ll know what m is equal to—in other words, it will let us know the number of miles in our word problem.
We can start by getting rid of the 60 on the left side by subtracting it from both sides.
The only thing left to get rid of is .5. Since it’s being multiplied with m, we’ll do the reverse and divide both sides of the equation with it.
.5m / .5 is m and 300 / 0.50 is 600, so m = 600. In other words, the answer to our problem is 600—we now know Jada drove 600 miles.
Step 5: Check the problem.
To make sure we solved the problem correctly, we should check our work. To do this, we can use the answer we just got—600—and calculate backward to find another of the quantities in our problem. In other words, if our answer for Jada’s distance is correct, we should be able to use it to work backward and find another value, like the total cost. Let’s take another look at the problem.
The rate to rent a small moving van is $30 per day, plus $0.50 per mile. Jada rented a van to drive to her new home. It took 2 days, and the van cost $360. How many miles did she drive?
According to the problem, the van costs $30 per day and $0.50 per mile. If Jada really did drive 600 miles in 2 days, she could calculate the cost like this:
$30 per day and $0.50 per mile
30 ⋅ day + .5 ⋅ mile
30 ⋅ 2 + .5 ⋅ 600
60 + 300
360
According to our math, the van would cost $360, which is exactly what the problem says. This means our solution was correct. We’re done!
While some word problems will be more complicated than others, you can use these basic steps to approach any word problem. On the next page, you can try it for yourself.
Practice!
Let’s practice with a couple more problems. You can solve these problems the same way we solved the first one—just follow the problem-solving steps we covered earlier. For your reference, these steps are:
- Read through the problem carefully, and figure out what it’s about.
- Represent unknown numbers with variables.
- Translate the rest of the problem into a mathematical expression.
- Solve the problem.
- Check your work.
If you get stuck, you might want to review the problem on page 1. You can also take a look at our lesson on writing algebraic expressions for some tips on translating written words into math.
Problem 1
Try completing this problem on your own. When you’re done, move on to the next page to check your answer and see an explanation of the steps.
A single ticket to the fair costs $8. A family pass costs $25 more than half of that. How much does a family pass cost?
Problem 2
Here’s another problem to do on your own. As with the last problem, you can find the answer and explanation to this one on the next page.
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
Problem 1 Answer
Here’s Problem 1:
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
Answer: $29
Let’s solve this problem step by step. We’ll solve it the same way we solved the problem on page 1.
Step 1: Read through the problem carefully
The first in solving any word problem is to find out what question the problem is asking you to solve and identify the information that will help you solve it. Let’s look at the problem again. The question is right there in plain sight:
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
So is the information we’ll need to answer the question:
- A single ticket costs $8.
- The family pass costs $25 more than half the price of the single ticket.
Step 2: Represent the unknown numbers with variables
The unknown number in this problem is the cost of the family pass. We’ll represent it with the variable f.
Step 3: Translate the rest of the problem
Let’s look at the problem again. This time, the important facts are highlighted.
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
In other words, we could say that the cost of a family pass equals half of $8, plus $25. To turn this into a problem we can solve, we’ll have to translate it into math. Here’s how:
- First, replace the cost of a family pass with our variable f.
- Next, take out the dollar signs and replace words like plus and equals with operators.
- Finally, translate the rest of the problem. Half of can be written as 1/2 times, or 1/2 ⋅ :
f equals half of $8 plus $25
f = half of 8 + 25
f = 1/2 ⋅ 8 + 25
Step 4: Solve the problem
Now all we have to do is solve our problem. Like with any problem, we can solve this one by following the order of operations.
- f is already alone on the left side of the equation, so all we have to do is calculate the right side.
- First, multiply 1/2 by 8. 1/2 ⋅ 8 is 4.
- Next, add 4 and 25. 4 + 25 equals 29 .
f = 1/2 ⋅ 8 + 25
f = 4 + 25
f = 29
That’s it! f is equal to 29. In other words, the cost of a family pass is $29.
Step 5: Check your work
Finally, let’s check our work by working backward from our answer. In this case, we should be able to correctly calculate the cost of a single ticket by using the cost we calculated for the family pass. Let’s look at the original problem again.
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
We calculated that a family pass costs $29. Our problem says the pass costs $25 more than half the cost of a single ticket. In other words, half the cost of a single ticket will be $25 less than $29.
- We could translate this into this equation, with s standing for the cost of a single ticket.
- Let’s work on the right side first. 29 — 25 is 4.
- To find the value of s, we have to get it alone on the left side of the equation. This means getting rid of 1/2. To do this, we’ll multiply each side by the inverse of 1/2: 2.
1/2s = 29 — 25
1/2s = 4
s = 8
According to our math, s = 8. In other words, if the family pass costs $29, the single ticket will cost $8. Looking at our original problem, that’s correct!
A single ticket to the fair costs $8. A family pass costs $25 more than half that. How much does a family pass cost?
So now we’re sure about the answer to our problem: The cost of a family pass is $29.
Problem 2 Answer
Here’s Problem 2:
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
Answer: $70
Let’s go through this problem one step at a time.
Step 1: Read through the problem carefully
Start by asking what question the problem is asking you to solve and identifying the information that will help you solve it. What’s the question here?
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
To solve the problem, you’ll have to find out how much money Mo gave to charity. All the important information you need is in the problem:
- The amount Flor donated is three times as much the amount Mo donated
- Flor and Mo’s donations add up to $280 total
Step 2: Represent the unknown numbers with variables
The unknown number we’re trying to identify in this problem is Mo’s donation. We’ll represent it with the variable m.
Step 3: Translate the rest of the problem
Here’s the problem again. This time, the important facts are highlighted.
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
The important facts of the problem could also be expressed this way:
Mo’s donation plus Flor’s donation equals $280
Because we know that Flor’s donation is three times as much as Mo’s donation, we could go even further and say:
Mo’s donation plus three times Mo’s donation equals $280
We can translate this into a math problem in only a few steps. Here’s how:
- Because we’ve already said we’ll represent the amount of Mo’s donation with the variable m, let’s start by replacing Mo’s donation with m.
- Next, we can put in mathematical operators in place of certain words. We’ll also take out the dollar sign.
- Finally, let’s write three times mathematically. Three times m can also be written as 3 ⋅ m, or just 3m.
m plus three times m equals $280
m + three times m = 280
m + 3m = 280
Step 4: Solve the problem
It will only take a few steps to solve this problem.
- To get the correct answer, we’ll have to get m alone on one side of the equation.
- To start, let’s add m and 3m. That’s 4m.
- We can get rid of the 4 next to the m by dividing both sides by 4. 4m / 4 is m, and 280 / 4 is 70.
m + 3m = 280
4m = 280
m = 70.
We’ve got our answer: m = 70. In other words, Mo donated $70.
Step 5: Check your work
The answer to our problem is $70, but we should check just to be sure. Let’s look at our problem again.
Flor and Mo both donated money to the same charity. Flor gave three times as much as Mo. Between the two of them, they donated $280. How much money did Mo give?
If our answer is correct, $70 and three times $70 should add up to $280.
- We can write our new equation like this:
- The order of operations calls for us to multiply first. 3 ⋅ 70 is 210.
- The last step is to add 70 and 210. 70 plus 210 equals 280.
70 + 3 ⋅ 70 = 280
70 + 210 = 280
280 = 280
280 is the combined cost of the tickets in our original problem. Our answer is correct: Mo gave $70 to charity.
/en/algebra-topics/distance-word-problems/content/
Word problems are a great way to prepare your students for using math in real life. However, many students find them intimidating.[1]
First, introduce word problems so that your students will understand why they’re used. Next, explain how to use the CUBES approach to solving word problems. You can then model the process for your students and have them practice it on their own.
-
1
Explain the purpose of word problems. Without proper context, some students may view word problems as unnecessarily complicated. However, they’re actually intended to help students use math to solve real world problems, such as building a fence, buying enough food for a party, or adjusting a recipe. The math is still the same, but the way the problem is presented is more relevant to real life.[2]
- Ask students to work with a partner or group to identify ways that they might use math in real life. Next, have them turn that situation into a math-based story.
- For example, they might say they use math when dividing into teams at recess. They could turn that into a story like this: “If the class has 20 students and we need 2 teams to play, how many students will be on each team?”
-
2
Help students create a word problem that fits their life.[3]
Have them follow the process above to create a math story-problem specific to their own life. This helps the lesson feel relevant to students, which gets them engaged. It also helps them reach higher levels of learning.[4]
- Suggest that they incorporate a hobby or favorite interest. For example, they might write a problem that has to do with a sport they play or a problem that centers around a favorite animal.
- Have them put their name in the problem.
- Here’s an example: “If Alex wants to score 10 soccer goals over the second half of the season and there are 5 more games left to play, how many goals should Alex score per game to stay on track?”
-
3
Take out the numbers to help students focus on the question. Students need to know what the problem is asking if they’re going to solve it, but recognizing the question is hard. It’s easy to get hung up on the numbers! Rewrite a basic word problem without the numbers, then ask students to determine what it’s asking.[5]
- You may need to do this several times for students to understand.
- Your sample problem might look like this: “Sarah is buying pizza for her slumber party. If each pizza has a certain number of slices and she knows how many guests are coming, how can she estimate the number of pizzas she needs. What other information might she need to arrive at the answer?” Your students should point out that Sarah would need to know how many pieces of pizza her guests will eat on average. She could then multiple that number of slices by the number of guests. To get the number of pizzas, she’d then divide the total number of slices by the number of slices in a pizza.
-
4
Tell students to illustrate word problems. When students are first working with word problems, illustrating them can help better understand the context. They can draw the action of the problem, the items depicted in the problem, or whatever helps them.[6]
- For example, when determining how many pizzas Sarah will need to buy for her slumber party, the students could draw circles to represent the pizzas and stick figures to represent Sarah and her guests.
- However, stress that this is not a requirement for arriving at your answer, as some students may find this extra step frustrating.
- This can help visual learners better understand word problems.
-
5
Make sure the word problems match students’ language and math skills. Students must be able to understand what the problem is saying in order to know what it’s asking. Similarly, they won’t be able to arrive at the answer unless they know how to do the required math processes.
- For example, students who can only add numbers should be given word problems that only require addition.
-
1
Summarize the CUBES process for students. CUBES provides students with an easy-to-follow process for solving word problems that includes all of the relevant steps. It’s also easy for students to remember, as it offers a fun, math-related acronym. Here is what each letter stands for:[7]
- C-Circle the numbers.
- U-Underline the question.
- B-Box the keywords.
- E-Eliminate unnecessary information.
- S-Show your work.
-
2
Tell students to circle all of the numbers, including those in word form. It’s common for word problems to include numbers in word form, even if some numbers are written numerically. Circling them can make it easier for students to come back to them once they start to work through their solution.[8]
- Although some numbers may be extraneous information, it’s important that students circle all of them during this step. They can eliminate unnecessary information later.
- For example, your problem might look like this: “Katie asked 7 friends to sleepover on Friday, but only 4 can make it. She knows that she and each friend will eat 6 chicken nuggets, and each bag of frozen nuggets contains 15 nuggets. How many bags will she need to make sure she has enough nuggets?” Students should circle the 7, 4, 6, and 15.
-
3
Underline the question within the problem. The question is what the problem is asking the student to do. It’s what they should know once they’ve finished working the problem.[9]
- For example, they might need to know how much of something they’d need or how much of something they’d have left.
- When students are first learning word problems, the question should be at the end of the problem. It will often have a question mark at the end.
- In the problem above, the question is this: «How many bags will she need to make sure she has enough nuggets?”
-
4
Draw boxes around any keywords, such as “total” or “all together.” Keywords signal to the student what they’re expected to do to solve the problem, such as add, subtract, multiply, or divide.[10]
In some cases, synonyms of these words will be used. Students need to know how to identify these keywords, then they can box them in so that they won’t forget to use them.[11]
- For example, addition could be indicated by words like “in total” or “all together.” Similarly, subtraction should be signified by “difference” or “less.”
- In the problem above, students would draw boxes around «only,» which will indicate subtracting. They’ll also draw boxes around «how many» and «enough,» which suggest that they’ll need to first multiply and then divide.
-
5
Show students how to eliminate unnecessary information. Many word problems have unnecessary information that complicates the process. For example, the problem might contain unnecessary numbers or extra information. Students can avoid confusion by crossing out this information before they start working on a solution.[12]
- For example, the sample problem has extra numbers: “Katie asked 7 friends to sleepover on Friday, but only 4 can make it. She knows that she and each friend will eat 6 chicken nuggets, and each bag of frozen nuggets contains 15 nuggets. How many bags will she need to make sure she has enough nuggets?” The students could cross out the 7, since it isn’t necessary for arriving at the correct answer.
-
6
Ask students to show their work. Not only will this ensure they receive full credit, it also helps students check their work. If they get the wrong answer, they can identify where they made errors and correct it moving forward.[13]
- Here’s how we would solve Katie’s slumber party question:
- 4 friends are coming, plus Katie: 4 + 1 = 5 girls
- Each of them will eat 6 nuggets: 5 x 6 = 30 nuggets
- Each bag has 15 nuggets: 30 / 15 = 2 bags needed
- Answer: Katie needs to buy 2 bags of nuggets.
- Here’s how we would solve Katie’s slumber party question:
-
1
Allow students to follow along as you solve word problems. Provide them with a worksheet with the problems printed on it. Then, show them what they need to do, asking them to copy down what you write onto their paper. Go slowly, taking questions as they arise.[14]
- You will need to work through several problems until your students start to grasp the concepts. Make sure the problems you choose match your students’ language and math skills.
- You can work through the problems on the board or using a projector.
- For example, you could solve this problem: «Diego is baking cookies for his team’s bake sale. Last year, he earned $100 to help his team. This year, he wants his sales to reach $120. If each cookie will cost $0.75, how many cookies will Diego need to bake?»
-
2
Talk through your strategy as you solve the problem. Explain each step in detail so that students can understand your thought processes as you approach the problem.[15]
In addition to seeing what you write, they can get an idea of how you arrived there.[16]
- Say, «I’m going to start by circling the numbers.» Circle 100, 120, and 0.75.
- Next, say, «“Now I’m going to underline this, since I know it’s the question.” You’d underline this statement: «If each cookie will cost $0.75, how many cookies will Diego need to bake?»
- Say, “Now I’m going to cross this out, since the problem isn’t asking me about this.» You’d cross out the sentence about Diego earning $100 last year.
-
3
Show your work. This is essential for students to understand how to do it themselves. Don’t do any of the math in your head, even if it’s something really simple. Remember, your students should be copying down everything you write so that they can refer to it in the future.[17]
- Write the steps in order to make it easier for students to copy down.
- Here’s how to work out Diego’s problem:
- Diego needs to make $120 by selling cookies for $0.75: 120 / .75 = 160
- Answer: He will need to bake 160 cookies.
-
4
Point out parts of the problem that could have tripped you up. After you arrive at your answer, help students see where you could have made errors. For example, you might have included unnecessary numbers, or you may have gotten tripped up over extra information.[18]
- Not only does this show students what to watch out for, it also shows them that mistakes can happen to anyone.
- For example, you might have accidentally used the information about Diego earning $100 last year, which isn’t relevant to the question.
-
5
Call on students to identify the next step once they catch on. This gets students to start thinking the process through on their own. At first, pause and ask students to reflect on what they need to do next. Then, call on a student. Ask a different student to supply the next step.[19]
- If a student gets the answer wrong, praise the effort, then ask another student to help. Say, “Great enthusiasm, James! We’re almost to that step, but we need to do something else first. Amy, could you help us out?”
-
1
Allow students to work in groups or pairs while they learn the process. Give the students a few practice problems to solve together. This provides built-in supports for struggling students and helps students solidify their knowledge as they explain their reasoning to their classmates. Move about the room to ensure that they are on track.[20]
- Remind them to use CUBES to solve their problems.
- You can make sure students are on track by listening to what they are saying. Are they correctly explaining the process? Do they appear to be confused? Use your judgement to determine if you need to offer guidance.
- Praise groups that are on track. Say, «Excellent work!» or «I’m proud of the progress you’re making!»
-
2
Ask students to write their own problems, then switch papers. This is a fun way to deepen your students’ understanding of word problems. Students can develop real word story questions that relate to their lives, then they can have fun solving their friends’ stories.[21]
- It’s a good idea to have them do this in groups, as it increases the quality of the questions. Also, it’ll allow you to check each group’s questions before they switch papers, which would be harder if each student created questions.
- Not only is this fun for students, but it encourages higher-level learning.
-
3
Provide students with practice problems to solve individually. Students will be ready for individual practice after they’ve watched you model the process and have worked in groups or pairs. At this point, you can work with individual students who are having difficulty grasping the concept. Help them work through the CUBES process one-on-one until they start to grasp it.
- You can make up your own worksheets, or you can download them for free online.[22]
Make sure that the word problems you give them match their language and math skills. - When making your own worksheets, it’s best to work through the problems before you give them to students to make sure all of the information is present. Also, stick to topics your students will understand, such as sports, pets, and food.
- If a student seems to be struggling, you may want to invite them to tutoring.[23]
- You can make up your own worksheets, or you can download them for free online.[22]
Sample Word Problems and Illustrated CUBES Process
Add New Question
-
Question
How can I help my children solve word problems?
Daron Cam is an Academic Tutor and the Founder of Bay Area Tutors, Inc., a San Francisco Bay Area-based tutoring service that provides tutoring in mathematics, science, and overall academic confidence building. Daron has over eight years of teaching math in classrooms and over nine years of one-on-one tutoring experience. He teaches all levels of math including calculus, pre-algebra, algebra I, geometry, and SAT/ACT math prep. Daron holds a BA from the University of California, Berkeley and a math teaching credential from St. Mary’s College.
Academic Tutor
Expert Answer
Have them look for keywords within the problem that help you figure out the steps you need to take.
-
Question
What does keywords mean in math?
Daron Cam is an Academic Tutor and the Founder of Bay Area Tutors, Inc., a San Francisco Bay Area-based tutoring service that provides tutoring in mathematics, science, and overall academic confidence building. Daron has over eight years of teaching math in classrooms and over nine years of one-on-one tutoring experience. He teaches all levels of math including calculus, pre-algebra, algebra I, geometry, and SAT/ACT math prep. Daron holds a BA from the University of California, Berkeley and a math teaching credential from St. Mary’s College.
Academic Tutor
Expert Answer
Keywords tell you what you need to do to solve a word problem. For instance, «total» and «all together» mean you need to add. «Difference» and «less» mean you need to subtract.
Ask a Question
200 characters left
Include your email address to get a message when this question is answered.
Submit
-
Word problems are difficult for many learners, so have patience.
-
Encourage students to keep trying, even if they don’t get it at first.
-
You can find several different acronyms for solving word problems, but CUBES incorporates all of the steps.
-
Make sure that the practice problems you choose don’t include mathematical concepts that are beyond your students. When in doubt, work through the problem yourself before giving it to your young learners.
References
About this article
Thanks to all authors for creating a page that has been read 8,920 times.
Did this article help you?
Confession: It was my first year teaching and one month before the state test, I realized that I had failed to expose my students to enough word problems for them to be successful and confident with them. I was so caught up in the pacing guide and using the math textbook that I failed to make the math real world and situational for my students. Big mistake on my part and I had to spend the next month doubling down on word problems to make up for it.
Since that first year, I have learned a lot and started introducing word problems of the day as part of my math review or mini-lessons. On this post, I am sharing a free word problem of the day starter pack for you to use the first 20 days of school! This free word problem starter pack for 4th and 5th grade will set you on the right path of regular word problem practice and you won’t make the same mistake I did!
I touched on this a bit already but here are some more benefits of using a word problem of the day routine in your classroom:
- Continually exposes your students to word problems in a variety of contexts and with a variety of operations.
- When you use skill-based sets, you can ensure you only expose your students to word problems with skills they have been taught.
- Regular practice with word problem will lead to greater student success and, more importantly, confidence.
- Word problems will be a normal part of instruction and not seen as difficult or hard by students.
About the Word Problem of the Day Starter Pack
What skills are covered?
There are two versions, labeled Set A and Set B.
Set A has problems for addition and subtraction with 4-digit numbers and basic multiplication and division problems. There are also five multi-step problems. This set is ideal for the beginning of 4th grade (or the beginning of 5th graders who struggle with math).
Set B has problems for addition and subtraction with 6-digit numbers and multi-digit multiplication and division (multiplying by 1-digit only and dividing with 1-digit divisors). There are also five multi-step problems. This set is ideal for the beginning of 5th grade.
About the Format
Each set is available in three formats.
One problem per page format: This format works well for projecting the problems via a smartboard or printing them out to place at a math center.
One problem per page with student workspace: This format works well for giving each student a word problem daily. There is ample space for the students to organize and show their work.
One week per page: This format works well for paper-saving classrooms. All of the word problems for the week are on one page.
Using Review Skills to Begin the Year
I use the first month of school to really set the expectations and model for the students what I expect when they are solving word problems (read more about how we show the love with word problems here).
And it is so important that when teaching expectations and modeling a process, you use review skills that the students are already familiar with and have achieved mastery with. It is incredibly difficulty to try and teach students how you want them to solve a word problem using a math skill that they have not mastered yet. It will frustrate them and take away from the focus.
For that reason, I use these word problem of the day starter packs that contain skills from the previous grade level. This allows students to focus on the routine of solving a word problem daily and the process you want them to take before, during, and after solving a word problem.
Word Problem of the Day Routine
Speaking of the process, here is a brief run-down of how I use word problem of the day:
- I read the word problem and tell the students to focus on what is happening in the word problem.
- I ask for volunteers to reread the word problem and this time we focus on what the word problem is asking us.
- I ask 1-2 volunteers to retell the word problem in their own words.
- As a class, we restate the question into a sentence with a blank for the answer (this really ensures we know what the question is asking us).
- I give the students 1-2 minutes of private think time to think about how they will solve the problem.
- The students then get another 3-5 minutes of independent time to solve the problem. As the students are solving the problem, I monitor their understanding, ask guiding questions, and look for examples to share.
- We share what we think the answer is, how we got the answer, how we know it is correct, and the we plug it into our sentence (from step 4) and see if it makes sense.
The entire process takes about 15 minutes but is so valuable…and it does speed up as the year goes on and you can eliminate some of the steps.
How do I get the FREE word problem of the day starter pack?
Just put your email address in the box below and the starter packs (both Set A and Set B) will be sent to your inbox!
FREE Word Problem of the Day Packs
Join my email list to get the Word Problem of the Day Starter Packs for FREE! If you are already on my email list, you can sign up again for this freebie without getting duplicate emails. 🙂
Where can I buy more word problem of the day packs to continue this?
Once you finish with the starter packs, I have grade level sets available for purchase so you can continue your word problem of the day routine all year. The bundles include three main sets with three sets inside of the main sets for a total of 180 word problems. And the best parts are that the third set of each main set contains multi-step problems AND the sets are skill-based so you can ensure your students are only seeing word problems for skills you have taught. You can also mix and match the sets as you please.